lOMoARcPSD| 36991220 HCMC UNIVERSITY OF FINAL EXAMINATION
TECHNOLOGY AND EDUCATION
SEMESTER 2 – ACADEMIC YEAR 2021-2022
Faculty of International Education
Course name: Linear Algebra and Algebraic Structures Course ID: MATH143001E Exam Code: 1. Number of pages: 2
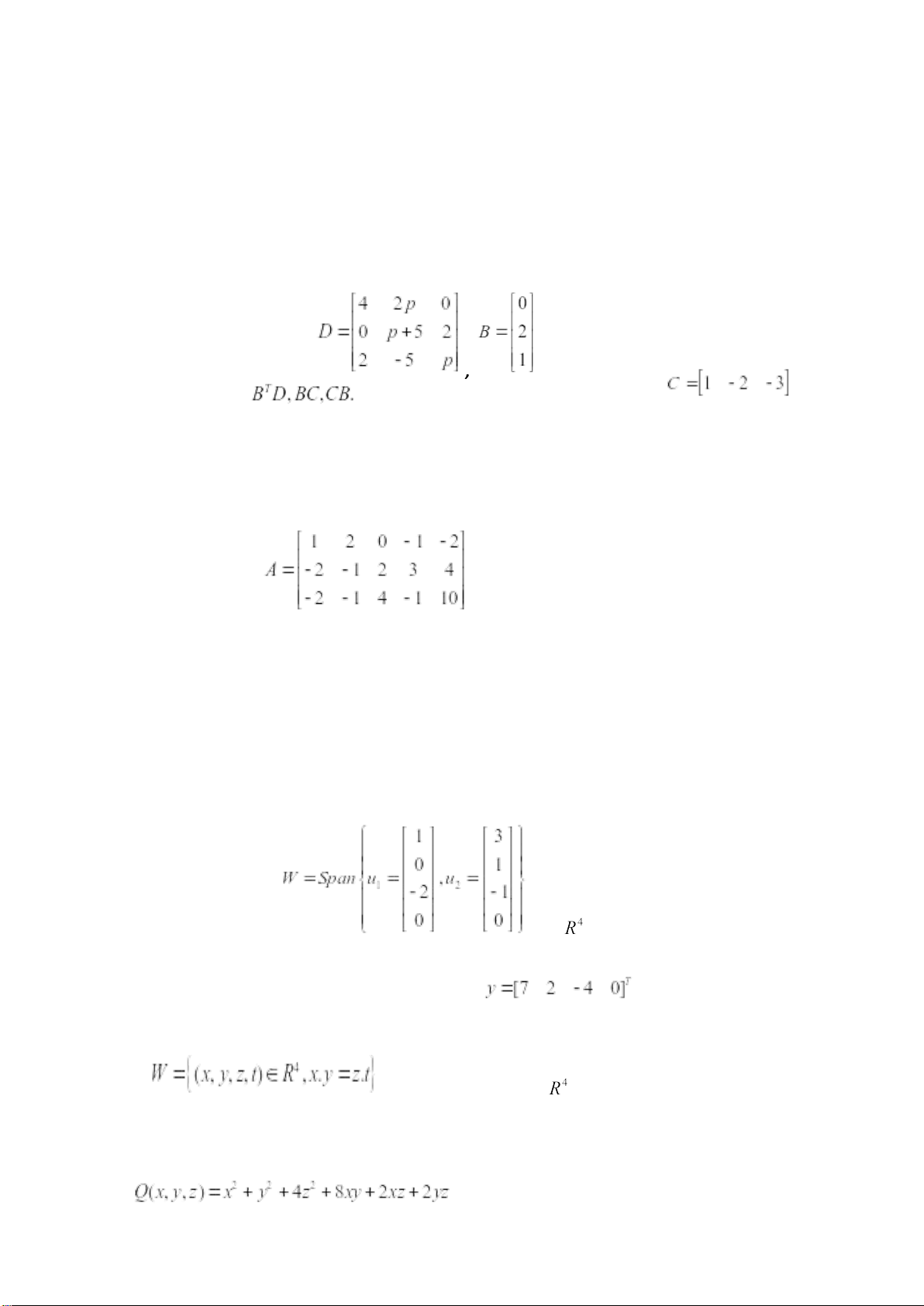
Duration: 90 minutes. Open-book. Question 1: (2pts) Given the matrices , and . a) Calculate
b) Find all values p such that the matrix D is invertible.
Question 2: (2pts) Given the matrix a) Find LU-factorization of A. b)
Find bases and dimensions of Col(A) and Nul(A).
Question 3: (1pt) Given the subspace in .
a) By using Gram-Schimdt process, find an orthogonal basis of W.
b) Find the orthogonal projection of the vector onto W.
Question 4: (1pts) Is a vector subspace of ? Explain why? Question 5: (3pts)
Given the following quadratic form
a) Find the symmetric matrix A that represents the quadratic form Q. lOMoARcPSD| 36991220
Document ID: BM1/QT-PĐBCL-RĐTV-E
b) Assume that a≤b≤c are all the eigenvalues of the matrix A, find a+b+c.
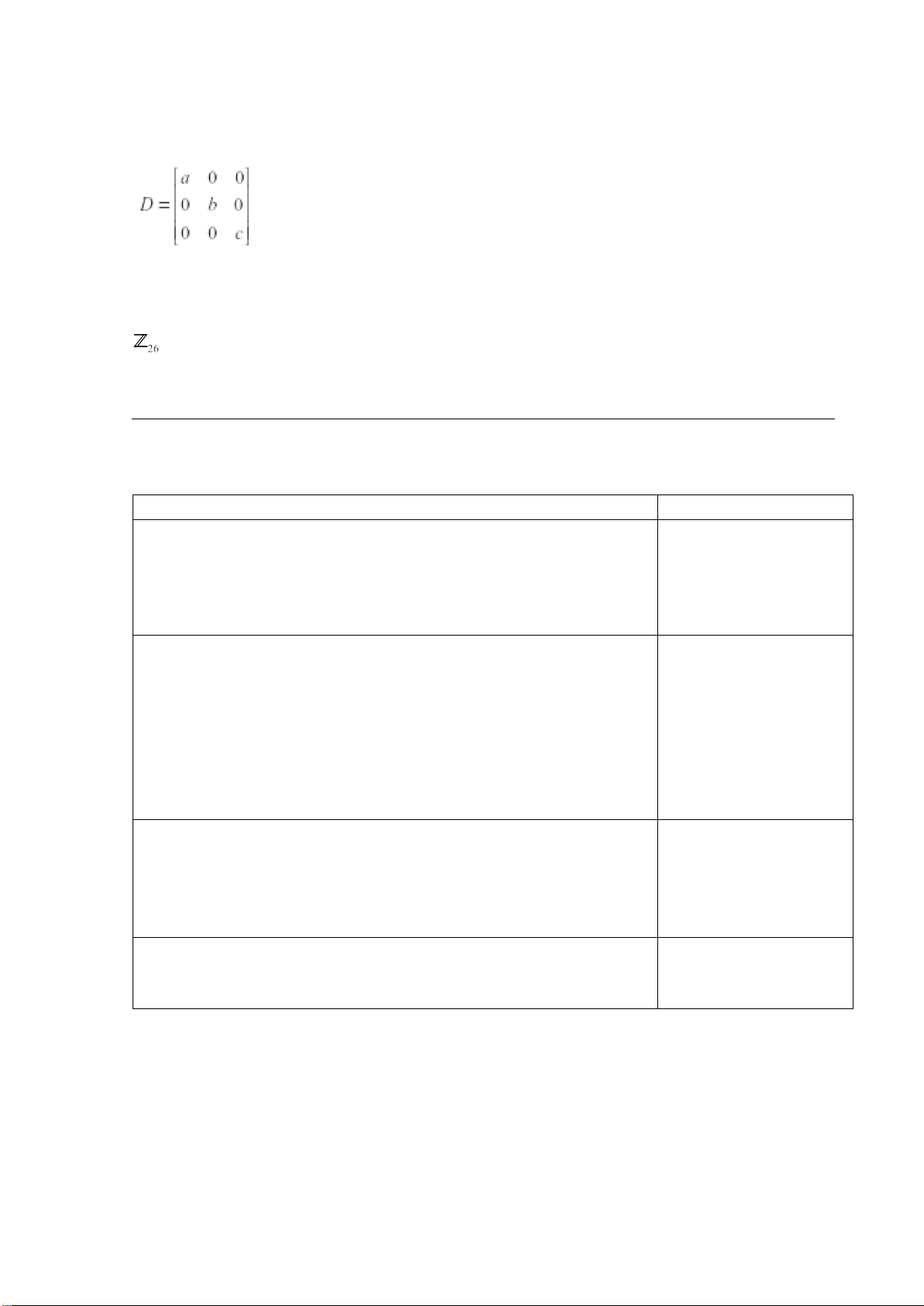
c) Find the matrix P such that A=PDPT , in which D is the diagonal matrix Question 6: (1pts)
Alice encrypts message M by using the encryption function f(x)=5x-6 where x is in the ring
. Then Alice sends the encrypted message to Bob. Bob receives the message “YSSJ”. Find the message M.
Note: Proctors are not allowed to give any unauthorized explanations.
Expected Learning Outcomes Assessed in Questions
[ELO G2.2]: Perform the matrix operations, compute the determinant 1
and the inverse of a square matrix, solve the linear equations, 2
proficiency in using row reduction, apply row reduction to compute
the determinant and the inverse of a matrix, know how to apply to linear models.
[ELO G2.3]: Perform almost problems of vector space: determine a 3
subspace, a combination of a system of vectors, the (in) dependence of 4
a system of vectors; determine bases, dimension of a space, the
coordinate systems of a vector; compute the change-of-coordinates
matrix; calculate the coordinate of a vector relative to a orthogonal
basis or to a orthonormal basis, find the orthogonal projection of a
vector onto a subspace; proficiency in using Gram-Schmidt process.
[ELO G2.4]: Express a linear transformation as a matrix, find the 5
image and the kernel of a transformation; find the eigenvalues and
eigenvectors of a square matrix; (orthogonally) diagonalize a
square matrix; find the sign of a quadratic forms, transform a
quadratic form into one with no cross-product term.
[ELO G2.5]Construct a binary operations; determine whether a set 6
with (an) operation(s) is a group, a ring or a field; use some
algebraic cryptosystems to encrypt or decrypt messages. 23/05/2022
Approved by program chair (signed and named) lOMoARcPSD| 36991220
Document ID: BM1/QT-PĐBCL-RĐTV-E Page: 1/1