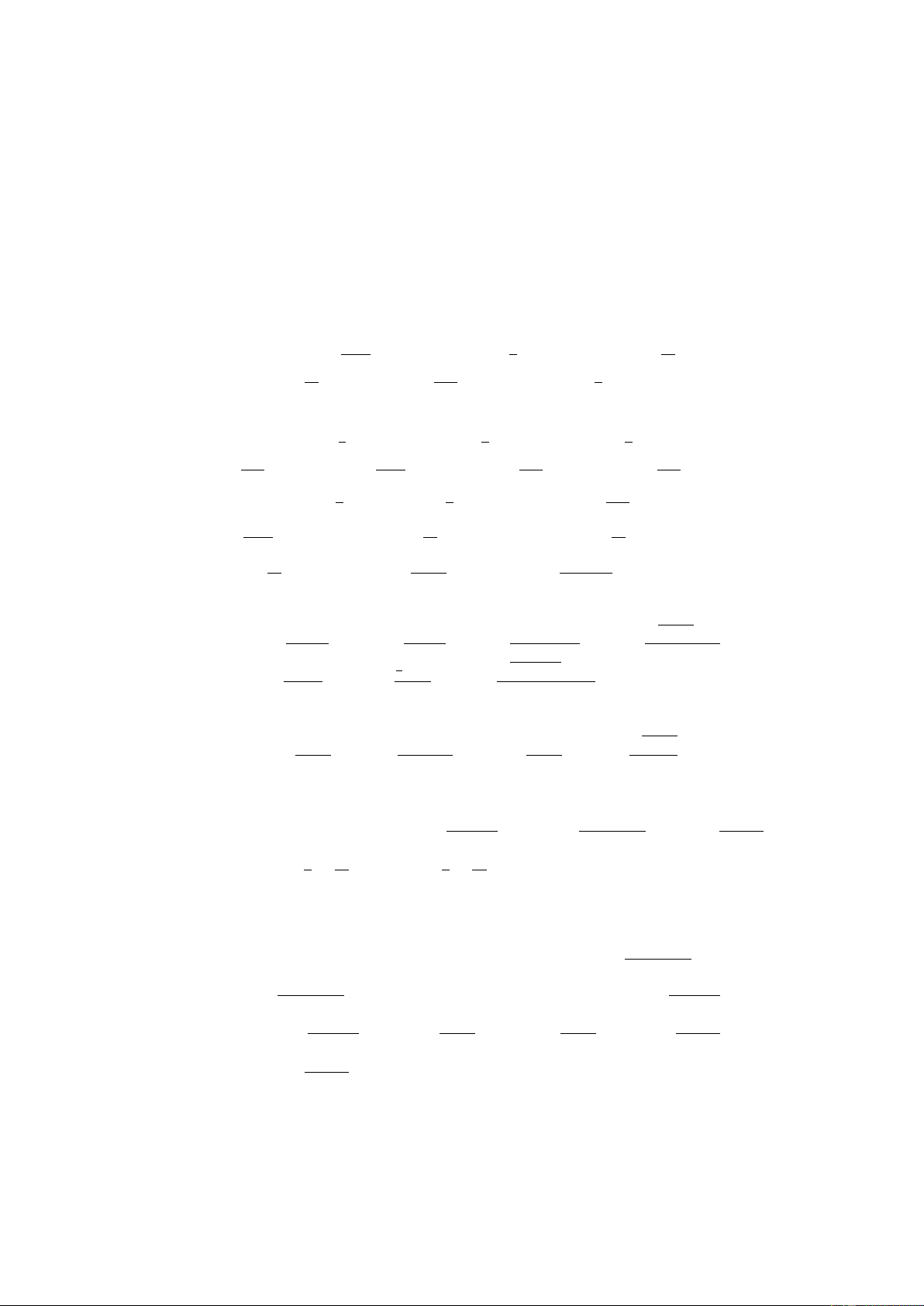

Preview text:
lOMoARcPSD|35973522 MATHEMATICS 1 6.10.2023
? - denotes harder problems for interested students; we won’t solve them
during tutorials, interested students can present their solutions by themselves
RL - problem due to Rafa l Lochowski
1. Compute limits of the following sequences: n n n (a) an = 1 + 2 ; (b) b ; (c?) c ; (d) 100·n n = 1 1 n n = 1 + 1 n2 (n2) ⇣ ⌘3n (n2) d n n = 1 3 ; (e) e ; (f?) f . n2 n = n+2 n = 1 3 n
2. Compute limits of the following sequences: n n n (a) an = 1 1 ; (b) j ; (c) h ; (d) d n n = 1 + 2n n = 1 + 4n n = ⇣ ⌘n ⇣ ⌘n ⇣ ⌘2n+1 n+5 n; (e) e 3n+1 ; (f) f n ; (g) g n−1 ; n n = 3n+4 n = n+1 n = n+3 n ⇣ ⌘3n (h) h −n+3 n = 1 3 ; (i) i ; (j) j 1 + 1 ; (k) k n n 1 4 n n = n+2 n = ⇣ ⌘n2 n2+6 2n+1 n . (l?) l ; (m?) m ; (o?) o n2 n = 1 + 1 2n n = 1 1 n2 n = 2n+1 ⇣ ⌘n2 ⇣ ⌘3n2 +4n−7 1 1 ; (p n2+2 n2+3n+1 ?) p ; (r?) r . n2 n = 2n2+1 n = n2+5n−1
3. (RL) Evaluate the limit if it exists: p x2 9 x2 9 x2 + x 6 x + 6 3 (a) lim ; (b) lim ; (c) lim ; (d) lim ; x→3 x 3 x→−3 x + 3 x→2 x 2 x→3 x 3 p 1 t4 1 + 1 100 + h 10 (e) lim ; (f) lim t ; (g) lim . t→1 1 t3 t→−1 t + 1 h→0 h
4. (RL) Determine the infinite limit: p 2 x 2 x ex x + 6 (a) lim ; (b) lim ; (c) lim ; (d) lim . x→3+ x 3 x→3 (x 3)2 x→5− x 5 x→3 |x 3|
5. (RL) Find the limit if it exists. If the limit does not exists, explain why. 2x + 12 2x 1 2 |x| (a) lim (2x + |x 3|); (b) lim ; (c) lim ; (d) lim ; x→3 x→−6 |x + 6| x→0.5− |2x3 x2| x→−2 2 + x ✓ 1 1 ◆ ✓ 1 1 ◆ (e) lim ; (f) lim . t→0− t |t| t→0+ t |t|
6. (RL) Find the limit (finite or infinite) if it exists. If the limit does not exists, explain why. 2x 1 (a) lim (x + |x 3|); (a’) lim (x + |x 3|); (b) lim ; (b’) x→+∞ x→−∞ x→+∞ |2x3 x2| 2x 1 2x + 12 lim ; (c) lim cos x; (c’) lim cos x; (d) lim ; x→−∞ |2x3 x2| x→−∞ x→+∞ x→−∞ |x + 7| 2x + 12 sin x sin x 2 |x| (d’) lim ; (e) lim ; (e’) lim ; (f) lim ; x→+∞ |x + 7| x→−∞ x + 6 x→+∞ x + 6 x→−∞ 2 + x 2 |x| (f’) lim . x→+∞ 2 + x 1
Downloaded by Di?p ??ng Ng?c (tanphatthpt@gmail.com) lOMoARcPSD|35973522
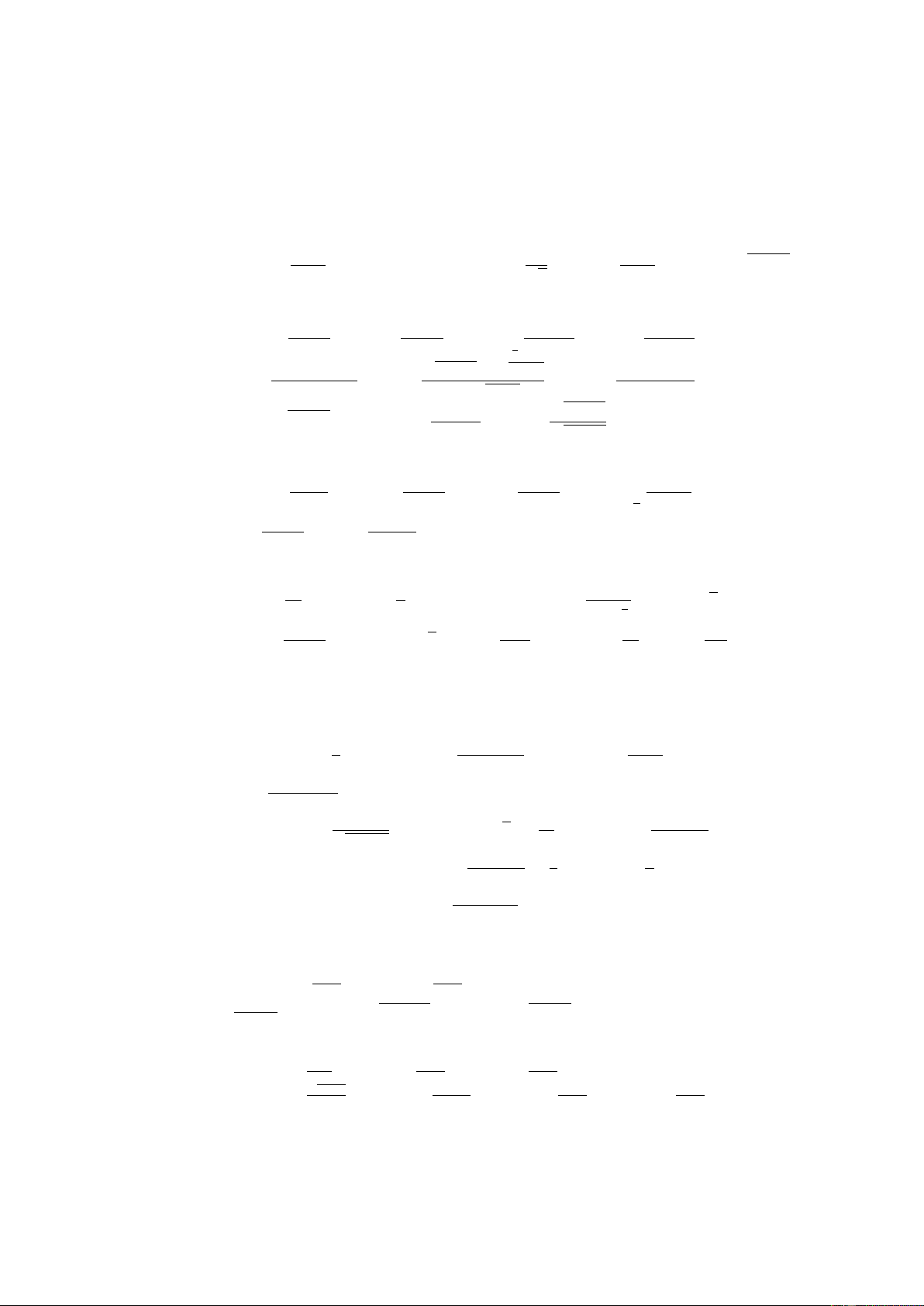
7. Compute the following limits: (a) lim (2x
7); (b) lim (3 + 2x3); (c) lim (1 x2); (d) lim (5 x7); x→4 x→1 x→−∞ x→∞ 2x 1 1 p (e) lim
; (f) lim (3−x + 1); (g) lim p ; (h) lim ; (i) lim x2 9. x→∞ x + 1 x→∞ x→0+ x x→2+ x 2 x→−3−
8. Compute the following limits: x2 + 4 x2 1 4x2 1 27 x3 (a) lim ; (b) lim ; (c) lim ; (d) lim ; (e) x→2 x + 2 x→2 x 2 x 1 →− 2x + 1 x→3 x 3 2 p p 3x2 + 5x 2 x2 + 1 x + 1 x2 5x + 4 lim ; (f) lim p ; (g) lim ; (h) x→−2 4x2 + 9x + 2 x→0 1 x + 1 x→∞ x(x 5) p p 2x + 3x 1 + x2 lim ( x2 + 1 x); (i) lim ; (j) lim p . x 3 →∞ x→∞ 3x + 1 x→∞ 1 x3
9. Compute the following limits: sin 3x tan 5x sin 6x cos x (a) lim ; (b) lim ; (c) lim ; (d*) lim ; (e) x→0 4x x→0 7x x→0 tan 5x x π → ⇡ 2x 2 5x 1 1 e2x lim ; (f) lim . x→0 x x→0 tan x
10. (*) Justify that the following limits do not exist: 1 1 1 p (a) lim
; (b) lim sin ; (c) lim cos (x2); (d) lim ; (e) lim [ x]; 1 x→0 x3 x→0+ x x→∞ x→0 1 + e x x →4 x p 1 1 2[x] (f) lim ; (g) lim sin x; (h) lim ; (i) lim cos ; (j) lim ; x→2 4 x2 x→∞ x→π sin x x→0− x2 x→∞ 2x (k) lim ex(1 + sin x). x→∞
11. (*) Justify the following equalities (try to use the squeeze theorem for functions): ✓ 1 ◆ x2 + sin x [x] (a) lim x sin = 0; (b) lim = 1; (c) lim = 1; (d) x→0 x x→∞ x2 cos x x→∞ x + 1 ln (2x + 1) lim = log3 2; (e) lim (x 2)2[x] = 0; (f) lim (2 sin x x) = x→∞ ln (3x + 1) x→2 x→∞ 1 p 1 2 + sin x 1; (g) lim p = 1; (h) lim x cos = 0; (i) lim = x→0− x2 x x→0 x2 x→∞ x2 [3ex] + 2 3 1
0; (j) lim ex+sin2 x = 0; (k) lim = ; (l) lim x3 = 0; (m) x→−∞ x→∞ [2ex] + 1 2 x→0 x 1 lim (x2 sin x) = 1; (n) lim = 1; (o) lim 2x(2 + cos x) = x→∞ x→0+ 2x sin x x→∞ 1.
12. (RL) Find all asymptotes and sketch the grapf of a function f .
(a) f (x) = x3 ; (b) f (x) = x2+1 ; (c) f (x) = (x + |x 3|); (d*) f (x) = x2+1 x3 |2x2−1| p p ; (e) f (x) = x2 + 4x; (f) f (x) = x2 + 4. |2x−1|
13. Determine asymptotes of the following functions:
(a) a(x) = sin x ; (b) b(x) = x3−1 ; (c) c(x) = 1 ; (d) d(x) = e−x sin x+x; x x−1 1−x2 √ (e) e(x) = 1+x2 ; (f) f(x) = x3 ; (g) g(x) = 1 ; (h) h(x) = 1−x2 . x (x+1)2 ex−1 x+1 2
Downloaded by Di?p ??ng Ng?c (tanphatthpt@gmail.com) lOMoARcPSD|35973522
14. (RL) Explain why the function f is discontinuous at the given number a.
Sketch the graph of the function. ( 1 if x 6= 2, (a) f (x) = 1 , a = 2; (b) f (x) = x+2 a = 2; (c) x+2 1 if x = 2, 8 ( cos x if x < 0, ex if x < 0, > < f (x) = a = 0; (d) f (x) = 0 if x = 0, a = 0 x2 if x 0, > :1 x2 if x > 0,
15. (RL) How would you ”remove the discontinuity of f ”, in other words, how
would you define f (2) in order to make f continuous on R. 8ex−2 if x < 2, √ > < (a) f (x) =
x+2−2 ; (b) f (x) = x3−8 ; (c) f (x) = 2 if x = 2 ; (d) x−2 x4−16 > :x 1 if x > 2 8cos (x 2) if x < 2, > < f (x) = 0 if x = 2, . > :x2 x 1 if x > 2.
16. (RL) For what values of x is f continuous? ( ( 0 if x is rational, 0 if x is rational, (a) f (x) = (b) f (x) = (c*) 2 if x is irrational, x if x is irrational, (p2 if x is rational, f (x) = x if x is irrational.
17. Verify the continuity of the following functions: 8 ( e1/x, if x < 0 x2 2, if x 0 > < (a) a(x) = ; (b) b(x) = ln (x + 1), if x 2 (0, e2 1) ; 2x + 1, if x > 0 > :x2 1, if x e2 1 8cos x + 1, if x 1 > < (c) c(x) = x + 2, if x 2 ( 1, 1) . > :2x−1 + 2, if x 1 3
Downloaded by Di?p ??ng Ng?c (tanphatthpt@gmail.com)