Portfolio- Management - Tài liệu tham khảo | Đại học Hoa Sen

1.2.1 Concept
A portfolio is a form of investment structure that consists of a combination of
many different kinds of assets. The goal of this structure is to maximize investment
returns while simultaneously minimizing investment risks.
Each investor should diversify their holdings over a variety of asset classes and
investment vehicles, including gold, foreign currency, real estate, bonds, equities, open-ended funds, and so on.
If you just invest in one type of asset class, it is important to diversify your
holdings across that one asset class.
Have a clear and simple understanding of the set of channels through which you assign your assets. 1.2.2 Role Profit maximization
Making a profit is the primary objective of any and all investments. Investors will
be better able to evaluate the potential expansion of a variety of sectors and fields
by building investment portfolios, which will allow them to assign a higher priority
to the distribution of their investment portfolios to various fields and businesses.
From that point on, investors are presented with a plethora of chances to increase
their profits and the effectiveness of their investments. The mitigation of danger
The majority of investors choose to mitigate risk by constructing diversified investment portfolios.
The diversification of the portfolio will assist investors in offsetting risks between
asset classes in the event that certain industries experience a decline or that certain
types of investments experience fluctuations. As a result, the repercussions of
financial risks will be reduced. the most reduced.
1.2.3 The Principle of Diversification
Diversifying your portfolio is an investment strategy that involves dividing your
investment assets across a range of asset classes. The goal of this strategy is to
lower risk while simultaneously increasing the potential for profit. Diversifying
your portfolio allows you to hold a number of assets, such as stocks, bonds, and
other assets. Rather than placing your entire investment in a single stock, bond, or
investment channel, diversifying your portfolio enables you to own a variety of
assets, such as cash, real estate, gold, or other products.
When you diversify your holdings in the market, you bring the level of risk
associated with your investments back into balance. When something impacts one
area of investment, it is still possible for other areas to help compensate for the
loss. This serves to lower the overall risk that is associated with your portfolio,
which, in turn, boosts your portfolio's profitability over the long term.
Diversification of your holdings, on the other hand, does not in and of itself
guarantee your portfolio's ultimate safety. There are still a number of factors that
could have an effect on the market as a whole or on the various asset classes that
make up your portfolio. Therefore, diversification of investment portfolios is
merely one of the numerous techniques to lessen the risk of investing. 1.2.4 Portfolio management Actively:
Active portfolio management is a form of investment management that makes use
of the active participation of humans. When determining whether to buy or sell a
company or how to build the portfolio, proactive administrators will rely on
analytical and forecasting information as well as the judgment and experience of
the portfolio manager or management team.
These active portfolio managers are not going to subscribe to the efficient market
hypothesis. They are of the opinion that it is feasible to make money on the stock
market by locating stocks that are mispriced. Passive:
Index investing is another name for passive portfolio management, which is also used sometimes.
The goal here is to amass earnings, reduce risks, and keep fees to a bare minimum
by way of establishing a diversified portfolio.
As a consequence of this, investors who adopt the passive portfolio management
strategy will buy stocks that are included in prominent indices. Such as the TCBF
bond fund or the TCBS TCEF stock fund, and keep it in your investment portfolio
for an extended period of time.
When it comes to having success as an investor, one of the most important things
to do is keep a portfolio that is adequately diversified. Moreover, one of the most
efficient ways to achieve this diversification is by constructing a portfolio
comprised of fund certificates. By holding all or representative shares of a fund,
fund certificates disperse the risk across a large area and spare investors the need to
make frequent purchases and sales of securities. Because of this, the fees and
operational costs associated with these funds are far lower than those associated with actively managed funds.
Passive portfolio managers, as opposed to active portfolio managers, do not
attempt to profit from short-term price swings or attempt to time the market. The
fundamental presumption underlying this approach is that a dynamic market will,
as time passes, result in rising levels of profitability. 1.2.5. CAPM Model
The capital asset pricing model, also known as the CAPM model, is a model that
defines the relationship between risk and expected return and is used to value high- risk securities.
The CAPM demonstrates that the expected return of a security or portfolio will be
equal to that of risk-free securities plus the risk premium. If the anticipated income
does not meet the required minimum income, then the investor will not make the investment. Formula: Advantages:
Simple and straightforward to implement. Disavantages:
Using only the most recent beta does not reveal the complete scope of stability over time.
In actuality, there are not a large number of such assumptions upon which to rely.
Systemic risk is not entirely disregarded by investors.
1.2.6. Portfolio management according to the Markowitz model.
1.2.6.1. Introduction to the Markowitz model.
More than forty years ago, a graduate student in economics from the University of
Chicago, in quest of a thesis topic, ran to a stockbroker who promised to research
the stock market. Harry Markowitz accepted the suggestion and developed a theory
that became a cornerstone of financial economics and changed investment practice.
That earned him part of the 1990 Nobel Prize in Economics. A fundamental
concept of economics is that due to the shortage of resources, all economic
decisions are made in response to the scarcity of resources. confront trade-offs.
Markowitz recognizes investors who confront a trade-off between risk and
expected reward. Investment decisions are not only about owning stocks but also
how to divide the number of shares. It was a question of "Portfolio Selection", the
title of which was a feature article published in the March 1952 issue of the
Financial Review. Markowitz developed critical line algorithms by extending the
methods of linear programming in both the paper and his following work. The
critical line algorithms determine all potential portfolios that simultaneously reduce
risk (as defined by variance or standard deviation) while maintaining the same
level of expected return and maximizing expected return for a given level of
investment. risk level. The efficient limit is formed by these portfolios whenever
the expected return space is graphed within standard deviations of that space.
When an investor is putting together their portfolio, they will have to make a
choice between the amount of risk they are willing to take and the amount of return
they anticipate receiving. The majority of limits represent the optimal level of
diversification for a portfolio. This is due to the fact that diversification is an
effective method for accomplishing the goal of reducing risk.
In the process of selecting a portfolio consisting of common stocks, Harry
Markowitz established the "mean-variance" concept. In the course of the last
twenty years, mean-variance analysis has become more prevalent in the process of
asset allocation. The process of selecting an investment portfolio in which each
component is an asset class rather than a single stock is referred to as asset
allocation. When compared to the selection of portfolios, asset allocation is often
seen as a more fruitful use of mean-variance analysis for a number of reasons.
Analysis using the "mean-variance" method involves knowledge not only of the
expected returns and standard deviations for each asset but also of the correlation
of returns for each asset pair. Unlike the challenge of selecting stocks for a stock
portfolio, which involves hundreds of stocks (and, consequently, thousands of
correlations), the problem of selecting asset classes often involves only a few asset
classes (such as a small number of asset classes). Gold, real estate, cash, stocks,
and bonds are included in this category. In addition, the absence of correlation
across assets presents a chance to cut down on the total risk posed by the portfolio.
The benefits of diversifying a stock portfolio are somewhat limited due to the fact
that stocks frequently go together. On the other hand, correlations across different
asset classes are often rather modest and, in some instances, even negative. As a
result, "mean-variance" is an effective technique in asset allocation for locating big
risk reduction possibilities via diversification. 1.2.6.2. Assumptions
As with any model, it is vital to understand the assumptions of mean-variance
analysis in order to utilize it successfully. First, mean-variance analysis is based on
an individual phase model of the investment. At the beginning of this time,
investors divide their funds across several asset classes, assigning a non-negative weight to each.
During the period, each asset created a random rate of return thus at the conclusion
of the term his assets were altered by the weighted average of the returns. In asset
volume selection, investors are faced with linear limitations, one of which is that
the weights must sum up to one. Based on the game theory work of Von Neumann and Morgenstern:
The investor attempts to maximize the expected return on total assets.
All investors have the same estimated single-period investment.
All investors are risk-averse; that is, they are willing to take greater risk if
they are compensated by a higher expected return.Investment decisions are
made based on expected return and risk.
All markets are totally efficient (e.g., no taxes and no “transaction costs”).
Investors base their decisions on expected return and risk; therefore, the
efficient frontier is an equation of the expected rate of return and the
variance (or standard deviation) of the rate. profit margins.”
1.2.6.3. Optimal Portfolio Selection
Markowitz demonstrates that the correlation (variance) of asset returns, in addition
to the weight of the assets and the standard rate of return, is a crucial factor in
determining the risk of an asset investment. The link between the potential
advantages of a DM and its risks (fluctuations) is described by its effective margin
lines. It could be represented by a boundary line on a risk-to-expected-return graph
for DMIs. The lowest degree of risk or risk necessary to meet the desired rate of
return can be predicted to yield the highest profitable margin lines. For the theory
of DMD construction and valuation, effective boundary lines are crucial. To
demonstrate the advantages of diversification, use the idea of an effective boundary line.
1.2.6.4. Formula of Markowitz Model Yielding:
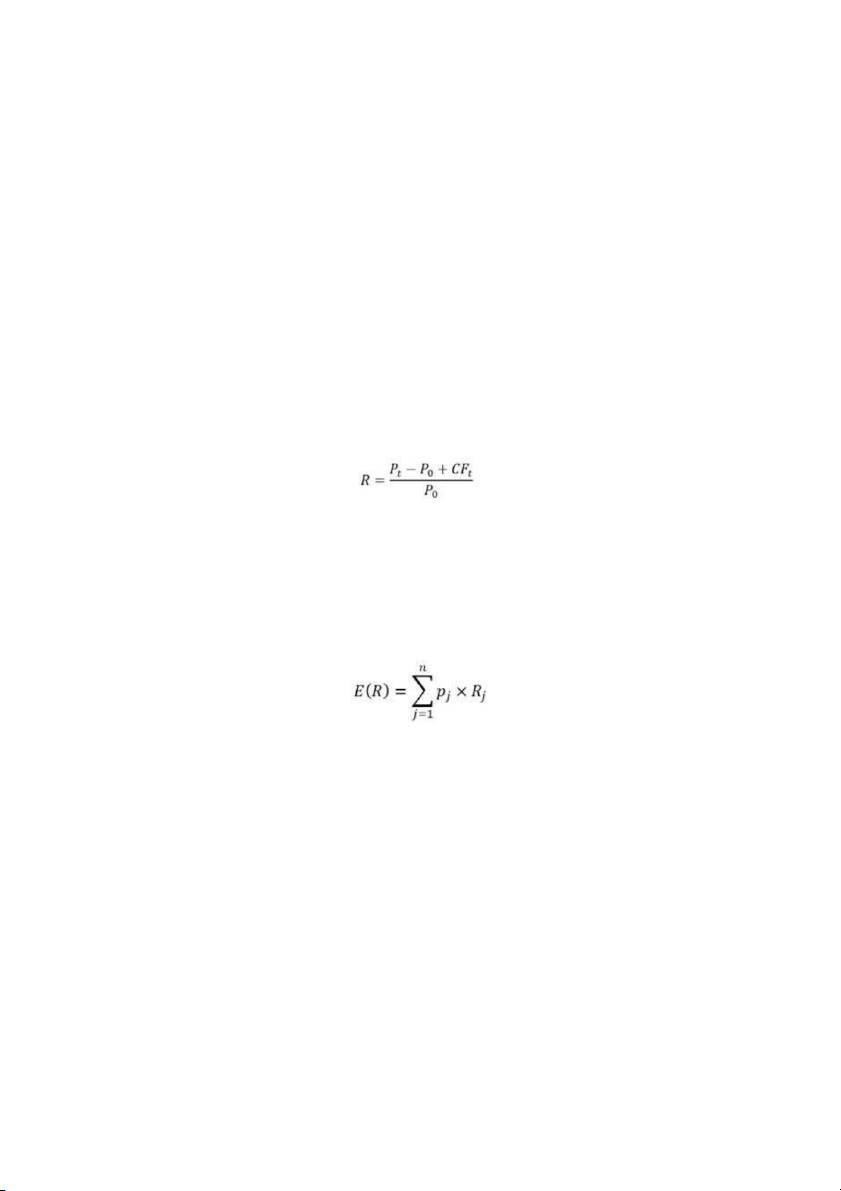
Where: P : The price of the asset in the period t
Po : The price of the asset in the period 0
CFo : Dividend cash flow during the period from to t Expected rate of return:
With Ry : The rate of return of the risky asset in the situation j.
Pj : Likelihood of the rate of return Rj.
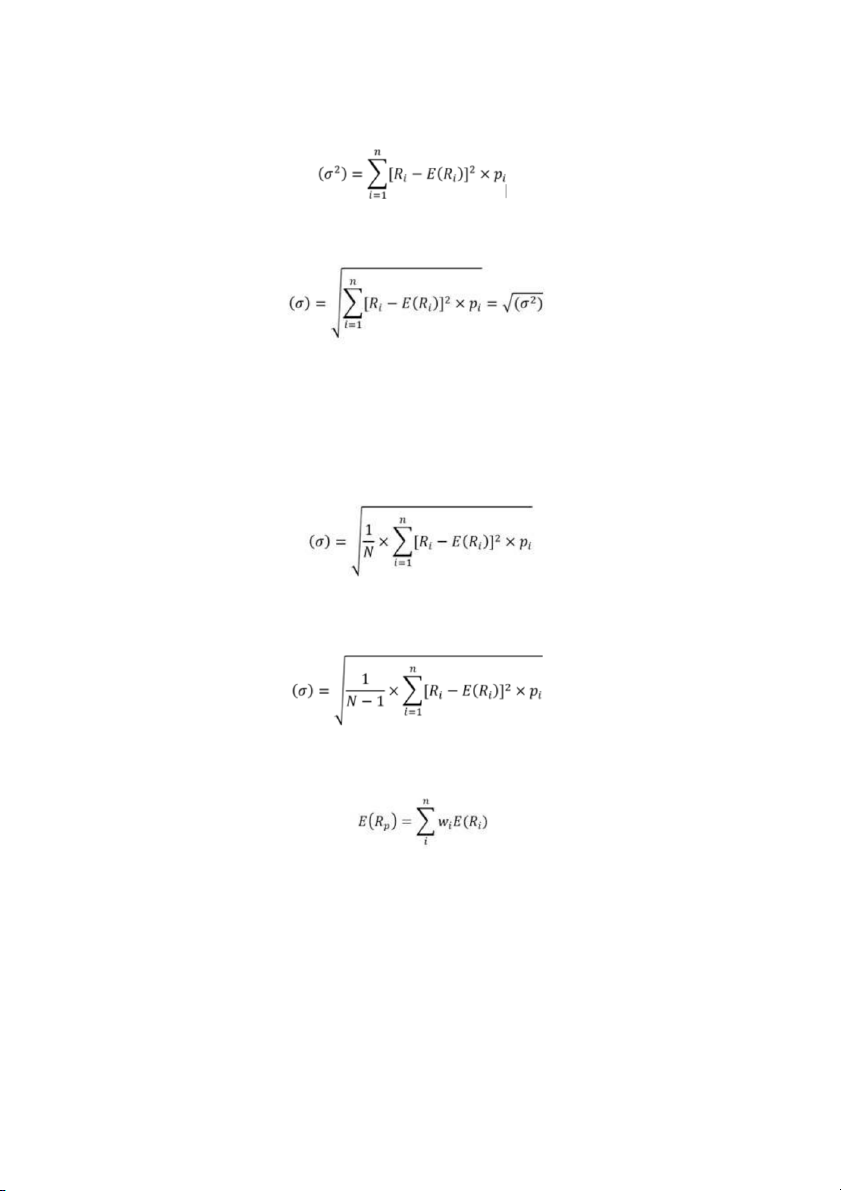
Variance, standard deviation of return for a risky asset: Variance: Standard deviation:
The variance values, however, will be derived from a sample of N observations and
will be based on the standard deviation of the empirical return values.
As a result, below is the formula for an N-observation sample: If N ≥ 30: If N < 30:
• Expected rate of return of a portfolio: With: Where:
: Expected rate of return of the portfolio
: The weight of i item in the portfolio
: The expected rate of return of the security i.
n : Number of securities in the portfolio
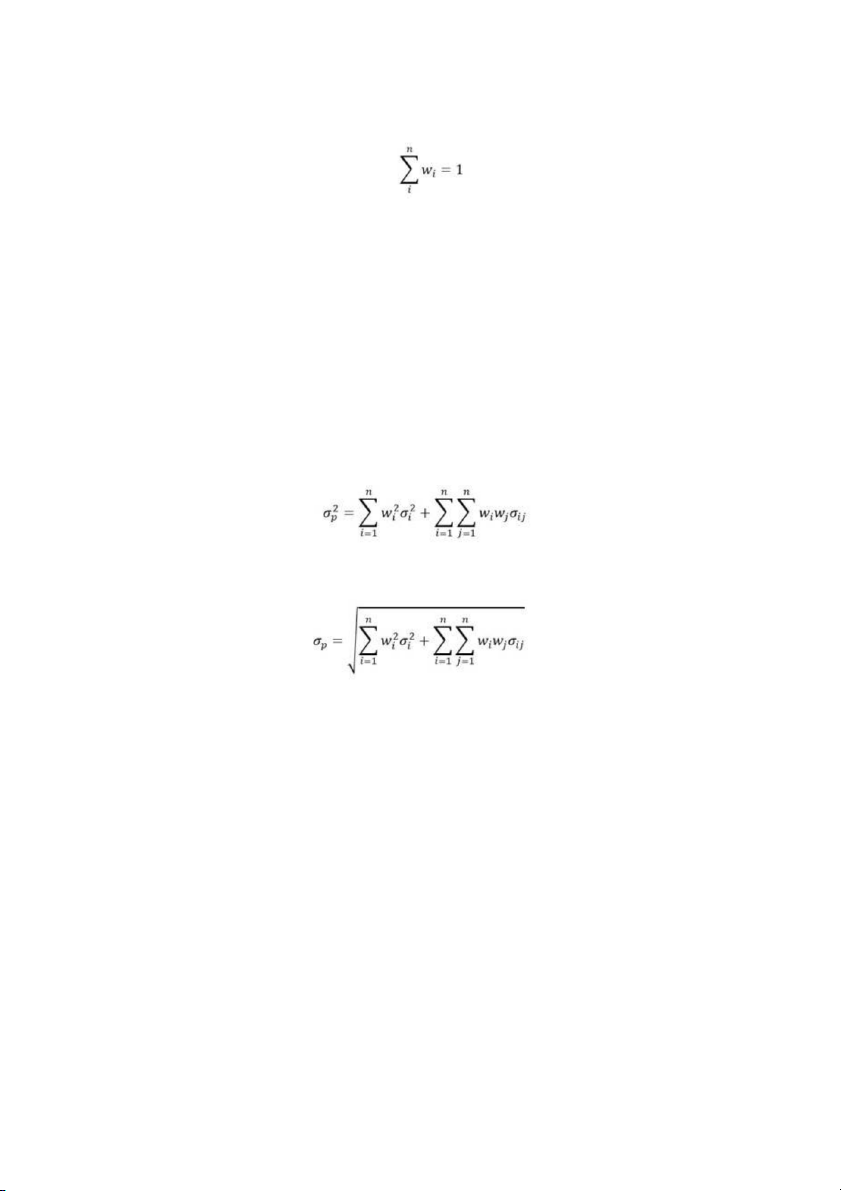
Variance and standard deviation of return on portfolio: Vairiance: Standard deviation:
Standard deviation of the return on the portfolio
The share of the i (or j) asset in the portfolio
Standard deviation of the rate of return on the i asset
The covariance between the return on the i asset and the j asset in the portfolio. Correlation Coefficient: Where:
: Correlation coefficient between the return on asset A and asset B.
The value of is in the range [-1;+1] The standard deviation of Standard Deviation of
1.2.6.5. Benefit and Drawback of Markowitz model: Advantages:
Optimize your portfolio such that you get the highest possible projected rate of
return while taking the fewest possible risks. - Disavantages:
In order to obtain the exact formula, an excessive number of data points with a
regularly distributed distribution are required; however, the documents and
financial statements do not yet fulfill this criteria. Risk-free assets have not yet
been accounted for in the Markowitz model. SOME STOCKS SELECTION METHODS
1.3.1. CANSLIM stock selection method
One of the most successful stock investors in the United States is William J. Oneil,
who founded CANSLIM stock selection. When he first began out, he focused a lot
of his limited study time on learning about the performance and experience of
"high money" in the stock market. After much work and investigation, he was
successful in developing a strategy that may be used to select high-quality stocks.
For this study, the "Wall Street man," or one of the 13 persons who helped him
achieve recognition and fortune, made millions of dollars in earnings from stocks
each year and started the investment research firm William J. O'Neil & Company.
7 elements in the CANSLIM model:
C − Current Quarterly Earnings Per Share
A − Annual Earnings Increases
N − New products (New management, New highs, new tops) S − Supply and demand
L − Leader and laggard I − Institutional sponsorship M − Market direction