lOMoARcPSD|359 747 69
ASSIGNMENT 5 – CONFIDENCE INTERVAL & HYPOTHESIS TESTING
DEADLINE: Monday, April-24-2017 Question 01:
An economist wishes to estimate the average family income in a certain population. The
population standard deviation is known to be $3,500, and the economist uses a random sample of size n = 55.
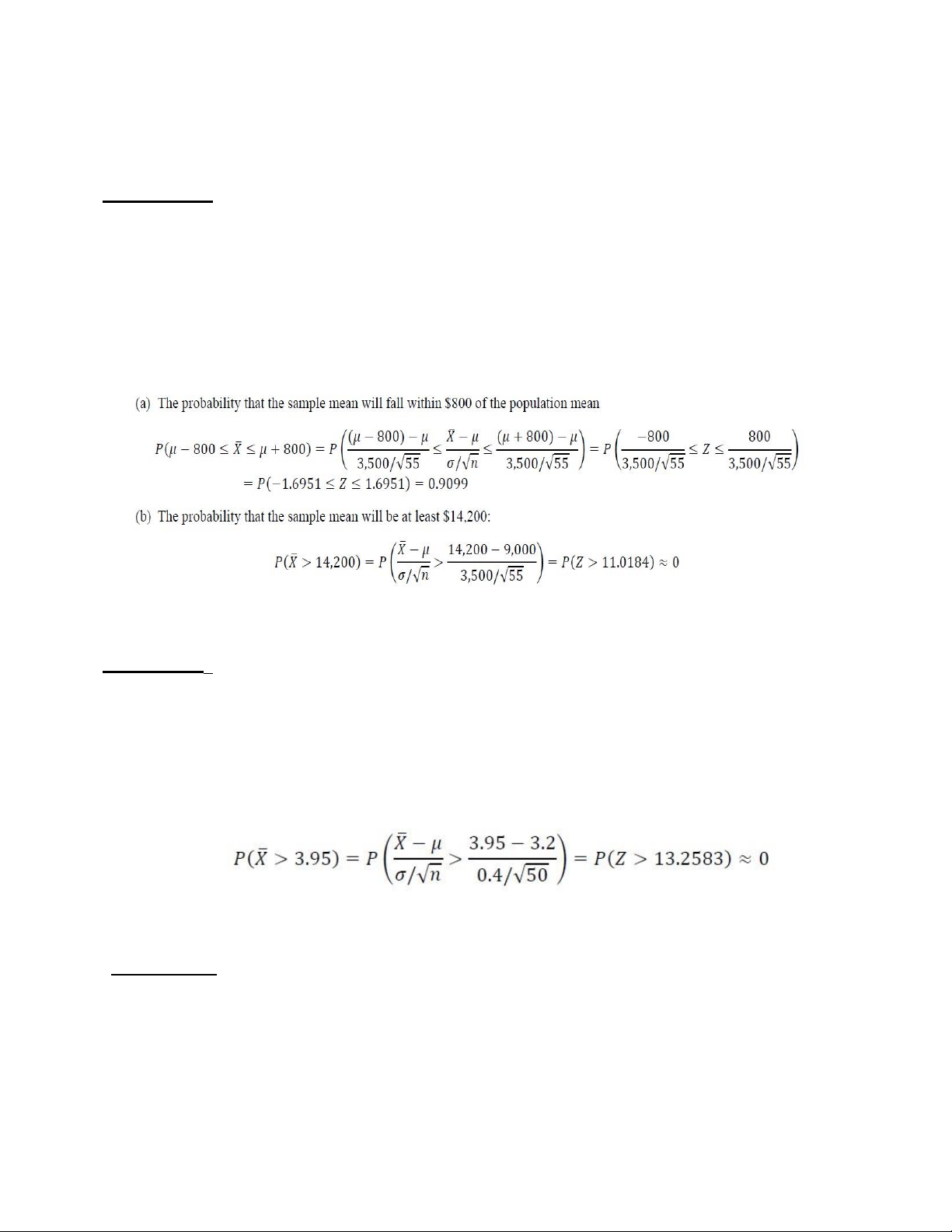
a) What is the probability that the sample mean will fall within $800 of the population mean?
b) Given know we know that the population mean is $9,000. What is the probability that the
sample mean will be at least $14,200? Solution: Question 02:
According to a recent article in Worth, the average price of a house on Marco Island, Florida, is
$3.2 million. Assume that the standard deviation of the prices is $400,000. A random sample of
50 houses is taken and the average price is computed. What is the probability that the sample mean exceeds $3.95 million? Solution: Question 03:
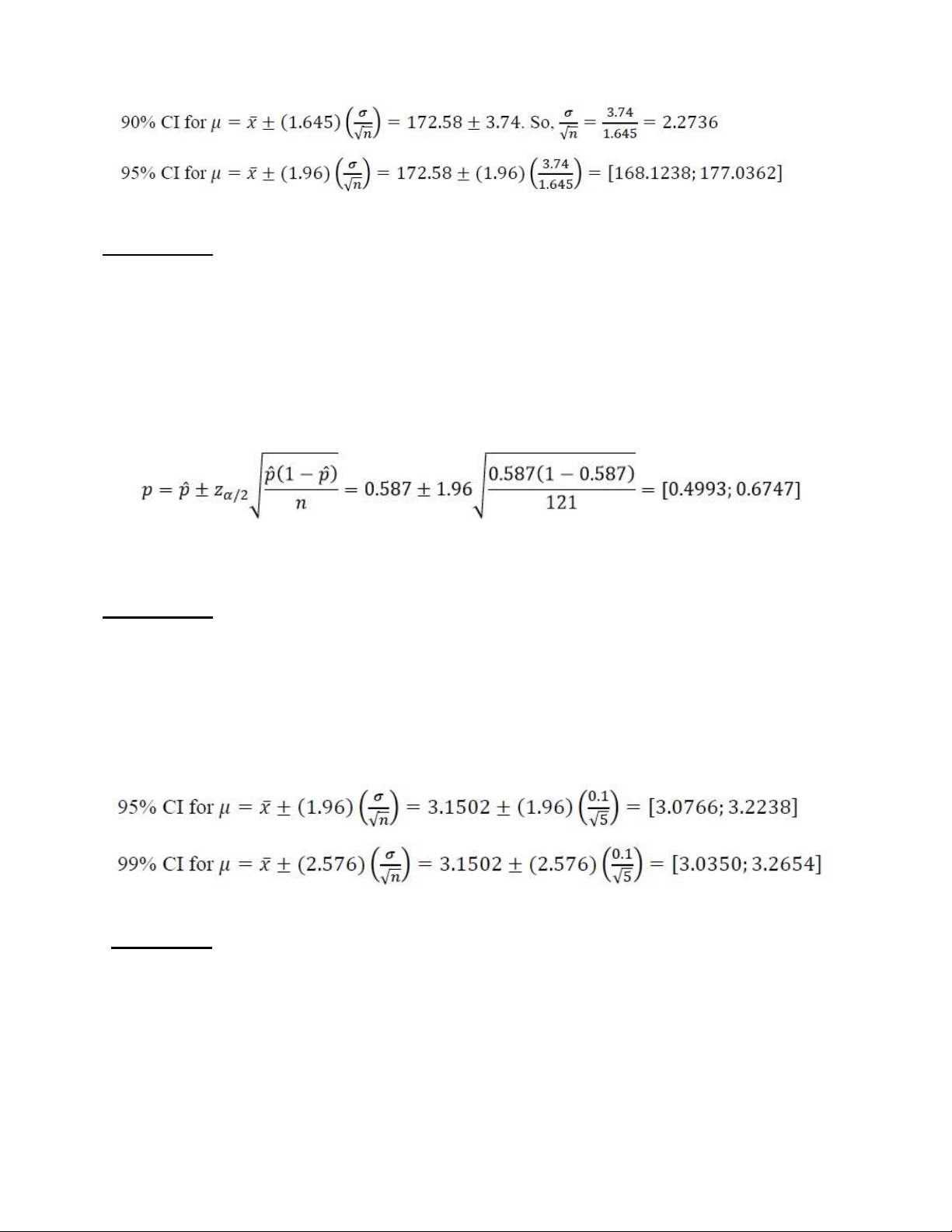
A statistician estimates the 90% confidence interval for the mean of a normally distributed
population as 172.58 3.74 at the end of a sampling experiment, assuming a known population
standard deviation. What is the 95% confidence interval? Solution: lOMoARcPSD|359 747 69 Question 04:
The Java computer language, developed by Sun Microsystems, has the advantage that its
programs can run on types of hardware ranging from mainframe computers all the way down to
handheld computing devices or even smart phones. A test of 121 randomly selected
programmers revealed that 71 preferred Java to their other most used computer languages.
Construct a 95% confidence interval for the proportion of all programmers in the population
from which the sample was selected who prefer Java. Solution: Question 05:
An electric scale gives a reading equal to the true weight plus a random error that is normally
distributed with mean 0 and standard deviation σ = .1 mg. Suppose that the results of five
successive weighings of the same object are as follows: 3.142, 3.163, 3.155, 3.150, 3.141.
a) Determine a 95 percent confidence interval estimate of the true weight.
b) Determine a 99 percent confidence interval estimate of the true weight. Solution: Question 06:
Certain eggs are stated to have reduced cholesterol content, with an average of only 2.5%
cholesterol. A concerned health group wants to test whether the claim is true. The group believes
that more cholesterol may be found, on the average, in the eggs. A random sample of 100 eggs
reveals a sample average content of 5.2% cholesterol, and a sample standard deviation of 2.8%.
Does the health group have cause for action? Solution: lOMoARcPSD|359 747 69 Question 07:
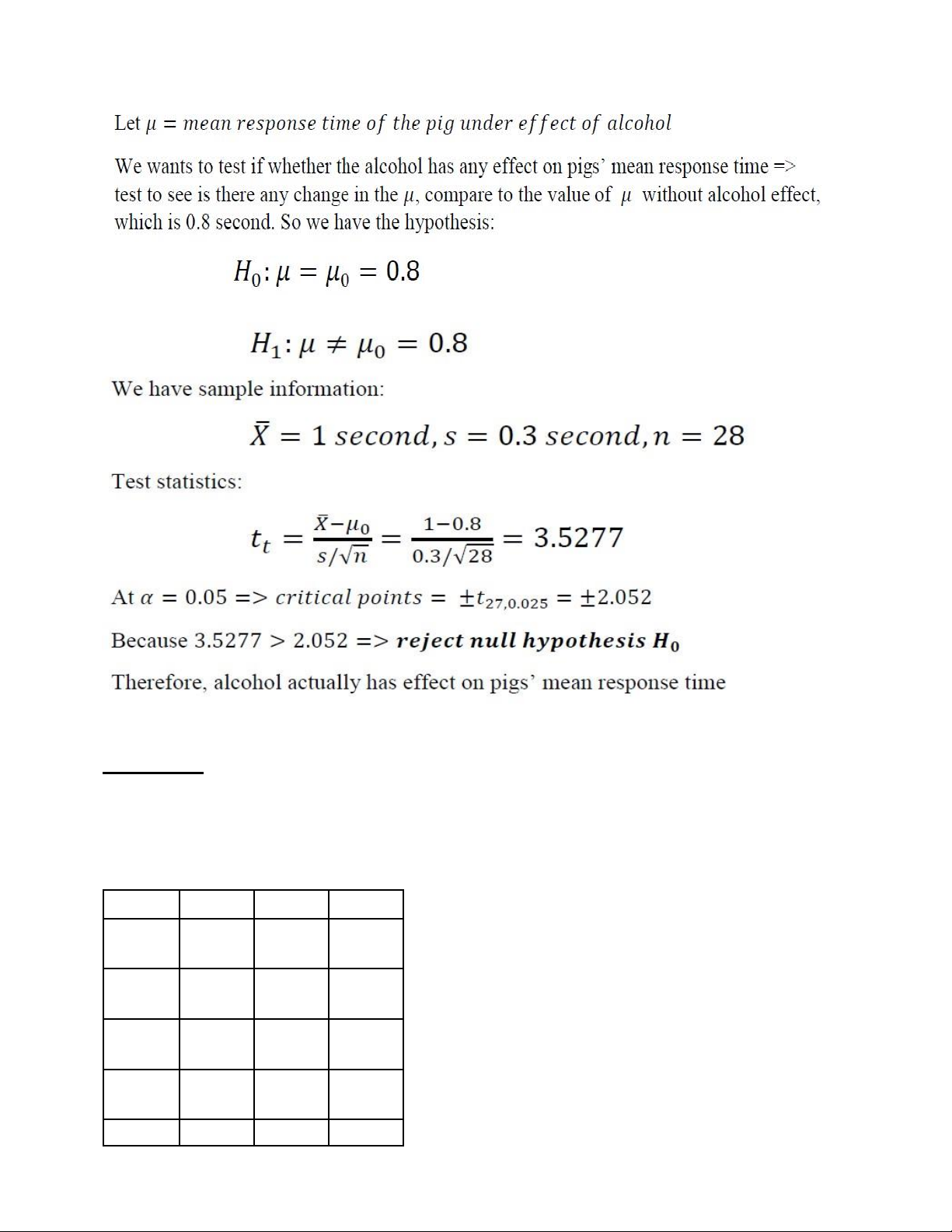
The mean response time of a species of pigs to a stimulus is 0.80 seconds. Twenty eight pigs were
given 2 oz of alcohol and then tested. If their average response time was 1.0 seconds with a
standard deviation of 0.30 seconds, can we conclude that alcohol affects the mean response time?
Use the 5 percent level of significance. Solution: lOMoARcPSD|359 747 69 Question 08:
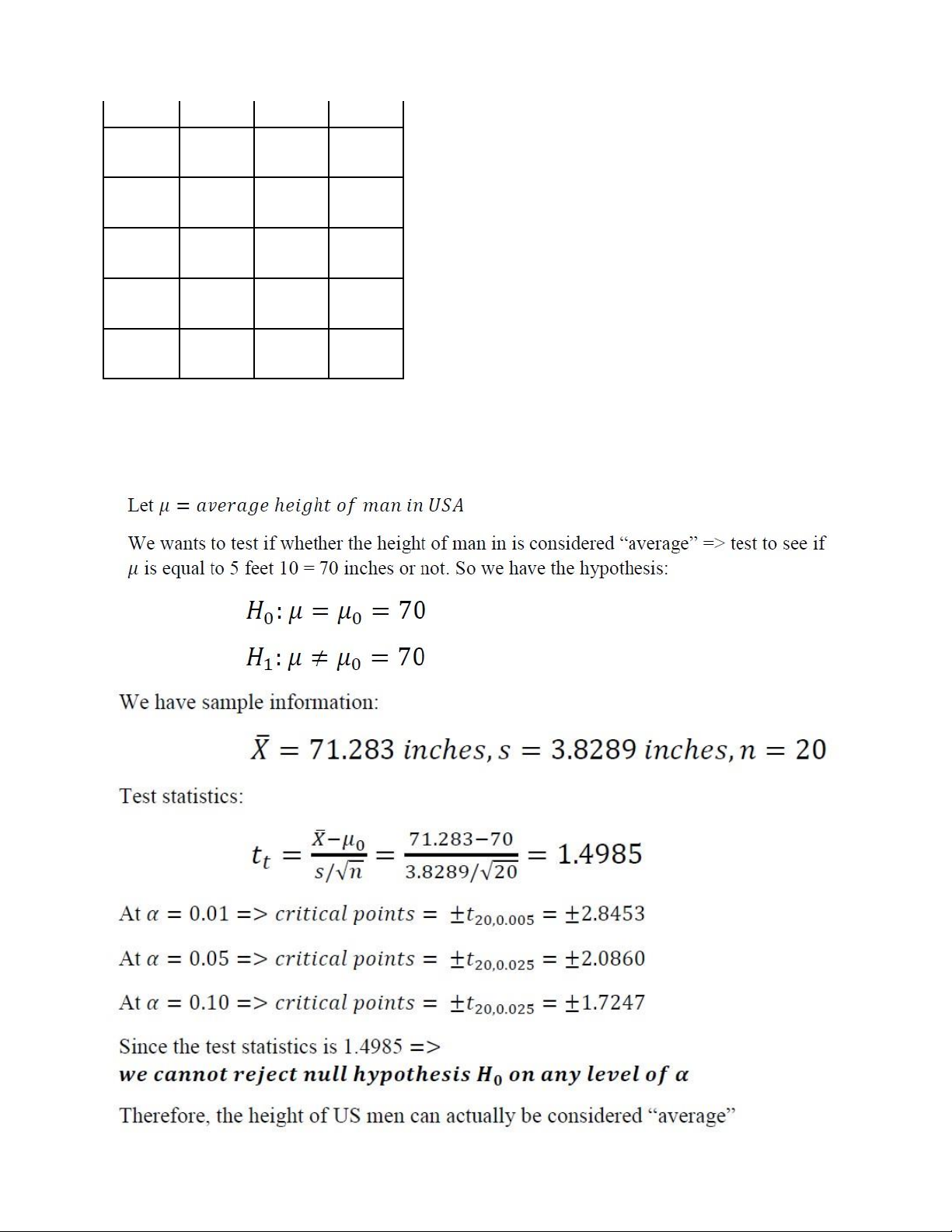
It is known that the average height of a man residing in the United States is 5 feet 10 inches and
the standard deviation is 3 inches. To test the hypothesis that men in your city are “average,” a
sample of 20 men have been chosen. The heights of the men in the sample follow: Man Height Man Height 1 72 11 68.5 2 68.1 12 71.6 3 69.2 13 77.4 4 72.8 14 69.96 5 71.2 15 70.9 lOMoARcPSD|359 747 69 6 72.2 16 79.1 7 70.8 17 73.2 8 74 18 65.8 9 66 19 64.9 10 70.3 20 77.7
What do you conclude? Explain what assumptions you are making. Solution: lOMoARcPSD|359 747 69 Question 09:
Efforts are under way to make the U.S. automobile industry more efficient and competitive so
that it will be able to survive intense competition from foreign automakers. An industry analyst is
quoted as saying, “GM is sized for 60% of the market, and they only have 43%.” General Motors
needs to know its actual market share because such knowledge would help the company make
better decisions about trimming down or expanding so that it could become more efficient. A
company executive, pushing for expansion rather than for cutting down, is interested in proving
that the analyst’s claim that GM’s share of the market is 43% is false and that, in fact, GM’s true
market share is higher. The executive hires a market research firm to study the problem and carry
out the hypothesis test she proposed. The market research agency looks at a random sample of
5,500 cars throughout the country and finds that 2,521 are GM cars. What should be the
executive’s conclusion? How should she present her results to GM’s vice president for operations? Solution Question 10: lOMoARcPSD|359 747 69
According to Money, the average amount of money that a typical person in the United States
would need to make him or her feel rich is at least $1.5 million. A researcher wants to test this 2
claim. A random sample of 100 people in the United States reveals that their mean “amount to
feel rich” is $2.3 million and the standard deviation is $0.5 million. Conduct the test. Solution: