International University - VNUHCM School/Department Name ----------------- Final Examination
Date:06/JUNE/2022; Duration: 120 minutes
Open book; Online; Laptops are allowed.
SUBJECT: Name of course (ID: IS024IU ) Probabilistic Models in Operations Research
Approval by the School/Department of ? Lecturer: Signature Signature Full name:
Full name: Phan Nguyen Ky Phuc Proctor 1 Proctor 2 Signature Signature Full name: Full name: STUDENT INFO Student name: Student ID:
INSTRUCTIONS: the total of point is 100 (equivalent to 40% of the course) 1. Purpose:
• Test your knowledge in 1,2 (CLOi)
• Test your knowledge, skill in 1,2 (CLOi)
International University – VNUHCM Student Name:………... …………. School/Department Name Student ID: ………………………. ----------------- 2. Requirements:
• Read carefully each question and answer it following the requirements
• Write the answers and draw models CLEAN and TIDY directly in the exam paper QUESTIONS Q1. (15 marks)
The dollar amount of damage involved in an automobile accident is an exponential random variable with
mean 1000. Of this, the insurance company only pays that amount exceeding (the deductible amount of) 400. a)
Find the probability that when an accident occurs, the company has to pay for the damage (7pts) b)
Find the expected value that the insurance company pays per accident, i.e. E[(X-400)+] (8pts) Q2. (25 marks)
A queuing model with 2 servers A, B. The service rates of these servers are , . A person can be served either by
A or B then leaves the system. If a person decides to enter the system and two servers are free, then his
probability to select server A or B are . Assume that the arrival rate is . If the arrival finds n customers already
in the station, then he will enter the system with probability with a) Define the state space (5pts)
b) Draw the transition graph (5pts)
c) Write down the balance equations (5pts)
d) Find the expected number of people in the system (5pts)
e) Find the expected time that a person spends in the system (5pts)
Note: Don’t make any calculation just write down the formula Q3. (25 marks)
A small barbershop, operated by two barbers A and B, has room for at most two customers. The customers are
always served by A first. After being severed by A, if B is free then the customer moves to B, otherwise he
leaves the system. Potential customers arrive at a Poisson rate of 3 per hour, and the service rate of the barber A and B are 2,4 per hour
a) Define the state space (5pts)
b) Draw the transition graph (5pts)
c) Write down the balance equations (5pts)
d) What is the average number of customers in the shop? (5pts)
e) What is the proportion of potential customers that enter the shop? (5pts) Q4. (15 marks)
A system has 3 servers, namely A, B, and C. The are two types of request, namely 1 and 2 can be handled by the system. /32
International University – VNUHCM Student Name:………... …………. School/Department Name Student ID: ………………………. -----------------
For request type 1, it must follow the sequence C-A-C.
For request type 2, it must follow the sequence B-C.
It is assumed that the service time at A, B and C follow exponential distribution
The processing rate of request type 1 on server A, and C are 1, 2
The processing rate of request type 2 on server B and C are 3, 2.
If the request of type 1 arrives and there is a request type 2 in server C. Find the expectation of total time
that the request type 1 spend in the system (8 pts) If the request of type 2 arrives and there is a request type
1 in server C for the 2nd service. Find the expectation of total time that the request type 2 spend in the system. (7 pts)
Note: In the state space, you need to differentiate how many times the request type 1 has visit C. Q6. (20 marks)
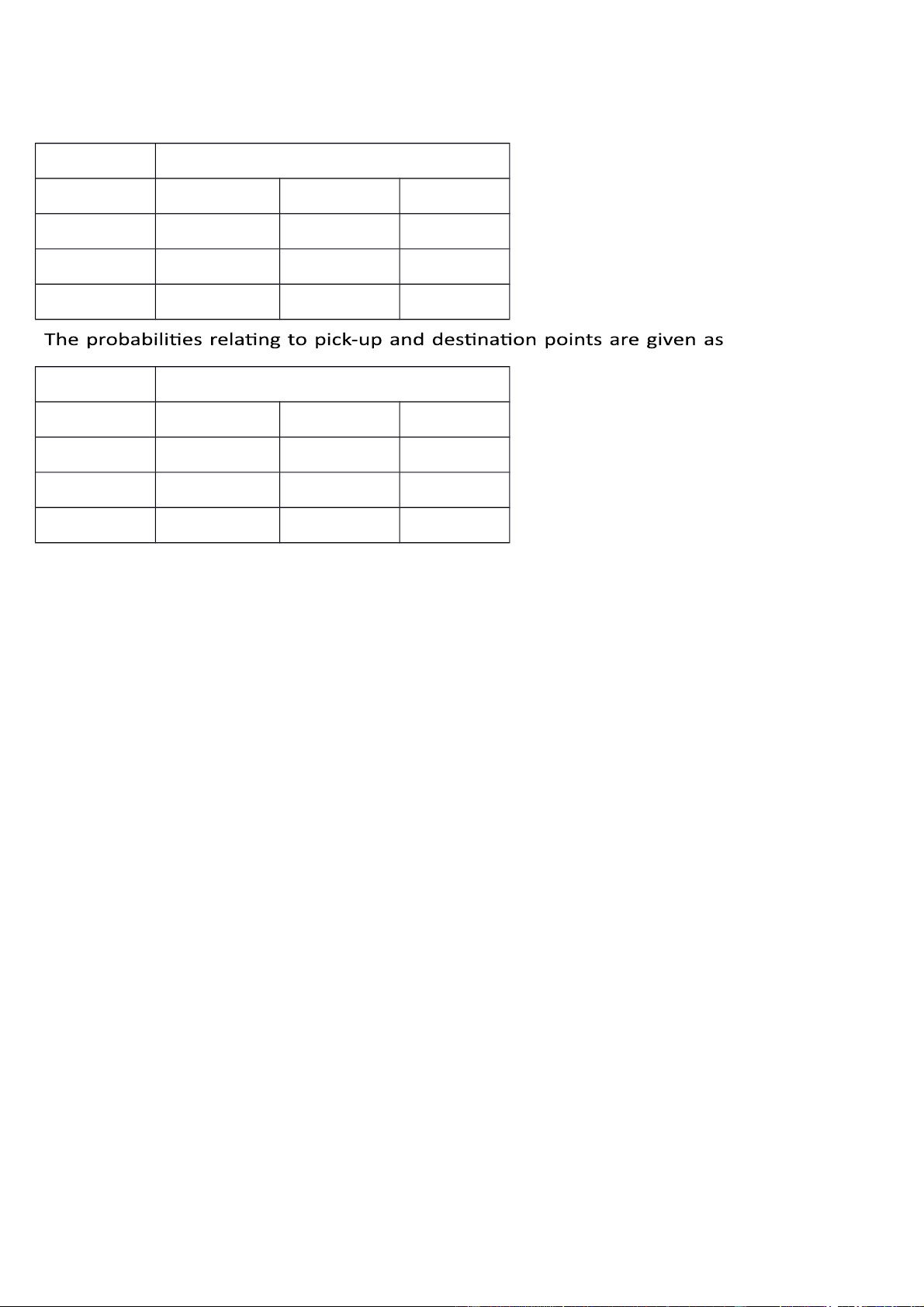
A taxi driver provides service in 3 zones A, B, and C of a city. Following table given the fare prices between the
pick-up and destination points Destination Pick up A B C A 7 10 12 B 10 6 13 C 12 13 5 Destination Pick up A B C A 0.4 0.3 0.3 B 0.2 0.6 0.2 C 0.3 0.2 0.5
a) Find the long run probability in each state.
b) Find the taxi driver’s average profit per trip. – END –