Tài liệu tự học giới hạn của hàm số – Nguyễn Trọng

Tài liệu gồm 87 trang được biên soạn bởi thầy Nguyễn Trọng, hướng dẫn tự học chuyên đề giới hạn của hàm số, thuộc chương trình Đại số và Giải tích 11 (Toán 11) chương 4 bài số 2.

79 40 lượt tải Tải xuống
Chia sẻ Báo cáo tài liệu

Chủ đề: Chương 5: Giới hạn. Hàm số liên tục (KNTT)

Môn: Toán 11

Thông tin:

87 trang 8 tháng trước

Tác giả:

Profile Kim Oanh
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
1
CHƯƠNG 4 GIỚI HẠN
BÀI 2. GIỚI HẠN CỦA HÀM SỐ
A. TÓM TẮT LÝ THUYẾT
Định nghĩa 1 (Giới hạn của hàm số tại một điểm).
Giả sử
( )
;ab
là một khoảng chứa điểm
0
x
và
f
là một hàm số xác định trên tập hợp
( )
0
;\a b x
. Ta nói rằng hàm số
f
có giới hạn là số thực
L
khi
dần đến
0
x
(hoặc tại điểm
0
x
) nếu
với mọi dãy số
( )
n
x
trong tập hợp
( )
0
;\a b x
mà
0
lim
n
xx=
ta đều có
( )
lim
n
f x L=
.
Khi đó ta viết
( )
0
lim
xx
f x L
→
=
hoặc
( )
fx L→
khi
0
xx→
.
Định nghĩa 2 (Giới hạn của hàm số tại vô cực).
Giả sử hàm số
f
xác định trên khoảng
( )
;a +
. Ta nói rằng hàm số
f
có giới hạn là số thực
L
khi
x
dần tới
+
nếu với mọi dãy số
( )
n
x
trong khoảng
( )
;a +
mà
lim
n
x =+
ta đều có
( )
lim
n
f x L=
.
Khi đó ta viết
( )
lim
x
f x L
→+
=
hoặc
( )
fx L→
khi
x →+
.
GIỚI HẠN HỮA HẠN
GIỚI HẠN VÔ CỰC
Giới hạn đặc biệt
1)
0
0
lim
xx
xx
→
=
.
2)
0
lim
xx
cc
→
=
( )
c
.
Giới hạn đặc biệt
1)
lim
k
x
x
→+
=+
. 2)
lim 0
x
k
c
x
→
=
.
3)
0
1
lim
x
x
−
→
=−
. 4)
0
1
lim
x
x
+
→
=+
.
5)
( )
khi 2 0
li
khi 2
m
x
k
kk
k
x
→−
+
=
−
Định lí
Nếu
( )
0
lim
xx
f x L
→
=
và
( )
0
lim
xx
g x M
→
=
thì
1)
( ) ( )
0
lim
xx
f x g x L M
→
=


.
2)
( ) ( )
0
lim . .
xx
f x g x L M
→
=


.
3)
( )
( )
0
lim
xx
fx
L
g x M
→
=
với
0M
.
Nếu
( )
0fx
và
( )
0
lim
xx
f x L
→
=
thì
( )
0
lim
xx
f x L
→
=
và
( )
0
lim
xx
f x L
→
=
.
Định lí 1
Nếu
( )
0
lim 0
xx
f x L
→
=
và
( )
0
lim
xx
fx
→
= 
thì
( ) ( )
( )
( )
0
0
0
khi .lim 0
lim .
khi .lim 0
xx
xx
xx
L g x
f x g x
L g x
→
→
→
+
=


−
.
Nếu
( )
0
lim 0
xx
gx
→
=
thì
( )
( )
( )
( )
0
khi . 0
lim
khi . 0
xx
L g x
fx
gx
L g x
→
+
=
−
.
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
2
Giới hạn một bên
( ) ( ) ( )
0
00
lim li ilm m
xx
xx
xx
f x f x Lf x L
+−
→
→→
= ==
.
B. DẠNG TON V BÀI TẬP
Dạng 1. Tnh giới hạn vô định dạng
0
0
, trong đ t thc v mu thc l cc đa thc.
Phương php giải:
Khử dạng vô định bằng cách phân tch thnh tch bằng cách chia Hooc – nơ (đầu rơi, nhân tới, cộng
cho), ri sau đó đơn giản biểu thức để khử dạng vô định.
VÍ DỤ
Ví dụ 1. Tnh giới hạn
2
2
2
2 3 14
lim
4
x
xx
A
x
→
+−
=
−
. Đs:
11
4
A =
.
Li giải
Ta có
2
2
2 2 2
7
2(x 2)(x )
2 3 14 2 7 11
2
lim lim lim
4 (x 2)(x 2) 2 4
x x x
x x x
A
xx
→ → →
−+
+ − +
= = = =
− − + +
! Cn nhớ:
( )( )
2
12
( ) af x x bx c a x x x x= + + = − −
với
12
,xx
l 2 nghim của phương trnh
( )
0fx=
. Hc sinh thưng quên nhân thêm
a
.
Ví dụ 2. Tnh giới hạn
32
32
2
2 5 2 3
lim
4 13 4 3
x
x x x
A
x x x
→
− − −
=
− + −
. Đs:
11
17
A =
.
Li giải
( )
( )
( )
( )
2
3 2 2
3 2 2
2
3 3 3
3 2 1
2 5 2 3 2 1 11
lim lim lim
4 13 4 3 4 1 17
3 4 1
x x x
x x x
x x x x x
A
x x x x x
x x x
→ → →
− + +
− − − + +
= = = =
− + − − +
− − +
Nhn xt: Bng chia Hooc – nơ (đu rơi, nhân ti cng cho) như sau:
Phân tch
32
2 5 2 3x x x− − −
thnh tch s:
( )
( )
3 2 2
2 5 2 3 3 2 1x x x x x x − − − = − + +
Phân tch
32
4 13 4 3x x x− + −
thnh tch s:
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
3
( )
( )
3 2 2
4 13 4 3 3 4 1x x x x x x − + − = − − +
.
Ví dụ 3. Tnh giới hạn
100
50
1
21
lim
21
x
xx
A
xx
→
−+
=
−+
. Đs:
49
24
A =
.
Li giải
Ta có
( )
( )
( )
( )
99
100 100
50 50
49
1 1 1
11
2 1 ( ) ( 1)
lim lim lim
2 1 ( ) ( 1)
11
x x x
x x x
x x x x x
A
x x x x x
x x x
→ → →
− − −
− + − − −
= = =
− + − − −
− − −
( )
( )
( )
( )
( )
( )
( )
( )
( )
( )
98 97 96
48 47 46
1
99 98 97 2
49 48 47 2
1
1 .... 1 1
lim
1 .... 1 1
1 .... 1
lim
1 .... 1
x
x
x x x x x x x
x x x x x x x
x x x x x x
x x x x x x
→
→
− + + + + + − −
=
− + + + + + − −
− + + + + + −
=
− + + + + + −
( )
( )
99 98 97 2
49 48 47 2
1
.... 1
98 49
lim
48 24
.... 1
x
x x x x x
x x x x x
→
+ + + + + −
= = =
+ + + + + −
!Cn nhớ: Hng đng thc
( )
( )
1 2 2
1 1 .... 1 .
n n n
x x x x x x
−−
− = − + + + + +
Chứng minh: Xt cp s nhân
2 3 1
1, , , ,....,
n
x x x x
−
c
s hng v
1
1, .u q x==
Khi đ
( )
( )
2 1 2 1
1
11
1 ... 1. 1 1 1 ... .
11
nn
n n n
n
qx
S x x x u x x x x x
qx
−−
−−
= + + + + = = − = − + + + +
−−
BÀI TẬP ÁP DỤNG
Bài 1. Tnh các giới hạn sau:
1)
2
2
2
32
lim
4
x
xx
A
x
→
−+
=
−
. ĐS:
1
4
A =
. 2)
2
2
1
1
lim
34
x
x
A
xx
→
−
=
+−
. ĐS:
2
5
A =
.
3)
2
2
3
7 12
lim
9
x
xx
A
x
→
−+
=
−
. ĐS:
1
6
A =−
. 4)
2
2
5
9 20
lim
5
x
xx
A
xx
→
−+
=
−
. ĐS:
1
5
A =
.
5)
2
2
3
3 10 3
lim
56
x
xx
A
xx
→
−+
=
−+
. ĐS:
8A =
. 6)
2
2
1
23
lim
21
x
xx
A
xx
→
+−
=
−−
. ĐS:
4
3
A =
.
7)
4
2
2
16
lim
68
x
x
A
xx
→−
−
=
++
. ĐS:
16A =−
. 8)
1
23
lim
54
x
xx
A
xx
→
−−
=
−+
.ĐS:
4
3
A =−
.
9)
3
2
2
8
lim
32
x
x
A
xx
→
−
=
−+
. ĐS:
12A =
. 10)
3
2
2
8
lim
11 18
x
x
A
xx
→−
+
=
++
. ĐS:
12
7
A =
.
Bài 2. Tnh các giới hạn sau:
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
4
1)
32
2
1
2 5 2 1
lim
1
x
x x x
A
x
→
− + +
=
−
. ĐS:
1A =−
. 2)
3
4
1
32
lim
43
x
xx
A
xx
→
−+
=
−+
. ĐS:
1
2
A =
.
3)
32
32
1
2 5 4 1
lim
1
x
x x x
A
x x x
→−
+ + +
=
+ − −
. ĐS:
1
2
A =
. 4)
43
32
1
1
lim
5 7 3
x
x x x
A
x x x
→
− − +
=
− + −
. ĐS:
3
2
A =−
.
5)
32
2
3
2 3 9 7 3
lim
3
x
x x x
A
x
→−
− + + +
=
−
. ĐS:
18 19 3
6
A
+
=
.
6)
32
42
3
5 3 9
lim
89
x
x x x
A
xx
→
− + +
=
−−
. ĐS:
0A =
.
7)
3
42
1
1
lim
43
x
x
A
xx
→
−
=
−+
. ĐS:
3
4
A =
. 8)
3
2
1 12
lim
28
x
A
xx
→

=−

−−

. ĐS:
1
2
A =
.
9)
22
2
11
lim
3 2 5 6
x
A
x x x x
→

=+

− − − −

. ĐS:
2A =−
.
10)
23
1
11
lim
21
x
A
x x x
→

=−

+ − −

. ĐS:
1
9
A =
.
Bài 3. Tnh các giới hạn sau:
1)
20
30
1
21
lim
21
x
xx
A
xx
→
−+
=
−+
. ĐS:
8
14
A =
. 2)
50
2
1
1
lim
32
x
x
A
xx
→
−
=
−+
. ĐS:
50A =−
.
3)
( )
2
1
1
lim
1
n
x
x nx n
A
x
→
− + −
=
−
(Với
l số nguyên). ĐS:
2
2
nn
A
−
=
.
4)
( )
( )
1
2
1
1
lim
1
n
x
x n x n
A
x
+
→
− + +
=
−
. ĐS:
( )
1
2
nn
A
+
=
.
5)
23
23
1
...
lim
...
n
m
x
x x x x n
A
x x x x m
→
+ + + + −
=
+ + + + −
(
,mn
l số nguyên) . ĐS:
( )
( )
1
1
nn
A
mm
+
=
+
.
6)
1
lim
11
mn
x
mn
A
xx
→

=−

−−

. ĐS:
2
mn
A
−
=
.
LỜI GIẢI
Bài 1. 1) Ta có
( )( )
( )( )
2
2
2 2 2
12
3 2 1 1
lim lim lim
4 2 2 2 4
x x x
xx
x x x
A
x x x x
→ → →
−−
− + −
= = = =
− − + +
.
2) Ta có
( )( )
( )( )
2
2
1 1 1
11
1 1 2
lim lim lim
3 4 1 4 4 5
x x x
xx
xx
A
x x x x x
→ → →
−+
−+
= = = =
+ − − + +
.
3) Ta có
( )( )
( )( )
2
2
3 3 3
34
7 12 4 1
lim lim lim
9 3 3 3 6
x x x
xx
x x x
A
x x x x
→ → →
−−
− + −
= = = = −
− − + +
.
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
5
4) Ta có
( )( )
( )
2
2
5 5 5
45
9 20 4 1
lim lim lim
5 5 5
x x x
xx
x x x
A
x x x x x
→ → →
−−
− + −
= = = =
−−
.
5) Ta có
( )( )
( )( )
2
2
3 3 3
3 1 3
3 10 3 3 1
lim lim lim 8
5 6 2 3 2
x x x
xx
x x x
A
x x x x x
→ → →
−−
− + −
= = = =
− + − − −
.
6) Ta có
( )( )
( )( )
2
2
1 1 1
13
2 3 3 4
lim lim lim
2 1 1 2 1 2 1 3
x x x
xx
x x x
A
x x x x x
→ → →
−+
+ − +
= = = =
− − − + +
.
7) Ta có
( )( )
( )
( )( )
( )
( )
( )
22
4
2
2 2 2
2 2 4 2 4
16
lim lim lim 16
6 8 2 4 4
x x x
x x x x x
x
A
x x x x x
→− →− →−
− + + − +
−
= = = = −
+ + + + +
.
8) Ta có
( )( )
( )( )
( )
( )
1 1 1
1 3 3
2 3 4
lim lim lim
3
54
1 4 4
x x x
x x x
xx
A
xx
x x x
→ → →
− + +
−−
= = = = −
−+
− − −
.
9) Ta có
( )
( )
( )( )
( )
( )
22
3
2
2 2 2
2 2 4 2 4
8
lim lim lim 12
3 2 2 1 1
x x x
x x x x x
x
A
x x x x x
→ → →
− + + + +
−
= = = =
− + − − −
.
! Cn nh: Hng đng thc
( )
( )
3 3 2 2
a b a b a ab b+ = + − +
v
( )
( )
3 3 2 2
a b a b a ab b− = − + +
.
10) Ta có
( )
( )
( )( )
( )
( )
22
3
2
2 2 2
2 2 4 2 4
8 12
lim lim lim
11 18 2 9 9 7
x x x
x x x x x
x
A
x x x x x
→− →− →−
+ − + − +
+
= = = =
+ + + + +
.
Bài 2. 1)
( )
( )
( )( )
2
3 2 2
2
1 1 1
1 2 3 1
2 5 2 1 2 3 1
lim lim lim 1
1 1 1 1
x x x
x x x
x x x x x
A
x x x x
→ → →
− − −
− + + − −
= = = = −
− − + +
.
2)
( ) ( )
( )
( )
2
3
2
42
2
1 1 1
12
3 2 2 1
lim lim lim
4 3 2 3 2
1 2 3
x x x
xx
x x x
A
x x x x
x x x
→ → →
−+
− + +
= = = =
− + + +
− + +
.
3)
( ) ( )
( ) ( )
2
32
2
32
1 1 1
1 2 1
2 5 4 1 2 1 1
lim lim lim
1 1 2
11
x x x
xx
x x x x
A
x x x x
xx
→− →− →−
++
+ + + +
= = = =
+ − − −
+−
.
4)
( )
( )
( ) ( )
2
2
4 3 2
2
32
1 1 1
11
1 1 3
lim lim lim
5 7 3 3 2
13
x x x
x x x
x x x x x
A
x x x x
xx
→ → →
− + +
− − + + +
= = = = −
− + − −
−−
.
5) Ta có
( ) ( )
( )
( )( )
2
32
2
33
3 2 3 2 3 7 3 3
2 3 9 7 3
lim lim
3
33
xx
x x x
x x x
A
x
xx
→− →−

+ − + + +
− + + +

= = −

−
+−


( )
2
3
2 3 2 3 7 3 3
18 19 3
lim
6
3
x
xx
x
→−

− + + +
+

= − =

−

.
6) Ta có
( )( )
( )( )
( )
( )( )
( )
( )
2
32
42
22
3 3 3
1 3 1 3
5 3 9
lim lim lim 0
89
3 3 1 3 1
x x x
x x x x
x x x
A
xx
x x x x x
→ → →
− − − −
− + +
= = = =
−−
− + + + +
.
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
6
7) Ta có
( )
( )
( )
( )
( )
( )
22
3
42
3 2 3 2
1 1 1
1 1 1
13
lim lim lim
4 3 4
1 3 3 3 3
x x x
x x x x x
x
A
xx
x x x x x x x
→ → →
− − − − − − −
−
= = = =
−+
− + − − + − −
.
8) Ta có
( )
( )
3
3
3
22
1 12 12 16
lim lim
28
28
xx
xx
A
xx
xx
→→
−+

= − =

−−
−−

( )( )
( )
( )
2
2
2
2
22
42
41
lim lim
2 4 2
2 2 4
xx
xx
x
xx
x x x
→→
+−
+
= = =
++
− + +
.
9) Ta có
( )( )
22
22
22
22
1 1 5 6 3 2
lim lim
3 2 5 6
3 2 5 6
xx
x x x x
A
x x x x
x x x x
→→
− − + − −

= + =

− − − −
− − − −

( )
( ) ( )( )
( )( )
2
2
22
22
2
lim lim 2
31
2 3 1
xx
x
xx
x x x
→→
−
= = = −
−−
− − −
.
10) Ta có
( )( ) ( )( )
3 2 3 2
23
2 3 2 3
1 1 1
1 1 1 2 1
lim lim lim
21
2 1 2 1
x x x
x x x x x x
A
x x x
x x x x x x
→ → →
− − − + − − +

= − = =

+ − −
+ − − + − −

( ) ( )
( ) ( )
( )
( )
( )
2
2
2
2
11
11
11
lim lim
9
21
1 2 1
xx
xx
x
x x x
x x x x
→→
−+
+
= = =
+ + +
− + + +
.
Bài 3. 1) Ta có
( )
( )
( )
( )
( )
( )
19
20
20
30 30
29
1 1 1
11
1
21
lim lim lim
2 1 1
11
x x x
x x x
x x x
xx
A
x x x x x
x x x
→ → →
− − −
− − −
−+
= = =
− + − − −
− − −
( )
( )
( )
( )
( )
( )
( )
( )
( )
( )
18 17 19 18
28 27 29 28
11
1 ... 1 1 1 ... 1
lim lim
1 ... 1 1 1 ... 1
xx
x x x x x x x x x x
x x x x x x x x x x
→→
− + + + + − − − + + + −
==
− + + + + − − − + + + −
( )
( )
19 18
29 28
1
... 1
18 9
lim
28 24
... 1
x
x x x
x x x
→
+ + + −
= = =
+ + + −
.
2) Ta có
( )
( )
( )( )
49 48
50 49 48
2
1 1 1
1 ... x 1
1 ... x 1
lim lim lim 50
3 2 1 2 2
x x x
x x x
x x x
A
x x x x x
→ → →
− + + + +
− + + + +
= = = = −
− + − − −
3) Ta có
( )
( )
( )
( )
22
11
11
1
lim lim
11
n
n
xx
x n x
x nx n
A
xx
→→
− − −
− + −
==
−−
( )
( )
( )
( )
12
2
1
1 ... x 1 1
lim
1
nn
x
x x x n x
x
−−
→
− + + + + − −
=
−
( )
( )
( )
12
12
2
11
1 ... x 1
... x 1
lim lim
1
1
nn
nn
xx
x x x n
x x n
x
x
−−
−−
→→
− + + + + −
+ + + + −
==
−
−
1 2 2
1
1 1 ... x 1 1
lim
1
nn
x
x x x
x
−−
→
− + − + + − + −
=
−
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
7
( )
( )
( )
( )
( )
2 3 3 4
1
1 ... x 1 1 ... x 1 ... 1
lim
1
n n n n
x
x x x x x x x
x
− − − −
→
− + + + + + − + + + + + + −
=
−
( ) ( )
2 3 3 4
1
lim ... x 1 ... x 1 ... 1
n n n n
x
x x x x
− − − −
→

= + + + + + + + + + + +

( ) ( )
2
1 2 ... 1
2
nn
nn
−
= − + − + + =
.
4) Ta có
( )
( )
( )
( )
( )
( )
( )
( )
1
1
2 2 2
1 1 1
1 1 1
1
lim lim lim
1 1 1
nn
n
x x x
x x n x x x n x
x n x n
A
x x x
+
+
→ → →
− − − − − −
− + +
= = =
− − −
( )
( )
( )
( )
( )
( )
( )
1 2 1
22
11
1 ... x 1 1 1 ... x
lim lim
11
n n n n
xx
x x x x n x x x x n
xx
− − −
→→
− + + + + − − − + + + −
==
−−
1 2 1 2
11
... x 1 1 ... x 1 1
lim lim
11
n n n n
xx
x x x n x x x
xx
−−
→→
+ + + + − − + − + + − + −
==
−−
( )
( )
( )
( )
( )
1 2 2 3
1
1 ... x 1 1 ... x 1 ... 1
lim
1
n n n n
x
x x x x x x x
x
− − − −
→
− + + + + + − + + + + + + −
=
−
( ) ( )
1 2 2 3
1
lim ... x 1 ... x 1 ... 1
n n n n
x
x x x x
− − − −
→

= + + + + + + + + + + +

( ) ( )
( )
1
1 2 ... 1
2
nn
n n n
+
= + − + − + + =
.
5) Ta có
2 3 1 2
2 3 1 2
11
... 1 1 ... 1 1
lim lim
... 1 1 ... 1 1
n n n
m m m
xx
x x x x n x x x x
A
x x x x m x x x x
−
−
→→
+ + + + − − + − + + − + −
==
+ + + + − − + − + + − + −
( )
( )
( )
( )
( )
( )
( )
( )
( )
( )
1 2 2 3
1 2 2 3
1
1 ... x 1 1 ... x 1 ... 1
lim
1 ... x 1 1 ... x 1 ... 1
n n n n
m m m m
x
x x x x x x x
x x x x x x x
− − − −
− − − −
→
− + + + + + − + + + + + + −
=
− + + + + + − + + + + + + −
( ) ( )
( ) ( )
1 2 2 3
1 2 2 3
1
... x 1 ... x 1 ... 1
lim
... x 1 ... x 1 ... 1
n n n n
m m m m
x
x x x x
x x x x
− − − −
− − − −
→
+ + + + + + + + + + +
=
+ + + + + + + + + + +
( ) ( )
( ) ( )
( )
( )
1
1 2 ... 1 1
lim
1 2 ... 1 1
x
n n n n n
m m m m m
→
+ − + − + + +
==
+ − + − + + +
.
6) Ta có
11
11
lim lim
1 1 1 1 1 1
m n m n
xx
m n m n
A
x x x x x x
→→

= − = − − −

− − − − − −

11
11
lim lim
1 1 1 1
mn
xx
mn
x x x x
→→
= − − −
− − − −
V
( )
( )
( ) ( )
2 1 2 1
1 1 1
1 ... x 1 1 ... 1 x
1
lim lim lim
1 1 1 1 x
mm
m m m
x x x
m x x x x
m
x x x
−−
→ → →
− + + + + − + − + + −

− = =

− − − −

( ) ( )
( )
( )
( )
22
21
1
1 1 1 .... 1 ...
lim
1 1 ...
m
m
x
x x x x x
x x x x
−
−
→

− + + + + + + + +

=
− + + + +
( )
( )
22
21
1
1 1 .... 1 ...
1 2 3 ... 1 1
lim
1 ... 2
m
m
x
x x x x
mm
x x x m
−
−
→
+ + + + + + + +
+ + + + − −
= = =
+ + + +
Tương tự ta có
1
11
lim
1 1 2
n
x
nn
xx
→
−

−=

−−

Vậy
1
11
lim
1 1 2 2 2
mn
x
m n m n m n
xx
→
− − −

− = − =

−−

.
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
8
Dạng 2. Tính giới hạn vô định dạng
0
0
, trong đ t thc và mu thc có cha căn thc.
Phương php giải:
Nhân lượng liên hợp để khử dạng vô định.
VÍ DỤ
Ví dụ 1. Tính giới hạn
6
33
lim
6
x
x
B
x
→
−+
=
−
. Đs:
1
6
B =−
.
Li giải
Ta có:
( )( )
( )
( )
66
3 3 3 3
33
lim lim
6
6 3 3
xx
xx
x
B
x
xx
→→
− + + +
−+
==
−
− + +
( )
( )
( )
( )
( )
666
93
6 1 1 1
lim lim lim
6
3 3 3 6 3
6 3 3 6 3 3
xxx
x
x
x
x x x x
→→→
−+
− − −
= = = = = −
+ + + +
− + + − + +
Ví dụ 2. Tính giới hạn
3
2
3 2 5 6
lim
2
x
xx
E
x
→
+ − −
=
−
. Đs:
1E =−
.
Li giải
Ta có
3
3
2 2 2
3 2 2 2 5 6
3 2 2 2 5 6
lim lim lim
2 2 2
x x x
AB
xx
xx
E
x x x
3
22
2
3
3
3 2 8
3 2 2
lim lim
2
2 3 2 2. 3 2 4
xx
x
x
A
x
x x x
2
22
2
3
3
3
3
32
31
lim lim
4
3 2 2. 3 2 4
2 3 2 2. 3 2 4
xx
x
xx
x x x
2 2 2
4 5 6 5 2
2 5 6
lim lim lim
2
2 2 5 6 2 2 5 6
x x x
xx
x
B
x
x x x x
2
55
lim
4
2 2 5 6
x
xx
Suy ra
15
1
44
E A B
.
Ví dụ 3. Tính giới hạn
3
1
5 3 2
lim
1
x
x
L
x
→−
−+
=
+
. Đs:
5
12
L =
.
Li giải
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
9
Ta có:
3
11
2
3
3
5 3 8
5 3 2
lim lim
1
1 5 3 2. 5 3 4
xx
x
x
L
x
x x x
2
11
2
3
3
3
3
51
55
lim lim
12
5 3 2. 5 3 4
1 5 3 2. 5 3 4
xx
x
xx
x x x
.
Ví dụ 4. Tính giới hạn
3
2
3 2 3 2
lim
2
x
xx
E
x
→
+ − −
=
−
. Đs:
1
2
E
−
=
.
Li giải
Ta có
3
3
2 2 2
3 2 2 3 2 2
3 2 2 3 2 2
lim lim lim
2 2 2
x x x
xx
xx
E
x x x
22
2
3
3
3 2 8 3 2 4
lim lim
2 3 2 2
2 3 2 2. 3 2 4
xx
xx
xx
x x x
22
2
3
3
3 2 3 2
lim lim
2 3 2 2
2 3 2 2. 3 2 4
xx
xx
xx
x x x
2
22
3
3
3 3 1 3 1
lim lim
4 4 2
3 2 2
3 2 2. 3 2 4
xx
x
xx
.
Ví dụ 5. Tính giới hạn
3
0
1 2 . 1 4 1
lim
x
xx
F
x
→
+ + −
=
. Đs:
7
F
3
=
.
Li giải
3
3
00
1 2 . 1 4 1 1 2 1
1 2 . 1 4 1
lim lim
xx
x x x
xx
F
xx
3
00
1 2 . 1 4 1
1 2 1
lim lim
xx
xx
x
xx
00
2
3
3
1 2 . 1 4 1
1 2 1
lim lim
1 2 1
1 4 1 4 1
xx
xx
x
xx
x x x
2
00
3
3
4. 1 2 2 4 7
lim lim 1
33
1 2 1
1 4 1 4 1
xx
x
x
xx
.
BÀI TẬP ÁP DỤNG
Bài 1. Tính các giới hạn sau:
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
10
1)
8
8
lim
31
x
x
B
x
. Đs:
6B
2)
2
1
42
lim
1
x
xx
B
x
. Đs:
1
4
B
3)
2
3
23
lim
26
x
x x x
B
x
. Đs:
1
4
B
4)
2
2
22
lim
4
x
x
B
x
. Đs:
1
16
B
5)
2
2
2 3 2
lim
4
x
x
B
x
. Đs:
3
16
B
6)
2
9
3
lim
9
x
x
B
xx
. Đs:
1
54
B
7)
2
2
22
lim
2 10
x
x
B
xx
. Đs:
1
36
B
8)
2
1
7 2 2
lim
1
x
xx
B
x
. Đs:
1
3
B
9)
2
2
1
2 5 2 8
lim
32
x
x x x
B
xx
. Đs:
5
2
B
Bài 2. Tính các giới hạn sau:
1)
1
3 1 3
lim
83
x
xx
B
x
. Đs:
3B
2)
1
32
lim
4 5 3 6
x
x
B
xx
. Đs:
3
2
B
3)
2
22
lim
13
x
xx
B
xx
. Đs:
1
4
B
4)
3
1 3 5
lim
2 3 6
x
xx
B
xx
. Đs:
3B
5)
2
4
1
21
lim
x
x x x
B
xx
. Đs:
0B
6)
4
1
4 3 1
lim
1
x
x
B
x
. Đs:
1B
7)
2
2
2
2 1 2 5
lim
13
x
xx
B
xx
. Đs:
25
3
B
Bài 3. Tính các giới hạn sau:
1)
0
9 16 7
lim
x
xx
L
x
. Đs:
7
24
B
2)
1
2 2 5 4 5
lim
1
x
xx
L
x
. Đs:
4
3
B
3)
3
2 6 2 2 8
lim
3
x
xx
L
x
. Đs:
5
6
L
4)
2
2
2 1 8
lim
2
x
x x x
L
x
. Đs:
8L
5)
6
5 4 2 3 84
lim
6
x
x x x
L
x
. Đs:
74
3
L
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
11
6)
0
1 4 1 6 1
lim
x
xx
L
x
. Đs:
5L
7)
2
0
4 3 2 1 3 1
lim
21
x
x x x
L
xx
. Đs:
5
2
L
8)
2
1
3 7 4 3 2 2 1
lim
21
x
x x x
L
xx
. Đs:
17
16
L
9)
2
0
4 4 9 6 5
lim
x
xx
L
x
. Đs:
5
12
L
10)
2
2
1
6 3 2 5
lim
1
x
x x x
L
x
. Đs:
11
6
L
Bài 4. Tính các giới hạn sau:
1)
3
2
42
lim
2
x
x
L
x
. Đs:
1
3
L
2)
3
0
11
lim
x
x
L
x
. Đs:
1
3
L
3)
3
2
3
12
lim
3
x
x
L
x
. Đs:
1
2
L
4)
3
1
72
lim
1
x
x
L
x
. Đs:
1
6
L
5)
3
8
2
lim
2 9 5
x
x
L
x
. Đs:
5
12
L
6)
3
3
1
1
lim
21
x
x
L
x
. Đs:
1L
7)
3
3
2
1
10 2 1
lim
32
x
xx
L
xx
. Đs:
3
2
L
8)
3
2
2
8 11 7
lim
32
x
xx
L
xx
. Đs:
7
54
L
9)
32
3
1
73
lim
1
x
xx
L
x
. Đs:
1
4
L
10)
3
0
2 1 8
lim .
x
xx
L
x
Đs:
11
12
L
11)
2
3
2
2
2 4 11 7
lim .
4
x
x x x
L
x
Đs:
5
72
L
12)
3
2
0
4 . 8 3 4
lim .
x
xx
L
xx
Đs:
1L
Bài 5. Tính các giới hạn sau:
1)
0
11
lim .
n
x
ax
F
x
Đs:
a
n
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
12
2)
0
11
lim .
nm
x
ax bx
F
x
Đs:
ab
nm
3)
0
11
lim ( 0).
11
n
m
x
ax
F ab
bx
Đs:
am
bn
4)
0
11
lim .
11
nm
x
ax bx
F
x
Đs:
2
ab
nm
LỜI GIẢI
Bài 1. 1)
8 8 8
8 3 1 8 3 1
8
lim lim lim
91
31
3 1 3 1
x x x
x x x x
x
B
x
x
xx
88
8 3 1
lim lim 3 1 6
8
xx
xx
x
x
.
2)
22
2
11
2
4 2 4 2
42
lim lim
1
1 4 2
xx
x x x x
xx
B
x
x x x
2
2
111
22
1
4 4 1
lim lim lim
4
42
1 4 2 1 4 2
xxx
xx
x x x
xx
x x x x x x
.
3)
22
2
33
2
2 3 2 3
23
lim lim
26
2 6 2 3
xx
x x x x x x
x x x
B
x
x x x x
33
22
3
1
lim lim
4
2 3 2 3 2 2 3
xx
xx
x
x x x x x x x
.
4)
2
2
22
2 2 2 2
22
lim lim
4
4 2 2
xx
xx
x
B
x
xx
2
2
lim
2 2 2 2
x
x
x x x
2
11
lim
16
2 2 2
x
xx
.
5)
2
22
2 2 2
2 3 2 2 3 2
4 3 2
2 3 2
lim lim lim
4
4 2 3 2 4 2 3 2
x x x
xx
x
x
B
x
x x x x
22
32
33
lim lim
16
2 2 2 3 2 2 2 3 2
xx
x
x x x x x
.
6)
2
2
9 9 9
33
39
lim lim lim
9
9 3 9 3
x x x
xx
xx
B
xx
x x x x x x
9
11
lim
54
3
x
xx
.
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
13
7)
2
22
2 2 2
lim lim
2 10
2 2 5 2 2
xx
xx
B
xx
x x x
2
11
lim
36
2 5 2 2
x
xx
.
8)
2
2
2
11
7 2 2
7 2 2
lim lim
1
1 7 2 2
xx
xx
xx
B
x
x x x
2
2
1
23
lim
1 7 2 2
x
xx
x x x
1
13
lim
1 1 7 2 2
x
xx
x x x x
1
31
lim
3
1 7 2 2
x
x
x x x
.
9)
2
2
2
2
11
22
2 5 2 8
2 5 2 8
lim lim
32
3 2 2 5 2 8
xx
x x x
x x x
B
xx
x x x x x
2
11
2 2 2
1 2 17
2 19 17
lim lim
3 2 2 5 2 8 1 2 2 5 2 8
xx
xx
xx
x x x x x x x x x x
1
2
2 17
5
lim
2
2 2 5 2 8
x
x
x x x x
.
Bài 2. 1)
1 1 1
2 1 8 3 2 8 3
3 1 3
lim lim lim 3
8 3 3 1 3
1 3 1 3
x x x
x x x
xx
B
x x x
x x x
2)
1
32
lim
4 5 3 6
x
x
B
xx
1
1 4 5 3 6
lim
1 3 2
x
x x x
xx
1
4 5 3 6
lim
32
x
xx
x
3
2
.
3)
2
22
lim
13
x
xx
B
xx
2
2 1 3
lim
2 2 2 2
x
x x x
x x x
2
13
lim
2 2 2
x
xx
xx
1
4
.
4)
3
1 3 5
lim
2 3 6
x
xx
B
xx
3
2 3 2 3 6
lim
3 1 3 5
x
x x x
x x x
3
2 2 3 6
lim
1 3 5
x
xx
xx
3
.
5)
2
4
1
21
lim
x
x x x
B
xx
2
1
22
21
lim
1 2 2 1
x
x x x
x x x x x x x
2
1
22
1
lim
1 2 2 1
x
x
x x x x x x x
1
22
1
lim
2 2 1
x
x
x x x x x x
0
.
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
14
6)
4
1
4 3 1
lim
1
x
x
B
x
1
32
4
44
41
lim
1 4 3 4 3 4 3 1
x
x
x x x x
32
1
4
44
4
lim
4 3 4 3 4 3 1
x
x x x
1
.
7)
2
2
2
2 1 2 5
lim
13
x
xx
B
xx
22
2
22
2 2 4 1 3
lim
2 2 1 2 5
x
x x x x
x x x x
2
2
2
2 1 3
lim
2 1 2 5
x
xx
xx
25
3
.
Bài 3. 1)
0
9 16 7
lim
x
xx
L
x
0
9 3 9 4
lim
x
xx
x
0
9 3 16 4
lim
x
xx
xx
x
0
11
lim
9 3 16 4
x
xx
7
24
.
2)
1
2 2 5 4 5
lim
1
x
xx
L
x
1
2 2 2 5 4 3
lim
x
xx
x
1
2 1 5 1
2 2 2 5 4 3
lim
1
x
xx
xx
x
1
25
lim
2 2 2 5 4 3
x
xx
4
3
.
3)
3
2 6 2 2 8
lim
3
x
xx
L
x
3
2 6 6 2 2 2
lim
3
x
xx
x
3
6 9 2 2 4
2
6 3 2 2 2
lim
3
x
xx
xx
x
3
33
22
6 3 2 2 2
lim
3
x
xx
xx
x
3
22
lim
6 3 2 2 2
x
xx
5
6
.
4)
2
2
44
2 2 2
21
lim
2
x
xx
x x x
xx
x
2
2
2
2 2 2
21
lim
2
x
x
x x x
xx
x
2
2
lim 2 2
21
x
x
xx
xx
8
.
5)
6
5 4 2 3 84
lim
6
x
x x x
L
x
6
5 4 2 3 3 5 4 16 96
lim
6
x
x x x x
x
2
2
2 1 8
lim
2
x
x x x
L
x
22
2
2 1 2 4
lim
2
x
x x x x
x
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
15
6
5 4 2 3 3 16 6
lim
6
x
x x x
x
6
26
5 4 16 6
2 3 3
lim
6
x
x
xx
x
x
6
10 8
lim 16
2 3 3
x
x
x
74
3
.
6)
0
1 4 1 6 1
lim
x
xx
L
x
2
0
24 10 1 1
lim
x
xx
x
2
0
2
24 10 1 1
lim
24 10 1 1
x
xx
x x x
0
2
24 10
lim
24 10 1 1
x
xx
x x x
2
0
24 10
lim
24 10 1 1
x
x
xx
5
.
7)
2
1
4 3 2 1 3 1
lim
21
x
x x x
L
xx
22
1
4 3 2 1
21
lim
11
x
xx
xx
xx
2
2
22
1
4 3 2 1
21
lim
1 2 1 1 4 3 2 1
x
xx
xx
x x x x x x
1
14
lim
2 1 4 3 2 1
x
x x x x
5
2
.
8)
2
1
3 7 4 3 2 2 1
lim
21
x
x x x
L
xx
2
1
4 3 7 2 2 1 2
lim
1
x
x x x x
x
2
2
2
1
4 2 1 4
16 48 14 49
2 2 2 1
7 4 3
lim
1
x
xx
x x x
xx
xx
x
22
2
1
1 4 1
2 2 2 1
7 4 3
lim
1
x
xx
xx
xx
x
1
14
lim
7 4 3 2 2 2 1
x
x x x x
17
16
.
9)
2
0
4 4 9 6 5
lim
x
xx
L
x
2
0
4 4 2 9 6 3
lim
x
x x x x
x
22
2
0
4 4 4 4 9 6 6 9
4 4 2 9 6 3
lim
x
x x x x x x
x x x x
x
22
2
0
2 4 3 9 6 3
lim
x
xx
x x x x
x
0
11
lim
2 4 4 9 6 3
x
x x x x
5
12
.
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
16
10)
2
2
1
6 3 2 5
lim
1
x
x x x
L
x
2
2
1
2 2 1 6 3
lim
1
x
x x x
x
2
2
2
1
6 3 4 4
21
6 3 2
lim
1
x
x x x
x
xx
x
2
2
2
1
1
21
6 3 2
lim
1
x
x
x
xx
x
1
1
lim 2
6 3 2
x
xx
11
6
.
Bài 4. 1)
3
2
42
lim
2
x
x
L
x
2
3
2
3
48
lim
2 16 2 4 4
x
x
x x x
3
2
2
3
4
lim
16 2 4 4
x
xx
1
3
.
2)
3
0
11
lim
x
x
L
x
0
2
3
3
11
lim
1 1 1
x
x
x x x
2
0
3
3
1
lim
1 1 1
x
xx
1
3
.
3)
3
2
3
12
lim
3
x
x
L
x
2
3
2
3
22
3
9
lim
3 1 2 1 4
x
x
x x x
2
3
3
22
3
3
lim
1 2 1 4
x
x
xx
1
2
.
4)
3
1
72
lim
1
x
x
L
x
1
2
3
3
1
lim
1
. 7 2 7 4
1
x
x
x
xx
x
2
1
3
3
1
lim
7 2 7 4
x
x
xx
1
6
.
5)
3
8
2
lim
2 9 5
x
x
L
x
3
2
3
8
8
24
lim
2 16
2 9 5
x
x
xx
x
x
8
2
3
3
2 9 5
lim
2 2 4
x
x
xx
5
12
.
6)
3
3
1
1
lim
21
x
x
L
x
3
2
3
1
2
3
3
1
1
lim
1
2 2 1
x
x
xx
x
xx
2
3
3
3
2
1
3
2 2 1
lim
1
x
xx
xx
1
.
7)
3
3
2
1
10 2 1
lim
32
x
xx
L
xx
3
3
1
10 2 2 1
lim
12
x
xx
xx
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
17
3
2
33
3
3
1
22
1
10 2 2 10 2 4
lim
12
x
x
x
xx
xx
2
2
33
3
3
1
2 1 1
1
10 2 2 10 2 4
lim
12
x
x x x
x
xx
xx
2
2
33
3
3
1
21
1
10 2 2 10 2 4
lim
2
x
xx
xx
x
3
2
.
8)
3
2
2
8 11 7
lim
32
x
xx
L
xx
3
2
2
8 11 3
lim
32
x
x
xx
2
2
73
lim
32
x
x
xx
2
2
3
3
8 11 27
lim
1 2 8 11 3 8 11 9
x
x
x x x x
2
79
lim
1 2 7 3
x
x
x x x
2
2
3
3
8
lim
1 8 11 3 8 11 9
x
x x x
2
1
lim
1 7 3
x
xx
8 1 7
27 7 54
.
9)
3 2 3 2
33
1 1 1
7 3 7 2 3 2
lim lim lim
1 1 1
x x x
x x x x
L
x x x
32
11
22
33
3
3
7 8 3 4
lim lim
1 3 2
1 7 2 7 4
xx
xx
xx
x x x
32
11
22
33
3
3
11
lim lim
1 3 2
1 7 2 7 4
xx
xx
xx
x x x
2
22
11
33
3
3
1 1 1 1 1
lim lim
4 2 4
32
7 2 7 4
xx
x x x
x
xx
.
10)
33
0 0 0
2 1 8 2 1 2 8 2
lim lim lim
x x x
x x x x
L
x x x
00
2
3
3
4 1 4
88
lim lim
2 1 2
8 2 8 4
xx
x
x
xx
x x x
2
00
3
3
4 1 1 11
lim lim 1
12 12
2 1 2
8 2 8 4
xx
x
xx
.
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
18
11)
22
33
2 2 2
2 2 2
2 4 11 7 2 4 11 3 7 3
lim lim lim
4 4 4
x x x
x x x x x x
L
x x x
2
3
2
22
2
2 2 2
3
3
2 4 11 27 7 9
lim lim
4 7 3
4 2 4 11 3 2 4 11 9
xx
x x x
xx
x x x x x
2
22
2
2 2 2
3
3
2 2 4
2
lim lim
4 7 3
4 2 4 11 3 2 4 11 9
xx
xx
x
xx
x x x x x
22
2
22
3
3
24
1
lim lim
2 7 3
2 2 4 11 3 2 4 11 9
xx
x
xx
x x x x x
1 1 5
9 24 72
12)
3
3
22
00
4 . 8 3 2 2 4 4
4 . 8 3 4
lim lim
xx
x x x
xx
L
x x x x
3
22
00
00
2
3
3
00
2
3
3
4 . 8 3 2
2 4 4
lim lim
4 . 8 3 8 2 4 4
lim lim
1 4 2
1 8 3 2 8 3 4)
4 .3 2
lim lim
1 4 2
1 8 3 2 8 3 4
11
1.
22
xx
xx
xx
xx
x
x x x x
x x x
x x x
x x x x
x
xx
x x x
Bài 5. 1)
00
12
1 1 1 1
lim lim
1 1 ... 1 1
n
xx
nn
n
nn
ax ax
F
x
x ax ax ax
12
0
lim .
1 1 ... 1 1
nn
x
n
nn
aa
n
ax ax ax
2)
00
1 1 1 1
11
lim lim
nm
nm
xx
ax bx
ax bx
F
xx
00
1 1 1 1
lim lim .
nm
xx
ax bx a b
x x n m
3)
00
1 1 1 1 1
lim lim .
1 1 1 1
nn
mm
xx
ax ax
F
x
bx bx
x
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
19
Xét
0
11
lim ;
n
x
ax a
A
xn
0
11
lim
m
x
bx b
B
xm
1
..
a am
F
b
n bn
m
4)
00
1 1 1 1
11
lim lim
1 1 1 1
nm
nm
xx
ax bx
ax bx
F
xx
00
1 1 1 1
lim lim
1 1 1 1
nm
xx
ax bx
xx
00
1 1 1 1
lim . lim .
1 1 1 1
nm
xx
ax x bx x
xx
xx
Ta có
0
11
lim
n
x
ax a
A
xn
0
11
lim
m
x
bx b
B
xm
0 0 0
11
lim lim lim 1 1 2
11
11
x x x
xx
x
Cx
x
x
.2 .2 2
a b a b
F
n m n m
.
Dạng 3. Giới hạn của hàm số khi
x →
.
Phương php giải:
- Đối với dạng đa thức không căn, ta rút bậc cao và áp dụng công thức khi
x →+
1.
lim
k
x
x
→+
= +
2.
2
lim
21
k
x
khik l
x
khik l
→−
+ =
=
− = +
3.
lim 0
k
x
c
x
→+
=
(c hằng số)
- Đối với dạng phân số không căn, ta làm tương tự như giới hạn dãy số, tức rút bậc cao nhất của tử
và mẫu, sau đó áp dụng công thức trên.
- Ngoài việc đưa ra khỏi căn bậc chẵn cần có trị tuyệt đối, học sinh cần phân biệt khi nào đưa ra ngoài
căn, khi nào liên hợp. Phương pháp suy luận cũng tương tự như giới hạn của dãy số, nhưng cần phân
biệt khi
x →+
hoặc
x → −
VÍ DỤ
Ví dụ 1. Tính giới hạn
( )
32
lim 6 9 1 .
x
A x x x
→+
= − − + +
Đs: .
Li giải
3
23
6 9 1
lim 1
x
Ax
x x x
(vì
3
lim
x
x
và
23
6 9 1
lim 1 1
x
x x x
).
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
20
Ví dụ 2. Tính giới hạn
3
23
31
lim
2 6 6
x
xx
B
xx
. Đs:
1
6
.
Li giải
3
23
23
3
3
3
31
31
1
1
1 0 0 1
lim lim
26
26
0 0 6 6
6
6
xx
x
xx
xx
B
x
xx
xx
.
Ví dụ 2. Tính giới hạn
2
lim 1 2
x
C x x x
. Đs: .
Li giải
2
22
1 1 1 1
lim 1 2 lim 1 2
xx
C x x x x
x x x x
→− →−
= + + + = + + +
22
1 1 1 1
lim 1 2 lim 2 1
xx
x x x
x x x x
→− →−


= − + + + = − + + = −





(Vì
lim
x
x
và
2
11
lim 2 1 2 1 1 0
x
xx
).
BÀI TẬP ÁP DỤNG
Bài 1. Tính các giới hạn sau:
1)
32
lim 3 2
x
A x x
. Đs: . 2)
32
lim 3 1
x
A x x
. Đs: .
3)
42
lim 2 1
x
A x x
. Đs: . 4)
42
lim 2 3
x
A x x
. Đs: .
5)
42
lim 6
x
A x x
. Đs: .
Bài 2. Tính các giới hạn sau:
1)
18
lim
21
x
x
B
x
. Đs:
4B
.
2)
2
lim
1
x
x
B
x
. Đs:
1B
.
3)
43
4
2 7 15
lim
1
x
xx
B
x
. Đs:
2B
.
4)
3
32
2 3 4
lim
1
x
xx
B
xx
. Đs:
2B
.
5)
2
3
37
lim
21
x
xx
B
x
. Đs:
0B
.
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
21
6)
32
2
lim
3 4 3 2
x
xx
B
xx
. Đs:
2
9
B
.
7)
34
7
4 3 2 1
lim
22
x
xx
B
x
. Đs:
8B
.
8)
20 30
50
2 3 3 2
lim
12
x
xx
B
x
. Đs:
30
3
2
B
.
9)
2
33
lim
4
x
xx
B
x
. Đs:
B
.
10)
3
2 2 3
lim
5
x
xx
B
x
. Đs:
B
.
Bài 3. Tính các giới hạn sau:
1)
2
lim 3 2 10
x
C x x x
. Đs:
17
2
.
2)
42
21
lim
12
x
xx
C
x
. Đs: .
3)
2
lim 4 4 1 2 13
x
C x x x
. Đs: 14.
4)
2
lim 5
x
C x x x
. Đs:
9
2
.
5)
2
lim 2 1
x
C x x
. Đs: .
6)
2
lim 4 2021
x
C x x x
. Đs:
2019
.
7)
22
lim 1
x
C x x x
. Đs:
1
2
.
8)
2
23
lim
5
x
x
C
xx
. Đs: -2.
9)
4 2 2
lim 4 3 1 2
x
C x x x
. Đs:
3
4
.
10)
2
2
lim
23
x
x x x
C
x
. Đs:
1
2
.
11)
2
lim 1 1
x
C x x x
. Đs:
3
2
.
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
22
12)
2
lim
10
x
x x x
C
x
. Đs:
2
.
13)
22
lim 4 9 21 4 7 13
x
C x x x x
. Đs:
1
2
14)
22
2
2
4 3 7 1
lim
2 1 . 3
x
x x x x
C
x x x
. Đs: 1.
15)
2
lim 4 4 1 2 3 .
x
C x x x
Đs: 4
16)
3
3
lim 1 .
53
x
x
Cx
xx
Đs: -1
17)
2
lim 16 3 4 5 .
x
C x x x
Đs:
43
8
18)
3
52
2
lim .
3
x
xx
Cx
xx
Đs:
2
19)
2
lim 3 1
x
C x x x
. Đs:
5
2
Bài 4. Tính các giới hạn sau:
1)
3
52
2
lim .
3
x
xx
x
xx
. Đs:
2
.
2)
2
23
lim
5
x
x
xx
. Đs: 2.
3)
2
2
2 3 1
lim
4 1 1
x
x x x
xx
. Đs: 4.
4)
4 2 2
23
lim
52
x
x x x x
xx
Đs:
21
2
.
5)
2
2
2 1 3 4 5
lim
1 3 2 9 10
x
x x x
x x x
. Đs:
8
3
.
6)
3
23
3 1 1 8
lim
69
x
xx
x
. Đs:
1
6
.
7)
2
2
2 1 3
lim
5
x
xx
xx
. Đs:
2
5
.
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
23
8)
2
2
4 3 1
lim
9 3 5 3
x
x x x
x x x
. Đs:
1
4
9)
22
21
lim
x
x
x x x x
. Đs: 1.
10)
2
83
lim
6 4 3
x
x
x x x
. Đs: 2.
11)
2
2
1 7 2
lim
3 2 5 3
x
xx
x x x
. Đs:
1
.
12)
2 1 2
lim
1
x
xx
x
. Đs:
1
.
Bài 5. Tính các giới hạn sau:
2
1) lim
x
x x x
. Đs: .
2
2) lim 4
x
x x x
. Đs:
2
.
3) lim 2 2
x
xx
. Đs: 0.
22
4) lim 1
x
x x x
. Đs:
1
2
.
2
5) lim 4 1 2
x
x x x
. Đs: 0.
2
6) lim 3 5 1
x
x x x
. Đs:
5
2
.
3
32
7) lim 27 3
x
x x x
. Đs:
1
27
.
2
8) lim 2 4 2 1
x
x x x
. Đs:
1
2
.
2
9) lim 2 3 4 4 3
x
x x x
. Đs:
4
.
4 2 2
10) lim 4 3 1 2
x
x x x
. Đs:
3
4
.
2
11) lim 4 3 1 2 4
x
x x x
. Đs:
19
4
.
2
12) lim 4 4 1 2 3
x
x x x
. Đs:
.
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
24
3
23
2
4
13) lim
2 4 3
x
x x x
x x x
. Đs:
16
9
.
3
3
14) lim 8 1 2 1
x
xx
. Đs:
1
.
LỜI GIẢI
Bài 1. 1)
3
3
32
lim 1
x
Ax
xx
, (vì
3
lim
x
x
và
3
32
lim 1 1 0
x
xx
).
2)
33
33
3 1 3 1
lim 1 , ì lim à lim 1 1 0
x x x
A x v x v
x x x x
.
3)
44
2 4 2 4
2 1 2 1
lim 1 , ì lim à lim 1 1 0
x x x
A x v x v
x x x x
.
4)
44
2 4 2 4
2 3 2 3
lim 1 , ì lim à lim 1 1 0
x x x
A x v x v
x x x x
.
5)
44
2 4 2 4
1 6 1 6
lim 1 , ì lim à lim 1 1 0
x x x
A x v x v
x x x x
.
Bài 2. 1)
1
1
8
8
1 8 0 8
lim lim lim 4
1
1
2 1 2 0
2
2
x x x
x
x
x
x
B
x
x
x
x
.
2)
2
2
1
1
2 1 0
lim lim lim 1
1
1
1 1 0
1
1
x x x
x
x
x
x
B
x
x
x
x
.
3)
4
43
4
4
4
4
4
4
7 15
7 15
2
2
2 7 15 2 0 0
lim lim lim 2
1
1
1 1 0
1
1
x x x
x
xx
xx
xx
B
x
x
x
x
.
4)
3
3
2 3 2 3
32
3
33
3 4 3 4
22
2 3 4 2 0 0
lim lim lim 2
1 1 1 1
1 1 0 0
11
x x x
x
xx
x x x x
B
xx
x
x x x x
.
5)
2
3
37
lim
21
x
xx
B
x
3
2 3 2 3
3
33
3 1 7 3 1 7
0 0 0
lim lim 0
11
20
22
xx
x
x x x x x x
x
xx
.
6)
32
2
lim
3 4 3 2
x
xx
B
xx
2
2
24
lim
3 4 3 2
x
xx
xx
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
25
3
3
22
44
22
lim lim
4 2 4 2
3 3 3 3
xx
x
xx
x
x x x x
2 0 2
3 0 3 0 9
7)
34
7
4 3 2 1
lim
22
x
xx
B
x
34
34
77
31
42
4 0 2 0
lim 8
3 2 0
2
x
xx
x
.
8)
20 30
50
2 3 3 2
lim
12
x
xx
B
x
20 30
20 30
30
50 50
32
23
2 0 3 0
3
lim
2
1 2 0
2
x
xx
x
.
9)
2
33
lim
4
x
xx
B
x
2
2
2
13
13
3
3
lim lim . ,
4
4
1
1
xx
x
xx
xx
x
x
x
x
2
13
3
ì lim à lim 3
4
1
xx
xx
v x v
x
.
10)
3
2 2 3
lim
5
x
xx
B
x
3
23
23
2
23
23
2
2
lim lim . ,
5
5
1
1
xx
x
xx
xx
x
x
x
x
23
2
23
2
ì lim à lim 2
5
1
xx
xx
v x v
x
.
Bài 3. 1)
2
lim 3 2 10
x
C x x x
2
10 lim 3 2
x
x x x
2
32
10 lim
32
x
x
x x x
2
32
10 lim
32
x
x
x x x
2
2
3
10 lim
32
11
x
x
xx
3 17
10
22
.
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
26
2)
42
21
lim
12
x
xx
C
x
2
2 4 2 4
1 1 1 1
22
lim lim
1
1
2
2
xx
x
x x x x
x
x
x
x
,
24
11
2
2
ì lim à lim 0
1
2
2
xx
xx
v x v
x
3)
2
lim 4 4 1 2 13
x
C x x x
2
13 lim 4 4 1 2
x
x x x
22
2
4 4 1 4
13 lim
4 4 1 2
x
x x x
x x x
2
2
41
13 lim
41
42
x
x
xx
xx
2
1
4
13 lim
41
42
x
x
x
xx
xx
2
1
4
13 lim
41
42
x
x
x
xx
xx
2
1
4
13 lim 14
41
42
x
x
xx
4)
2
lim 5
x
C x x x
11
5 lim 1 5 lim 1 1
xx
x x x
xx
1
11
19
5 lim 5 lim
2
11
1 1 1 1
xx
x
x
xx
.
5)
2
lim 2 1
x
C x x
22
11
lim 2 lim 1 2
xx
x x x
xx
,
2
1
ì lim 1 2 1 2 0 à lim
xx
v v x
x
.
6)
2
lim 4 2021
x
C x x x
44
2021 lim 1 2021 lim 1 1
xx
x x x
xx
4
11
44
2021 lim 2021 lim 2021 2019
2
44
1 1 1 1
xx
x
x
xx
.
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
27
7)
22
22
1
lim 1 lim
1
xx
x
C x x x
x x x
22
2
11
lim lim
1 1 1 1
1 1 1 1
1
1
1
lim
2
11
11
xx
x
xx
x x x x
x x x x
x
xx
8)
2
23
lim
5
x
x
C
xx
22
2
3
23
20
lim lim 2
1 5 1 5 1 0 0
11
xx
x
x
xx
x
x x x x
.
9)
4 2 2
lim 4 3 1 2
x
C x x x
22
24
31
lim 4 2
x
xx
xx
2 4 2
2
2 4 2 4
3 1 1
4 4 3
3
lim lim
4
3 1 3 1
4 2 4 2
xx
x x x
x
x x x x
.
10)
2
2
lim
23
x
x x x
C
x
1
12
lim
23
x
xx
x
x
1
1
12
12
1 2 1
lim lim
3
3
22
2
2
xx
x
x
x
x
x
x
.
11)
2
lim 1 1
x
C x x x
2
11
1 lim 1
x
xx
xx
2
11
1 lim 1 1
x
x
xx
2
2
11
11
1 lim
11
11
x
xx
x
xx
2
1
1
13
1 lim 1
22
11
11
x
x
xx
.
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
28
12)
2
lim
10
x
x x x
C
x
11
11
11
lim lim 2
10
10 1
1
xx
x
xx
x x x
x
x
.
13)
22
lim 4 9 21 4 7 13
x
C x x x x
22
9 21 7 13
lim 4 4
x
xx
x x x x
22
22
9 21 7 13
44
lim
9 21 7 13
44
x
x x x x
x
x x x x
22
34
2
21
lim
2 2 2
9 21 7 13
44
x
x
x x x x
.
14)
22
2
2
4 3 7 1
lim
2 1 . 3
x
x x x x
C
x x x
3
2 3 2 3
22
2
2
44
3 7 1 3 7 1
4
lim lim 1
2 .1
1 3 1 3
2 . 1 2 . 1
xx
xx
x
x x x x x x x x
xx
x x x x
15)
2
lim 4 4 1 2 3
x
C x x x
2
41
3 lim 4 2
x
xx
xx
2
2
41
44
3 lim
41
42
x
xx
x
xx
2
1
4
4
3 lim 3 4
4
41
42
x
x
xx
.
16)
3
3
lim 1
53
x
x
Cx
xx
2
3 2 2
33
11
1 1 0 1
lim 1 lim 1 1. 1
5 3 5 3
0 0 1
11
xx
xx
x
xx
x
x x x x
.
17)
2
lim 16 3 4 5
x
C x x x
33
5 lim 16 4 5 lim 16 4
3
16 16
3 3 3 43
5 lim 5 lim 5 5 .
4 4 8 8
33
16 4 16 4
xx
xx
x x x
xx
x
x
xx
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
29
18)
3
52
2
lim
3
x
xx
Cx
xx
3
2
2
5
35
35
1
1
2
2
lim lim 2.
13
13
1
1
xx
x
x
x
x
x
xx
xx
19)
2
lim 3 1
x
C x x x
2
11
3 lim 1
x
xx
xx
2
11
3 lim 1 1
x
x
xx
2
2
11
11
3 lim
11
11
x
xx
x
xx
2
1
1
15
3 lim 3
22
11
11
x
x
xx
.
Bài 4.
1)
33
5 2 2 5 2
24
1
2
22
lim . lim . . lim 1 . 2
13
33
x x x
x
x
x x x x
x
xx
x x x x x
x
xx
.
2)
2
22
3
2
23
23
lim lim lim 2
1 5 1 5
5
11
x x x
x
x
x
xx
x
x x x x
.
3)
2
22
2
22
1 2 1 2 1
1 3 1 1 3
2 3 1 1 3
lim lim lim 4
21
1 1 1
4 1 1
4 1 4 1
x x x
xx
x x x
x x x x x
xx
xx
x x x
.
4)
22
4 2 2
22
2
1 3 1 3
2 1 2 1
2 3 2 1
lim lim lim
55
5 2 2
22
x x x
xx
x x x x
x x x x
xx
x
xx
.
5)
2
22
2
22
1 5 1 1 5
2 1 3 4 2 3 4
2 1 3 4 5 8
lim lim lim
3
1 10 1 1 10
1 3 2 9 10
1 3 2 9 3 2 9
x x x
xx
x x x
x x x x x
x x x
xx
x x x x x
.
6)
3
23
2 3 2 3
1 1 1 1
3 1 8 3 1 8
3 1 1 8 1
lim lim lim
9
6 9 6 9 6
6
x x x
xx
xx
x x x x
xx
x
.
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
30
7)
2
22
13
3
2 1 1
2 1 1
2 1 3
2
lim lim lim
1
5 5 5
5
x x x
xx
xx
xx
x
x x x x
x
.
8)
2
22
2
22
3 1 3 1
4 1 4
4 3 1 1
lim lim lim
4
1 3 1 3 3
9 3 5 3
9 5 3 9 5
x x x
xx
x x x
x x x x
x x x
xx
x x x x x
.
9)
22
1
2
2 1 2 1 2
lim lim lim 1
2
1 1 1 1
1 1 1 1
x x x
xx
x
x x x x
xx
x x x x
.
10)
2
22
3
8
8 3 8 3
lim lim lim 2
1 3 1 3
6 4 3
6 4 6 4
x x x
xx
x
x x x
xx
x x x x
.
11)
2
22
2
22
1 1 2
1 7 2 1 7
1 7 2
lim lim lim 1
3 2 3 2 3
3 2 5 3
1 5 3 1 5
x x x
xx
xx
x x x
x x x
x x x
x x x x x
.
12)
22
1 2 1 2
2 1 2
2 1 2
lim lim lim 1
1
11
1
x x x
xx
xx
x x x x
xx
x
.
Bài 5.
2
11
1) lim lim 1 lim 1 1
x x x
x x x x x x
xx
.
1
ì lim à lim 1 1 2
xx
V x v
x
.
22
2
2
44
2) lim 4 lim lim 2
4
4
11
x x x
x x x x
x x x
x x x
x
x
.
22
4
3) lim 2 2 lim lim 0
22
22
11
x x x
xx
xx
xx
x
xx
.
4 1 1
ì lim 0 à lim
2
22
11
xx
Vv
x
xx
.
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
31
22
22
22
2
1
1
4) lim 1 lim lim
11
1
11
x x x
x x x
x
x x x
x x x
xx
xx
2
1
1
1
lim
2
11
11
x
x
xx
.
2
2
2
2
2
4 1 2
3
5) lim 4 1 2 lim lim
41
4 1 2
12
x x x
x x x
x x x
x x x
xx
xx
2
3
lim 0
4 1 2
11
x
x
x x x
.
2
2
2
2
2
3 5 1
54
6) lim 3 5 1 lim lim
35
3 5 1
11
x x x
x x x
x
x x x
x x x
xx
xx
2
4
5
5
lim
2
3 5 1
11
x
x
x x x
.
3 2 3
3
32
2
33
3 2 3 2 2
27 27
7) lim 27 3 lim
27 3 27 9
xx
x x x
x x x
x x x x x x
2
22
2 2 2
3 3 3 3
11
lim lim
27
1 1 1 1
27 3 27 9 27 3 27 9
xx
x
x x x
x x x x
.
22
2
2
2
4 4 2 1 2 1
8) lim 2 4 2 1 lim lim
21
2 4 2 1
24
x x x
x x x x
x x x
x x x
xx
xx
2
1
2
1
lim
2
21
24
x
x
xx
.
2
2
2
22
2 3 4 4 3
16 6
9) lim 2 3 4 4 3 lim lim
2 3 4 4 3 2 3 4 4 3
x x x
x x x
x
x x x
x x x x x x
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
32
22
6
16
16 6
lim lim 4
4 3 3 4 3
2 3 4 2 4
xx
x
x
xx
x x x x x
4 2 4 2
4 2 2
4 2 2
22
24
4 3 1 4 3 1
10) lim 4 3 1 2 lim lim
31
4 3 1 2
42
x x x
x x x x
x x x
x x x
xx
xx
2
24
1
3
3
lim
4
31
42
x
x
xx
.
2
2
2
2
2
4 3 1 2 4
19 15
11) lim 4 3 1 2 4 lim lim
31
4 3 1 2 4
4 2 4
x x x
x x x
x
x x x
x x x
xx
xx
2
15
19
19
lim
4
3 1 4
42
x
x
x x x
.
2
2
2
2
2
4 4 1 2 3
16 8
12) lim 4 4 1 2 3 lim lim
41
4 4 1 2 3
4 2 3
x x x
x x x
x
x x x
x x x
xx
xx
2
8
16
lim 4
4 1 3
42
x
x
x x x
.
13)
3
2 3 3 2 3 2
2
22
2
33
2 2 3 2 3
4 4 2 4 3
lim lim .
4 4 3
2 4 3
44
xx
x x x x x x x x x
xxx
x x x
x x x x x x
2
33
3
24
4 16
lim . .
39
44
1 1 1
x
x
xx
14)
3
3
3
3
2
2
33
33
8 1 2 1
lim 8 1 2 1 lim
8 1 2 1 8 1 2 1
xx
xx
xx
x x x x
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
33
2
2
2
33
33
12 6 2
lim
8 1 2 1 8 1 2 1
x
xx
x x x x
2
2
2
33
33
62
12
lim 1.
1 1 1 1
8 2 8 2
x
xx
x x x x
Dạng 4. Giới hạn một bên
0
xx
+
→
hoặc
0
xx
−
→
.
Phương php giải:
- Sử dụng các định lý về giới hạn hàm số
Chú ý:
0 0 0
0x x x xxx
+
−→
0 0 0
0x x x xxx
−
−→
VÍ DỤ
V dụ 1. Tính giới hạn
1
23
lim .
1
x
x
A
x
+
→
−
=
−
Đs:
.−
Li giải
Vì
1
11
lim 2 3 1 0
23
lim 1 0 lim
1
1 1 1 0
x
xx
x
x
xA
x
x x x
.
V dụ 2. Tính giới hạn
2
15
lim .
2
x
x
A
x
+
→
−
=
−
Đs:
.−
Li giải
Vì
2
22
lim 15 13 0
15
lim 2 0 lim
2
2 2 2 0
x
xx
x
x
xA
x
x x x
.
V dụ 3. Tính giới hạn
3
2
lim .
3
x
x
A
x
−
→
−
=
−
Đs:
.−
Li giải
Vì
( )
( )
3
33
lim 2 1 0
2
lim 3 0 lim
3
3 3 3 0
x
xx
x
x
xA
x
x x x
−
−−
→
→→
−
− = −
−
− = = = −
−
→ −
.
V dụ 4. Tính giới hạn
2
1
lim .
24
x
x
A
x
+
→
+
=
−
Đs:
.+
Li giải
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
34
Vì
( )
( )
2
22
lim 1 3 0
1
lim 2 4 0 lim
24
2 2 2 4 0
x
xx
x
x
xA
x
x x x
+
++
→
→→
+
+ =
+
− = = = +
−
→ −
.
V dụ 5. Tính giới hạn
( )
2
4
5
lim .
4
x
x
A
x
−
→
−
=
−
Đs:
.−
Li giải
Vì
( )
( )
( )
( )
4
2
2
44
2
lim 5 1 0
5
lim 4 0 lim
4
4 4 0
x
xx
x
x
xA
x
xx
−
−−
→
→→
−
− = −
−
− = = = −
−
→ −
.
V dụ 6. Tính giới hạn
( )
2
3
38
lim .
3
x
x
A
x
−
→
−
=
−
Đs:
.+
Li giải
Vì
( )
( )
( )
( )
3
2
2
33
2
lim 3 8 1 0
38
lim 3 0 lim
3
3 3 0
x
xx
x
x
xA
x
xx
−
−−
→
→→
−
− =
−
− = = = +
−
→ −
.
V dụ 7. Tính giới hạn
( )
( )
2
2
3
2 5 3
lim .
3
x
xx
A
x
+
→−
+−
=
+
Đs:
.−
Li giải
Ta có
( )
( )
( )
( )( )
( )
( )
2
22
3 3 3
2 1 3
2 5 3 2 1
lim lim lim
3
33
x x x
xx
x x x
x
xx
+ + +
→ − → − → −
−+
+ − −
==
+
++
Vì
( )
( )
( )
( )
( )
( )
( )
3
2
2
33
lim 2 1 7 0
2 5 3
lim 3 0 lim
3
3 3 3 0
x
xx
x
xx
xA
x
x x x
+
++
→−
→ − → −
+
− = −
+−
+ = = = −
+
→ − − +
.
V dụ 8. Tính giới hạn
2
2
11
lim .
24
x
A
xx
−
→

=−

−−

Đs:
.−
Li giải
Ta có:
( )( )
2
22
1 1 1
lim lim
2 4 2 2
xx
x
A
x x x x
−−
→→
+

= − =

− − − +

Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
35
Vì
( )
( )( )
( )( )
2
2
22
lim 1 3 0
11
lim 2 2 0 lim
24
2 2 2 2 0
x
xx
x
x x A
xx
x x x x
−
−−
→
→→
−
+ =

− + = = − = −



−−

→ − +
.
V dụ 9. Tính giới hạn
2
2
2
lim .
2 5 2
x
x
B
xx
−
→
−
=
−+
Đs:
1
.
3
−
Li giải
Vì
2 2 2 2x x x x
−
→ − = −
Do đó
( )( )
22
2 1 1
lim lim
2 2 1 2 1 3
xx
x
B
x x x
−−
→→
−−
= = = −
− − −
.
V dụ 10. Tính giới hạn
3
3
lim .
5 15
x
x
B
x
+
→
−
=
−
Đs:
1
.
5
Li giải
Vì
3 3 3 3x x x x
+
→ − = −
Do đó
( )
33
3 1 1
lim lim
5 3 5 5
xx
x
B
x
−−
→→
−
= = =
−
.
BÀI TẬP ÁP DỤNG
Bài 1. Tính các giới hạn sau:
1)
3
1
1
lim .
23
x
x
A
xx
−
→
−
=
+−
Đs:
1
.
7
−
2)
2
2
lim .
2
x
x
B
x
→
−
=
−
Đs: Không tn tại.
3)
2
3
9
lim .
3
x
x
C
x
→
−
=
−
Đs: Không tn tại.
Bài 2. Tính các giới hạn sau:
1)
2
2
1
2 2 1 3
lim .
21
x
x x x x
C
xx
−
→
− + − +
=
−+
Đs:
7
.
4
2)
2
2
lim .
11
x
x
C
x
−
→
−
=
−−
Đs:
2.−
3)
2
2
3
7 12
lim .
9
x
xx
D
x
−
→
−+
=
−
Đs:
1
.
6
4)
2
2
2
56
lim .
4
x
xx
D
x
−
→
−+
=
−
Đs:
1
.
2
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
36
5)
23
1
11
lim .
x
xx
D
xx
−
→
− + −
=
−
Đs:
1.
6)
( )
32
1
5
lim 1 .
23
x
x
Dx
xx
+
→
+
=−
+−
Đs:
0.
7)
3
2
1
32
lim .
54
x
xx
D
xx
−
→
−+
=
−+
Đs:
3
.
3
Bài 3. 1) Tính giới hạn
( )
1
lim
x
C f x
→
=
với
( )
42
3
5 6 1
.
31
x x x khi x
fx
x x khi x
− −
=
−
Đs:
2−
2) Tính giới hạn
( )
1
lim
x
C f x
→
=
với
( )
2
31
.
1 7 2 1
x khi x
fx
x khi x
−
=
− +
Đs:
2−
.
3) Tính giới hạn
( )
2
lim
x
C f x
→−
=
với
( )
32
2
.
1
10 2
x
khi x
fx
x
x khi x
−
−
=
+
+ −
Đs:
8
.
Bài 4. Tìm
m
để hàm số
( )
3
22
1
1
1
1
x
khi x
fx
x
mx x m khi x
+
−
=
+
− + −
có giới hạn tại
1.x =−
Đs:
1m =
hoặc
2m =−
.
LỜI GIẢI
Bài 1. 1)
3
1
1
lim .
23
x
x
A
xx
−
→
−
=
+−
Vì
( )
1 1 1 1 .x x x x
−
→ − = − −
Do đó
( )
( )
( )
2
2
11
1
11
lim lim .
2 2 3 7
1 2 2 3
xx
x
A
xx
x x x
−−
→→
−−
−
= = = −
++
− + +
2)
2
2
lim .
2
x
x
B
x
→
−
=
−
+) Vì
( )
2 2 2 2x x x x
−
→ − = − −
nên
( )
( )
22
2
lim lim 1 1
2
xx
x
x
−−
→→
−−
= − = −
−
.
+) Vì
2 2 2 2x x x x
+
→ − = −
nên
22
2
lim lim1 1
2
xx
x
x
−−
→→
−
==
−
.
Suy ra
22
22
lim lim
22
xx
xx
xx
−+
→→
−−
−−
nên không tn tại giới hạn của
2
2
lim .
2
x
x
B
x
→
−
=
−
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
37
3)
2
3
9
lim .
3
x
x
C
x
→
−
=
−
Ta có
3
3 . 3
lim .
3
x
xx
C
x
→
−+
=
−
Do đó:
+)
( )
2
3 3 3
9
3 . 3
lim lim lim 3 6.
33
x x x
x
xx
x
xx
+ + +
→ → →
−
−+
= = + =
−−
+)
( )
( )
2
3 3 3
9
3 . 3
lim lim lim 3 6.
33
x x x
x
xx
x
xx
− − −
→ → →
−
− − +
= = − + = −
−−
Suy ra giới hạn của
2
3
9
lim
3
x
x
C
x
→
−
=
−
không tn tại.
Bài 2. 1)
2
2
1
2 2 1 3
lim .
21
x
x x x x
C
xx
−
→
− + − +
=
−+
Vì
( )
1 1 0 1 1x x x x
−
→ − − = − −
. Do đó
( ) ( )
( )
( )
( )
2
2
1 1 1
2 1 1 3
2 3 4 3
lim lim lim
1
1
1 2 3
x x x
x x x x
x x x x
C
x
x
x x x
− − −
→ → →
− − − +
− + − −
= = =
−
−
− + +
( )( )
( )
( )
11
1 4 3
4 3 7
lim lim .
4
23
1 2 3
xx
xx
x
xx
x x x
−−
→→
−+
+
= = =
++
− + +
2)
2
2
lim .
11
x
x
C
x
−
→
−
=
−−
Vì
( )
2 2 0 2 2x x x x
−
→ − − = − −
. Do đó:
( )
( )
( )
( )
22
2 1 1
lim lim 1 1 2
11
xx
xx
Cx
x
−−
→→
− − − +

= = − − + = −

−−
.
3)
2
2
3
7 12
lim .
9
x
xx
D
x
−
→
−+
=
−
Ta có
( )( )
( )( )
3 3 3
34
3 . 4 4 1
lim lim lim .
3 . 3 3 6
33
x x x
xx
x x x
D
x x x
xx
− − −
→ → →
−−
− − −
= = = =
− + +
−+
4)
2
2
2
56
lim .
4
x
xx
D
x
−
→
−+
=
−
Ta có
( )( )
( )( )
2 2 2
23
2 . 3 3 1
lim lim lim .
2
2 . 2 2
22
x x x
xx
x x x
D
x x x
xx
− − −
→ → →
−−
− − −
= = = =
− + +
−+
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
38
5)
23
1
11
lim .
x
xx
D
xx
−
→
− + −
=
−
Ta có
( )
( )
( )
2
2
1 1 1
11
11
11
lim lim lim 1.
1
1
x x x
xx
xx
x
D
x
xx
xx
− − −
→ → →
− − −
− − −
−−
= = = =
−
−
6)
( )
32
1
5
lim 1 .
23
x
x
Dx
xx
+
→
+
=−
+−
Ta có
( ) ( )
( )
( )
( )( )
2
2
2
11
1 5 1 5
lim lim 0.
33
1 3 3
xx
x x x x
D
xx
x x x
++
→→


− + − +

= − = − =

++

− + +



7)
3
2
1
32
lim .
54
x
xx
D
xx
−
→
−+
=
−+
Ta có
( ) ( )
( )( )
( )
( )( )
2
1 1 1
12
12
23
lim lim lim .
1 4 1 4 4 3
x x x
xx
xx
x
D
x x x x x
− − −
→ → →
−+
−+
+
= = = =
− − − − −
Bài 3. 1) Ta có:
+)
( )
( )
3
11
lim lim 3 2.
xx
f x x x
−−
→→
= − = −
+)
( )
( )
42
11
lim lim 5 6 5 6 1 2.
xx
f x x x x
++
→→
= − − = − − = −
+) Vì
( ) ( )
11
lim lim 2
xx
f x f x
−+
→→
= = −
nên hàm số
( )
fx
có giới hạn tại
1x =
và
( )
1
lim 2.
x
fx
→
=−
2) Ta có:
+)
( ) ( )
11
lim lim 3 2.
xx
f x x
−−
→→
= − = −
+)
( )
(
)
2
11
lim lim 1 7 2 2.
xx
f x x
++
→→
= − + = −
+) Vì
( ) ( )
11
lim lim 2
xx
f x f x
−+
→→
= = −
nên
( )
1
lim 2.
x
C f x
→
= = −
3) Ta có:
+)
( )
( )
( )
22
32
lim lim 8.
1
xx
x
fx
x
−−
→ − → −
−
==
+
+)
( )
( )
( )
( )
22
lim lim 10 8.
xx
f x x
++
→ − → −
= + =
+)Vì
( )
( )
( )
( )
22
lim lim 8
xx
f x f x
−+
→ − → −
==
nên
( )
2
lim 8.
x
C f x
→−
==
Bài 4. Ta có:
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
39
+)
( )
( )
( ) ( )
( )
3
2
1 1 1
1
lim lim lim 1 3.
1
x x x
x
f x x x
x
− − −
→ − → − → −
+
= = − + =
+
+)
( )
( )
( )
( )
2 2 2
11
lim lim 1.
xx
f x mx x m m m
++
→ − → −
= − + = + +
+) Để hàm số có giới hạn tại
1x =−
thì
22
1
3 1 2 0 .
2
m
m m m m
m
=
= + + + − =
=−
Dạng 5. Giới hạn của hàm số lượng giác
Phương php giải:
- Sử dụng các định lý về giới hạn hàm số
- Sử dụng các công thức biến đổi lượng giác
- Lưu ý:
0
sin
lim 1
x
x
x
VÍ DỤ
V dụ 1. Tnh giới hạn
2
6
2sin 1
lim .
4cos 3
x
x
A
x
→
−
=
−
Đs:
1
.
2
A =−
Li giải
Ta có:
( )
22
2
6 6 6 6
2sin 1 2sin 1 2sin 1 1 1
lim lim lim lim .
4cos 3 1 4sin 1 2sin 2
4 1 sin 3
x x x x
x x x
A
x x x
x
→ → → →
− − − −
= = = = = −
− − +
−−
V dụ 2. Tnh giới hạn
2
4
2sin 1
lim .
2cos 1
x
x
A
x
→
−
=
−
Đs:
1
.
2
A =−
Li giải
Ta có:
( )
22
2
4 4 4 4
2sin 1 2sin 1 2sin 1 1 1
lim lim lim lim .
2cos 1 1 2sin 2
2 1 sin 1
1 2sin
x x x x
x x x
A
xx
x
x
→ → → →
− − − −
= = = = = −
−−
−−
+
V dụ 3. Tnh giới hạn
0
cos4 1
lim .
sin4
x
x
A
x
→
−
=
Đs:
0.A =
Li giải
Ta có:
2 2 2 2
00
cos4 1 cos 2 sin 2 cos 2 sin 2
lim lim
sin4 2sin2 cos2
xx
x x x x x
A
x x x
→→
− − − −
==
2
00
2sin 2 sin2
lim lim 0.
2sin2 cos2 cos2
xx
xx
x x x
→→
−−
= = =
V dụ 4. Tnh giới hạn
0
1 sin2 cos2
lim .
1 sin2 cos2
x
xx
A
xx
→
−−
=
+−
Đs:
1.A =−
Li giải
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
40
Ta có:
( )
( )
22
22
00
1 2sin cos cos sin
1 sin2 cos2
lim lim .
1 sin2 cos2
1 2sin cos cos sin
xx
x x x x
xx
A
xx
x x x x
→→
− − −
−−
==
+−
+ − −
( )
( )
2
2
0 0 0
2sin sin cos
2sin 2sin cos sin cos
lim lim lim 1.
2sin 2sin cos 2sin sin cos sin cos
x x x
x x x
x x x x x
x x x x x x x x
→ → →
−
−−
= = = = −
+ + +
BÀI TẬP ÁP DỤNG
Bài 1. Tính các giới hạn sau:
1)
0
1 sin2 cos2
lim .
1 sin2 cos2
x
xx
A
xx
→
+−
=
−−
Đs:
1.A =−
2)
0
sin2
lim .
1 sin2 cos2
x
x
A
xx
→
=
−−
Đs:
1.A =−
3)
0
sin7 sin5
lim .
sin
x
xx
A
x
→
−
=
Đs:
2.A =
4)
0
sin5 sin3
lim .
sin
x
xx
A
x
→
−
=
Đs:
2.A =
5)
0
1 cos
lim .
sin
x
x
A
x
→
−
=
Đs:
0.A =
6)
3
cos3 2cos2 2
lim .
sin3
x
xx
A
x
→
++
=
Đs:
23
.
3
A =
7)
2
1 sin 2 cos2
lim .
cos
x
xx
A
x
→
++
=
Đs:
2.A =
Bài 2. Tính các giới hạn sau:
1)
0
1 cos
lim .
1 cos
x
ax
B
bx
→
−
=
−
Đs:
2
.
a
B
b

=


2)
0
sin5
lim .
x
x
B
x
→
=
Đs:
5.B =
3)
3
0
sin5 .sin3 .sin
lim
45
x
x x x
B
x
→
=
. Đs:
1
.
3
B =
4)
2
0
1 cos
lim
x
x
B
x
→
−
=
. Đs:
1
.
2
B =
5)
0
1 cos5
lim
1 cos3
x
x
B
x
→
−
=
−
. Đs:
25
9
B =
. 6)
2
0
1 cosa
lim
x
x
B
x
→
−
=
. Đs:
2
2
a
B =
.
7)
2
0
1 cos 2
lim
sin
x
x
B
xx
→
−
=
. Đs:
4B =
. 8)
3
0
sin tan
lim
x
xx
B
x
→
−
=
. Đs:
1
.
2
B =−
9)
3
0
tan sin
lim
sin
x
xx
B
x
→
−
=
. Đs:
1
2
B =
. 10)
3
0
1 cos
lim
sin
x
x
B
xx
→
−
=
. Đs:
3
2
B =
.
Bài 3. Tính các giới hạn sau:
1)
( )
2
4
0
cos8 1 sin 3
lim
3.
x
xx
B
x
→
−
=
. Đs:
48B =−
. 2)
0
1 2 1
lim
sin2
x
x
B
x
→
−+
=
. Đs:
1
2
B
−
=
.
3)
2
0
1 cos cos2
lim
x
x
B
x
→
−
=
. Đs:
3
2
B =
. 4)
3
2
0
1 cos
lim
tan
x
x
B
x
→
−
=
. Đs:
1
6
B =
.
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
41
5)
3
2
4
tanx 1
lim
2sin 1
x
B
x
→
−
=
−
. Đs:
1
.
3
B =
6)
3
0
1 tan 1 sin
lim
x
xx
B
x
→
+ − +
=
. Đs:
1
4
B =
. 7)
( )
2
0
1 cos
lim .
11
x
x
B
x
→
−
=
−−
Đs:
2B =
.
8)
2
2
0
1 cos
lim
x
xx
B
x
→
+−
=
. Đs:
1B =
. 9)
0
1 2 1 sin
lim
3 4 2
x
xx
B
xx
→
− + +
=
+ − −
. Đs:
0B =
.
10)
3
2
0
2 1 1
lim
sin
x
xx
B
x
→
+ − +
=
. Đs:
1.
Bài 4. Tính các giới hạn sau:
1)
4
lim tan2 tan .
4
x
C x x
→


=−




Đs:
1
2
C =
2)
( )
2
1 cos
lim .
x
x
C
x
→
+
=
−
Đs:
1
2
C =
3)
( )
2
sin 1
lim .
43
x
x
C
xx
→
−
=
−+
Đs:
1
.
2
C
−
=
4)
sin sin
lim .
xa
xa
C
xa
→
−
=
−
Đs:
cos .Ca=
LỜI GIẢI
Bài 1. 1)
( )
( )
22
22
00
1 2sin cos cos sin
1 sin2 cos2
lim lim .
1 sin2 cos2
1 2sin cos cos sin
xx
x x x x
xx
A
xx
x x x x
→→
+ − −
+−
==
−−
− − −
( )
( )
2
2
0 0 0
2sin sin cos
2sin 2sin cos sin cos
lim lim lim 1.
2sin 2sin cos 2sin sin cos sin cos
x x x
x x x
x x x x x
x x x x x x x x
→ → →
+
++
= = = = −
− − −
2)
( )
22
00
sin2 2sin cos
lim lim .
1 sin2 cos2
1 2sin cos cos sin
xx
x x x
A
xx
x x x x
→→
==
−−
− − −
( )
2
0 0 0
2sin cos 2sin cos cos
lim lim lim 1.
2sin 2sin cos 2sin sin cos sin cos
x x x
x x x x x
x x x x x x x x
→ → →
= = = = −
− − −
3)
0 0 0
sin7 sin5 2cos6 .sin
lim lim lim2cos6 2
sin sin
x x x
x x x x
Ax
xx
→ → →
−
= = = =
.
4)
0 0 0
sin5 sin3 2cos4 .sin
lim lim lim2cos4 2
sin sin
x x x
x x x x
Ax
xx
→ → →
−
= = = =
.
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
42
5)
2
0 0 0
2sin sin
1 cos
22
lim lim lim 0
sin
2sin .cos cos
2 2 2
x x x
xx
x
A
x x x
x
→ → →
−
= = = =
.
6)
( )
3 2 2
3
33
4cos 3cos 2 cos sin 2
cos3 2cos2 2
lim lim
sin3 3sin 4sin
xx
x x x x
xx
A
x x x

→→
− + − +
++
==
−
( )
( )
( )
2
32
2
2
33
cos 4cos 3 4cos
4cos 3cos 4cos
lim lim
sin 3 4sin
sin 3 4 1 cos
xx
x x x
x x x
xx
xx

→→
−+
−+
==

−
−−

( )
( )( )
( )( )
( )
( )
2
2
3 3 3
cos 2cos 1 4
cos 2cos 3 2cos 1 cos 2cos 3
23
lim lim lim .
sin 2cos 1 2cos 1 sin 2cos 1 3
sin 4cos 1
x x x
xx
x x x x x
x x x x x
xx
→ → →

+−
+ − +

= = = =
− + +

−

7)
2
22
1 sin2 cos2 2cos 2sin cos
lim lim
cos cos
xx
x x x x x
A
xx

→→
+ + +
==
( )
( )
22
2cos cos sin
lim lim2 cos sin 2.
cos
xx
x x x
xx
x

→→
+
= = + =
Bài 2. 1)
2
2
2
0 0 0
2
2sin sin
1 cos
2 2 2
lim lim lim . . .
1 cos
2sin sin
2 2 2
x x x
ax ax bx
ax a a
A
bx ax bx
bx b b
→ → →


−

= = = =


−



(Vì
0
sin
2
lim 1
2
x
ax
ax
→
=
và
0
2
lim 1
sin
2
x
bx
bx
→
=
).
2)
00
sin5 sin5
lim lim 5. 5
5
xx
xx
B
xx
→→

= = =


. (Vì
0
sin5
lim 1
5
x
x
x
→
=
).
3)
3
00
sin5 .sin3 .sin sin5 sin3 sin 1 1
lim lim . . .
45 5 3 3 3
xx
x x x x x x
B
x x x x
→→

= = =


(Vì
0
sin5
lim 1
5
x
x
x
→
=
,
0
sin3
lim 1
3
x
x
x
→
=
,
0
sin
lim 1
x
x
x
→
=
).
4)
2
2
2
00
2sin
1 cos 1
2
lim lim
2
.4
2
xx
x
x
B
x
x
→→
−
= = =



, (vì
2
2
0
sin
2
lim 1
2
x
x
x
→
=



.
5)
2
2
2
2
0 0 0
2
2
53
5
sin .
2sin
1 cos5 25 25
22
2
lim lim lim .
3
1 cos3 9 9
53
2sin
.sin
2
22
x x x
xx
x
x
B
x
x
xx
→ → →




−


= = = =

−





Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
43
(Vì
2
2
0
5
sin
2
lim 1
5
2
x
x
x
→
=



và
2
0
2
3
2
lim 1
3
sin
2
x
x
x
→



=
).
6)
2
22
2
2
00
2sin
1 cosa
2
lim lim .
42
2
xx
ax
x a a
B
x
ax
→→


−

= = =






, (vì
2
2
0
sin
2
lim 1
2
x
ax
ax
→
=



).
7)
2 2 2
2
0 0 0
sin 2 4sin .cos sin
lim lim lim .4cos 4
.sin .sin
x x x
x x x x
Bx
x x x x x
→ → →

= = = =


, (vì
0
sinx
lim 1
x
x
→
=
).
8)
3 3 3
0 0 0
sin
sin
sin tan sin .cos sin
cos
lim lim lim
cos
x x x
x
x
x x x x x
x
B
x x x x
→ → →
−
−−
= = =
( )
2
2
3
00
sin
sin 1 cos
2sin 2 1
2
lim lim . .
cos 4cos 2
2
xx
x
xx
x
x x x x
x
→→


−−
− − −

= = =






.
(vì
0
sinx
lim 1
x
x
→
=
và
2
2
0
sin
2
lim 1
2
x
x
x
→



=



).
9)
3 3 3
0 0 0
sin
sin
tan sin sin sin .cos
cos
lim lim lim
sin sin sin xcos
x x x
x
x
x x x x x
x
B
x x x
→ → →
−
−−
= = =
2
2
0 0 0
2 2 2
2sin
1 cos 1 1
2
lim lim lim
sin x.cos 2
4.sin .cos .cos cos .cos
2 2 2
x x x
x
x
x x x
x
xx
→ → →
−
= = = =
10)
( )
( )
( )
22
2
00
2sin 1 cos cos
1 cos 1 cos cos
2
lim lim
sin
2 .sin .cos
22
xx
x
xx
x x x
B
xx
xx
x
→→
++
− + +
==
2
0
sin
1 cos cos 3
2
lim .
2
2cos
22
x
x
xx
xx
→


++
==



, (vì
0
sin
2
lim 1
2
x
x
x
→
=
).
Bài 3. 1)
( )
( )
( ) ( )
2
2
22
4
44
0 0 0
cos8 1 sin 3
cos8 1 sin 3
2sin 4 sin 3
lim lim lim
3
3 cos8 1 3 cos8 1
x x x
xx
xx
xx
B
x
x x x x
→ → →
−
−
−
= = =
++
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
44
=
22
0
sin4 sin3 96
lim . . 48
43
cos8 1
x
xx
xx
x
→

−
=−

+


2)
00
1 2 1 2 1 1
lim lim .
sin2 sin2 2
1 2 1
xx
xx
B
xx
x
→→
− + −

= = = −

++

3)
( )
( )
( )
22
2
2
22
0 0 0
1 cos 1 2sin
1 cos cos2 1 cos cos2
lim lim lim
1 cos cos2 1 cos cos2
x x x
x
x x x x
B
x
x x x x x x
→ → →
−−
−−
= = =
++
( )
( ) ( )
2 2 2 2
2 2 2
22
00
2
2
0
sin cos cos 1 2sin
sin 2sin cos
lim lim
1 cos cos2 1 cos cos2
sinx 1 2cos 3
lim . .
2
1 cos cos2
xx
x
x x x x
x x x
x x x x x x
x
x
xx
→→
→
+ − −
+
==
++

+

==


+



4)
(
)
3
2
2
00
3
2
3
2
1 cos 1 cos
lim lim
sin
tan
1 cos cos
cos
xx
xx
B
x
x
xx
x
→→
−−
==
++
(
)
(
)
22
2
00
33
2 2 2 2 2
33
4sin cos
cos 1
2
lim lim
6
2sin cos 1 cos cos 2cos 1 cos cos
22
xx
x
x
x
x x x
x x x x
x
→→
= = =
+ + + +
.
5)
( )
(
)
3
2
3
2 2 2
3
44
tanx 1 tan 1
lim lim
2sin 1
sin cos tan tan 1
xx
x
B
x
x x x x

→→
−−
==
−
− + +
( )
(
)
( )
(
)
33
2 2 2 2
33
44
sin cos
11
cos
lim lim .
3
sin cos tan tan 1 cos sin cos tan tan 1
xx
xx
x
x x x x x x x x x

→→
−
= = =
− + + + + +
6)
( )
3
3
00
1 tan 1 sin tan sin
lim lim
1 tan 1 sin
xx
x x x x
B
x
x x x
→→
+ − + −
==
+ + +
( ) ( )
( )
2
33
00
2
0
2sin sin
sin sin cos
2
lim lim
cos 1 tan 1 sin cos 1 tan 1 sin
sin
sin 2 1
2
lim . .
4
4 1 tan 1 sin
2
xx
x
x
x
x x x
x x x x x x x x
x
x
x
x
xx
→→
→
−
==
+ + + + + +





==


+ + +




Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
45
7)
( )
( )
( )
2
2
2
2
2
2
0 0 0
2sin 1 1 sin
2 1 1
1 cos
22
lim lim lim . 2
4
11
2
x x x
xx
x
x
x
B
x
x
x
→ → →


+−

+−

−

= = = =


−−




.
8)
(
)
(
)
2 2 2 2 2
2
0 0 0
2 2 2 2
1 cos 1 cos sin
lim lim lim
1 cos 1 cos
x x x
x x x x x x
B
x
x x x x x x
→ → →
+ − + − +
= = =
+ + + +
2
2
22
0
sin 1 1 1 1
=lim . 1
22
1 cos 1 cos
x
x
x
x x x x
→

+ = + =

+ + + +

.
9)
0 0 0
1 2 1 sin 1 2 1 sin
lim =lim lim
3 4 2 3 4 2 3 4 2
x x x
x x x x
B
x x x x x x
→ → →
− + + − +
=+
+ − − + − − + − −
( )
( )
( )
( )
( )
( )
( )
( )
2
00
00
2 3 4 2 sin 3 4 2
lim lim
1
1 2 1
2 3 4 2
sin 3 4 2
lim lim .
1
1 1 2 1
4 4 0.
xx
xx
x x x x x x
xx
x x x
xx
x x x
xx
xx
→→
→→
− + + + + + +
=+
−+
− − + +
− + + +

+ + +
=+


−−
− − + +

=−=
10)
3 3 3
2 2 2
0 0 0 0
2 1 1 2 1 1 1 1 2 1 1 1 1
lim lim lim lim
sin sin sin sin
x x x x
x x x x x x
B
x x x x
→ → → →
+ − + + − + − + + − − +
= = = +
( )
( )
2
00
2
3
22
3
2
lim lim 1
sin 2 1 1
sin 1 1 1
xx
xx
xx
x x x
→→
−
= + =

++
+ + + +


.
Bài 4. 1)
4
lim tan 2 tan
4
x
C x x
→


=−




Đặt
4
tx
=−
, vì
0.
4
xt
→ →
Khi đó:
( )
2
0 0 0
cos2 1
lim tan 2 ( 1)tan lim cot 2 tan lim
2 2cos 2
t t t
t
C t t t t
t
→ → →


= + − = = =




.
2)
( )
2
1 cos
lim
x
x
C
x
→
+
=
−
Đặt
tx
=−
, vì
0.xt
→ →
Khi đó:
2
22
00
2sin
1 cos 1
2
lim lim .
2
tt
t
t
C
tt
→→
−
= = =
3)
( )
2
sin 1
lim
43
x
x
C
xx
→
−
=
−+
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
46
Đặt
tx
=−
, vì
1 0.xt→ →
Khi đó:
( ) ( )
( )( ) ( )
2
0
sin 1 sin 1
sint 1
lim lim lim
4 3 1 3 2 2
x x t
xx
C
x x x x t t

→ → →
−−
= = = = −
− + − − −
.
4)
sin sin
lim
xa
xa
C
xa
→
−
=
−
Đặt
t x a=−
. vì
0.x a t→ →
Khi đó:
( )
00
2
2cos .sin
sin sin
22
lim lim cos
2.
2
tt
t a t
t a a
Ca
t
t
→→
+
+−
= = =
.
C. BÀI TẬP RÈN LUYỆN
Bài 1. Tính các giới hạn sau:
1.
2
3
3
lim .
6
x
x
xx
→
−
−−
ĐS:
1
5
2.
2
3
2 15
lim .
3
x
xx
x
→
+−
−
ĐS : 8
3.
2
3
3
lim
23
x
x
xx
→−
+
+−
ĐS:
1
4
4.
2
2
2
32
lim .
4
x
xx
x
→
−+
−
ĐS:
1
.
4
5.
2
2
2
32
lim .
4
x
xx
x
→−
++
−
ĐS:
1
4
−
6.
2
2
3
7 12
lim .
9
x
xx
x
→
−+
−
ĐS:
1
6
−
.
7.
2
2
1
1
lim .
34
x
x
xx
→
−
+−
ĐS:
2
5
8.
2
2
2
6
lim .
4
x
xx
x
→
+−
−
ĐS:
5
.
4
9.
2
2
2
2 3 14
lim .
4
x
xx
x
→
+−
−
ĐS:
11
.
4
10.
2
2
3
9
lim .
43
x
x
xx
→
−
−+
ĐS:
3
11.
2
2
2
3 10
lim .
4 18
x
xx
xx
→
−−
+−
ĐS:
11
7
. 12.
2
2
5
5
lim .
25
x
xx
x
→
−
−
ĐS:
1
.
2
13.
2
2
2
4
lim .
2 10 12
x
x
xx
→
−
−+
ĐS: 2 14.
2
2
2
4
lim .
26
x
x
xx
→
−
−−
ĐS:
4
.
7
−
15.
2
2
3
56
lim .
3
x
xx
xx
→
−+
−
ĐS:
1
.
3
16.
2
2
5
9 20
lim .
5
x
xx
xx
→
−+
−
ĐS:
1
.
5
17.
2
2
3
3 10 3
lim .
56
x
xx
xx
→
−+
−+
ĐS: 8 18.
2
2
3
23
lim .
21
x
xx
xx
→
+−
−−
ĐS: 4
19.
32
2
3
56
lim .
9
x
x x x
x
→
−+
−
ĐS:
1
.
2
−
20.
4
2
2
16
lim .
68
x
x
xx
→−
−
++
ĐS:
16.−
21.
3
2
2
8
lim .
56
x
x
xx
→
−
−+
ĐS: 12 22.
3
2
2
8
lim .
11 18
x
x
xx
→−
+
++
ĐS:
12
.
7
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
47
23.
2
3
2
22
lim .
22
x
xx
x
→
− − +
−
ĐS:
2 2 1
.
6
−
24.
3
2
2
8
lim .
32
x
x
xx
→
−
−+
ĐS:
12.
25.
3
2
2
22
lim .
2
x
x
x
→−
+
−
ĐS:
32
.
2
−
26.
( )
3
0
11
lim .
x
x
x
→
+−
ĐS:
3.
27.
( )
3
0
1 27
lim .
x
x
x
→
+−
ĐS:
27.
28.
4
2
3
27
lim .
2 3 9
x
xx
xx
→
−
−−
ĐS:
9.
29.
32
2
5 10 8
lim .
2
x
x x x
x
→
− + −
−
ĐS:
2.
30.
32
2
1
2 5 2 1
lim .
1
x
x x x
x
→
− + +
−
ĐS:
1.−
31.
3
2
2
24
lim .
4
x
xx
x
→
−−
−
ĐS:
5
.
2
32.
32
2
2
32
lim .
6
x
x x x
xx
→−
++
−−
ĐS:
2
.
5
−
33.
2
3
2
2 10
lim .
6
x
xx
xx
→−
−−
−+
ĐS:
9
.
11
−
34.
32
2
1
1
lim .
21
x
x x x
xx
→
− − +
−+
ĐS:
2.
35.
2
3
2
4
lim .
32
x
x
xx
→
−
−−
ĐS:
4
.
9
36.
32
2
2
22
lim .
4
x
x x x
x
→
− − +
−
ĐS:
3
.
4
37.
2
32
1
34
23
lim
x
xx
xx
→
+−
+−
. ĐS:
5
8
38.
32
2
1
3 4 2 3
lim
3 2 1
x
x x x
xx
→
− − +
−−
. ĐS:
1
4
−
39.
32
2
2
52
lim
32
x
x x x
xx
→
+ − −
−+
ĐS: 11 40.
3
2
1
2 5 3
lim
32
x
xx
xx
→
−+
−+
ĐS: -1
41.
2
32
2
28
lim
3 4 6
x
xx
x x x
→−
−−
+ − +
ĐS:
6
19
−
. 42.
3
42
1
1
lim
43
x
x
xx
→
−
−+
ĐS:
3
4
.
43.
32
42
3
5 3 9
lim
89
x
x x x
xx
→
− + +
−−
ĐS:
0
. 44.
32
42
1
3
6 5 4 1
lim
9 8 1
x
x x x
xx
→
− + −
+−
ĐS:
2
5
.
45.
1
23
lim
54
x
xx
xx
→
+−
−+
ĐS:
4
3
−
. 46.
3
4
1
32
lim
43
x
xx
xx
→
−+
−+
ĐS:
1
2
.
47.
54
2
2
22
lim
4
x
x x x
x
→
− + −
−
ĐS:
17
4
. 48.
43
32
1
1
lim
5 7 3
x
x x x
x x x
→
− − +
− + −
ĐS:
3
2
−
.
49.
32
32
3
2 5 2 3
lim
4 13 4 3
x
x x x
x x x
→
− − −
− + −
ĐS:
11
17
. 50.
32
32
1
2 5 4 1
lim
1
x
x x x
x x x
→−
+ + +
+ − −
ĐS:
1
2
.
51.
32
32
3
2 5 2 3
lim
4 12 4 12
x
x x x
x x x
→
− − −
− + −
ĐS:
11
20
. 52.
3
2
3
2
1
21
lim
( 1)
x
xx
x
→
−+
−
ĐS:
1
9
.
53.
4 3 2
32
2
2 8 7 4 4
lim
3 14 20 8
x
x x x x
x x x
→−
+ + − −
+ + +
ĐS:
7
4
−
. 54.
32
2
3
2 3 9 7 3
lim
3
x
x x x
x
→−
− + + +
−
ĐS:
73
6
55.
4 3 2
4 3 2
1
5 9 7 2
lim
3 3 2
x
x x x x
x x x x
→
− + − +
− + + −
ĐS:
. 56.
5 4 3 2
2
1
5
lim
1
x
x x x x x
x
→
+ + + + −
−
ĐS:
15
2
.
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
48
57.
2
1
12
lim
11
x
xx
→

−

−−

ĐS:
1
2
. 58.
3
2
1 12
lim
28
x
xx
→

−

−−

ĐS:
1
2
.
59.
22
2
11
lim
3 2 5 6
x
x x x x
→

+

− + − +

ĐS:
2−
. 60.
2
2
2 3 26
lim
24
x
xx
xx
→−
−−

−

+−

ĐS:
7
2
.
61.
23
1
11
lim
21
x
x x x
→

−

+ − −

ĐS:
2
9
. 62.
0
(1 )(1 2 )(1 3 ) 1
lim
x
x x x
x
→
+ + + −
ĐS:
.
63.
1
1
lim
1
n
m
x
x
x
→
−
−
ĐS:
n
m
. 64.
2
1
1
lim
( 1)
n
x
x nx n
x
→
− + −
−
ĐS:
( 2)( 1)
2
nn−−
.
65.
100
50
1
21
lim
21
x
xx
xx
→
−+
−+
ĐS:
. 66.
2
1
...
lim
1
n
x
x x x n
x
→
+ + + −
−
ĐS:
( 1)
2
nn+
.
Li giải
1.
( )( )
2
3 3 3
3 3 1 1
lim lim =lim .
6 2 3 2 5
x x x
xx
x x x x x
→ → →
−−
==
− − + − +
2.
( )( )
( )
2
3 3 3
35
2 15
lim lim =lim 5 8
33
x x x
xx
xx
x
xx
→ → →
−+
+−
= + =
−−
3.
2
3
3
lim
23
x
x
xx
→−
+
+−
( )( )
3
3
lim
31
x
x
xx
→−
+
=
+−
4.
2
2
2
32
lim
4
x
xx
x
→
−+
−
( )( )
( )( )
2
12
lim
22
x
xx
xx
→
−−
=
−+
3
11
lim .
14
x
x
→−
==
−
2
11
lim .
24
x
x
x
→
−
==
+
5.
( )( )
( )( )
2
2
2 2 2
12
3 2 1 1
lim lim = lim .
4 2 2 2 4
x x x
xx
x x x
x x x x
→− →− →−
++
+ + +
= = −
− − + −
6.
( )( )
( )( )
2
2
3 3 3
34
7 12 4 1
lim lim =lim .
9 3 3 3 6
x x x
xx
x x x
x x x x
→ → →
−−
− + −
= = −
− − + +
7.
( )( )
( )( )
2
2
1 1 1
11
1 1 2
lim lim =lim
3 4 1 4 4 5
x x x
xx
xx
x x x x x
→ → →
−+
−+
==
+ − − + +
8.
( )( )
( )( )
2
2
2 2 2
23
6 3 5
lim lim =lim .
4 2 2 2 4
x x x
xx
x x x
x x x x
→ → →
−+
+ − +
==
− − + +
9.
( )( )
( )( )
2
2
2 2 2
2 2 7
2 3 14 2 7 11
lim lim =lim .
4 2 2 2 4
x x x
xx
x x x
x x x x
→ → →
−+
+ − +
==
− − + +
10.
( )( )
( )( )
2
2
3 3 3
33
93
lim lim =lim 3.
4 3 3 1 1
x x x
xx
xx
x x x x x
→ → →
−+
−+
==
− + − − −
11.
( )( )
( )( )
2
2
2 2 2
2 3 5
3 10 3 5 11
lim lim =lim .
4 18 2 4 9 4 9 17
x x x
xx
x x x
x x x x x
→ → →
−+
− − +
==
+ − − + +
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
49
12.
( )
( )( )
2
2
5 5 5
5
51
lim lim =lim .
25 5 5 5 2
x x x
xx
x x x
x x x x
→ → →
−
−
==
− − + +
13.
( )( )
( )( ) ( )
2
2
2 2 2
22
42
lim lim lim 2.
2 10 12 2 2 3 2 3
x x x
xx
xx
x x x x x
→ → →
−+
− − −
= = =
− + − − −
14.
( )( )
( )( )
2
2
2 2 2
22
4 2 4
lim lim lim .
2 6 2 2 3 2 3 7
x x x
xx
xx
x x x x x
→ → →
−+
− − − −
= = =
− − − + +
15.
( )( )
( )
2
2
3 3 3
23
5 6 2 1
lim lim =lim .
3 2 2 3
x x x
xx
x x x
x x x x
→ → →
−−
− + −
==
−−
16.
( )( )
( )
2
2
5 5 5
45
9 20 4 1
lim lim =lim .
5 5 5
x x x
xx
x x x
x x x x x
→ → →
−−
− + −
==
−−
17.
( )( )
( )( )
2
2
3 3 3
3 3 1
5 6 3 1
lim lim =lim 8.
3 2 3 2
x x x
xx
x x x
x x x x x
→ → →
−−
− + −
==
− − − −
18.
( )( )
( )( )
2
2
3 3 3
13
2 3 3
lim lim =lim 4.
2 1 1 2 1 2 1
x x x
xx
x x x
x x x x x
→ → →
−+
+ − +
==
− − − − −
19.
( )( )
( )( )
( )
32
2
3 3 3
2 3 2
5 6 1
lim lim =lim
9 3 3 3 2
x x x
x x x x x
x x x
x x x x
→ → →
− − −
−+
= = −
− − + − −
20.
( )
( )( )
( )( )
( )
( )
22
4
2
2 2 2
4 2 2 4 2
16
lim lim = lim 16
6 8 2 4 4
x x x
x x x x x
x
x x x x x
→− →− →−
+ − + + −
−
= = −
+ + + + +
21.
3
2
2
8
lim
56
x
x
xx
→
−
−+
=
( )
( )
( )( )
2
2
2 4 2
lim
23
x
x x x
xx
→
− + +
−−
22.
( )
( )
( )( )
2
3
2
22
2 2 4
8
lim lim
11 18 2 9
xx
x x x
x
x x x x
→− →−
+ − +
+
=
+ + + +
( )
2
2
24
lim 12.
3
x
xx
x
→
− + +
==
−
2
2
2 4 12
lim .
97
x
xx
x
→−
−+
==
+
23.
( )( )
( )( )
2
32
2
2 2 2
2 1 2
2 2 1 2 2 2 1
lim lim = lim .
6
2 2 2 2
2 2 2
x x x
xx
x x x
x x x
x x x
→ → →
− − +
− − + − + −
==
− + +
− + +
24.
( )
( )
( )( )
2
32
2
2 2 2
2 2 4
8 2 4
lim lim =lim 12.
3 2 1 2 1
x x x
x x x
x x x
x x x x x
→ → →
− + +
− + +
==
− + − − −
25.
( )( )
( )( )
2
32
2
2 2 2
2 2 2
2 2 2 2 3 2
lim lim = lim .
22
2
22
x x x
x x x
x x x
x
x
xx
→− →− →−
− − +
+ − +
= = −
−
−
−+
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
50
26.
( )
( )
3
32
2
0 0 0
11
33
lim lim lim 3 3 3.
x x x
x
x x x
xx
xx
→ → →
+−
++
= = + + =
27.
( )
( ) ( )
( ) ( )
2
3
2
0 0 0
3 3 3 9
1 27
lim lim =lim 3 3 3 9 27.
x x x
x x x
x
xx
xx
→ → →

+ + + +
+−


= + + + + =

28.
( )
( )
( )( )
( )
22
4
2
3 3 3
3 3 9 3 9
27
lim lim =lim 9.
2 3 9 3 2 3 2 3
x x x
x x x x x x x
xx
x x x x x
→ → →
− + + + +
−
==
− − − + +
29.
( )
( )
( )
2
32
2
2 2 2
2 3 4
5 10 8
lim lim =lim 3 4 2.
22
x x x
x x x
x x x
xx
xx
→ → →
− − +
− + −
= − + =
−−
30
( )
( )
( )( )
2
3 2 2
2
1 1 1
1 2 3 1
2 5 2 1 2 3 1
lim lim =lim 1.
1 1 1 1
x x x
x x x
x x x x x
x x x x
→ → →
− − −
− + + − −
= = −
− − + +
31.
( )
( )
( )( )
2
32
2
2 2 2
2 2 2
2 4 2 2 5
lim lim =lim .
4 2 2 2 2
x x x
x x x
x x x x
x x x x
→ → →
− + +
− − + +
==
− − + +
32.
( )( )
( )( )
( )
32
2
2 2 2
1 2 1
3 2 2
lim lim = lim .
6 2 3 3 5
x x x
x x x x x
x x x
x x x x x
→− →− →−
+ + +
++
= = −
− − + − −
33.
( )( )
( )
( )
2
32
2
2 2 2
2 2 5
2 10 2 5 9
lim lim lim .
6 2 3 11
2 2 3
x x x
xx
x x x
x x x x
x x x
→− →− →−
+−
− − +
= = = −
− + − +
+ − +
34.
( ) ( )
( )
( )
2
32
2
2
1 1 1
11
1
lim . lim =lim 1 2.
21
1
x x x
xx
x x x
x
xx
x
→ → →
−+
− − +
= + =
−+
−
35.
( )( )
( )( ) ( )
2
22
3
2 2 2
22
4 2 4
lim lim =lim .
3 2 9
2 1 1
x x x
xx
xx
xx
x x x
→ → →
−+
−+
==
−−
− + +
36.
( )
( )
( )( )
2
3 2 2
2
2 2 2
21
2 2 1 3
lim lim =lim .
4 2 2 2 4
x x x
xx
x x x x
x x x x
→ → →
−−
− − + −
==
− − + +
37.
2
3 2 2 2
1 1 1
3 4 ( 1)( 4) 4 5
lim lim lim
2 3 ( 1)(2 3 3) 2 3 3 8
x x x
x x x x x
x x x x x x x
→ → →
+ − − + +
= = =
+ − − + + + +
38
3 2 2 2
2
1 1 1
3 4 2 3 ( 1)(3 3) 3 3 1
lim lim lim
3 2 1 ( 1)(3 1) 3 1 4
x x x
x x x x x x x x
x x x x x
→ → →
− − + − − − − −
= = = −
− − − + +
39.
3 2 2 2
2
2 2 2
5 2 ( 2)( 3 1) 3 1
lim lim lim 11
3 2 ( 2)( 1) 1
x x x
x x x x x x x x
x x x x x
→ → →
+ − − − + + + +
= = =
− + − − −
.
40.
3 2 2
2
1 1 1
2 5 3 ( 1)(2 2 3) 2 2 3
lim lim lim 1
3 2 ( 2)( 1) 2
x x x
x x x x x x x
x x x x x
→ → →
− + − + − + −
= = = −
− + − − −
.
41.
2
3 2 2 2
2 2 2
2 8 ( 2)( 4) 4 6
lim lim lim
3 4 6 ( 2)(3 2 3) 3 2 3 19
x x x
x x x x x
x x x x x x x x
→− →− →−
− − + − −
= = = −
+ − + + − + − +
.
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
51
42.
3 2 2
4 2 3 2 3 2
1 1 1
1 ( 1)( 1) 1 3
lim lim lim
4 3 ( 1)( 3 3) 3 3 4
x x x
x x x x x x
x x x x x x x x x
→ → →
− − − − − − − −
= = =
− + − + − − + − −
.
43.
3 2 2 2
4 2 3 2 3 2
3 3 3
5 3 9 ( 3)( 2 3) 2 3
lim lim lim 0
8 9 ( 3)( 3 3) 3 3
x x x
x x x x x x x x
x x x x x x x x x
→ → →
− + + − − − − −
= = =
− − − + + + + + +
.
44.
3 2 2 2
4 2 3 2 3 2
1 1 1
3 3 3
6 5 4 1 (3 1)(2 1) 2 1 2
lim lim lim
9 8 1 (3 1)(3 3 1) 3 3 1 5
x x x
x x x x x x x x
x x x x x x x x x
→ → →
− + − − − + − +
= = =
+ − − + + + + + +
.
45.
1 1 1
2 3 ( 1)( 3) 3 4
lim lim lim
3
5 4 ( 1)( 4) 4
x x x
x x x x x
x x x x x
→ → →
+ − − + +
= = = −
− + − − −
.
46.
32
4 2 2 2
1 1 1
3 2 ( 2)( 2 1) 2 1
lim lim lim
4 3 ( 2 1)( 2 3) 2 3 2
x x x
x x x x x x
x x x x x x x x
→ → →
− + + − + +
= = =
− + − + + + + +
.
47.
5 4 4 4
2
2 2 2
2 2 ( 2)( 1) 1 17
lim lim lim
4 ( 2)( 2) 2 4
x x x
x x x x x x
x x x x
→ → →
− + − − + +
= = =
− − + +
.
48.
4 3 2 2 2
3 2 2
1 1 1
1 ( 2 1)( 1) 1 3
lim lim lim
5 7 3 ( 2 1)( 3) 3 2
x x x
x x x x x x x x x
x x x x x x x
→ → →
− − + − + + + + +
= = = −
− + − − + − −
.
49.
3 2 2 2
3 2 2 2
3 3 3
2 5 2 3 ( 3)(2 1) 2 1 11
lim lim lim
4 13 4 3 ( 3)(4 1) 4 1 17
x x x
x x x x x x x x
x x x x x x x x
→ → →
− − − − + + + +
= = =
− + − − − + − +
.
50.
3 2 2
3 2 2
1 1 1
2 5 4 1 (2 1)( 2 1) 2 1 1
lim lim lim
1 ( 1)( 2 1) 1 2
x x x
x x x x x x x
x x x x x x x
→− →− →−
+ + + + + + +
= = =
+ − − − + + −
.
51.
3 2 2 2
3 2 2 2
3 3 3
2 5 2 3 ( 3)(2 1) 2 1 11
lim lim lim
4 12 4 12 4( 3)( 1) 4( 1) 20
x x x
x x x x x x x x
x x x x x x
→ → →
− − − − + + + +
= = =
− + − − + +
.
52.
3
22
33
2
33
2 2 2 2 2
1 1 1
3 3 3
2 1 ( 1) 1 1
lim lim lim
( 1) 9
( 1) ( 1) ( 1)
x x x
x x x
x
x x x x x
→ → →
− + −
= = =
−
− + + + +
.
53.
4 3 2 2 2 2
3 2 2
2 2 2
2 8 7 4 4 (2 1)( 4 4) 2 1 7
lim lim lim
3 14 20 8 (3 2)( 4 4) 3 2 4
x x x
x x x x x x x x
x x x x x x x
→− →− →−
+ + − − − + + −
= = = −
+ + + + + + +
.
54.
3 2 2
2
33
2 3 9 7 3 ( 3)(2 (3 2 3) 7 3 3)
lim lim
3
( 3 )( 3 )
xx
x x x x x x
x
xx
→− →−
− + + + + − − + −
=
−
−+
2
3
2 (3 2 3) 7 3 3 7 3
lim
6
3
x
xx
x
→−
− − + −
==
−
55.
4 3 2 3
4 3 2 2
1 1 1
5 9 7 2 ( 1) ( 2) 1
lim lim lim 0
3 3 2 ( 1) ( 2)( 1) 1
x x x
x x x x x x x
x x x x x x x x
→ → →
− + − + − − −
= = =
− + + − − − + +
.
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
52
56.
5 4 3 2 4 3 2
2
11
5 ( 1)( 2 3 4 5)
lim lim
1 ( 1)( 1)
xx
x x x x x x x x x x
x x x
→→
+ + + + − − + + + +
=
− − +
4 3 2
1
2 3 4 5 15
lim
12
x
x x x x
x
→
+ + + +
==
+
.
57.
2
1 1 1
1 2 1 1 1
lim lim lim
1 1 ( 1)( 1) 1 2
x x x
x
x x x x x
→ → →
−

− = = =

− − − + +

.
58.
3 2 2
2 2 2
1 12 ( 2)( 4) 4 1
lim lim lim
2 8 ( 2)( 2 4) 2 4 2
x x x
x x x
x x x x x x x
→ → →
− + +

− = = =

− − − + + + +

.
59.
22
2 2 2
1 1 2( 2) 2
lim lim lim 2
3 2 5 6 ( 2)( 3)( 1) ( 3)( 1)
x x x
x
x x x x x x x x x
→ → →
−

+ = = = −

− + − + − − − − −

.
60.
2
2 2 2
2 3 26 2( 5)( 2) 2( 5) 7
lim lim lim
2 4 ( 2)( 2) 2 2
x x x
x x x x x
x x x x x
→− →− →−
− − − + −

− = = =

+ − − + −

.
61.
2 3 2 2
1 1 1
1 1 ( 1)( 1) 1 2
lim lim lim
2 1 ( 1)( 2)( 1) ( 2)( 1) 9
x x x
x x x
x x x x x x x x x x
→ → →
− + +

− = = =

+ − − − + + + + + +

.
62.
( )
2
2
0 0 0
(1 )(1 2 )(1 3 ) 1 (6 11 6)
lim lim lim 6 11 6 6
x x x
x x x x x x
xx
xx
→ → →
+ + + − + +
= = + + =
.
63.
1 2 1 2
1 2 1 2
1 1 1
1 ( 1)( ... 1) ... 1
lim lim lim
1 ( 1)( ... 1) ... 1
n n n n n
m m m m m
x x x
x x x x x x x x n
x x x x x x x x m
− − − −
− − − −
→ → →
− − + + + + + + + +
= = =
− − + + + + + + + +
.
64.
12
22
11
1 ( 1)( ... 1) n( 1)
lim lim
( 1) ( 1)
n n n
xx
x nx n x x x x x
xx
−−
→→
− + − − + + + + − −
=
−−
1 2 2
1
( 1) ( 1) ... ( 1) ( 1)
lim
1
nn
x
x x x x
x
−−
→
− + − + + − + −
=
−
( )
2 3 3 4
1
lim ( ... 1) ( ... 1) ... 1
n n n n
x
x x x x x x
− − − −
→
= + + + + + + + + + + +
( 2)( 1)
( 2) ( 3) ... 2 1
2
nn
nn
−−
= − + − + + + =
65.
100
50
1
21
lim
21
x
xx
xx
→
−+
−+
.
99 98
49 48
1
( 1)( ... 1) ( 1)
lim
( 1)( ... 1) ( 1)
x
x x x x x
x x x x x
→
− + + + + − −
=
− + + + + − −
99 98
49 48
1
... 49
lim
... 24
x
x x x
x x x
→
+ + +
==
+ + +
66.
22
11
... ( 1) ( 1) ... ( 1)
lim lim
11
nn
xx
x x x n x x x
xx
→→
+ + + − − + − + + −
=
−−
12
1
( 1) ( 1)( 1) ... ( 1)( ... 1)
lim
1
nn
x
x x x x x x x
x
−−
→
− + − + + + − + + + +
=
−
12
1
lim(1 ( 1) ... ( ... 1))
nn
x
x x x x
−−
→
= + + + + + + + +
( 1)
1 2 3 ... n
2
nn+
= + + + + =
Bài 2. Tính các giới hạn sau:
1.
1
32
lim
1
x
x
x
→
+−
−
ĐS:
1
4
. 2.
2
2
lim
31
x
x
x
→−
+
+−
ĐS:
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
53
3.
6
33
lim
6
x
x
x
→
−+
−
ĐS:
1
6
−
. 4.
8
8
lim
31
x
x
x
→
−
−+
ĐS:
6−
.
5.
2
1
42
lim
1
x
xx
x
→−
+ + −
+
ĐS:
1
4
−
. 6.
2
3
3
lim
26
x
x x x
x
→
−−
−
ĐS:
1
4
.
7.
2
2
22
lim
4
x
x
x
→
+−
−
ĐS:
1
16
. 8.
2
2
2 3 2
lim
4
x
x
x
→
−−
−
ĐS:
3
16
−
.
9.
2
3
9
lim
12
x
x
x
→
−
+−
ĐS: 24. 10.
2
9
3
lim
9
x
x
xx
→
−
−
ĐS:
1
54
−
.
11.
2
7
49
lim
23
x
x
x
→
−
−−
ĐS:
56−
. 12.
2
1
23
lim
1
x
xx
x
→
−+
−
ĐS:
7
8
.
13.
2
3
32
lim
3
x
xx
xx
→
−+
−
ĐS:
2
9
. 14.
2
2
1
lim
21
x
xx
xx
→
−
−−
ĐS:
.
15.
2
2
4 1 3
lim
2
x
x
xx
→
+−
−
ĐS:
1
3
. 16.
2
4
3 3 3
lim
4
x
x
xx
→
−−
−
ĐS:
1
8
.
17.
2
2
22
lim
2 10
x
x
xx
→
+−
+−
ĐS:
1
4
. 18.
2
2
32
lim
11
x
xx
x
→
−+
−−
ĐS:
.
19.
2
4
34
lim
53
x
xx
x
→
−−
+−
ĐS:
30
. 20.
2
1
3 1 2
lim
2
x
x
xx
→
+−
+−
ĐS:
1
4
.
21.
2
1
1
lim
1
x
x
x
→
−
−
ĐS:
1
4
. 22.
2
2
3 3( 1)
lim
3 4 1
x
xx
x
→
−+
−+
ĐS:
12−
.
23.
3
2
0
11
lim
x
x
xx
→
+−
+
ĐS:
0
. 24.
3
2
2
lim
13
x
x
x
→−
+
−−
ĐS:
1
2
−
.
25.
2
2
1
21
lim
x
xx
xx
→
−−
−
ĐS:
0
. 26.
2
2
2 5 5
lim
2
x
xx
xx
→
+ + −
−
ĐS:
2
3
.
27.
2
1
lim
2 7 4
x
xx
xx
→
−
+ + −
ĐS:
3
4
. 28.
2
1
2 7 2
lim
1
x
xx
x
→−
− + −
−
ĐS:
1
6
.
29.
2
2
1
2 5 2 8
lim
32
x
x x x
xx
→−
+ − + +
++
ĐS:
5
2
. 30.
2
5 6 2
lim
2
x
xx
x
→
− − +
−
ĐS:
1
.
31.
1
33
lim
3 2 2
x
x
xx
→−
+
+ − +
ĐS:
6
. 32.
22
2
3
2 6 2 6
lim
43
x
x x x x
xx
→
− + − + −
−+
ĐS:
1
3
−
.
33.
2
4
1
21
lim
4
x
x x x
x
→−
+ + − −
+
ĐS:
0
. 34.
2
22
lim
73
x
x
x
→
−+
+−
ĐS:
3
2
−
.
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
54
35.
9
3
lim
52
x
x
x
→
−
−−
ĐS:
2
3
−
. 36.
1
3 1 3
lim
83
x
xx
x
→
+ − +
+−
ĐS:
3
.
37.
2
22
lim
13
x
xx
xx
→
+−
− − −
ĐS:
1
4
−
. 38.
1
32
lim
4 5 3 6
x
x
xx
→
+−
+ − +
ĐS:
3
2
.
39.
3
1 3 5
lim
2 3 6
x
xx
xx
→
+ − −
+ − +
ĐS:
3−
. 40.
2
2
2
2 1 2 5
lim
13
x
xx
xx
→
+ − +
+ − +
ĐS:
25
3
.
41.
2
1
1
lim
33
x
x
x x x
→
−
+ + −
ĐS:
4
3
−
. 42.
4
1
4 3 1
lim
1
x
x
x
→
+−
−
ĐS:
1
.
43.
4 3 2
2
1 3 3
lim
22
x
x x x x
x
→
− + − + +
−
ĐS:
1
.
Li giải
1. Ta có
1 1 1
3 2 ( 3 2)( 3 2) 1 1
lim lim lim
14
( 1)( 3 2) 3 2
x x x
x x x
x
x x x
→ → →
+ − + − + +
= = =
−
− + + + +
.
2. Ta có
2 2 2
2 ( 2)( 3 1)
lim lim lim( 3 1) 2
3 1 ( 3 1)( 3 1)
x x x
x x x
x
x x x
→− →− →−
+ + + +
= = + + =
+ − + − + +
.
3. Ta có
6 6 6
3 3 (3 3)(3 3) 1 1
lim lim lim
66
( 6)(3 3) 3 3
x x x
x x x
x
x x x
→ → →
− + − + + + −
= = = −
−
− + + + +
4. Ta có
8 8 8
8 ( 8)(3 1) 3 1
lim lim lim 6
1
3 1 (3 1)(3 1)
x x x
x x x x
x x x
→ → →
− − + + + +
= = = −
−
− + − + + +
.
5. Ta có
2
22
1 1 1
4 2 ( 1) 1
lim lim lim
14
( 1)( 4 2) 4 2
x x x
x x x x x
x
x x x x x
→− →− →−
+ + − +
= = = −
+
+ + + − + + −
.
6. Ta có
2 2 2
2
33
2 3 ( 2 3 )( 2 3 )
lim lim
26
(2 6)( 2 3 )
xx
x x x x x x x x x
x
x x x x
→→
− − − − − +
=
−
− − +
22
33
( 3) 1
lim lim
4
2( 3)( 2 3 ) 2( 2 3 )
xx
x x x
x x x x x x x
→→
−
= = =
− − + − +
.
7. Ta có
2
2 2 2
2 2 2 1 1
lim lim lim
4 16
( 2)( 2)( 2 2) ( 2)( 2 2)
x x x
xx
x
x x x x x
→ → →
+ − −
= = =
−
− + + − + + −
.
8. Ta có
2
2 2 2
2 3 2 3(2 ) 3 3
lim lim lim
4 16
( 2)( 2)(2 3 2) ( 2)(2 3 2)
x x x
xx
x
x x x x x
→ → →
− − −
= = = −
−
− + + − + + −
.
9. Ta có
2
3 3 3
9 ( 3)( 3)( 1 2)
lim lim lim ( 3)( 1 2) 24
1 2 ( 1 2)( 1 2)
x x x
x x x x
xx
x x x
→ → →
− + − + +

= = + + + =

+ − + − + +
.
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
55
10. Ta có
2
9 9 9
3 9 1 1
lim lim lim
9 54
(9 )( 3) ( 3)
x x x
xx
xx
x x x x x
→ → →
− − −
= = = −
−
− + +
.
11. Ta có
2
77
49 ( 7)( 7)(2 3)
lim lim
2 3 (2 3)(2 3)
xx
x x x x
x x x
→→
− − + + −
=
− − − − + −
77
( 7)( 7)(2 3)
lim lim( 7)(2 3) 56
7
xx
x x x
xx
x
→→
− + + −
= = − + + − = −
−
12. Ta có
2
2
1 1 1
2 3 4 3 4 3 7
lim lim lim
18
( 1)( 1)(2 3) ( 1)(2 3)
x x x
x x x x x
x
x x x x x x x
→ → →
− + − − +
= = =
−
− + + + + + +
.
13. Ta có
2
2
3 3 3
3 2 2 3 1 2
lim lim lim
39
( 3)( 3 2 ) ( 3 2 )
x x x
x x x x x
xx
x x x x x x x
→ → →
− + − − +
= = =
−
− + + + +
.
14. Ta có
2 2 2
2
2
1 1 1
( 1)( 2 1) ( 2 1)
lim lim lim
2 1 ( 1)
21
x x x
x x x x x x x x x
x x x
xx
→ → →
− − − + − +
= = =
− + − − −
−−
.
15. Ta có
2
2 2 2
4 1 3 4( 2) 4 1
lim lim lim
23
( 2)( 4 1 3) ( 4 1 3)
x x x
xx
xx
x x x x x
→ → →
+ − −
= = =
−
− + + + +
.
16. Ta có
2
4 4 4
3 3 3 3( 4) 3 1
lim lim lim
48
( 4)( 3 3 3) ( 3 3 3)
x x x
xx
xx
x x x x x
→ → →
− − −
= = =
−
− − + − +
.
17. Ta có
2
2 2 2
2 2 ( 2 2)( 2 2) 2
lim lim lim
2 10
( 2)(2 5)( 2 2) (2 5)( 2)( 2 2)
x x x
x x x x
xx
x x x x x x
→ → →
+ − + − + + −
==
+−
− − + + − − + +
2
11
lim
4
(2 5)( 2 2)
x
xx
→
= = −
− + +
18. Ta có
2
2 2 2
3 2 ( 1)( 2)( 1 1)
lim lim lim( 1)( 1 1) 2
1 1 ( 1 1)( 1 1)
x x x
x x x x x
xx
x x x
→ → →
− + − − − +
= = − − + =
− − − − − +
.
19. Ta có
2
4 4 4
3 4 ( 1)( 4)( 5 3)
lim lim lim( 1)( 5 3) 30
4
53
x x x
x x x x x
xx
x
x
→ → →
− − + − + +
= = + + + =
−
+−
.
20. Ta có
2
1 1 1
3 1 2 3( 1) 3 1
lim lim lim
24
( 1)( 2)( 3 1 2) ( 2)( 3 1 2)
x x x
xx
xx
x x x x x
→ → →
+ − −
= = =
+−
− + + + + + +
.
21. Ta có
2
1 1 1
1 1 1 1
lim lim lim
14
( 1)( 1)( 1) ( 1)( 1)
x x x
xx
x
x x x x x
→ → →
−−
= = =
−
+ − + + +
.
22. Ta có
2
22
3 4( 1) ( 2)(3 2)(3 4 1)
lim lim
3 4 1 (3 4 1)(3 4 1)
xx
x x x x x
x x x
→→
− + − + + +
=
− + − + + +
2
( 2)(3 2)(3 4 1)
lim
4(2 )
x
x x x
x
→
− + + +
=
−
2
(3 2)(3 4 1)
lim 12
4
x
xx
→
+ + +
= = −
.
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
56
23. Ta có
3 3 2
2
33
0 0 0
11
lim lim lim 0
( 1)( 1 1) ( 1)( 1 1)
x x x
x x x
xx
x x x x x
→ → →
+−
= = =
+
+ + + + + +
.
24. Ta có
33
22
3
2 2 2
2 ( 2)( 1 3) 1 3 1
lim lim lim
( 2)( 2 4) ( 2 4) 2
13
x x x
x x x x
x x x x x
x
→− →− →−
+ + − + − +
= = =
− + − + − − +
−−
.
25. Ta có
22
2
22
1 1 1
2 1 ( 1) ( 1)
lim lim lim 0
( 1)( 2 1) ( 2 1)
x x x
x x x x
xx
x x x x x x x
→ → →
− − − − − −
= = =
−
− − + − +
26. Ta có
2
2
22
2 5 5 12 20
lim lim
2
( 2)( 2 5 ( 5))
xx
x x x x
xx
x x x x
→→
+ + − − + −
=
−
− + − +
22
( 2)( 10) ( 10) 2
lim lim
3
( 2)( 2 5 ( 5)) ( 2 5 ( 5))
xx
x x x
x x x x x x x
→→
− − − − −
= = =
− + − + + − +
27. Ta có
2
2
1 1 1
( 1)( 2 7 ( 4) ( 2 7 ( 4) 3
lim lim lim
10 9 ( 9) 4
2 7 4
x x x
x x x x x x x x x
x x x
xx
→ → →
− − + − − + − −
= = =
− + − − −
+ + −
28. Ta có
2
2
11
2 7 2 2 3
lim lim
1
( 1)( 1)(( 2) 7 2 )
xx
x x x x
x
x x x x
→− →−
− + − − −
=
−
− + − − −
1
31
lim
6
( 1)(( 2) 7 2 )
x
x
x x x
→−
+
==
− − − −
29. Ta có
2
2
2
11
2 5 2 8 2 17 5
lim lim
3 2 2
( 2)((2 5) 2 8)
xx
x x x x
xx
x x x x
→− →−
+ − + + +
==
++
+ + + + +
30. Ta có
2 2 2
5 6 2 4( 2) 4
lim lim lim 1
2
( 2)( 5 6 2) ( 5 6 2)
x x x
x x x
x
x x x x x
→ → →
− − + −
= = =
−
− − + + − + +
.
31. Ta có
11
3 3 3( 1)( 3 2 2)
lim lim
1
3 2 2
xx
x x x x
x
xx
→− →−
+ + + + +
=
+
+ − +
1
lim3( 3 2 2) 6
x
xx
→−
= + + + =
.
32. Ta có
22
2
22
33
2 6 2 6 4 1
lim lim
4 3 3
( 1)( 2 6 2 6)
xx
x x x x
xx
x x x x x
→→
− + − + − −
==
−+
− − + + + −
.
33. Ta có
2
4
22
11
2 1 1
lim lim 0
( 1)( 2 1 )
xx
x x x x
xx
x x x x x x
→− →−
+ + − − +
==
+
− + + + + −
.
34. Ta có
22
2 2 7 3 3
lim lim
2
7 3 2 2
xx
xx
xx
→→
− + + +
= − = −
+ − + +
.
35. Ta có
99
3 5 2 2
lim lim
3
5 2 3
xx
xx
xx
→→
− − +
= − = −
− − +
.
36. Ta có
11
3 1 3 2( 8 3)
lim lim 3
8 3 3 1 3
xx
x x x
x x x
→→
+ − + + +
==
+ − + + +
.
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
57
37. Ta có
22
2 2 1 3 1
lim lim
4
1 3 2 2
xx
x x x x
x x x x
→→
+ − − + −
= = −
− − − + +
.
38. Ta có
11
3 2 4 5 3 6 3
lim lim
2
4 5 3 6 3 2
xx
x x x
x x x
→→
+ − + + +
==
+ − + + +
.
39. Ta có
33
1 3 5 2 3 6
lim 2lim 3
2 3 6 1 3 5
xx
x x x x
x x x x
→→
+ − + + + +
= − = −
+ − + + + +
.
40. Ta có
22
22
22
2 1 2 5 1 3 2 5
lim lim2
3
1 3 2 1 2 5
xx
x x x x
x x x x
→→
+ − + + + +
==
+ − + + + +
.
41. Ta có
2
32
2
11
1 3 ( 3 ) 4
lim lim
5 4 3 3
33
xx
x x x x
x x x
x x x
→→
− + − −
= = −
− + − −
+ + −
.
42. Ta có
4
32
11
4
44
4 3 1 4
lim lim 1
1
(4 3) (4 3) 4 3 1
xx
x
x
x x x
→→
−−
==
−
− + − + − +
.
43. Ta có
4 3 2
2
1 3 3
lim 1
22
x
x x x x
x
→
− + − + +
=
−
.
Bài 3. Tính các giới hạn sau:
1.
3
2
42
lim
2
x
x
x
→
−
−
. ĐS:
1
3
. 2.
3
1
5 3 2
lim
1
x
x
x
→−
−+
+
ĐS:
5
12
.
3.
3
2
0
11
lim
x
x
xx
→
−−
+
ĐS:
1
3
. 4.
3
1
2 5 3
lim
1
x
x
x
→
−+
−
ĐS:
5
12
−
.
5.
3
2
3
3
lim
12
x
x
x
→
−
−−
ĐS:
2
. 6.
3
1
1
lim
12
x
x
x
→
−
+−
ĐS:
3
.
7.
3
2
1
54
lim
21
x
xx
xx
→
−−
−−
ĐS:
2
9
. 8.
3
1
1
lim
1
x
x
x
→
−
−
ĐS:
3
.
9.
3
3
2
3
27
lim
1 4 28
x
x
xx
→
−
+ − +
ĐS:
54
. 10.
3
3
3
52
lim
30
x
x
xx
→
+−
+−
ĐS:
1
336
.
11.
3
3
2
1
10 2 1
lim
32
x
xx
xx
→−
+ + −
++
. ĐS:
3
2
. 12.
3
2
1
1
lim
32
x
x
x
→
−
+ + −
ĐS:
2
3
.
13.
3
1
1
lim
72
x
x
x
→
−
+−
. ĐS:
6
. 14.
2
3
1
32
lim
1
x
x
x
→−
+−
+
ĐS:
3
2
−
.
15.
33
1
21
lim
1
x
xx
x
→
−−
−
. ĐS:
2
3
. 16.
3
2
1
1
lim
21
x
x
x
→
−
−+
ĐS:
1
.
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
58
17.
3
3
1
1
lim
4 4 2
x
x
x
→
−
+−
. ĐS:
1
. 18.
33
2
1
2
lim
1
x
xx
x
→−
++
−
ĐS:
1
3
−
.
19.
33
3
1
9 2 6
lim
1
x
xx
x
→
+ + −
+
. ĐS:
1
12
. 20.
3
3
3
19 2
lim
4 3 3
x
x
x
→
−+
−−
ĐS:
27
8
−
.
21.
33
2
0
11
lim
4
x
xx
xx
→
+ − −
−
. ĐS:
1
6
−
. 22.
3
3
1
2 1 1
lim
1
x
x
x
→
−−
−
ĐS:
2
9
.
23.
3
2
32
lim
3 2 2
x
xx
x
→
+−
−−
. ĐS:
1−
. 24.
3
4
0
11
lim
2 1 1
x
x
x
→
+−
+−
ĐS:
2
3
.
Li giải
1) Ta có
3
3
2
22
3
4 2 4 1
lim lim .
23
16 2 4 4
xx
x
x
xx
→→
−
==
−
++
2) Ta có
( )
3
2
11
3
3
5 3 2 5 5
lim lim .
1 12
5 3 2 5 3 4
xx
x
x
xx
→− →−
−+
==
+
− − − +
3) Ta có
( ) ( )
( )
3
2
00
2
3
3
1 1 1 1
lim lim .
3
1 1 1 1
xx
x
xx
x x x
→→
−−
==
+
+ + − + −
4) Ta có
( )
3
2
11
3
3
2 5 3 5 5
lim lim .
1 12
4 2 5 3 5 3
xx
x
x
xx
→→
− + −
= = −
−
+ + + +
5) Ta có
( )
2
3
22
3
3
2
33
1 2 1 4
3
lim lim 2.
3
12
xx
xx
x
x
x
→→
− + − +
−
==
+
−−
6) Ta có
( )
( )
2
3
3
3
11
1
lim lim 1 2 2 3.
12
xx
x
xx
x
→→
−
= − − + − =
+−
7) Ta có
( ) ( )
( )
2
3
2
11
2
3
3
5 4 4 2
lim lim .
2 1 9
2 1 5 4 5 4 4
xx
x x x x
xx
x x x
→→
− − − − +
==
−−
+ − + − +
8) Ta có
(
)
3
2
3
3
11
1
lim lim 1 3.
1
xx
x
xx
x
→→
−
= + + =
−
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
59
9) Ta có
3
3
2
3
27
lim
1 4 28
x
x
xx
→
−
+ − +
( )
( )
( ) ( )
( )
( )
( )
2
2
3
2 2 2
3
2
3
3 3 9 1 1 4 28 4 28
lim
3 2 9
x
x x x x x x x
x x x
→

− + + + + + + + +


=
− + +
( )
( ) ( )
( )
2
2
3
2 2 2
3
2
3
3 9 1 1 4 28 4 28
lim 72.
29
x
x x x x x x
xx
→

+ + + + + + + +


==
++
10) Ta có
( )
( )
3
3
33
2
2
3
3
5 2 1 1
lim lim .
30 336
3 10 5 5 4
xx
x
xx
x x x x
→→
+−
==
+−

+ + + + + +


11) Ta có
3
3
2
1
10 2 1
lim
32
x
xx
xx
→−
+ + −
++
( )( )
( )
( ) ( )
32
1
2
2
3
33
3
3 3 3 9
lim
1 2 10 2 1 10 2 1
x
x x x
x x x x x x
→−
− + +
=

+ + + + − + + −


( )
( )
( ) ( )
2
1
2
2
3
33
3
3 6 9 3
lim .
2
2 10 2 1 10 2 1
x
xx
x x x x x
→−
−+
==

+ + + − + + −


12) Ta có
( )
(
)
2
3
2
11
3
2
3
1 3 2 2
lim lim .
3
32
11
xx
xx
x
x x x
→→
− + +
==
+−
+ + +
13) Ta có
( )
2
3
3
3
11
7 2 7 4
1
lim lim 6.
7 2 1
xx
xx
x
xx
→→
+ + + +
−
==
+ − +
14) Ta có
( )
(
)
3
2
3
2
3
2
11
11
3 2 3
lim lim .
2
1
32
xx
x x x
x
x
x
→− →−
− − +
+−
= = −
+
++
15) Ta có
( ) ( )
33
2
11
3
2
3
3
2 1 1 2
lim lim .
3
1
2 1 2 1
xx
x x x
x
x x x x
→→
− − +
==
−
− + − +
16) Ta có
( )
2
3
3
3
3
3
2
11
3
2 2 1
1
lim lim 1.
21
1
xx
xx
x
x
xx
→→
− − − +
−
==
−+
++
17) Ta có
( )
(
)
2
3
3
3
3
11
3
2
3
4 4 2 4 4 4
1
lim lim 1.
4 4 2
41
xx
xx
x
x
xx
→→
+ + + +
−
==
+−
++
18) Ta có
( ) ( ) ( )
33
2
11
2
3
2
3
3
2 2 1
lim lim .
13
1 2 2
xx
xx
x
x x x x x
→− →−
++
= = −
−

− + − + +


Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
60
19) Ta có
33
3
1
9 2 6
lim
1
x
xx
x
→−
+ + −
+
( )
( ) ( )( ) ( )
1
22
2
33
3
31
lim .
2
1 9 9 2 6 2 6
x
x x x x x x
→−
==

− + + − + − + +


20) Ta có
( )
( )
( )
2
3
3
33
2
3
33
3
9 3 4 3 3
19 2 27
lim lim .
8
4 3 3
4 19 2 19 4
xx
x x x
x
x
xx
→→
− + − +
−+
= = −

−−
− − − − +


21) Ta có
( ) ( ) ( )
33
2
00
22
3
2
33
1 1 2 1
lim lim .
46
4 1 1 1
xx
xx
xx
x x x x
→→
+ − −
= = −
−

− + + − + −


22) Ta có
( )
( )
3
3
11
2
2
3
3
2 1 1 2 2
lim lim .
19
1 2 1 2 1 1
xx
x
x
x x x x
→→
−−
==
−

+ + − + − +


23) Ta có
( )
( )
( )
2
3
22
2
2
3
3
1 3 2 2
32
lim lim 1.
3 2 2
3 3 2 3 2
xx
xx
xx
x
x x x x
→→
− + − +
+−
= = −

−−
+ + + +


24) Ta có
( )( )
( )
4
3
4
00
2
3
3
2 1 1 2 1 1
1 1 2
lim lim .
3
2 1 1
2 1 1 1
xx
xx
x
x
xx
→→
+ + + +
+−
==

+−
+ + + +


Bài 4. Tính các giới hạn sau:
1)
0
9 16 7
lim .
x
xx
x
→
+ + + −
ĐS:
7
24
2)
1
2 2 5 4 5
lim .
1
x
xx
x
→
+ + + −
−
ĐS:
4
3
3)
3
2 6 2 2 8
lim .
3
x
xx
x
→
+ + − −
−
ĐS:
5
6
4)
0
2 1 4 4
lim .
x
xx
x
→
+ + + −
ĐS:
5
4
5)
2
2 7 7
lim .
2
x
x x x
x
→
+ + + −
−
ĐS:
8
3
6)
2
2
2 1 8
lim .
2
x
x x x
x
→
− + −
−
ĐS:
8
7)
( )
6
5 4 2 3 84
lim .
6
x
x x x
x
→
− − + −
−
ĐS:
74
3
8)
3
0
1 2 1 3
lim .
x
xx
x
→
+ − +
ĐS:
0
9)
3
32
1
73
lim .
1
x
xx
x
→
+ − +
−
ĐS:
1
4
−
10)
3
2
2
8 11 7
lim .
32
x
xx
xx
→
+ − +
−+
ĐS:
7
54
11)
3
0
2 1 8
lim .
x
xx
x
→
+ − −
ĐS:
13
12
12)
3
2
1
3 5 3
lim .
1
x
xx
x
→
+ − +
−
ĐS:
1
4
13)
2
3
1
75
lim .
1
x
xx
x
→
+ − −
−
ĐS:
7
12
14)
3
2
3 2 3 2
lim .
2
x
xx
x
→
+ − −
−
ĐS:
1
2
−
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
61
15)
3
2
3 2 5 6
lim .
2
x
xx
x
→
+ − −
−
ĐS:
1−
16)
3
2
2
2
2 4 11 7
lim .
4
x
x x x
x
→
+ + − +
−
ĐS:
5
.
72
17)
3
32
2
1
57
lim .
1
x
xx
x
→
− − +
−
ĐS:
11
24
−
18)
3
3
2
2
3 4 24 2 8 2 3
lim .
4
x
x x x
x
→
− + + − −
−
ĐS:
17
16
−
19)
3
2
2
1
3 2 4 2
lim .
32
x
x x x
xx
→
− − − −
−+
ĐS:
5
6
20)
3
2
1
2 1 3 2 2
lim .
1
x
x x x
x
→
− + − −
−
ĐS:
3
2
21)
3
2
4
2
0
1 1 2
lim .
x
xx
xx
→
+ − −
+
ĐS:
1
2
22)
3
4
2
6 7 2
lim .
2
x
xx
x
→
+ − +
−
ĐS:
13
96
−
23)
0
1 4 . 1 6 1
lim .
x
xx
x
→
+ + −
ĐS:
5
24)
3
0
1 2 . 1 4 1
lim .
x
xx
x
→
+ + −
ĐS:
7
3
25)
3
1
3 1. 2 2
lim .
1
x
xx
x
→
+ − −
−
ĐS:
1
12
26)
3
2
0
4 . 8 3 4
lim .
x
xx
xx
→
+ + −
+
ĐS:
1
27)
2
0
4 4 9 6 5
lim .
x
xx
x
→
+ + − −
ĐS:
5
12
−
28)
3
2
0
1 2 1 3
lim .
x
xx
x
→
+ − +
ĐS:
1
2
29)
( )
2
2
1
6 3 2 5
lim .
1
x
x x x
x
→
+ + −
−
ĐS:
11
6
30)
2
1
4 3 2 1 3 1
lim .
21
x
x x x
xx
→
− + − − +
−+
ĐS:
5
.
2
−
31)
2
1
3 7 4 3 2 2 1
lim .
21
x
x x x
xx
→
− − + + + −
−+
ĐS:
17
16
−
32)
2
2
2
44
lim .
2 8 2 2 3 4
x
xx
x x x
→
−+
+ − − + −
ĐS:
8
9
33)
3
2
32
1
6 2 2
lim .
1
x
xx
x x x
→
+−
− − +
ĐS:
1
8
34)
( )
3
22
2
2
2 6 5 3 9 7
lim
2
x
x x x x
x
→
− + − − +
−
ĐS:
1
2
35)
3
2
0
1 2 1 3
lim .
x
xx
x
→
+ − +
ĐS:
1
2
36)
3
2
0
1 4 1 6
lim .
x
xx
x
→
+ − +
ĐS:
2
Li giải
1)
0
9 16 7
lim .
x
xx
I
x
→
+ + + −
=
Ta có
0
9 3 16 4
lim
x
xx
I
xx
→

+ − + −
=+



( )( )
( )
( )( )
( )
0
9 3 9 3 16 4 16 4
lim
9 3 16 4
x
x x x x
x x x x
→

+ − + + + − + +

=+

+ + + +

( ) ( ) ( ) ( )
00
9 9 16 16
lim lim
9 3 16 4 9 3 16 4
xx
x x x x
x x x x x x x x
→→
+ − + −
= + = +
+ + + + + + + +
0
1 1 1 1 7
lim .
6 8 24
9 3 16 4
x
xx
→

= + = + =

+ + + +

Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
62
2)
1
2 2 5 4 5
lim .
1
x
xx
I
x
→
+ + + −
=
−
Ta có
1
2 2 2 5 4 3
lim
11
x
xx
I
xx
→

+ − + −
=+


−−

( )( )
( )
( )
( )( )
( )
( )
1
2 2 2 2 2 2 5 4 3 5 4 3
lim
1 2 2 2 1 5 4 3
x
x x x x
x x x x
→

+ − + + + − + +

=+

− + + − + +

( )
( )
( )
( )
1
2 2 4 5 4 9
lim
1 2 2 2 1 5 4 3
x
xx
x x x x
→

+ − + −

=+

− + + − + +

( )
( )
( )
( )
( )
( )
1
2 1 5 1
lim
1 2 2 2 1 5 4 3
x
xx
x x x x
→

−−

=+

− + + − + +

1
2 5 2 5 4
lim .
4 6 3
2 2 2 5 4 3
x
xx
→

= + = + =

+ + + +

3)
3
2 6 2 2 8
lim .
3
x
xx
I
x
→
+ + − −
=
−
Ta có
3
2 6 6 2 2 2
lim
33
x
xx
I
xx
→

+ − − −
=+


−−

( )( )
( )
( )
( )( )
( )
( )
3
2 6 3 6 3 2 2 2 2 2 2
lim
3 6 3 3 2 2 2
x
x x x x
x x x x
→

+ − + + − − − +

=+

− + + − − +

( )
( )
( )
( )
( )
3
2 6 9
2 2 4
lim
3 6 3 3 2 2 2
x
x
x
x x x x
→

+−
−−

=+

− + + − − +

( )
( )
( )
( )
( )
( )
3
2 3 2 3
lim
3 6 3 3 2 2 2
x
xx
x x x x
→

−−

=+

− + + − − +

3
2 2 2 2 5
lim .
6 4 6
6 3 2 2 2
x
xx
→

= + = + =

+ + − +

4)
0
2 1 4 4
lim .
x
xx
I
x
→
+ + + −
=
Ta có
0
2 1 2 4 2
lim
x
xx
I
xx
→

+ − + −
=+



( )( )
( )
( )( )
( )
0
2 1 1 1 1 4 2 4 2
lim
1 1 4 2
x
x x x x
x x x x
→

+ − + + + − + +

=+

+ + + +

( )
( ) ( )
0
2 1 1
44
lim
1 1 4 2
x
x
x
x x x x
→

+−
+−

=+

+ + + +

0
2 1 2 1 5
lim
2 4 4
1 1 4 2
x
xx
→

= + = + =

+ + + +

5)
2
2 7 7
lim .
2
x
x x x
I
x
→
+ + + −
=
−
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
63
Ta có
( )
2
2 2 2 2 4 7 3
lim
2
x
x x x x
I
x
→
− + + + − + + −
=
−
2
2 2 4 7 3
lim 2
22
x
xx
x
xx
→

+ − + −
= + + +


−−

( )( )
( )
( )
( )( )
( )
( )
2
2 2 2 2 2 7 3 7 3
2 lim
2 2 2 2 7 3
x
x x x x
x x x x
→

+ − + + + − + +

= + +

− + + − + +

2
2 1 2 1 8
2 lim 2 .
4 6 3
2 2 7 3
x
xx
→

= + + = + + =

+ + + +

6)
2
2
2 1 8
lim .
2
x
x x x
I
x
→
− + −
=
−
Ta có
( )
2
2
2 2 1 4 1 4 4
lim
2
x
x x x x
I
x
→
− − + − − + −
=
−
2
2
4 1 4 4
lim 2 1
22
x
xx
x
xx
→

− − −
= − + +


−−

( )( )
( )
( )
( )( ) ( )
( )
( )
22
4 1 1 1 1
2 2 4 1 1
2 lim 2 lim 2
2
2 1 1 2 1 1
xx
xx
x x x
x
x
x x x x
→→
− − − +
− + − −
= + + = + + +
−
− + + − + +
2
44
2 lim 2 2 4 8.
2
11
x
x
x
→

= + + + = + + =

−+

7)
( )
6
5 4 2 3 84
lim .
6
x
x x x
I
x
→
− − + −
=
−
Ta có
( )
6
5 30 2 3 26 2 3 78 6
lim
6
x
x x x x
I
x
→
− − + − − + −
=
−
( )
( )
6
26 2 3 3
5 6 2 3
6
lim
6 6 6
x
x
xx
x
x x x
→

−−
−−
−

= + +

− − −

( )( )
( )
( )
6
26 2 3 3 2 3 3
lim 5 2 3 1
6 2 3 3
x
xx
x
xx
→

− − − +

= − + +

− − +

( )
( )
( )
( )
( )
( )
66
26 2 3 9 26.2 6
15 lim 1 15 lim 1
6 2 3 3 6 2 3 3
xx
xx
x x x x
→→
− − −
= + + = + +
− − + − − +
6
52 52 74
15 lim 1 15 1 .
63
2 3 3
x
x
→
= + + = + + =
−+
8)
3
0
1 2 1 3
lim .
x
xx
I
x
→
+ − +
=
Ta có
33
00
1 2 1 1 1 3 1 2 1 1 1 3
lim lim
xx
x x x x
I
x x x
→→

+ − + − + + − − +
= = +



( )( )
( )
( )
( )
( )
( )
( )
2
33
3
0
2
3
3
1 1 3 1 1 3 1 3
1 2 1 1 2 1
lim
1 2 1
1 1 3 1 3
x
x x x
xx
xx
x x x
→

− + + + + +
+ − + +

=+

++
+ + + +


Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
64
( )
( )
( )
( )
( )
2
00
2
3
3
3
3
1 1 3
1 2 1 2 3
lim lim
1 2 1
1 2 1
1 1 3 1 3
1 1 3 1 3
xx
x
x
x
xx
xx
x x x
→→


−+

+ − −

= + = +


++
++
+ + + +
+ + + +



23
0.
23
−
= + =
9)
3
32
1
73
lim .
1
x
xx
I
x
→
+ − +
=
−
Ta có
3
32
1
7 2 2 3
lim
1
x
xx
I
x
→
+ − + − +
=
−
3
32
1
7 2 2 3
lim
11
x
xx
xx
→

+ − − +
=+


−−

(
)
( )
( )
( )
(
)
(
)
( )
(
)
2
33
3 3 3
3
22
1
2
2
3
33
3
7 2 7 2 7 4
2 3 2 3
lim
1 2 3
1 7 2 7 4
x
x x x
xx
xx
x x x
→


+ − + + + +

− + + +



=+


− + +
− + + + +




( )
( )
( )
( )
(
)
2
3
1
2
2
3
33
3
43
78
lim
1 2 3
1 7 2 7 4
x
x
x
xx
x x x
→


−+
+−

=+


− + +
− + + + +




( )
( )
( )
(
)
32
1
2
2
3
33
3
11
lim
1 2 3
1 7 2 7 4
x
xx
xx
x x x
→


−−

=+


− + +
− + + + +




( )
2
22
1
3
33
3
1 1 3 2 1
lim .
12 4 4
23
7 2 7 4
x
x x x
x
xx
→

+ + +

= − = − = −

++
+ + + +


10)
3
2
2
8 11 7
lim .
32
x
xx
I
xx
→
+ − +
=
−+
Ta có
33
2 2 2
22
8 11 3 3 7 8 11 3 3 7
lim lim
3 2 3 2 3 2
xx
x x x x
I
x x x x x x
→→

+ − + − + + − − +
= = +


− + − + − +

( )
( )
( )
( )
( )
( )
( )( )
( )
( )
2
33
3
2
2
2
2
3
3
8 11 3 8 11 3 8 11 9
3 7 3 7
lim
3 2 3 7
3 2 8 11 3 8 11 9
x
x x x
xx
x x x
x x x x
→

+ − + + + +
− + + +

=+

− + + +
− + + + + +


( )
( )
( )
( )
( )
( )
2
2
2
2
3
3
97
8 11 27
lim
3 2 3 7
3 2 8 11 3 8 11 9
x
x
x
x x x
x x x x
→

−+

+−
=+

− + + +
− + + + + +


Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
65
( )
( )( ) ( )
( )
( )( )
( )
2
2
3
3
82
2
lim
1 2 3 7
1 2 8 11 3 8 11 9
x
x
x
x x x
x x x x
→

−

−
=+

− − + +
− − + + + +


( ) ( )
( )
( )
( )
2
2
3
3
8 1 8 1 7
lim .
27 6 54
1 3 7
1 8 11 3 8 11 9
x
xx
x x x
→


= − = − =

− + +
− + + + +


11)
3
0
2 1 8
lim .
x
xx
I
x
→
+ − −
=
Ta có
33
00
2 1 2 2 8 2 1 2 2 8
lim lim
xx
x x x x
I
x x x
→→

+ − + − − + − − −
= = +



( )( )
( )
( )
( )
( )
( )
( )
2
33
3
0
2
3
3
2 8 4 2 8 8
2 1 1 1 1
lim
11
4 2 8 8
x
x x x
xx
xx
x x x
→

− − + − + −
+ − + +

=+

++
+ − + −


( )
( )
( )
( )
( )
0
2
3
3
2 1 1 8 8
lim
11
4 2 8 8
x
xx
xx
x x x
→

+ − − −

=+

++
+ − + −


( )
2
0
3
3
2 1 2 1 13
lim .
2 12 12
11
4 2 8 8
x
x
xx
→


= + = + =

++
+ − + −

12)
3
2
1
3 5 3
lim .
1
x
xx
I
x
→
+ − +
=
−
Ta có
33
22
11
3 5 2 2 3 3 5 2 2 3
lim lim
1 1 1
xx
x x x x
I
x x x
→→

+ − + − + + − − +
= = +


− − −

(
)
( )
( )
( )
( )( )
( )
( )
2
33
2 2 2
3
1
2
3
22
3
3 5 2 3 5 2 3 5 4
2 3 2 3
lim
1 2 3
1 3 5 2 3 5 4
x
x x x
xx
xx
x x x
→


+ − + + + +


− + + +


=+


− + +
− + + + +




( )
( )
( )
( )
( )
2
1
2
3
22
3
43
3 5 8
lim
1 2 3
1 3 5 2 3 5 4
x
x
x
xx
x x x
→


−+
+−

=+


− + +
− + + + +




( )
( )
( )
( )
( )
2
1
2
3
22
3
31
1
lim
1 2 3
1 3 5 2 3 5 4
x
x
x
xx
x x x
→


−
−

=+


− + +
− + + + +




Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
66
( )
( )
2
1
3
22
3
31
1 6 1 1
lim .
12 4 4
23
3 5 2 3 5 4
x
x
x
xx
→

+

= − = − =

++
+ + + +


13)
2
3
1
75
lim .
1
x
xx
I
x
→
+ − −
=
−
Ta có
22
33
11
7 2 2 5 7 2 2 5
lim lim
1 1 1
xx
x x x x
I
x x x
→→

+ − + − − + − − −
= = +


− − −

( )
( )
( )
( ) ( )
( )
(
)
(
)
( )
(
)
2
33
22
3
1
2
2
3
3
7 2 7 2 7 4
2 5 2 5
lim
1 2 5
1 7 2 7 4
x
x x x
xx
xx
x x x
→

+ − + + + +
− − + −

=+

− + −
− + + + +


( ) ( )
( )
( )
( )
(
)
2
1
2
2
3
3
45
78
lim
1 2 5
1 7 2 7 4
x
x
x
xx
x x x
→

−−

+−
=+

− + −
− + + + +


( ) ( )
( )
( )
(
)
2
1
2
2
3
3
11
lim
1 2 5
1 7 2 7 4
x
xx
xx
x x x
→


−−
=+

− + −
− + + + +


( )
22
1
3
3
1 1 1 2 7
lim .
12 4 12
25
7 2 7 4
x
x
x
xx
→

+

= + = + =

+−
+ + + +

14)
3
2
3 2 3 2
lim .
2
x
xx
I
x
→
+ − −
=
−
Ta có
33
22
3 2 2 2 3 2 3 2 2 2 3 2
lim lim
2 2 2
xx
x x x x
I
x x x
→→

+ − + − − + − − −
= = +


− − −

( )
( )
( )
( ) ( )
( )
( )( )
( )
( )
2
33
3
2
2
3
3
3 2 2 3 2 2 3 2 4
2 3 2 2 3 2
lim
2 2 3 2
2 3 2 2 3 2 4
x
x x x
xx
xx
x x x
→

+ − + + + +
− − + −

=+

− + −
− + + + +


( ) ( )
( )
( )
( )
( )
2
2
3
3
4 3 2
3 2 8
lim
2 2 3 2
2 3 2 2 3 2 4
x
x
x
xx
x x x
→

−−

+−
=+

− + −
− + + + +


( )
( ) ( )
( )
( )
( )
2
2
3
3
32
63
lim
2 2 3 2
2 3 2 2 3 2 4
x
x
x
xx
x x x
→

−

−
=+

− + −
− + + + +


( )
2
2
3
3
3 3 3 3 1
lim .
12 4 2
2 3 2
3 2 2 3 2 4
x
x
xx
→

−

= − = + = −

+−
+ + + +

Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
67
15)
3
2
3 2 5 6
lim .
2
x
xx
I
x
→
+ − −
=
−
Ta có
33
22
3 2 2 2 5 6 3 2 2 2 5 6
lim lim
2 2 2
xx
x x x x
I
x x x
→→

+ − + − − + − − −
= = +


− − −

( )
( )
( )
( ) ( )
( )
( )( )
( )
( )
2
33
3
2
2
3
3
3 2 2 3 2 2 3 2 4
2 5 6 2 5 6
lim
2 2 5 6
2 3 2 2 3 2 4
x
x x x
xx
xx
x x x
→

+ − + + + +
− − + −

=+

− + −
− + + + +


( ) ( )
( )
( )
( )
( )
2
2
3
3
4 5 6
3 2 8
lim
2 2 5 6
2 3 2 2 3 2 4
x
x
x
xx
x x x
→

−−

+−
=+

− + −
− + + + +


( )
( ) ( )
( )
( )
( )
2
2
3
3
32
10 5
lim
2 2 5 6
2 3 2 2 3 2 4
x
x
x
xx
x x x
→

−

−
=+

− + −
− + + + +


( )
2
2
3
3
3 5 3 5
lim 1.
12 4
2 5 6
3 2 2 3 2 4
x
x
xx
→

−

= − = + = −

+−
+ + + +

16)
3
2
2
2
2 4 11 7
lim .
4
x
x x x
I
x
→
+ + − +
=
−
Ta có
33
22
2 2 2
22
2 4 11 3 3 7 2 4 11 3 3 7
lim lim
4 4 4
xx
x x x x x x
I
x x x
→→

+ + − + − + + + − − +
= = +


− − −

(
)
( )
( ) ( )
( )( )
( )
( )
2
33
2 2 2
3
2
2
2
3
2 2 2
3
2 4 11 3 2 4 11 3 2 4 11 9
3 7 3 7
lim
4 3 7
4 2 4 11 3 2 4 11 9
x
x x x x x x
xx
xx
x x x x x
→


+ + − + + + + + +


− + + +


=+


− + +
− + + + + + +




( ) ( )
( )
( )
( )
2
2
2
2
3
2 2 2
3
97
2 4 11 27
lim
4 3 7
4 2 4 11 3 2 4 11 9
x
x
xx
xx
x x x x x
→


−+
+ + −

=+


− + +
− + + + + + +




( ) ( )
( )
( )
2
2
2
2
3
2 2 2
3
2 4 16 2
lim
4 3 7
4 2 4 11 3 2 4 11 9
x
x x x
xx
x x x x x
→


+ − −

=+


− + +
− + + + + + +




( )( )
( ) ( )
( )
( )
2
2
2
3
2 2 2
3
2 2 4
2
lim
4 3 7
4 2 4 11 3 2 4 11 9
x
xx
x
xx
x x x x x
→


−+
−

=+


− + +
− + + + + + +




Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
68
( )
( )
( )
( )
( )
2
2
3
22
3
24
1 12 1 5
lim .
108 24 72
2 3 7
2 2 4 11 3 2 4 11 9
x
x
xx
x x x x x
→


+
−

= − = + =


+ + +
+ + + + + + +




17)
3
32
2
1
57
lim .
1
x
xx
I
x
→
− − +
=
−
Ta có
33
3 2 3 2
2 2 2
11
5 2 2 7 5 2 2 7
lim lim
1 1 1
xx
x x x x
I
x x x
→→

− − + − + − − − +
= = +


− − −

(
)
(
)
( )
(
)
(
)
( )
( ) ( )
2
33
2 2 2
3
33
1
2
23
3
2 2 2
3
2 7 4 2 7 7
5 2 5 2
lim
1 5 2
1 4 2 7 7
x
x x x
xx
xx
x x x
→


− + + + + +

− − − +



=+


− − +
− + + + +




( )
(
)
( )
( ) ( )
2
3
1
2
23
3
2 2 2
3
87
54
lim
1 5 2
1 4 2 7 7
x
x
x
xx
x x x
→


−+
−−

=+


− − +
− + + + +




( )
(
)
( ) ( )
32
1
2
23
3
2 2 2
3
11
lim
1 5 2
1 4 2 7 7
x
xx
xx
x x x
→


−−

=+


− − +
− + + + +




( )
( )
(
)
( )
2
2
1
3
3
22
3
1
1 3 1 11
lim .
8 12 24
1 5 2
4 2 7 7
x
xx
xx
xx
→

− + +
−−

= + = − + = −

+ − +
+ + + +


18)
3
3
2
2
3 4 24 2 8 2 3
lim .
4
x
x x x
I
x
→
− + + − −
=
−
Ta có
3
3
2
2
3 4 24 6 2 2 8 8 2 3
lim
4
x
x x x
I
x
→
− − + + − + − −
=
−
1 2 3
3
3
2 2 2
2 2 2
3 4 24 6 2 2 8 8 2 3
lim lim lim
4 4 4
x x x
I I I
x x x
x x x
→ → →
− − + − − −
= + +
− − −
1
I
3
3
2
2
3 4 24 6
lim
4
x
x
x
→
−−
=
−
(
)
( )
2
3 3 3
3 3 3 2
2
2
33
2 3 3 2
3 4 24 2 4 24 4 24.2 2
lim
4 4 24 4 24.2 2
x
x x x
x x x
→

− − − + − +


=

− − + − +


Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
69
( )
( )
(
)
3
2
2
33
2 3 3 2
3 4 24 8
lim
4 4 24 4 24.2 2
x
x
x x x
→
−−
=

− − + − +


( )
( )
(
)
3
2
2
33
2 3 3 2
3.4 8
lim
4 4 24 4 24.2 2
x
x
x x x
→
−
=

− − + − +


( )
( )
( )( )
(
)
2
2
2
33
3 3 2
12 2 2 4
lim
2 2 4 24 4 24.2 2
x
x x x
x x x x
→
− + +
=

− + − + − +


( )
( )
(
)
2
2
2
33
3 3 2
12 2 4
lim
2 4 24 4 24.2 2
x
xx
x x x
→
− + +
=

+ − + − +


144
3
48
= − = −
.
2
I
2
2
22
lim
4
x
x
x
→
+−
=
−
( )( )
( )
( )
2
2
2 2 2 2
lim
4 2 2
x
xx
xx
→
+ − + +
=
− + +
( )
( )( )
( )
2
24
lim
2 2 2 2
x
x
x x x
→
+−
=
− + + +
( )
( )
11
lim
16
2 2 2
x
xx
→
−
= = −
+ + +
3
I
2
2
8 8 2 3
lim
4
x
x
x
→
−−
=
−
( )( )
( )
( )
2
2
8 1 2 3 1 2 3
lim
4 1 2 3
x
xx
xx
→
− − + −
=
− + −
( )
( )
( )( )
2
8 1 2 3
lim
22
x
x
xx
→
−−
=
+−
( )
( )( )
( )
2
8.2 2
lim
2 2 1 2 3
x
x
x x x
→
−
=
− + + +
( )
( )
2
16
lim
2 1 1 2 3
x
xx
→
=
+ + + −
16
2
8
==
1
32
16
I = − − +
17
16
=−
.
Bài 5. Tính các giới hạn sau:
1
( )
3
lim 2 3
x
xx
→+
−
ĐS:
+
2.
( )
32
lim 3 2
x
xx
→−
−+
ĐS:
−
3.
( )
32
lim 6 9 1
x
x x x
→+
− − + +
ĐS:
−
4.
( )
3
lim 3 1
x
xx
→−
− + −
ĐS:
+
5.
( )
42
lim 2 1
x
xx
→+
−+
ĐS:
+
6.
( )
42
lim 8 10
x
xx
→−
−+
ĐS:
+
7.
( )
42
lim 2 3
x
xx
→+
− + +
ĐS:
−
8.
( )
42
lim 6
x
xx
→−
− − +
ĐS:
−
9.
2
lim 3 4
x
xx
→
−+
ĐS:
+
10.
(
)
2
lim 2 1
x
xx
→−
++
ĐS:
+
11.
(
)
2
lim 1 2
x
x x x
→−
+ + +
ĐS:
−
12.
(
)
2
lim 4 1
x
x x x
→+
+ + −
ĐS:
+
13.
( )
lim 1 9 1
x
xx
→+
+ − +
ĐS:
−
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
70
14.
( )
lim 16 7 9 3
x
xx
→−
+ + +
ĐS: không tn tại giới hạn
Li giải
1.
( )
3
lim 2 3
x
I x x
→+
=−
Ta có
( )
3
lim 2 3
x
I x x
→+
=−
3
2
3
lim 2
x
x
x
→+

= − = +


. (vì
3
lim
x
x
→+
= +
và
2
3
lim 2 2 0
x
x
→+

− =


)
2.
( )
32
lim 3 2
x
I x x
→−
= − +
.
Ta có
( )
32
lim 3 2
x
I x x
→−
= − +
3
3
32
lim 1
x
x
xx
→−

= − + = −


. (vì
3
lim
x
x
→−
= −
và
3
32
lim 1 1
x
xx
→−

− + =


).
3.
( )
32
lim 6 9 1
x
I x x x
→+
= − − + +
.
Ta có
3
23
6 9 1
lim 1
x
Ix
x x x
→+

= − − + + = −


.
(vì
3
lim
x
x
→+
= +
và
23
6 9 1
lim 1 1 0
x
x x x
→+

− − + + = −


).
4.
( )
3
lim 3 1
x
I x x
→−
= − + −
Ta có
3
23
31
lim 1
x
Ix
xx
→−

= − + − = +


. (vì
3
lim
x
x
→−
= −
và
23
31
lim 1 1 0
x
xx
→−

− + − = −


).
5.
( )
42
lim 2 1
x
I x x
→+
= − +
Ta có
4
24
21
lim 1
x
Ix
xx
→+

= − + = +


. (vì
4
lim
x
x
→+
= +
và
24
21
lim 1 1 0
x
xx
→+

− + =


).
6.
( )
42
lim 8 10
x
I x x
→−
= − +
Ta có
4
24
8 10
lim 1 1 0
x
Ix
xx
→−

= − + =


(vì
4
lim
x
x
→−
= +
và
24
8 10
lim 1 1 0
x
xx
→−

− + =


)
7.
( )
42
lim 2 3
x
I x x
→+
= − + +
Ta có
4
24
23
lim 1
x
Ix
xx
→+

= − + + = −


. ( vì
4
lim
x
x
→+
= +
và
24
23
lim 1 1 0
x
xx
→+

− + + = −


).
8.
( )
42
lim 6
x
I x x
→−
= − − +
Ta có
4
24
16
lim 1
x
Ix
xx
→−

= − − + = −


. (vì
4
lim
x
x
→−
= +
và
24
16
lim 1 1
x
xx
→−

− − + = −


)
9.
2
lim 3 4
x
I x x
→
= − +
.
Ta có
2
2
34
lim 1
x
Ix
xx
→

= − +


2
34
lim 1
x
x
xx
→

= − + = +


.
(vì
lim
x
x
→
= +
và
2
34
lim 1 1 0
x
xx
→

− + =


).
10.
(
)
2
lim 2 1
x
I x x
→−
= + +
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
71
Ta có
(
)
2
lim 2 1
x
I x x
→−
= + +
2
1
lim 2 1
x
x
x
→−

= − + + = +



.
(vì
lim
x
x
→−
= −
và
2
1
lim 2 1 2 1 0
x
x
→−

− + + = − +



).
11.
(
)
2
lim 1 2
x
I x x x
→−
= + + +
2
11
lim 1 2
x
x
xx
→−

= − + + + = −



.
(vì
lim
x
x
→−
= −
,
2
11
lim 1 2 1 0
x
xx
→−

− + + + =



).
12.
(
)
2
lim 4 1
x
I x x x
→+
= + + −
2
11
lim 4 1
x
x
xx
→+

= + + − = +



(vì
lim
x
x
→+
= +
,
2
11
lim 4 1 1 0
x
xx
→+

= + + − =



).
13.
( )
lim 1 9 1
x
I x x
→+
= + − +
11
lim 1 9
x
x
xx
→+

= + − + = −



.
(vì
lim
x
x
→+
= +
,
11
lim 1 9
x
x
xx
→+

= + − + = −



).
14.
( )
lim 16 7 9 3
x
I x x
→−
= + + +
Tập xác định của hàm số
( )
16 7 9 3f x x x= + + +
là
1
;
3
D

= − +

.
Ta có khi
x → −
hàm số
( )
16 7 9 3f x x x= + + +
không xác định. Do đó
( )
lim 16 7 9 3
x
xx
→−
+ + +
không tn tại.
Bài 6. Tính các giới hạn sau:
1.
2
lim
1
x
x
x
→+
+
−
. ĐS:
1
2.
2
lim
1
x
x
x
→−
+
. ĐS:
3.
1
lim
21
x
x
x
→+
−
−
.ĐS:
1
2
−
4.
32
lim
1
x
x
x
→−
−
+
. ĐS:
3
5.
3
32
2 3 4
lim
1
x
xx
xx
→+
+−
− − +
. ĐS:
2−
6.
( )
( )
( )
2
2
3 2 1
lim
5 1 2
x
xx
x x x
→+
−
−+
. ĐS:
6
5
7.
43
4
2 7 15
lim
1
x
xx
x
→−
+−
+
. ĐS:
8.
( )
( )
( )
( )
2
3
4 1 7 1
lim
2 1 3
x
xx
xx
→+
+−
−+
. ĐS:
9.
( ) ( )
( )
22
4
1 5 2
lim
31
x
xx
x
→−
−+
+
. ĐS:
25
81
10.
( ) ( )
( )
( )
43
5
2
1 1 2
lim
2 2 3
x
xx
xx
→−
+−
++
.ĐS:
1
4
−
11.
( )
( )
( )
( )
2
2
2
2
22
lim
2 1 1
x
xx
xx
→−
++
+−
. ĐS:
−
12.
( ) ( )
( )
34
5
2
21
lim
12
x
xx
xx
→−
+−
−
. ĐS:
1
32
−
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
72
13.
32
2
lim
3 4 3 2
x
xx
xx
→−

−

−+

. ĐS:
2
9
14.
2
3
37
lim
21
x
xx
x
→−
−+
−
.ĐS:
0
15.
3
4
22
lim
23
x
xx
xx
→+
++
++
ĐS:
16.
( )
( )
( )
( )
2
3
4 1 7 1
lim
2 1 3
x
xx
xx
→+
+−
−+
ĐS:
0
17.
( )
( )
2
2
4 1 2 3
lim
61
x
xx
xx
→−
++
−+
ĐS:
−
18.
3
2
22
lim
23
x
xx
xx
→+
++
++
ĐS:
+
19.
43
3
22
lim
23
x
x x x
xx
→−
+ + +
++
ĐS:
−
20.
43
23
22
lim
2
x
x x x
xx
→+
+ + +
−
ĐS:
−
21.
43
11
lim
27
x
xx
x
→+
−+
−
ĐS:
+
22.
42
21
lim
12
x
xx
x
→+
+−
−
ĐS:
+
23.
4
lim
12
x
xx
x
→+
−
−
ĐS:
+
24.
( )( )
53
3
23
21
lim
21
x
xx
x x x
→+
+−
−+
ĐS:
1
25.
3
3
1
lim
21
x
xx
x
→+
++
+
ĐS:
1
26.
42
21
lim
12
x
xx
x
→+
+−
−
ĐS:
−
Li giải
1.
2
lim
1
x
x
I
x
→+
+
=
−
2
1
lim
1
1
x
x
x
x
x
→+

+


=

−


2
1
lim
1
1
x
x
x
→+

+


=

−


1=
.
2.
2
lim
1
x
x
I
x
→−
=
+
2
lim
1
1
x
x
x
x
→−

+


2
lim
1
1
x
x
→−
=
+
2=
.
3.
1
lim
21
x
x
I
x
→+
−
=
−
1
1
lim
1
2
x
x
x
x
x
→+

−


=

−


1
1
1
lim
1
2
2
x
x
x
→+
−
= = −
−
.
4.
32
lim
1
x
x
I
x
→−
−
=
+
2
3
lim
1
1
x
x
x
x
x
→−

−


=

+


2
3
lim
1
1
x
x
x
→−

−


=

+


3=
.
5.
3
32
2 3 4
lim
1
x
xx
I
xx
→+
+−
=
− − +
3
23
3
3
34
2
lim
11
1
x
x
xx
x
xx
→+

+−


=

− − +


23
3
34
2
lim 2
11
1
x
xx
xx
→+

+−


=−

− − +


.
6.
2
2
2
1
3 . 2
lim
12
51
x
xx
x
I
xx
xx
→+

−


=
−+
2
1
32
6
lim
12
5
51
x
x
xx
→+

−


==
−+
.
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
73
7.
43
4
2 7 15
lim
1
x
xx
I
x
→−
+−
=
+
4
4
4
4
7 15
2
lim
1
1
x
x
xx
x
x
→−

+−


=

+


4
4
7 15
2
lim 2
1
1
x
xx
x
→−

+−


==

+


.
8.
( )
( )
( )
( )
2
3
4 1 7 1
lim
2 1 3
x
xx
I
xx
→+
+−
=
−+
2
2
3
3
11
47
lim
13
21
x
xx
xx
xx
xx
→+
+−
=
−+
2
3
11
47
lim 0
13
21
x
x
xx
x
xx
→+
+−
==
−+
.
9.
( ) ( )
( )
22
4
1 5 2
lim
31
x
xx
I
x
→−
−+
=
+
22
22
4
4
12
15
lim
1
3
x
xx
xx
x
x
→−
−+
=

+


22
4
12
15
25
lim
81
1
3
x
xx
x
→−
−+
==

+


.
10.
( ) ( )
( )
( )
43
5
2
1 1 2
lim
2 2 3
x
xx
I
xx
→−
+−
=
++
43
43
5
52
2
11
12
lim
23
21
x
xx
xx
xx
xx
→−
+−
=
++
43
5
2
11
12
1
lim
4
23
21
x
xx
xx
→−
+−
= = −
++
.
11.
( )
( )
( )
( )
2
2
2
2
22
lim
2 1 1
x
xx
I
xx
→−
++
=
+−
2
4
2
2
22
2
22
11
lim
11
21
x
xx
xx
xx
xx
→−
++
=
+−
2
2
2
2
22
. 1 1
lim
11
21
x
x
xx
xx
→−
++
= = −
+−
(vì
lim
x
x
→−
= −
,
2
2
2
2
22
11
1
lim 0
2
11
21
x
xx
xx
→−
++
=
+−
).
12.
( ) ( )
( )
34
5
2
21
lim
12
x
xx
I
xx
→−
+−
=
−
34
34
5
52
21
11
lim
1
2.
x
xx
xx
xx
x
→−
+−
=

−


34
5
21
11
1
lim
32
1
2
x
xx
x
→−
+−
= = −

−


.
13.
32
2
lim
3 4 3 2
x
xx
I
xx
→−

=−

−+

.
Ta có
32
2
lim
3 4 3 2
x
xx
I
xx
→−

=−

−+

( )
( )
( )
( )
3 2 2
2
3 2 3 4
lim
3 4 3 2
x
x x x x
xx
→−
+ − −
=
−+
( )
( )
32
2
24
lim
3 4 3 2
x
xx
xx
→−
+
=
−+
3
2
4
2
lim
42
33
x
x
x
xx
xx
→−

+


=
−+
4
2
2
lim
42
9
33
x
x
xx
→−

+


==
−+
.
14.
2
3
37
lim
21
x
xx
I
x
→−
−+
=
−
2
2
3
3
3 1 7
lim
1
2
x
x
x x x
x
x
→−

−+


=

−


2
3
3 1 7
lim 0
1
2
x
x x x
x
→−

−+


=

−


.
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
74
Bài 7. Tính các giới hạn sau:
1.
1
23
lim
1
x
x
x
+
→
−
−
. ĐS:
−
2.
2
15
lim
2
x
x
x
+
→
−
−
. ĐS:
−
3.
3
2
lim
3
x
x
x
−
→
−
−
. ĐS:
+
4.
( )
2
4
5
lim
4
x
x
x
−
→
−
−
. ĐS:
−
5.
2
31
lim
2
x
x
x
−
→
−+
−
. ĐS:
+
6.
1
31
lim
1
x
x
x
−
→
−
−
. ĐS:
−
7.
2
65
lim
48
x
x
x
+
→
−
−
. ĐS:
−
8.
2
1
lim
24
x
x
x
+
→
+
−
. ĐS:
+
9.
3
3
lim
5 15
x
x
x
+
→
−
−
. ĐS:
1
5
10.
( )
3
71
lim
3
x
x
x
−
→−
−
+
.ĐS:
−
11.
2
2
2
lim
2 5 2
x
x
xx
−
→
−
−+
. ĐS:
1
3
12.
3
1
1
lim
23
x
x
xx
+
→
−
+−
. ĐS:
1
7
13.
3
1
1
lim
23
x
x
xx
−
→
−
+−
. ĐS:
1
7
−
14.
2
2
32
lim
2
x
xx
x
+
→
−+
−
.ĐS:
1
15.
2
3
9
lim
3
x
x
x
→
−
−
ĐS: không tn tại 16.
2
4
4
lim
20
x
x
xx
→
−
+−
ĐS: không tn tại
17.
2
2
lim
11
x
x
x
−
→
−
−−
ĐS:
2
18.
3
3
lim
5 11 2
x
x
x
−
→
−
−−
ĐS:
4
5
−
19.
3
2
2
lim
11
x
x
x
−
→
−
−−
ĐS:
3−
20.
2
3
5
25
lim
41
x
x
x
−
→
−
−−
ĐS:
30−
21.
( )
2
3
38
lim
3
x
x
x
+
→
−
−
ĐS:
+
22.
3
2
2
25 3
lim
2
x
x
xx
→
+−
−−
ĐS:
1
81
23.
2
2
32
lim
4 16
x
x
x
+
→
+
−
ĐS:
+
24.
0
lim
x
xx
xx
+
→
+
−
ĐS:
1−
25.
2
2
4
lim
2
x
x
x
+
→
−
−
ĐS:
0
26.
0
2
lim
x
xx
xx
+
→
+
−
ĐS:
2−
27.
( )
( )( )
1
21
lim
11
x
xx
xx
+
→−
++
+ − −
ĐS:
1
28.
2
2
3
69
lim
9
x
xx
x
−
→
−+
−
ĐS:
1
6
−
29.
2
2
1
43
lim
65
x
xx
xx
−
→
−+
− + −
ĐS:
−
30.
( )
2
54
1
32
lim
x
xx
xx
+
→−
++
+
ĐS:
31.
( )
2
2
lim 2
4
x
x
x
x
+
→
−
−
ĐS:
0
32.
( )
( )
3
2
1
lim 1
1
x
x
x
x
+
→−
+
−
ĐS:
33.
( )
2
1
5
lim 1
23
x
x
x
xx
+
→
+
−
+−
ĐS:
34.
1
1
lim
2 1 1
x
xx
xx
−
→
−
− + −
ĐS:
1
2
35.
0
1
lim 2
x
x
x
x
+
→

−



ĐS:
36.
( )
( )
2
2
3
2 5 3
lim
3
x
xx
x
+
→−
+−
+
ĐS:
−
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
75
37.
2
2
11
lim
24
x
xx
−
→

−

−−

ĐS:
−
38.
3
2
1
32
lim
54
x
xx
xx
−
→
−+
−+
ĐS:
3
5
−
Li giải
1
1
23
lim
1
x
x
x
+
→
−
= −
−
vì
( )
( )
1
1
lim 2 3 1
lim 1 0
1 0, 1
x
x
x
x
xx
+
+
→
→
+
− = −
−=
− →
.
2.
2
15
lim
2
x
x
x
+
→
−
= −
−
vì
( )
( )
2
2
lim 15 13
lim 2 0
2 0, 2
x
x
x
x
xx
+
+
→
→
+
− = −
−=
− →
.
3.
3
2
lim
3
x
x
x
−
→
−
= +
−
vì
( )
( )
3
3
lim 2 1
lim 3 0
3 0, 3
x
x
x
x
xx
−
−
→
→
−
− = −
−=
− →
.
4.
( )
2
4
5
lim
4
x
x
x
−
→
−
= −
−
vì
( )
( )
( )
4
2
4
2
lim 5 1
lim 4 0
4 0, 4
x
x
x
x
xx
−
−
→
→
−
− = −
−=
− →
.
5.
2
31
lim
2
x
x
x
−
→
−+
= +
−
vì
( )
( )
2
2
lim 3 1 5
lim 2 0
2 0, 2
x
x
x
x
xx
−
−
→
→
−
− + = −
−=
− →
.
6.
1
31
lim
1
x
x
x
−
→
−
= −
−
vì
( )
( )
1
1
lim 3 1 2
lim 1 0
1 0, 1
x
x
x
x
xx
−
−
→
→
−
−=
−=
− →
.
7.
2
65
lim
48
x
x
x
+
→
−
= −
−
vì
( )
( )
2
2
lim 6 5 4
lim 4 8 0
4 8 0, 2
x
x
x
x
xx
+
+
→
→
+
− = −
−=
− →
.
8.
2
1
lim
24
x
x
x
+
→
+
= +
−
vì
( )
( )
2
2
lim 1 3
lim 2 4 0
2 4 0, 2
x
x
x
x
xx
+
+
→
→
+
+=
−=
− →
.
9. Do
3x
+
→
nên
33xx− = −
suy ra
33
3
3
lim lim
5 15 5 15
xx
x
x
xx
++
→→
−
−
==
−−
3
11
lim
55
x
+
→
=
.
10.
( )
3
71
lim
3
x
x
x
−
→−
−
= −
+
vì
( )
( )
( )
( )
3
3
lim 7 1 22
lim 3 0
3 0, 3
x
x
x
x
xx
−
−
→−
→−
−
− = −
+=
+ → −
.
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
76
11. Do
2x
−
→
nên
22xx− = −
suy ra
( )( )
2
22
2
2
lim lim
2 5 2 2 1 2
xx
x
x
x x x x
−−
→→
−
−
=
− + − −
2
11
lim
2 1 3
x
x
−
→
==
−
.
12. Do
1x
+
→
nên
11xx− = −
suy ra
( )
( )
3
2
11
1
1
lim lim
23
1 2 2 3
xx
x
x
xx
x x x
++
→→
−
−
=
+−
− + +
2
1
11
lim
2 2 3 7
x
xx
+
→
=
++
.
13. Do
1x
−
→
nên
11xx− = −
suy ra
( )
( )
3
2
11
1
1
lim lim
23
1 2 2 3
xx
x
x
xx
x x x
−−
→→
−
−
=
+−
− + +
2
1
11
lim
2 2 3 7
x
xx
−
→
−
=−
++
.
14. Ta có
( )( )
2
3 2 1 2x x x x− + = − −
, do
2x
+
→
nên
2
3 2 0xx− +
, suy ra
( )( )
( )
2
2 2 2
32
12
lim lim lim 1 1
22
x x x
xx
xx
x
xx
+ + +
→ → →
−+
−−
= = − =
−−
.
15. Ta có
( )( )
2
33
9
33
lim lim
33
xx
x
xx
xx
→→
−
+−
=
−−
TH1:
3x
ta có
( )( )
( )
2
3 3 3
9
33
lim lim lim 3 6
33
x x x
x
xx
x
xx
+ + +
→ → →
−
+−
= = + =
−−
.
TH2:
3x
ta có
( )( )
( )
2
3 3 3
9
33
lim lim lim 3 6
33
x x x
x
xx
x
xx
− − −
→ → →
−
− + −
= = − − = −
−−
.
Do
22
33
99
lim lim
33
xx
xx
xx
+−
→→
−−
−−
nên không tn tại
2
3
9
lim
3
x
x
x
→
−
−
.
16. Ta có
( )( )
2
44
44
lim lim
45
20
xx
xx
xx
xx
→→
−−
=
−+
+−
TH1:
4x
, ta có
( )( )
2
4 4 4
4 4 1 1
lim lim lim
4 5 5 9
20
x x x
xx
x x x
xx
+ + +
→ → →
−−
= = =
− + +
+−
TH2:
4x
, ta có
( )( )
2
4 4 4
4 4 1 1
lim lim lim
4 5 5 9
20
x x x
xx
x x x
xx
+ − −
→ → →
− − − −
= = =
− + +
+−
Do
2
4
4
lim
20
x
x
xx
+
→
−
+−
2
4
4
lim
20
x
x
xx
−
→
−
+−
nên không tn tại
2
4
4
lim
20
x
x
xx
→
−
+−
.
17. Do
2x
−
→
nên
22xx− = −
suy ra
2
2
lim
11
x
x
x
−
→
−
−−
( )
( )
2
2 1 1
lim
11
x
xx
x
−
→
− − +
=
−−
( )
2
lim 1 1 2
x
x
−
→
− + =
.
18. Do
3x
−
→
nên
23xx− = −
suy ra
3
3
lim
5 11 2
x
x
x
−
→
−
−−
( )
( )
3
3 5 11 2
lim
5 11 4
x
xx
x
−
→
− − +
=
−−
( )
3
5 11 2
4
lim
55
x
x
−
→
− − +
= = −
.
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
77
19. Do
2x
−
→
nên
22xx− = −
suy ra
3
2
2
lim
11
x
x
x
−
→
−
−−
( )
(
)
2
33
2
2 1 1 1
lim
11
x
x x x
x
−
→
− − + − +
=
−−
(
)
( )
2
33
2
lim 1 1 1 3
x
xx
−
→
= − − + − + = −
.
20. Ta có
( )( )
2
25 5 5x x x− = − +
, do
5x
−
→
nên
2
25 0x −
, suy ra
2
3
5
25
lim
41
x
x
x
−
→
−
−−
( )
(
)
2
2
33
5
25 4 4 1
lim
41
x
x x x
x
−
→
− − + − +
=
−−
( )
( )
(
)
2
33
5
lim 5 4 4 1 30
x
x x x
−
→
= − + − + − + = −
.
21.
( )
2
3
38
lim
3
x
x
x
+
→
−
= +
−
, vì
( )
( )
( )
3
2
3
2
lim 3 8 1
lim 3 0
3 0, 3
x
x
x
x
xx
+
+
→
→
+
−=
−=
− →
.
22. Ta có
3
2
2
25 3
lim
2
x
x
xx
→
+−
−−
( )( )
(
)
2
2
33
25 27
lim
2 1 25 3 25 9
x
x
x x x x
→
+−
=
− + + + + +
( )
(
)
2
2
33
11
lim
81
1 25 3 25 9
x
x x x
→
==
+ + + + +
.
23.
2
2
32
lim
4 16
x
x
x
+
→
+
= +
−
, vì
( )
2
2
2
2
lim 3 2 8
lim 4 16 0
4 16 0, 2
x
x
x
x
xx
+
+
→
→
+
+=
−=
− →
.
24.
0
lim
x
xx
xx
+
→
+
−
( )
( )
00
1
1
lim lim 1
1
1
xx
xx
x
x
xx
++
→→
+
+
= = = −
−
−
.
25.
2
2
4
lim
2
x
x
x
+
→
−
−
( )( )
( )
22
22
lim lim 2 2 0
2
xx
xx
xx
x
++
→→
−+
= = − + =
−
.
26.
0
2
lim
x
xx
xx
+
→
+
−
=
( )
( )
00
2
2
lim lim 2
1
1
xx
xx
x
x
xx
++
→→
+
+
= = −
−
−
.
27. Ta có
( )
( )( )
1
21
lim
11
x
xx
xx
+
→−
++
+ − −
( )
( )
( )
11
2 1 2
lim lim 1
11
1 1 1
xx
x x x
x
xx
++
→ − → −
+ + +
= = =
−+
+ − +
.
28. Ta có
( )
2
2
6 9 3 3x x x x− + = − = −
, do
3x
−
→
nên
2
6 9 3x x x− + = −
, suy ra
2
2
3
69
lim
9
x
xx
x
−
→
−+
−
( )( )
2
2
3 3 3
6 9 3 1 1
lim lim lim
9 3 3 3 6
x x x
x x x
x x x x
− − −
→ → →
− + − −
= = = = −
− − + +
.
29. Do
1x
−
→
nên
10x−
, từ đó ta có
2
2
1
43
lim
65
x
xx
xx
−
→
−+
− + −
( )( )
( )( )
1
13
lim
15
x
xx
xx
−
→
−−
=
− − −
( )( )
1
13
lim
15
x
xx
xx
−
→
−−
=
− − −
( )
1
3
lim
15
x
x
xx
−
→
−
=
−−
1
13
lim .
5
1
x
x
x
x
−
→

−
= = −


−
−

.
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
78
vì
1
32
lim
54
x
x
x
−
→
−
=−
−
và
1
1
lim
1
x
x
−
→

= +

−

.
30.
( )
2
54
1
32
lim
x
xx
xx
+
→−
++
+
( )
( )( )
2
1
12
lim
1
x
xx
xx
+
→−
++
=
+
( )
( )
2
1
12
lim 0
x
xx
x
+
→−
++
==
.
31.
( )
2
2
lim 2
4
x
x
x
x
+
→
−
−
( )
( )( )
( )
22
2
lim 2 lim 0
2 2 2
xx
xx
x
x
x x x
++
→→
−
= − = =
− + +
.
32. Ta có
( )
( )
3
2
1
lim 1
1
x
x
x
x
+
→−
+
−
( )
( )
( )
( )( )
2
1
lim 1 1
11
x
x
x x x
xx
+
→−
= + − +
−+
( )
( )
( )
2
1
1
lim 1 0
1
x
xx
xx
x
+
→−
+
= − + =
−
.
33. Do
1x
+
→
nên
10x−
, vì thế ta có
( )
2
1
5
lim 1
23
x
x
x
xx
+
→
+
−
+−
( )( )
( )( )
2
1
51
lim
13
x
xx
xx
+
→

+−

=

−+

( )( )
1
51
lim 0
3
x
xx
x
+
→

+−

==

+

.
34.
( )
11
11
lim lim
2 1 1
1 2 1
xx
x x x x
xx
xx
−−
→→
−−
=
− + −
− + −
1
1
lim
2
21
x
x
x
−
→
==
+−
.
35.
0
1
lim 2
x
x
x
x
+
→

−



( )
2
0
1
lim 2
x
xx
x
+
→

−

=


( )
0
lim 2 1 0
x
xx
+
→
= − =
.
36.
( )
( )
2
2
3
2 5 3
lim
3
x
xx
x
+
→−
+−
+
( )
( )( )
( )
( )
2
33
2 1 3
21
lim lim
3
3
xx
xx
x
x
x
++
→ − → −
−+
−
= = = −
+
+
.
37.
2
2
11
lim
24
x
xx
−
→

−

−−

( )( )
2
21
lim
22
x
x
xx
−
→

+−
=


−+

2
11
lim .
22
x
x
xx
−
→
+

= = −

+−

.
38. Do
1x
−
→
nên
10x−
, suy ra
( )
2
1 1 1x x x− = − = −
nên ta có
3
2
1
32
lim
54
x
xx
xx
−
→
−+
−+
( )( )
( )( )
2
1
21
lim
14
x
xx
xx
−
→
+−
=
−+
( )
( )( )
1
12
lim
14
x
xx
xx
−
→
−+
=
−+
1
23
lim
45
x
x
x
−
→
−+
= = −
+
.
Bài 8. Tính các giới hạn sau:
1)
0
sin5
lim
x
x
x
→
. ĐS:
5
2)
0
tan2
lim
3
x
x
x
→
. ĐS:
2
3
3)
2
0
1 cos
lim
x
x
x
→
−
. ĐS:
1
2
4)
3
0
sin5 .sin3 .sin
lim
45
x
x x x
x
→
. ĐS:
1
3
5)
0
1 cos5
lim
1 cos3
x
x
x
→
−
−
6)
2
0
1 cos 2
lim
.sin
x
x
xx
→
−
. ĐS:
4
7)
( )
0
sin
lim 0
1 cos
x
x ax
a
ax
→
−
. ĐS:
2
a
8)
0
1 cos
lim
1 cos
x
ax
bx
→
−
−
. ĐS:
2
2
a
b
9)
( )
2
0
1 cos
lim ; 0
x
x
a
x
→
−
ĐS:
2
2
a
10)
3
0
sin tan
lim
x
xx
x
→
−
ĐS:
1
2
−
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
79
11)
3
0
tan sin
lim .
sin
x
xx
x
→
−
ĐS:
1
2
12)
sin sin
lim .
xa
xa
xa
→
−
−
ĐS:
cosa
13)
cos cos
lim
xb
xb
xb
→
−
−
ĐS:
sinb−
14)
0
1 2 1
lim
sin 2
x
x
x
→
−+
ĐS:
1
2
−
15)
( ) ( )
0
cos cos
lim
x
a x a x
x
→
+ − −
ĐS:
2sina−
16)
tan tan
lim
xc
xc
xc
→
−
−
ĐS:
2
1
cos c
17)
3
0
1 cos
lim
sin
x
x
xx
→
−
ĐS:
3
2
18)
22
22
sin sin
lim
xa
xa
xa
→
−
−
ĐS:
sin2
2
a
a
19)
2
0
cos cos
lim
x
xx
x

→
−
ĐS:
22
2

−
20)
( )
3
2
8
lim
tan 2
x
x
x
→−
+
+
ĐS:12
21)
0
1 cos cos2 cos3
lim .
1 cos
x
x x x
x
→
−
−
ĐS:1422)
( ) ( )
2
0
sin 2 2sin sin
lim .
x
a x a x a
x
→
+ − + +
ĐS:
( )
sin
−
23)
0
sin tan
lim ;( 0)
()
x
ax bx
ab
a b x
→
+
+
+
ĐS: 1 24)
2
0
cos3 cos5 cos7
lim
x
x x x
x
→
−
ĐS:
33
2
−
25)
0
cos cos cos
lim .
1 cos
x
ax bx cx
x
→
−
−
ĐS:
2 2 2
2
bac−−
26)
( ) ( )
0
sin sin
lim
tan( ) tan( )
x
a x a x
a x a x
→
+ − −
+ − −
ĐS:
3
cos a
27)
3
2
0
2 1 1
lim .
sin
x
xx
x
→
+ − +
ĐS: 1 28)
2
4
0
sin 2 sin sin4
lim
x
x x x
x
→
−
ĐS: 6
29)
2
cos
lim .
2
x
x
x
→−
+
ĐS: 1 30)
0
2
sin sin2
lim
1 sin
2
x
xx
x
x
→
−

−


ĐS: -1
31)
2
2
0
1 cos
lim .
x
xx
x
→
+−
ĐS: 1 32)
3
0
1 tan 1 sin
lim
x
xx
x
→
+ − +
ĐS:
1
4
33)
2
0
1 cos5 cos7
lim .
sin 11
x
xx
x
→
−
ĐS:
37
121
34)
1
32
lim
tan( 1)
x
x
x
→
+−
−
ĐS:
1
4
35)
( )
2
1 cos
lim .
x
x
x
→
+
−
ĐS:
1
2
36)
2
1
sin( 1)
lim
43
x
x
xx
→
−
−+
ĐS:
1
2
−
37)
2
2
0
1 cos2
lim .
x
xx
x
→
+−
ĐS:
5
2
38)
2
0
1 cos cos2
lim
x
xx
x
→
−
ĐS:
3
2
Li giải.
1)
00
sin5 sin5
lim lim 5 5
5
xx
xx
xx
→→

= =


.
2)
00
tan2 2 tan2 2
lim lim .
3 3 2 3
xx
xx
xx
→→

= =


Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
80
3)
2
2
22
0 0 0
2sin
sin
1 cos 1 1
2
2
lim lim lim 2
42
2
x x x
x
x
x
x
xx
→ → →








−



= = =









.
4)
3
00
sin5 sin3 sin 1 sin5 sin3 sin 1
lim lim
45 3 5 3 3
xx
x x x x x x
x x x x
→→

= =


.
5)
22
2
0 0 0
2
5 5 3
2sin sin
1 cos5 25 4 25
2 2 2
lim lim lim
3 5 3
1 cos3 4 9 9
2sin sin
2 2 2
x x x
x x x
x
x x x
x
→ → →


−

= = =
−



.
6)
2 2 2
2
0 0 0 0
1 cos 2 sin 2 4sin cos sin
lim lim lim lim 4cos 4
sin sin
x x x x
x x x x x
x
x x x x x x
→ → → →
−

= = = =


.
7)
2
2
0 0 0
2
sin sin 1 sin 4 2
2
lim lim lim
1 cos 2
2sin sin
22
x x x
ax
x ax x ax ax
a
ax ax
ax ax a a
→ → →





= = =

−





.
8)
22
2
22
22
0 0 0
2
2sin sin
1 cos 4
2 2 2
lim lim lim
1 cos 4
2sin sin
2 2 2
x x x
ax ax bx
ax a a
bx ax bx
bx b b
→ → →


−

= = =
−



.
9)
2
2
22
22
0 0 0
2sin sin
1 cos
22
lim lim lim 2
42
2
x x x
ax ax
ax a a
ax
xx
→ → →




−

= = =






.
10) Ta có
( )
33
00
sin cos 1
sin tan
lim lim
cos
xx
xx
xx
x x x
→→
−
−
=
2
2
3
00
2sin sin sin
2 sin 1 1
22
lim lim .
cos cos 4 2
2
xx
xx
x
x
x
x x x x
→→


−



= = − = −






11)
( )
( )
( )
3
2
0 0 0
sin 1 cos
tan sin 1 1
lim lim lim .
sin cos 1 cos 2
cos sin 1 cos
x x x
xx
xx
x x x
x x x
→ → →

−
−
= = =


+
−

12) Ta có
2cos sin
sin sin
22
lim lim
xa
xa
x a x a
xa
x a x a
→
→
+−
−
=
−−
sin
2
lim cos cos .
2
2
xa
xa
xa
a
xa
→
−


+
= =

−


Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
81
13) Ta có
2sin sin
cos cos
22
lim lim
x b x b
x b x b
xb
x b x b
→→
+−
−
−
=
−−
sin
2
lim sin sin .
2
2
xb
xb
xb
b
xb
→
−


+
= − = −

−


14) Ta có
( )
00
1 2 1 1 2 1
lim lim
sin 2
sin 2 1 2 1
xx
xx
x
xx
→→
− + − −
=
++
0
1 2 1
lim .
sin 2 2
1 2 1
x
x
x
x
→

= − = −

++

15) Ta có
00
2sin sin
cos( ) cos( )
22
lim lim
xx
a x a x a x a x
a x a x
xx
→→
+ + − + − +
−
+ − −
=
0
sin
lim 2sin 2sin .
x
x
aa
x
→

= − = −


16)
( )
( )
( )
2
sin sin
tan tan 1 1
lim lim lim
cos cos cos cos cos .
x c x c x c
x c x c
xc
x c x c x c x c x c c
→ → →
−−

−
= = =

− − −

17) Ta có
( )
( )
2
3
00
1 cos 1 cos cos
1 cos
lim lim
sin sin
xx
x x x
x
x x x x
→→
− + +
−
=
( )
22
2
00
2sin 1 cos cos sin
1 cos cos 1 3
22
lim lim .
22
2 sin cos cos
2 2 2 2
xx
xx
xx
xx
x x x x
x
→→

++

++
= = =



18) Ta có
( )( )
22
22
1 cos2 1 cos2
sin sin
22
lim lim
x a x a
xa
xa
x a x a x a
→→
−−
−
−
=
− − +
( ) ( )
2sin sin
cos2 cos2
lim lim
2( )( ) 2( )( )
x a x a
a x a x
ax
x a x a x a x a
→→
− + −
−
==
− + − +
sin( ) sin( ) sin 2
lim .
2
xa
a x a x a
x a a x a
→
+−

= =

+−

19) Ta có
( ) ( )
22
00
2sin sin
cos cos
22
lim lim
xx
xx
xx
xx

→→
+−
−
−
=
( )
( )
( )
( )
22
0
sin sin
22
lim 2 .
2 2 2
22
x
xx
xx
→
+−


+ − −
= − =

+−



20) Ta có
( )
( )
( )
( )
( )
2
3
2
2 2 2
2 2 4
2
8
lim lim lim 2 4 12.
tan 2 tan( 2) tan( 2)
x x x
x x x
x
x
xx
x x x
→− →− →−
+ − +
+

+
= = − + =

+ + +

21) Ta có
00
1 cos cos2 cos3 1 cos cos (1 cos2 ) cos cos2 (1 cos3 )
lim lim
1 cos 1 cos
xx
x x x x x x x x x
xx
→→
− − + − + −
=
−−
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
82
2
2
0
22
3
2sin
2sin
2
lim 1 cos cos cos2
2sin 2sin
22
x
x
x
x x x
xx
→


= + +



2 2 2
2
0
3
sin
sin
2 2 2
lim 1 4cos 9cos cos2 1 4 9 14.
3
sin sin sin
2 2 2
x
x x x
x
x x x
x x x
x
→




= + + = + + =





22) Ta có
( ) ( ) ( )
22
00
sin 2 2sin( ) sin 2sin cos 2sin
lim lim
xx
a x a x a a x x a x
xx
→→
+ − + + + − +
=
( )
2
22
00
4sin sin
2sin( )(cos 1)
2
lim lim
xx
x
ax
a x x
xx
→→
−+
+−
==
( )
2
0
sin
1
2
lim 4sin sin .
4
2
x
x
a x a
x
→





= − + = −






23) Ta có
0 0 0
sin tan sin tan
sin tan
lim lim lim 1.
( ) ( )
x x x
ax bx ax bx
ax bx a b
ax bx a b
ax bx ax bx
a b x a b x a b a b
→ → →
+
++
= = = =
+ + + +
24) Ta có
22
00
cos3 cos5 cos7 cos3 cos5 cos5 cos5 cos7
lim lim
xx
x x x x x x x x
xx
→→
− − + −
=
2
22
00
7
2sin4 sin 2cos5 sin
2sin4 sin cos5 (1 cos7 )
2
lim lim
xx
x
x x x
x x x x
xx
→→
−
+−
==
2
0
7
sin
sin 4 sin 49 49 33
2
lim 8 2 cos5 8 .
7
4 4 2 2
2
x
x
xx
x
x
xx
→





= − = − = −






25) Ta có
22
00
cos cos cos cos cos cos cos cos
lim lim
xx
ax bx cx ax bx bx bx cx
xx
→→
− − + −
=
( ) ( )
2
22
00
( ) ( )
2sin sin cos (1 cos ) 2sin sin 2cos sin
2 2 2 2 2
lim lim
xx
b a x b a x
a b x a b x cx
bx cx bx
xx
→→
−−
++
+ − −
==
( )
( )
2
2 2 2 2 2 2 2 2 2
0
()
sin sin sin
2 2 2
lim 2 2 cos .
()
4 4 2 2 2
22
2
x
b a x
a b x cx
b a c b a c b a c
bx
a b x cx
b a x
→

−
+



− − − −

= − = − =

+
−





Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
83
26) Ta có
( ) ( )
( ) ( )
00
sin( ) sin( ) 2cos sin
lim lim
sin 2
tan tan
cos cos
xx
a x a x a x
x
a x a x
a x a x
→→
+ − −
=
+ − −
+−
( ) ( )
3
0
cos cos cos
lim cos
cos
x
a a x a x
a
x
→
+−
==
27) Ta có
33
22
00
2 1 1 2 1 1 1 1
lim lim
cos sin
xx
x x x x
xx
→→
+ − + + − + − +
=
(
)
2
2
33
22
0
2
2 1 1
1 1 1
lim
sin
x
xx
x
xx
x
→
−
+
++
+ + + +
=
(
)
2
33
22
0
2
2 1 1
1 1 1
lim 1.
sin
x
xx
x
xx
x
x
→
−
++
+ + + +
==
28) Ta có
( )
2
44
00
sin2 sin2 2sin cos2
sin 2 sin sin4
lim lim
xx
x x x x
x x x
xx
→→
−
−
=
( )
44
00
3
4sin2 sin sin sin
2sin2 sin cos cos2
22
lim lim
xx
xx
xx
x x x x
xx
→→
−
==
0
3
sin sin
3 sin 2 sin
22
lim 4 6.
3
22
22
x
xx
xx
xx
xx
→


= =



29)
22
sin
cos
2
lim lim 1
22
xx
x
x
xx


→− →−

+


==
++
.
30)
( )
0 0 0
2
sin 1 2cos
sin sin2 sin 1 2cos
lim lim lim 1
cos cos
1 2sin
2
x x x
xx
x x x x
x
x x x x
x
→ → →
−
−−

= = = −



−


.
31) Ta có
22
22
00
1 cos 1 1 1 cos
lim lim
xx
x x x x
xx
→→
+ − + − + −
=
2
22
0
1 1 1 cos
lim
x
xx
xx
→

+ − −
=+



(
)
2
2
2
0
22
2sin
11
2
lim
11
x
x
x
x
xx
→


+−
=+

++


2
2
0
sin
1 1 1 1
2
lim 1
22
1
2
2
1
x
x
x
x
→





= + = + =


++




.
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
84
32)
( )
3
3
00
1 tan 1 sin 1 tan 1 sin
lim lim
1 tan 1 sin
xx
x x x x
x
x x x
→→
+ − + + − −
=
+ + +
( )
( )
3
0
sin 1 cos
lim
cos 1 tan 1 sin
x
xx
x x x x
→
−
=
+ + +
( )
2
3
0
2sin sin
2
lim
cos 1 tan 1 sin
x
x
x
x x x x
→
=
+ + +
( )
2
0
sin
2 sin 1 1
2
lim
44
cos 1 tan 1 sin
2
x
x
x
x
x
x x x
→





= =


+ + +




.
33)
( )
22
00
1 cos5 cos5 1 cos7
1 cos5 cos7
lim lim
sin 11 sin 11
xx
x x x
xx
xx
→→
− + −
−
=
22
22
0
57
2sin 2cos5 sin
22
lim
sin 11 sin 11
x
xx
x
xx
→


=+



22
22
0
57
sin sin
25 11 49 11
22
lim cos5
57
242 sin11 242 sin11
22
x
xx
xx
x
xx
xx
→



= +



25 49 37
242 242 121
= + =
.
34)
( )
( )
( )
11
3 2 3 4
lim lim
tan 1
tan 1 3 2
xx
xx
x
xx
→→
+ − + −
=
−
− + +
( )
1
1 1 1
lim
tan 1 4
32
x
x
x
x
→

−
= =


−
++

.
35)
( ) ( )
2
22
2cos
1 cos
2
lim lim
xx
x
x
xx


→→
+
=
−−
( )
2
2
2
2sin sin
11
22
lim lim
22
2
xx
xx
x
x


→→

− −




= = =

−

−





.
36)
( ) ( )
( )( )
( )
2
1 1 1
sin 1 sin 1 sin 1
11
lim lim lim .
4 3 1 3 1 3 2
x x x
x x x
x x x x x x
→ → →
− − −

= = = −

− + − − − −

.
37)
22
22
00
1 cos2 1 1 1 cos2
lim lim
xx
x x x x
xx
→→
+ − + − + −
=
(
)
2 2 2
2 2 2
00
22
1 1 1 cos2 1 1 2sin
lim lim
11
xx
x x x x
x x x
xx
→→


+ − − + −

= + = +



++



2
2
0
1 sin 1 5
lim 2 2
22
11
x
x
x
x
→


= + = + =




++

.
38)
( )
22
00
1 cos cos 1 cos2
1 cos cos2
lim lim
xx
x x x
xx
xx
→→
− + −
−
=
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
85
( )
( )
( )
2
2 2 2
2
00
2sin
cos 1 cos2
cos 1 cos2
1 cos
2
lim lim
1 cos2
xx
x
xx
xx
x
x x x
xx
→→


−

−
−

= + = +


+



( )
2
2
2
00
sin sin
1 1 sin 1 3
22
lim lim 1
2 2 2 2
1 cos2
1 cos2
xx
xx
x
x
x
xx
→→




= = = + =

+


+


Bài 9. Tính các giới hạn sau:
1)
0
cos3 cos
lim
cos5 cos
x
xx
xx
→
−
−
ĐS:
1
3
2)
2
6
1 2sin
lim
4cos 3
x
x
x
→
−
−
ĐS:
1
2
3)
2
1 sin 2 cos2
lim
cos
x
xx
x
→
++
ĐS: 24)
0
sin7 sin5
lim
sin
x
xx
x
→
−
ĐS: 2
5)
4
2 2cos
lim
sin
4
x
x
x
→
−

−


ĐS:
2
6)
3
0
1 cos
lim
sin
x
x
x
→
−
ĐS:
1
6
7)
3
3
sin cos
lim
sin3
x
xx
x
→
−
ĐS:
2
3
−
8)
4
lim tan2 .tan
4
x
xx
→


−




ĐS: 1
9)
3
cos3 2cos2 2
lim
sin3
x
xx
x
→
++
ĐS:
23
3
10)
3
2
3
tan 1
lim
2sin 1
x
x
x
→
−
−
ĐS:
1
12
−
Li giải
1)
0 0 0 0
sin
cos3 cos 2sin2 sin sin 1
lim lim lim lim
sin3
cos5 cos 2sin3 sin 2 sin3 3
3
3
x x x x
x
x x x x x
x
x
x x x x x
x
→ → → →
−−
= = = =
−−
.
2)
22
6 6 6
1 2sin 1 2sin 1 1
lim lim lim
4cos 3 1 4sin 1 2sin 2
x x x
xx
x x x
→ → →
−−
= = =
− − +
.
3)
( )
2
2 2 2
1 sin2 cos2 2cos sin2
lim lim lim 2cos 2sin 2
cos cos
x x x
x x x x
xx
xx
→ → →
+ + +
= = + =
.
4)
0 0 0
sin7 sin5 2cos6 sin
lim lim lim2cos6 2
sin sin
x x x
x x x x
x
xx
→ → →
−
= = =
.
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
86
5)
4 4 4
2
2 cos
2 cos cos
2
2 2cos
4
lim lim lim
sin sin sin
4 4 4
x x x
x
x
x
x x x
→ → →


−

−

−
==
− − −
4
4sin sin
8 2 8 2
lim
2sin cos
2 8 2 8
x
xx
xx


→
− + −
=
−−
4
2sin
82
lim 2
cos
28
x
x
x
→

+


==

−


6)
( )
2
3
2
00
3
3
22
1 cos cos
1 cos
lim lim
tan
sin 1 cos cos
xx
xx
x
x
x x x
→→
−
−
=

+ +


( )
2
0
3
3
2
cos 1
lim
6
1 cos 1 cos cos
x
x
x x x
→
==

+ + +


7)
( )
sin 3
3 3 3
3 3 3
2sin 3
3
sin 3
2sin
3
sin 3cos 2
3
lim lim lim lim
sin3 sin 3 3
sin 3
3
3
3
3
x x x
xx
x
x
x
xx
xx
x
x



→ → →
→ − − −






−







−
−



−

= = = = −
−+


−




−

−


.
8)
( )
2
2
4 4 4
2tan 1 tan 2tan
lim tan2 tan lim lim 1
4 1 tan 1 tan
1 tan
x x x
x x x
xx
xx
x
→ → →
−
− = = =



−+
+

.
9)
32
3
33
cos3 2cos2 2 4cos 3cos 4cos
lim lim
sin3 3sin 4sin
xx
x x x x x
x x x

→→
+ + − +
=
−
( )( )
( )( )
3
2cos 1 2cos 3 cos
lim
2sin 3 2sin 3 sin
x
x x x
x x x
→
−+
=
+−
( )
3
cos cos 2cos 3 cos
3
lim
3
x
x x x
→

−+


=
( )
( )
3
sin 2cos 3 cos
23
26
lim
3
cos 2sin 3 sin
26
x
x
xx
x
xx
→

− + +


==

++


10)
( )
( )
( )
2
3
2
2
2
33
44
tan 1 cos
tan 1
lim lim
2sin 1
1 tan . tan tan 1
xx
xx
x
x
x x x

→→
−
−
=
−

− + +


( )
( )
2
2
33
4
cos
lim
1 tan tan tan 1
x
x
x x x
→
−
=

+ + +


1
12
=−
.
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Page
87
Bài 10. Tính các giới hạn sau:
1)
0
cos4 1
lim
sin4
x
x
x
→
−
ĐS: 0 2)
0
1 sin2 cos2
lim
1 sin2 cos2
x
xx
xx
→
+−
−−
ĐS:
1−
3)
0
sin2
lim
1 sin2 cos2
x
x
xx
→
−−
ĐS:
1−
4)
0
1 cos
lim
sin
x
x
x
→
−
ĐS: 0
5)
0
sin5 sin3
lim
sin
x
xx
x
→
−
ĐS: 2 6)
0
11
lim
sin tan
x
xx
→

−


ĐS: 0
7)
2
4
2sin 1
lim
2cos 1
x
x
x
→
−
−
ĐS:
2
4
−
8)
6
sin
6
lim
1 2sin
x
x
x
→

−


−
ĐS:
23
3
9)
4
sin
4
lim
1 2sin
x
x
x
→

−


−
ĐS:
2
10)
0
2
lim cot
sin 2
x
x
x
→

−


ĐS: 0
| 1/87
| | Tải xuống

Có thể bạn quan tâm:

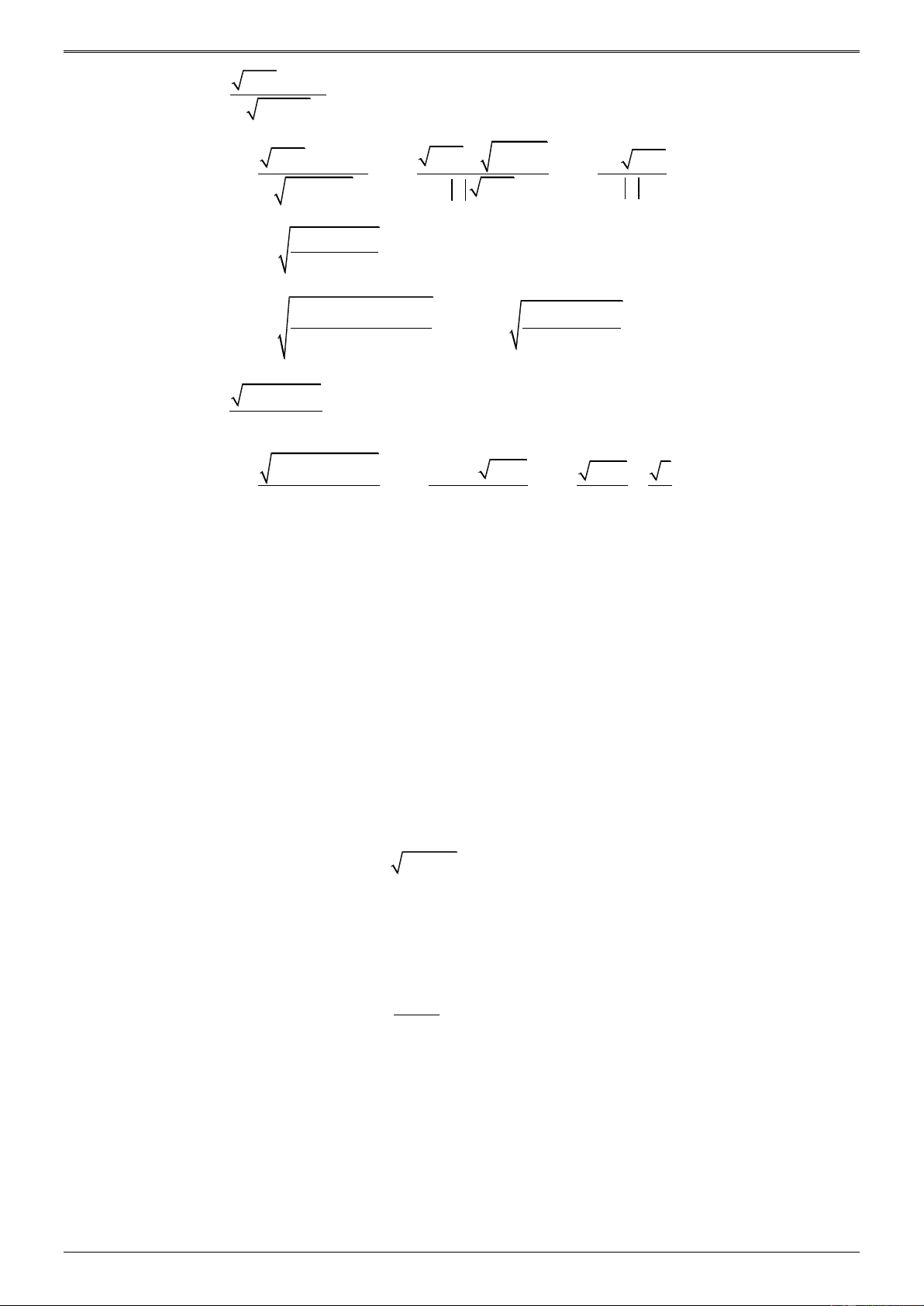
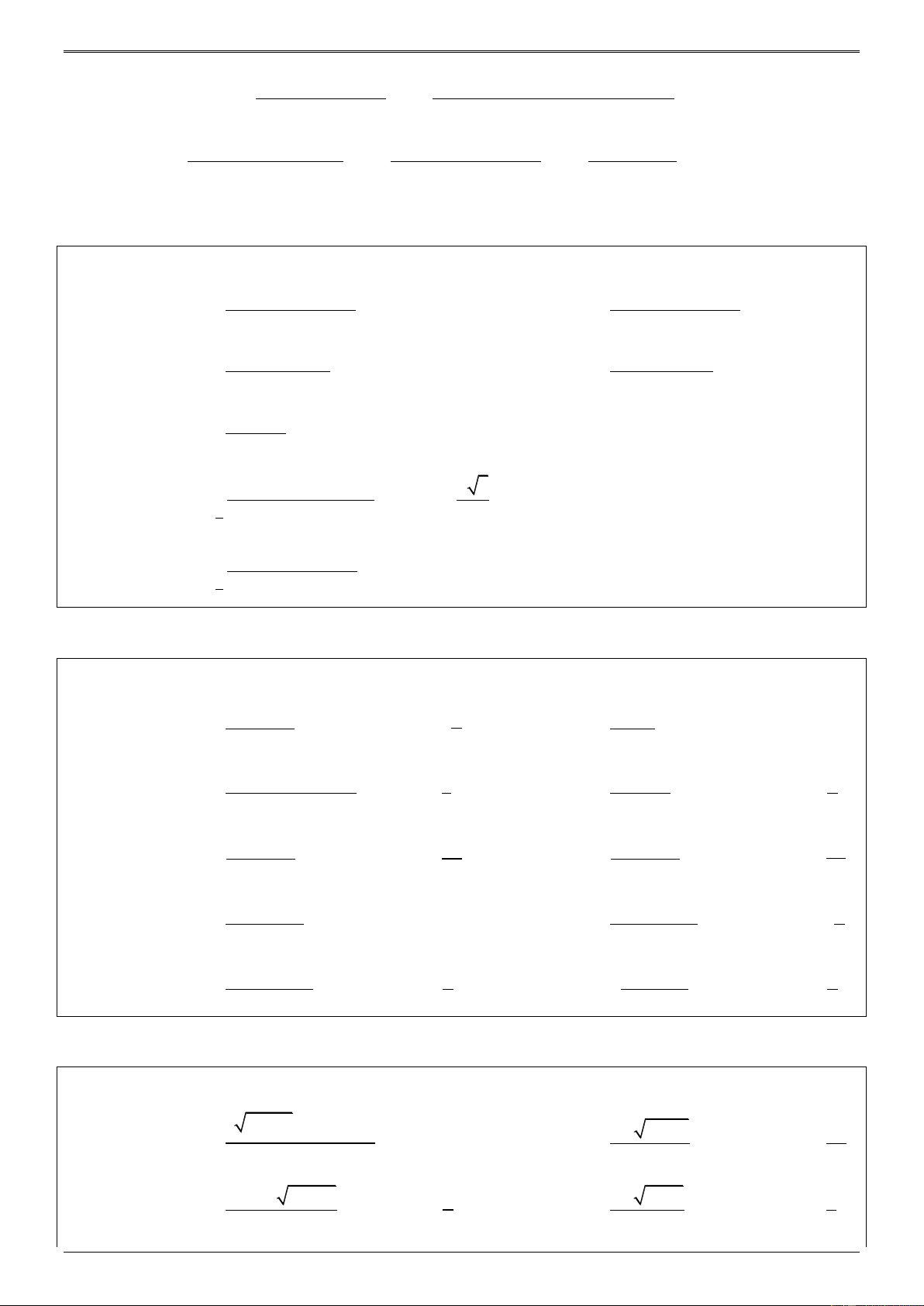
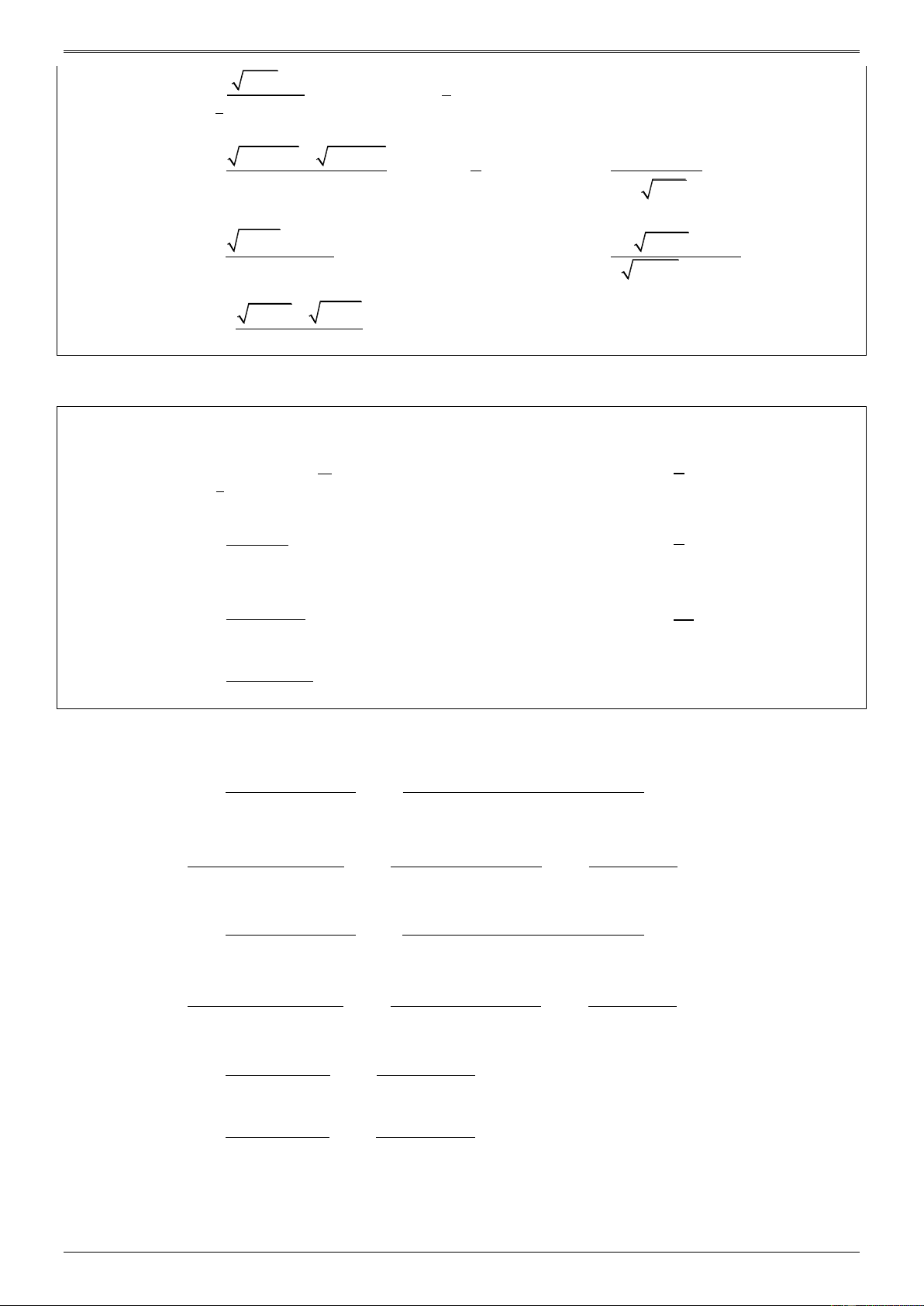
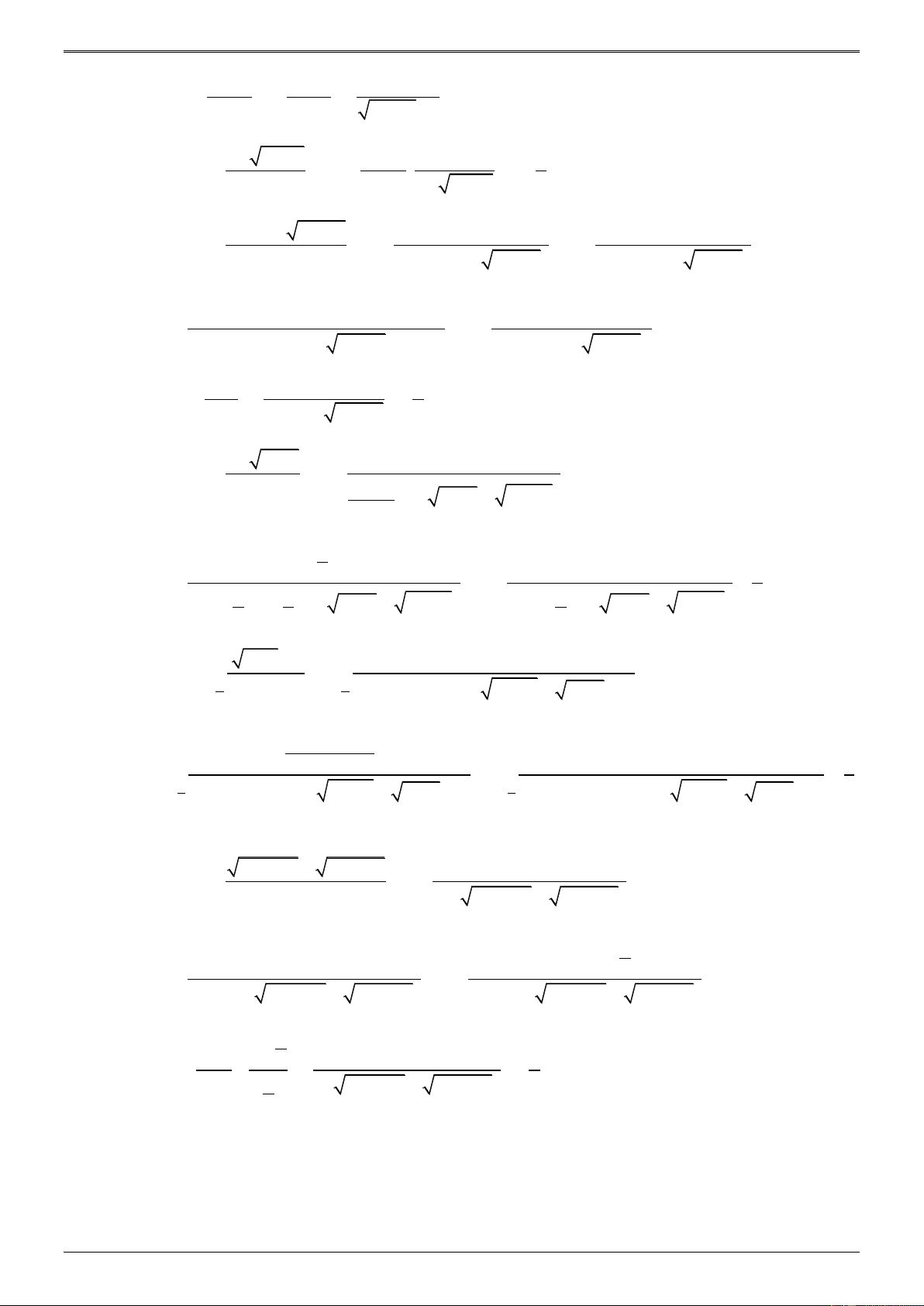
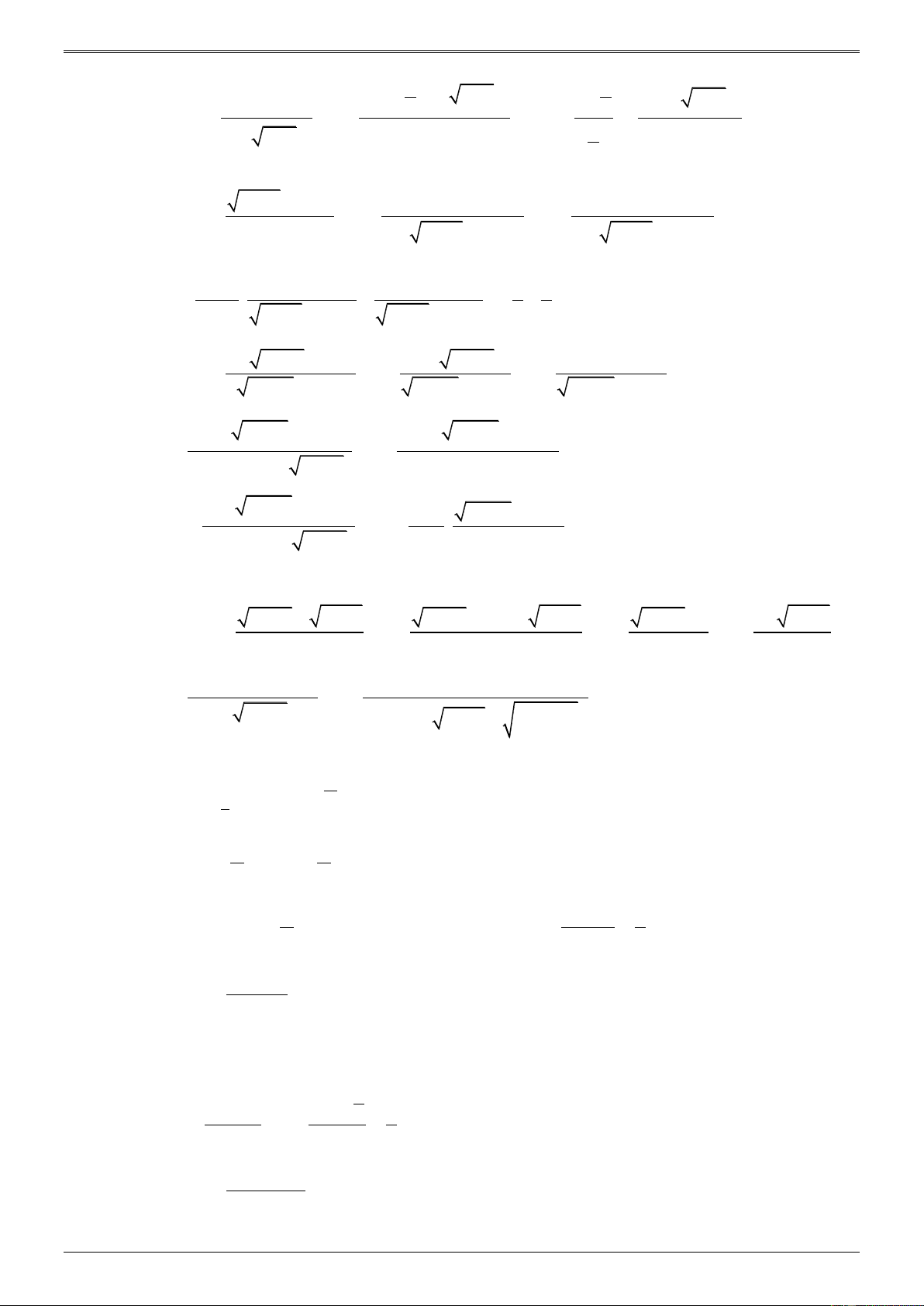
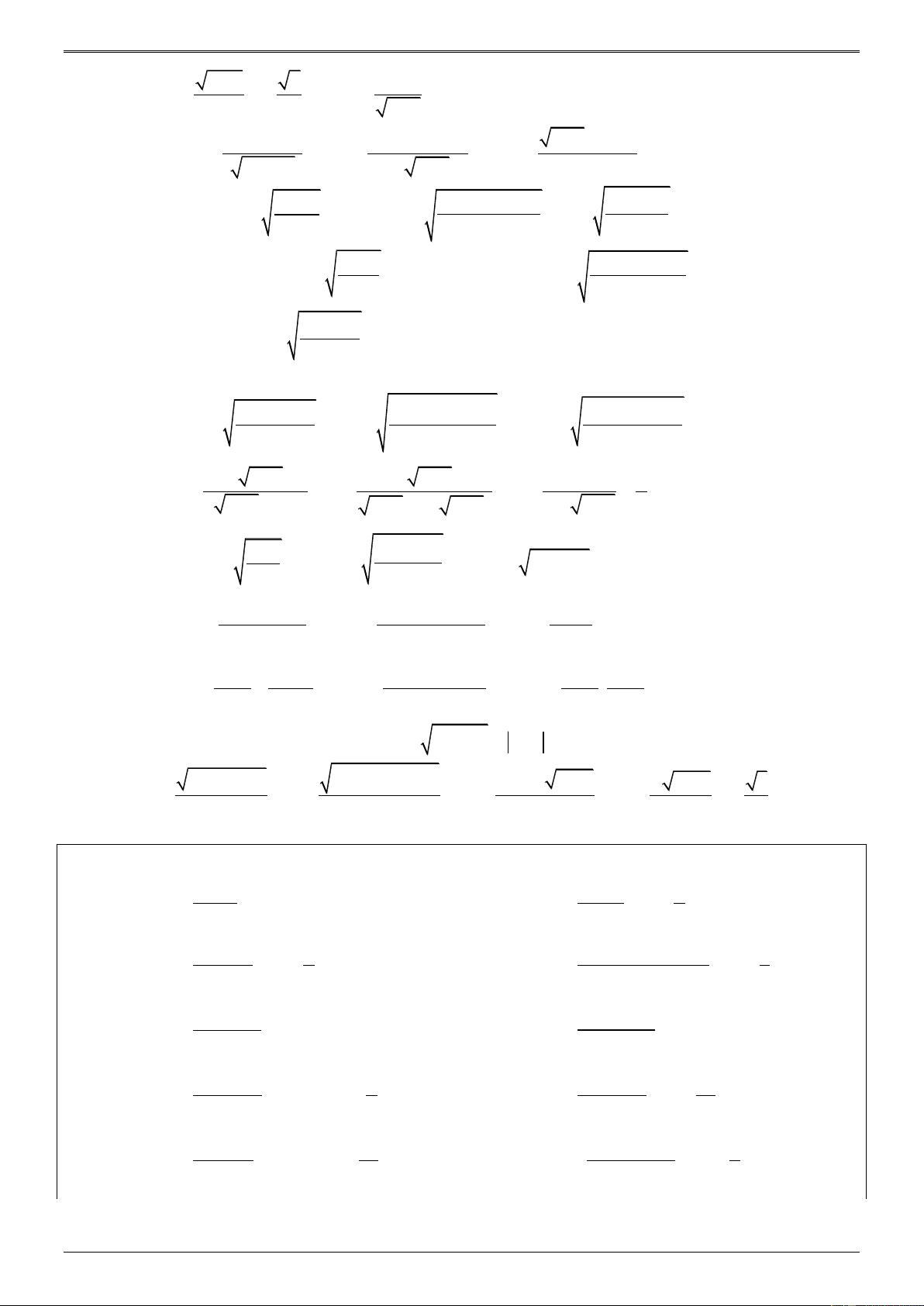
Preview text:

Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
CHƯƠNG 4 GIỚI HẠN
BÀI 2. GIỚI HẠN CỦA HÀM SỐ
A. TÓM TẮT LÝ THUYẾT
Định nghĩa 1 (Giới hạn của hàm số tại một điểm).
Giả sử (a;b) là một khoảng chứa điểm x và f là một hàm số xác định trên tập hợp 0
(a;b) \x . Ta nói rằng hàm số f có giới hạn là số thực L khi x dần đến x (hoặc tại điểm x ) nếu 0  0 0
với mọi dãy số ( x trong tập hợp (a;b) \ x mà lim x = x ta đều có lim f ( x = L . n ) 0  n ) n 0
Khi đó ta viết lim f (x) = L hoặc f ( x) → L khi x → x . 0 x→x0
Định nghĩa 2 (Giới hạn của hàm số tại vô cực).
Giả sử hàm số f xác định trên khoảng (a; +) . Ta nói rằng hàm số f có giới hạn là số thực
L khi x dần tới + nếu với mọi dãy số ( x trong khoảng (a;+) mà lim x = + ta đều có n ) n
lim f ( x = L . n )
Khi đó ta viết lim f (x) = L hoặc f ( x) → L khi x → + . x→+
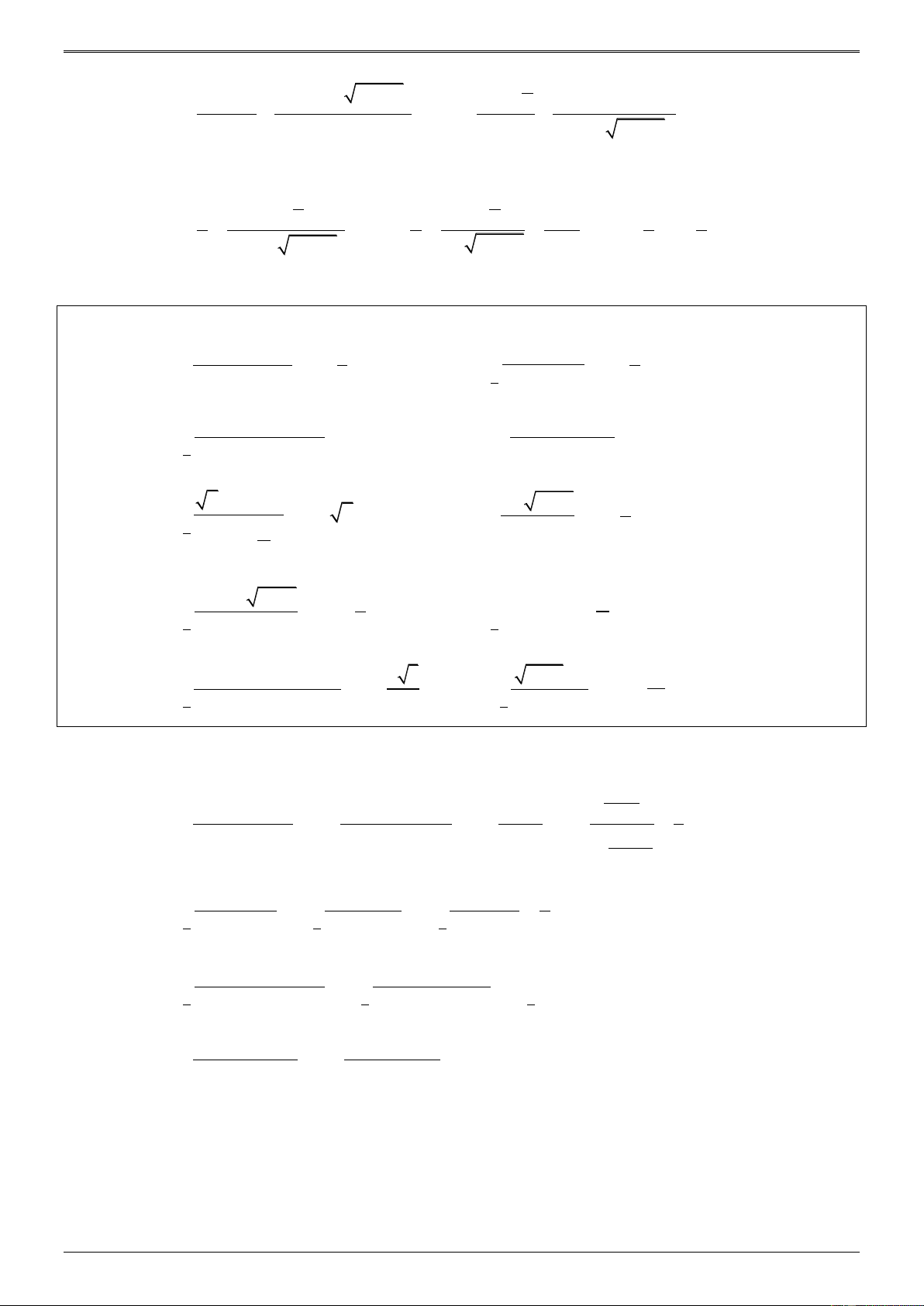
GIỚI HẠN HỮA HẠN GIỚI HẠN VÔ CỰC
Giới hạn đặc biệt
Giới hạn đặc biệt 1) lim x = x . c 0 = + = x→ 1) lim k x . 2) lim 0 . 0 x x→+ x k → x
2) lim c = c (c  ) . x→ 1 1 0 x 3) lim = − . 4) lim = + . − + x 0 → x x 0 → x
+ khi k 2 k k ( 0) 5) lim x =  x→−
− khi k  2 Định lí Định lí 1
Nếu lim f ( x) = L và lim g ( x) = M thì
Nếu lim f ( x) = L  0 và lim f ( x) =  thì x→x x→x x→x x→x 0 0 0 0 1) lim  f
 ( x)  g ( x) = L M  . + khi .
L lim g ( x)  0 x→  x→x 0 x lim  f
 ( x).g ( x) 0  =   . → 2) lim  f x x0 − khi . L lim g  (x)
 ( x).g ( x) = . L M  .  0 →  x→ x 0 x 0 x f ( x) L
Nếu lim g ( x) = 0 thì 3) lim = với M  0 . x→x0 x→ 0 x g ( x) M f ( x) + khi . L g  (x)  0
Nếu f ( x)  0 và lim f ( x) = L thì lim =  .
x→x0 g ( x) − khi . L g  (x)  x→x 0 0
lim f ( x) = L và lim
f ( x) = L . x→ → 0 x x 0 x 1 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Giới hạn một bên i
l m f ( x) = L  lim f ( x) = lim f ( x) = L . x + − → → → 0 x x 0 x x 0 x
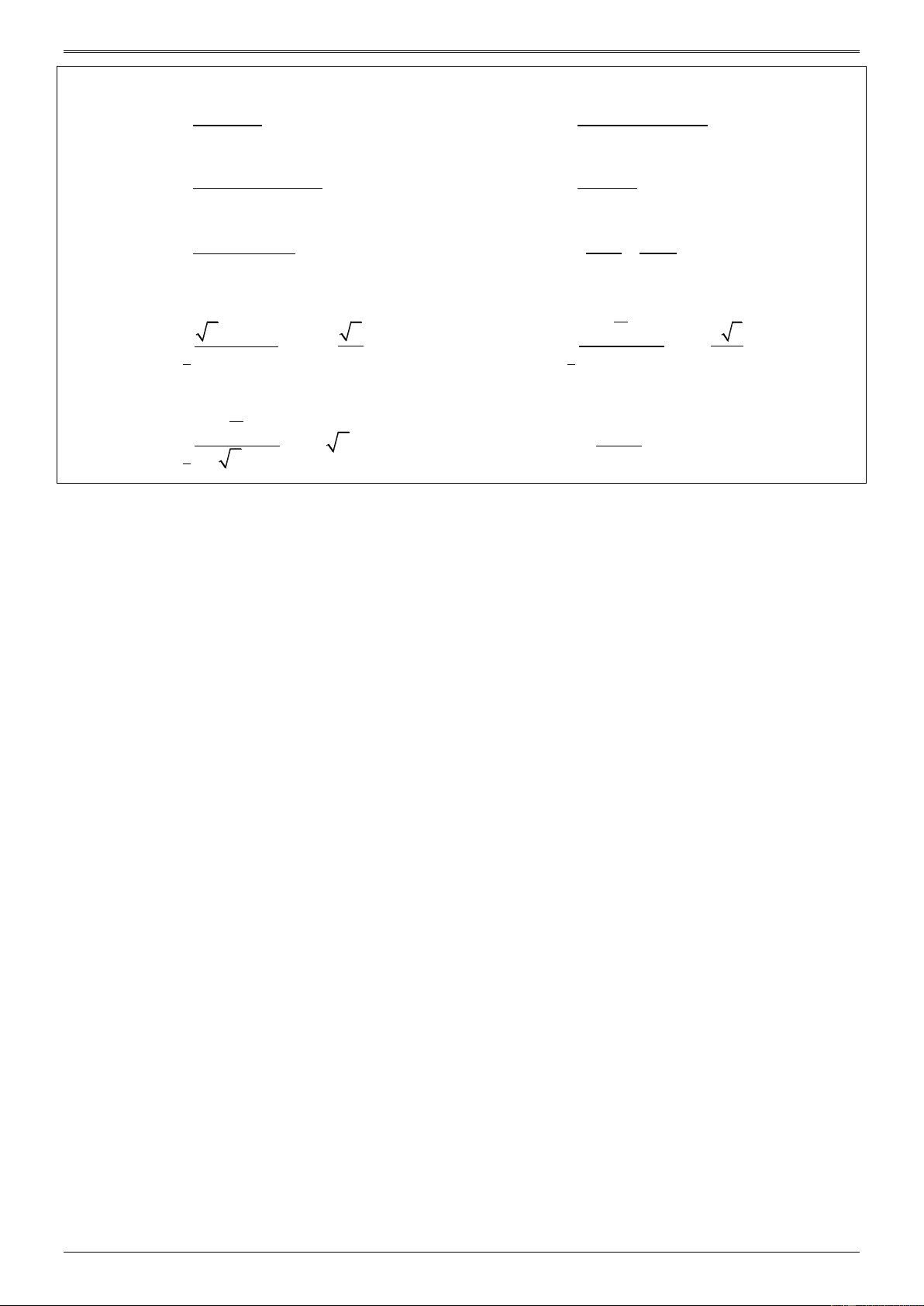
B. DẠNG TOÁN VÀ BÀI TẬP 0
Dạng 1. Tính giới hạn vô định dạng
, trong đó tử thức và mẫu thức là các đa thức. 0
Phương pháp giải:
Khử dạng vô định bằng cách phân tích thành tích bằng cách chia Hooc – nơ (đầu rơi, nhân tới, cộng
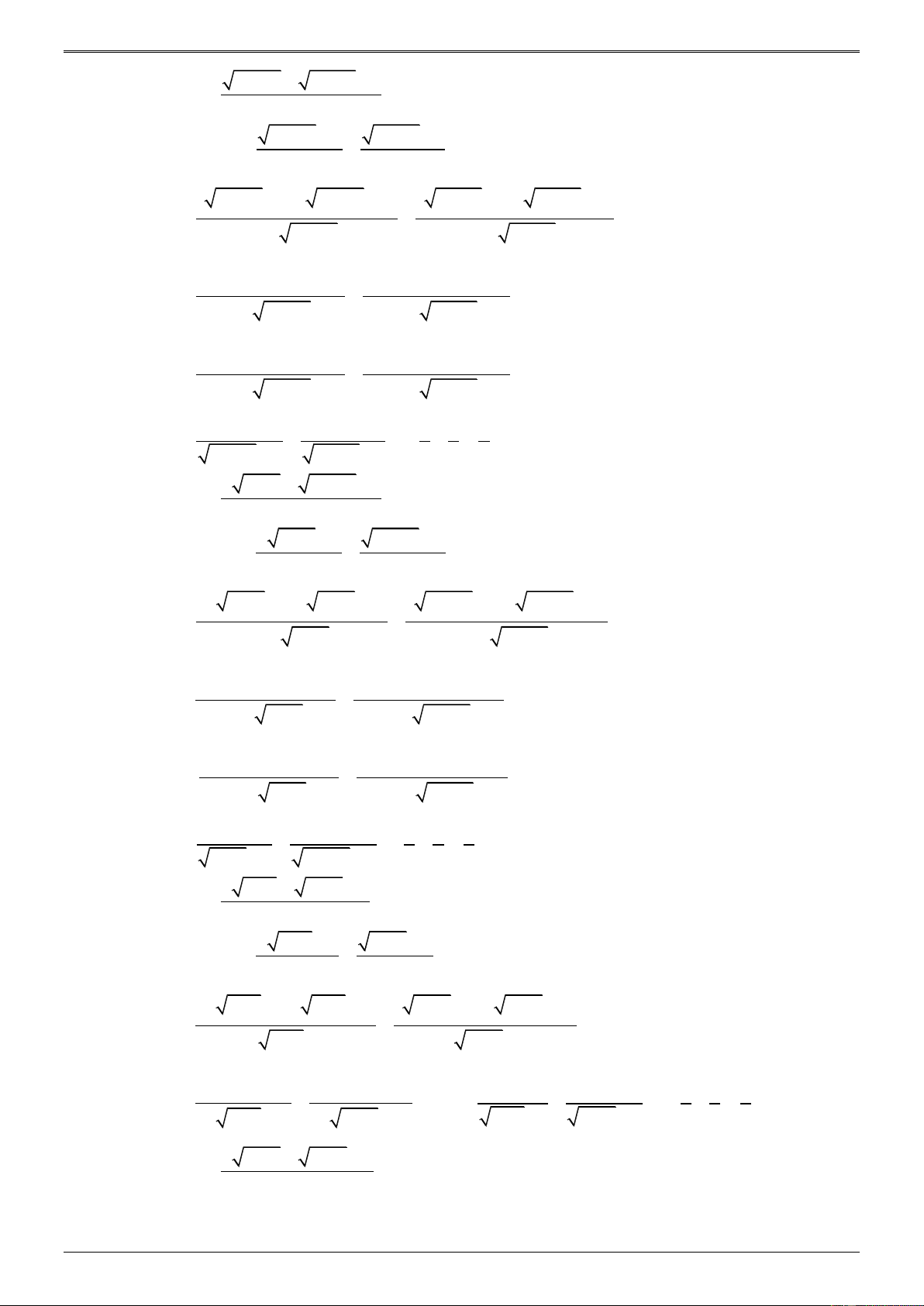
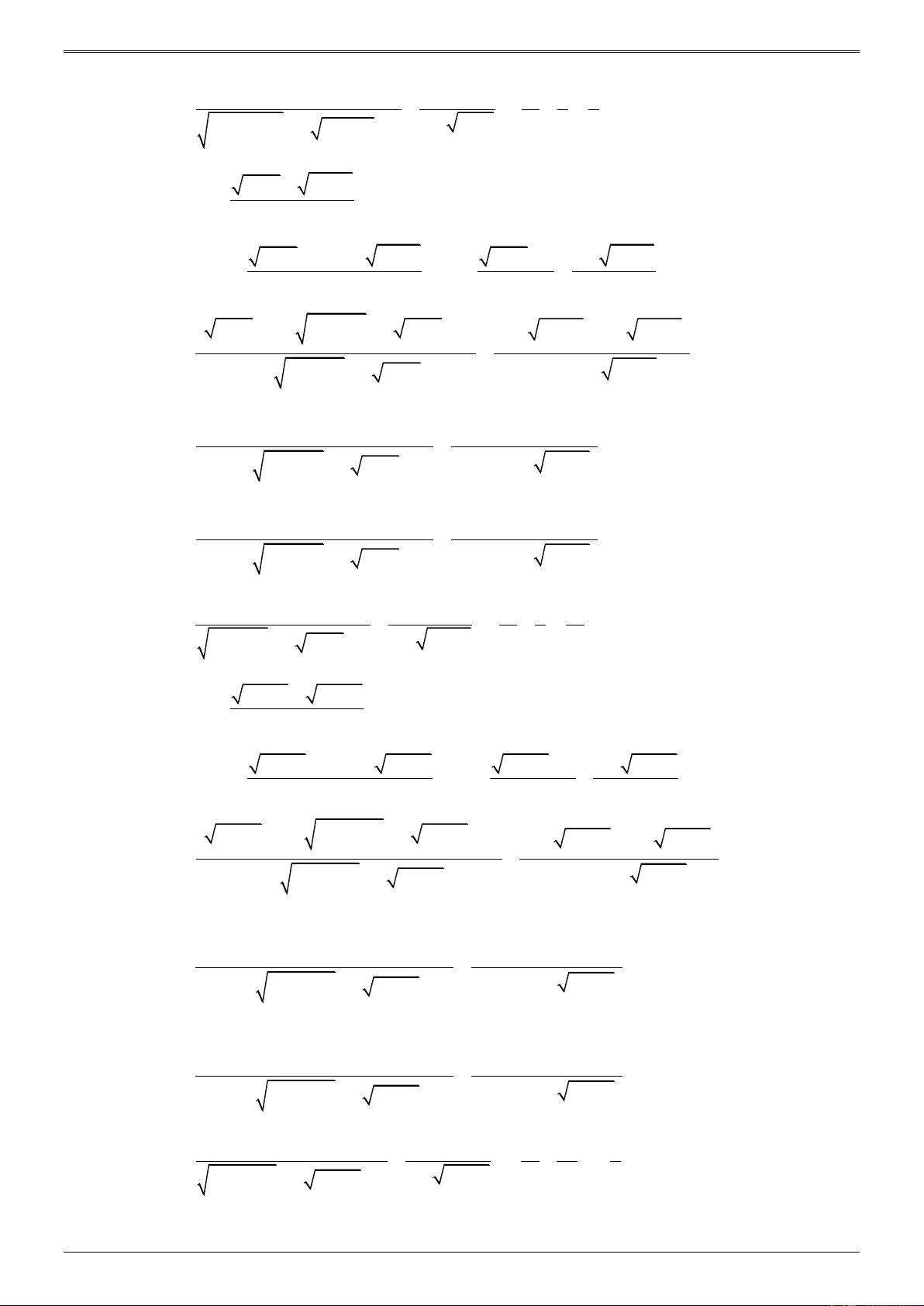
chéo), rồi sau đó đơn giản biểu thức để khử dạng vô định.  VÍ DỤ 2 2x + 3x −14 11
Ví dụ 1. Tính giới hạn A = lim . Đs: A = . 2 x→2 x − 4 4 Lời giải 7 − + 2 2(x 2)(x ) 2x + 3x −14 2x + 7 11 Ta có 2 A = lim = lim = lim = 2 x→2 x→2 x→2 x − 4 (x− 2)(x+ 2) x + 2 4 ! Cần nhớ: 2
f (x) = ax + bx + c = a ( x − x x − x
với x , x là 2 nghiệm của phương trình 1 ) ( 2 ) 1 2
f ( x) = 0 . Học sinh thường quên nhân thêm a . 3 2
2x − 5x − 2x − 3 11
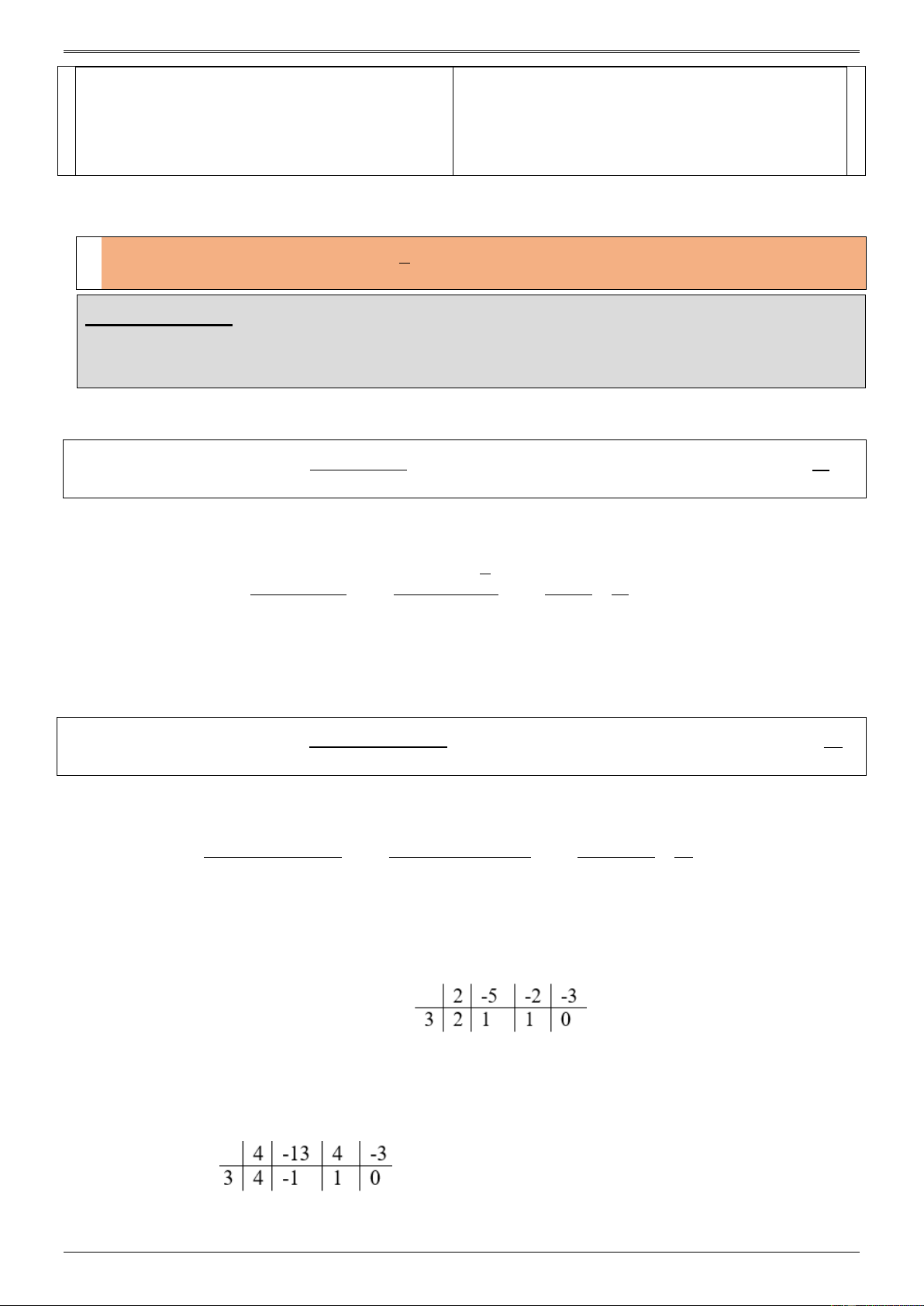
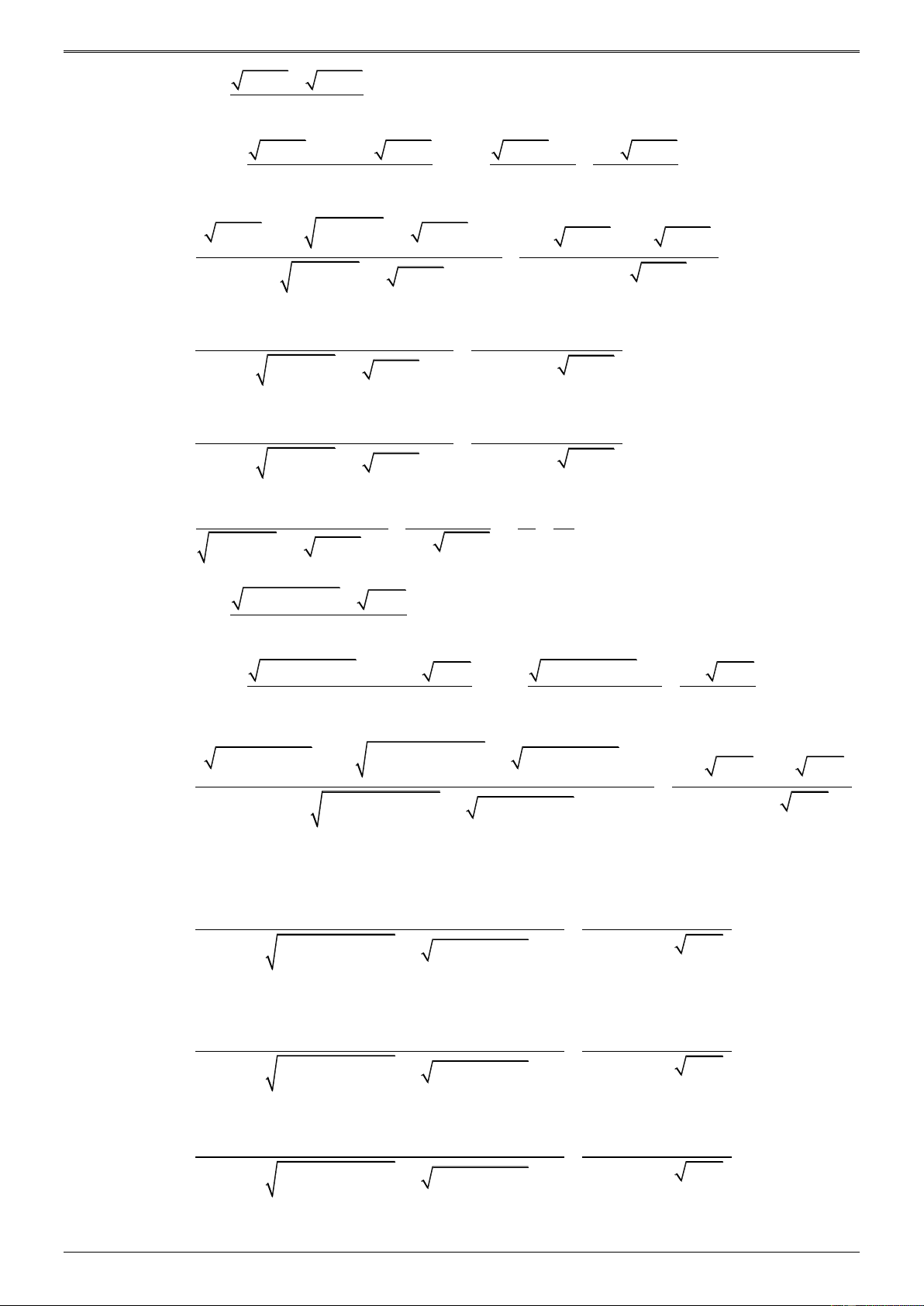
Ví dụ 2. Tính giới hạn A = lim . Đs: A = . 3 2
x→2 4x −13x + 4x − 3 17 Lời giải − − − (x −3)( 2 3 2 2x + x x x x + ) 2 1 2 5 2 3 2x + x +1 11 A = lim = lim = lim = 3 2 x→
4x −13x + 4x − 3 x→ (x −3)( 2 3 3 4x − x + ) 2 x 3 1
→ 4x − x +1 17
Nhận xét: Bảng chia Hooc – nơ (đầu rơi, nhân tới cộng chéo) như sau: Phân tích 3 2
2x − 5x − 2x − 3 thành tích số: 3 2
x − x − x − = (x − )( 2 2 5 2 3 3 2x + x + ) 1 Phân tích 3 2
4x −13x + 4x − 3 thành tích số: 2 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 3 2
x − x + x − = (x − )( 2 4 13 4 3 3 4x − x + ) 1 . 100 x − 2x +1 49
Ví dụ 3. Tính giới hạn A = lim . Đs: A = . 50 x 1 → x − 2x +1 24 Lời giải − + − − − x ( 99 100 100 x − ) 1 − ( x x x x x x − ) 1 2 1 ( ) ( 1) Ta có A = lim = lim = lim 50 50 x→ x − 2x +1 x→
(x − x) − (x −1) x→ x ( 49 1 1 1 x − ) 1 − ( x − ) 1 x ( x − ) 1 ( 98 97 96
x + x + x + .... + x + ) 1 − ( x − ) 1 = lim x→ x ( x − ) 1 ( 48 47 46 1
x + x + x + .... + x + ) 1 − ( x − ) 1 ( x − ) 1 ( 99 98 97 2
x + x + x + .... + x + x − ) 1 = lim x→ ( x − ) 1 ( 49 48 47 2 1
x + x + x + .... + x + x − ) 1 ( 99 98 97 2
x + x + x + .... + x + x − ) 1 98 49 = lim = = x→ ( 49 48 47 2 1
x + x + x + .... + x + x − ) 1 48 24
!Cần nhớ: Hằng đẳng thức n
x − = ( x − )( n 1 − n−2 2 1 1 x + x
+ ....+ x + x + ) 1 .
Chứng minh: Xét cấp số nhân 2 3 1 1, , , ,...., n x x x x − có n = = số hạng và u 1, q . x 1 Khi đó n − q −1 n x − n 1 2 1
S = 1+ x + x + ... + x = u =1. n
x −1= x −
+ x + x + + x − n ( ) 1 ( 2 n 1 1 ... . 1 ) q −1 x −1
BÀI TẬP ÁP DỤNG
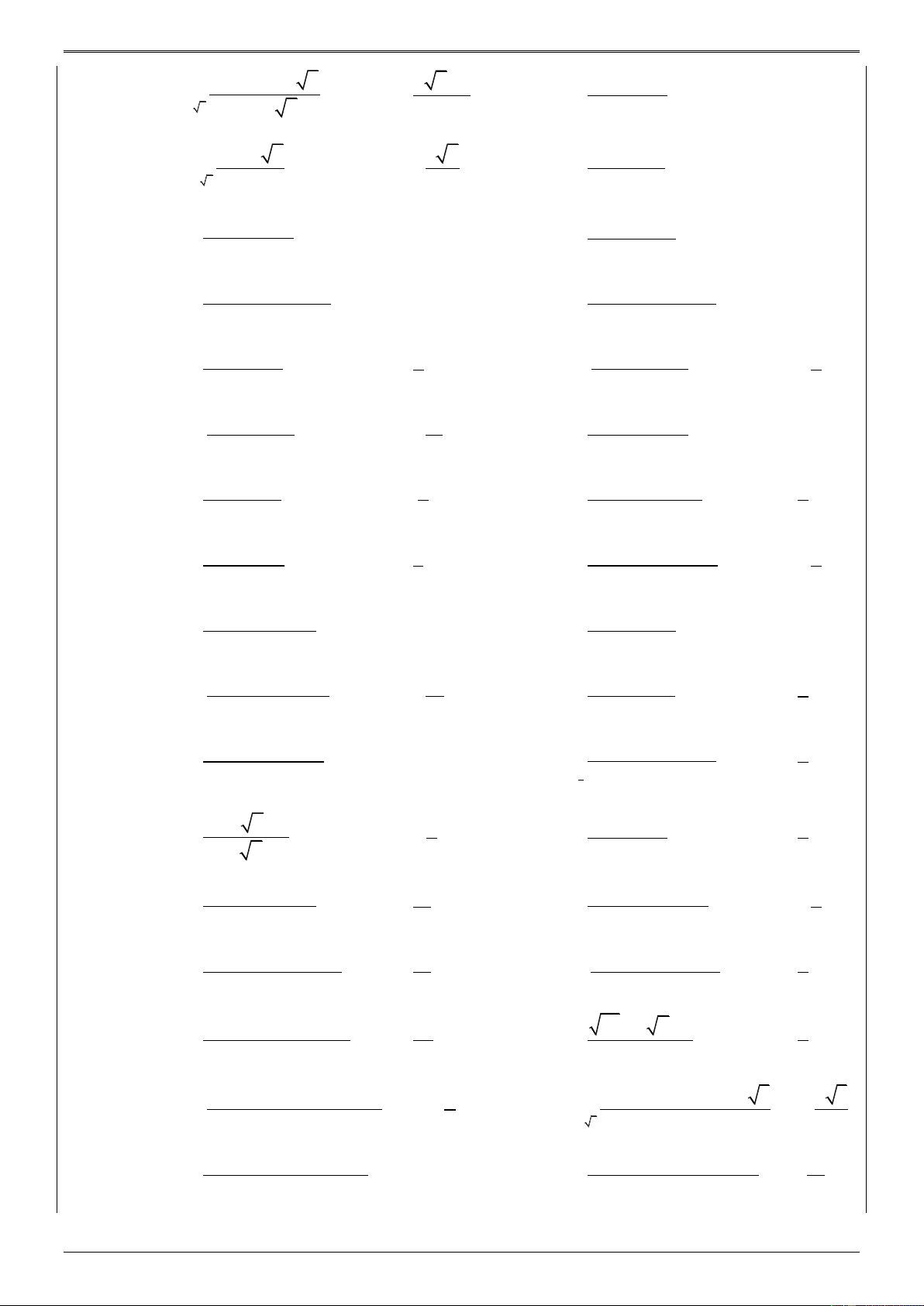
Bài 1. Tính các giới hạn sau: 2 x − 3x + 2 2 x −1 1) A = lim . ĐS: 1 A = . 2) A = lim . ĐS: 2 A = . 2 x→2 x − 4 4 2 x 1 → x + 3x − 4 5 2 x − 7x +12 2 x − 9x + 20 3) A = lim . ĐS: 1 A = − . 4) A = lim . ĐS: 1 A = . 2 x 3 → x − 9 6 2 x 5 → x − 5x 5 2 3x −10x + 3 2 x + 2x − 3 5) A = lim . ĐS: A = 8 . 6) A = lim . ĐS: 4 A = . 2 x 3 → x − 5x + 6 2 x 1
→ 2x − x −1 3 4 x −16 x − 2 x − 3 7) A = lim . ĐS: A = 16 − . 8) A = lim .ĐS: 4 A = − . 2 x 2
→− x + 6x + 8 x 1
→ x − 5 x + 4 3 3 x − 8 3 x + 8 9) A = lim . ĐS: A = 12 . 10) A = lim . ĐS: 12 A = . 2
x→2 x − 3x + 2 2 x 2
→− x +11x +18 7 Bài 2. Tính các giới hạn sau: 3 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 3 2
2x − 5x + 2x +1 3 x − 3x + 2 1) A = lim . ĐS: A = −1. 2) A = lim . ĐS: 1 A = . 2 x 1 → x −1 4 x 1 → x − 4x + 3 2 3 2
2x + 5x + 4x +1 4 3
x − x − x +1 3) A = lim . ĐS: 1 A = . 4) A = lim . ĐS: 3 A = − . 3 2 x 1 →−
x + x − x −1 2 3 2 x 1
→ x − 5x + 7x − 3 2 3 2
2x − 3x + x + 9 + 7 3 + 5) A = lim . ĐS: 18 19 3 A = . 2 x→− 3 3 − x 6 3 2
x − 5x + 3x + 9 6) A = lim . ĐS: A = 0 . 4 2 x 3 → x − 8x − 9 3 1− x  1 12  7) A = lim . ĐS: 3 A = . 8) A = lim −   . ĐS: 1 A = . 4 2 x 1 → x − 4x + 3 4 3
x→2  x − 2 x − 8  2  1 1  9) A = lim +   . ĐS: A = 2 − . 2 2
x→2  x − 3x − 2
x − 5x − 6   1 1  10) A = lim −   . ĐS: 1 A = . 2 3 x 1
→  x + x − 2 x −1 9 Bài 3. Tính các giới hạn sau: 20 x − 2x +1 50 x −1 1) A = lim . ĐS: 8 A = . 2) A = lim . ĐS: A = 50 − . 30 x 1 → x − 2x +1 14 2 x 1 → x − 3x + 2 n
x − nx + n −1 2 n − n 3) A = lim
(Với n là số nguyên). ĐS: A = . x→ (x − )2 1 1 2 n 1 x + − (n + ) 1 x + n n (n + ) 1 4) A = lim . ĐS: A = . x→ (x − )2 1 1 2 2 3
x + x + x + ... n + x − n n (n + ) 1 5) A = lim
( m, n là số nguyên) . ĐS: A = . 2 3 1
→ x + x + x + ... m x + x − m m (m + ) 1  m nm − n 6) A = lim −   . ĐS: A = . 1 → 1 m − x 1 n x − x  2  LỜI GIẢI 2 x − 3x + 2 (x − ) 1 ( x − 2) x −1 1 Bài 1. 1) Ta có A = lim = lim = lim = . 2 x→2 x→2 x − 4
(x −2)(x + 2) x→2 x + 2 4 2 x −1 (x − ) 1 ( x + ) 1 x +1 2 2) Ta có A = lim = lim = lim = . 2 x 1 → x 1 x + 3x − 4 → ( x − ) 1 ( x + 4) x 1 → x + 4 5 2 x − 7x +12 (x −3)(x −4) x − 4 1 3) Ta có A = lim = lim = lim = − . 2 x 3 → x 3 x − 9
→ ( x − 3)( x + 3) x 3 → x + 3 6 4 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 2 x − 9x + 20 (x −4)(x −5) x − 4 1 4) Ta có A = lim = lim = lim = . 2 x 5 → x 5 x − 5x → x ( x − 5) x 5 → x 5 2 3x −10x + 3 (3x − ) 1 ( x − 3) 3x −1 5) Ta có A = lim = lim = lim = 8 . 2 x 3 → x 3 x − 5x + 6
→ ( x − 2)( x − 3) x 3 → x − 2 2 x + 2x − 3 (x − ) 1 ( x + 3) x + 3 4 6) Ta có A = lim = lim = lim = . 2 x 1 → x 1 2x − x −1 → ( x − ) 1 (2x + ) x 1 1 → 2x +1 3 x −
(x −2)(x + 2)( 2x + 4) (x −2)( 2 4 x + 4 16 ) 7) Ta có A = lim = lim = lim = 1 − 6. 2 x→ 2 − x→ 2 x + 6x + 8 − (x + 2)(x + 4) x→ 2 − (x + 4) − + + x − x − ( x )1( x 3) ( x 3 2 3 ) 4 8) Ta có A = lim = lim = lim = − . x 1 → x 1 x − 5 x + 4 → ( x − ) 1 ( x − 4) x 1 → ( x − 4) 3 x −
(x −2)( 2x +2x +4) ( 2 3 x + 2x + 4 8 ) 9) Ta có A = lim = lim = lim =12. 2 x→2 x→2 x − 3x + 2 (x −2)(x − ) x→2 1 (x − ) 1
! Cần nhớ: Hằng đẳng thức 3 3 + = ( + )( 2 2 a b a b
a − ab + b ) và 3 3 − = ( − )( 2 2 a b a b
a + ab + b ) . x +
(x + 2)( 2x −2x+ 4) ( 2 3 x − 2x + 4 8 ) 12 10) Ta có A = lim = lim = lim = . 2 x→ 2 − x→ 2 x +11x +18 − (x + 2)(x +9) x→ 2 − (x +9) 7 − + + (x − ) 1 ( 2 3 2 2x − 3x x x x − ) 2 1 2 5 2 1 2x − 3x −1 Bài 2. 1) A = lim = lim = lim = 1 − . 2 x 1 → x 1 x −1 → (x − ) 1 ( x + ) x 1 1 → x +1 x − 3x + 2 (x − )2 3 1 ( x + 2) x + 2 1 2) A = lim = lim = lim = . 4 x→ x − 4x + 3 x→ ( x − )2 1 ( x + 2x + 3) 2 2 1 1 x 1 → x + 2x + 3 2
2x + 5x + 4x +1 (x + )2 3 2 1 (2x + ) 1 2x +1 1 3) A = lim = lim = lim = . 3 2 x→ 1 − x→ 1
x + x − x −1 − (x + )2 1 ( x − ) x→ 1 1 − x −1 2 − − + (x − )2 1 ( 2 4 3 x + x x x x + ) 2 1 1 x + x +1 3 4) A = lim = lim = lim = − . 3 2 x 1 → x 1
x − 5x + 7x − 3 → (x − )2 1 ( x − 3) x 1 → x − 3 2   + − + + +
x − x + x + + (x 3)  ( 2 3 2 2x (3 2 3)x 7 3 3 2 3 9 7 3 ) 5) Ta có A = lim = lim − 2 −   x→− 3 x→− 3 3 x  (x+ 3)( 3− x)    2
 2x −(3+2 3)x+7+3 3  18+19 3 lim   = − = . x→− 3  3 − x  6  
x − 5x + 3x + 9 (x − ) 1 ( x − 3)2 3 2 (x − ) 1 ( x − 3) 6) Ta có A = lim = lim = lim = 0. 4 2 2 2 x 3 → x 3 → x 3 → x − 8x − 9
(x −3)(x +3)(x + )1 (x +3)(x + )1 5 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC − (x − ) 1 ( 2 −x − x − ) 1 ( 2 3 −x − x x − ) 1 1 3 7) Ta có A = lim = lim = lim = . 4 2 x→ x − 4x + 3 x→ ( x − ) 1 ( 3 2
x + x − 3x − 3) x→ ( 3 2 1 1 1
x + x − 3x − 3) 4 3  1 12  x −12x +16 8) Ta có A = lim − = lim   3 x→  x − 2 x − 8 x→  (x −2)( 3 2 2 x − 8)
(x + 4)(x − 2)2 x + 4 1 = lim = = . x→ (x − 2) lim 2 (x + 2x + 4) 2 2 2
x→2 x + 2x + 4 2 2 2  1 1 
x − 5x − 6 + x − 3x − 2 9) Ta có A = lim + = lim   2 2
x→  x − 3x − 2 x − 5x − 6 x→ 
( 2x −3x−2)( 2 2 2 x − 5x − 6) 2( x − 2)2 2 = lim = = − . x→ (x −2) lim 2 2 2
(x −3)(x − ) x→2 1 (x −3)(x − ) 1 3 2 3 2  1 1 
x −1− x − x + 2
x − x − x +1 10) Ta có A = lim − = lim = lim   2 3
x→  x + x − 2 x −1 x→ 
( 2x + x−2)( 3x − )1 x→ ( 2x + x−2)( 3 1 1 1 x − ) 1 (x − )2 1 ( x + ) 1 x +1 1 = lim = = . x→ ( x − ) lim 2 1 ( x + 2)( 2 1 x + x + ) x 1 1 → ( x + 2)( 2 x + x + ) 1 9 20 x − 2x +1
x − x − ( x − ) 1 x ( 19 20 x − ) 1 − ( x − ) 1 Bài 3. 1) Ta có A = lim = lim = lim 30 30 x→ x − 2x +1 x→
x − x − ( x − ) 1 x→ x ( 29 1 1 1 x − ) 1 − ( x − ) 1 x ( x − ) 1 ( 18 17
x + x + ... + x + ) 1 − ( x − ) 1 (x − ) 1 ( 19 18
x + x + ... + x − ) 1 = lim = x→ x ( x − ) 1 ( lim 28 27
x + x + ... + x + ) 1 − ( x − ) 1 x→ ( x − ) 1 ( 29 28 1 1
x + x + ... + x − ) 1 ( 19 18
x + x + ... + x − ) 1 18 9 = lim = = . x→ ( 29 28 1
x + x + ... + x − ) 1 28 24 x − (x − ) 1 ( 49 48 50
x + x + ... + x+ ) 49 48 1 1
x + x + ... + x+1 2) Ta có A = lim = lim = lim = 5 − 0 2 x 1 → x 1 x − 3x + 2 → (x − ) 1 ( x − 2) x 1 → x − 2 − + − ( n n x − ) 1 − n ( x x nx n − ) 1 1 3) Ta có A = lim = x→ (x − ) lim 2 1 x→ (x − )2 1 1 1 (x − ) 1 ( n 1 − n−2 x + x +...+ x+ )
1 − n ( x − ) 1 = lim x→ (x − )2 1 1 (x − ) 1 ( n 1 − n−2 x + x +...+ x+1− n) n 1 − n−2 x + x +...+ x+1− n = lim = x→ (x − ) lim 2 1 x 1 1 → x −1 n 1 − n−2 2 x −1+ x
−1+...+ x −1+ x −1 = lim x 1 → x −1 6 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC (x − ) 1 ( n−2 n−3 x + x +...+ x+ ) 1 + ( x − ) 1 ( n−3 n−4 x + x + ...+ x+ ) 1 + ... + ( x − ) 1 = lim x 1 → x −1 − = n n lim ( n−2 n−3 x + x +...+ x+ ) 1 + ( n−3 n−4 x + x +...+ x+ )
1 + ... +1 = (n − ) + (n − ) 2 1 2 + ... +1 = . x 1 → 2 + + x − (n + ) n n n 1 x + n ( 1 1 x
− x) − n(x − ) 1 x (x − )
1 − n ( x − ) 1 4) Ta có A = lim = = x→ (x − ) lim lim 2 1 x→ (x − )2 1 x→ (x − )2 1 1 1 1 x ( x − ) 1 ( n 1 − n−2 x + x +...+ x+ )
1 − n ( x − ) 1 (x − ) 1 ( n n 1
x + x − + ... + x− n) = lim = x→ (x − ) lim 2 1 x→ (x − )2 1 1 1 n n 1 − 2 n n 1 − 2 x + x
+...+ x + x − n x −1+ x
−1+...+ x −1+ x −1 = lim = lim x 1 → x 1 x −1 → x −1 (x − ) 1 ( n 1 − n−2 x + x +...+ x+ ) 1 + ( x − ) 1 ( n−2 n−3 x + x + ...+ x+ ) 1 + ... + ( x − ) 1 = lim x 1 → x −1
= lim ( n 1− n−2 x + x +...+ x+ ) 1 + ( n−2 n−3 x + x +...+ x+ ) 1 + ... +1 x 1 → +
= n + (n − ) + (n − ) n (n ) 1 1 2 + ... +1 = . 2 2 3 n n n 1 − 2
x + x + x + ... + x − n x −1+ x
−1+...+ x −1+ x −1 5) Ta có A = lim = lim 2 3 m m m 1 − 2 x 1 → x 1
x + x + x + ... + x − m → x −1+ x
−1+...+ x −1+ x −1 (x − ) 1 ( n 1 − n−2 x + x +...+ x+ ) 1 + ( x − ) 1 ( n−2 n−3 x + x +...+ x+ ) 1 + ... + ( x − ) 1 = lim − − − − x→ ( x − ) 1 ( m 1 m 2 x + x +...+ x+ ) 1 + ( x − ) 1 ( m 2 m 3 1 x + x +...+ x+ ) 1 + ... + ( x − ) 1 ( n 1− n−2 x + x +...+ x+ ) 1 + ( n−2 n−3 x + x +...+ x+ ) 1 + ... +1 = lim − − − − x→ ( m 1 m 2 x + x +...+ x+ ) 1 + ( m 2 m 3 1 x + x +...+ x+ ) 1 + ... +1 n + (n − ) 1 + (n − 2) + ...+1 n (n + ) 1 = lim = . x 1 → m + (m − ) 1 + (m − 2) + ...+1 m (m + ) 1  m n   m 1   n 1  6) Ta có A = lim − = lim − − −       1 m n → 1 1− x 1− x →  1 m − x 1− x   1 n x x − x 1− x   m 1   n 1  = lim − − lim −     1 m → 1 1− x 1− x →  1 n x x − x 1− x m −   ( 2 m 1
1+ x + x + ... + x − ) (1− x)+( 2 1− x − m )+...+( m 1 1− x 1 ) Và lim − = lim = lim   1 m → 1 m → 1 1− x 1− x  1− x → 1− xm x x x (1− x) 1  + (1+ x) +....+  ( 2 m−2
1+ x + x + ... + x ) = lim − x→ (1− x)( 2 m 1 1
1+ x + x + ... + x ) 1+ (1+ x) + .... + ( 2 m−2
1+ x + x + ... + x
) 1+2+3+...+m−1 m−1 = lim = = 2 m 1 − x 1 → 1+ x + x + ... + x m 2   − Tương tự n 1 n 1 ta có lim − =   1 → 1 n x − x 1− x  2  m n
m −1 n −1 m − n 7 Vậy lim − = − =   . 1 → 1 m − x 1 n x − x  2 2 2 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC  0
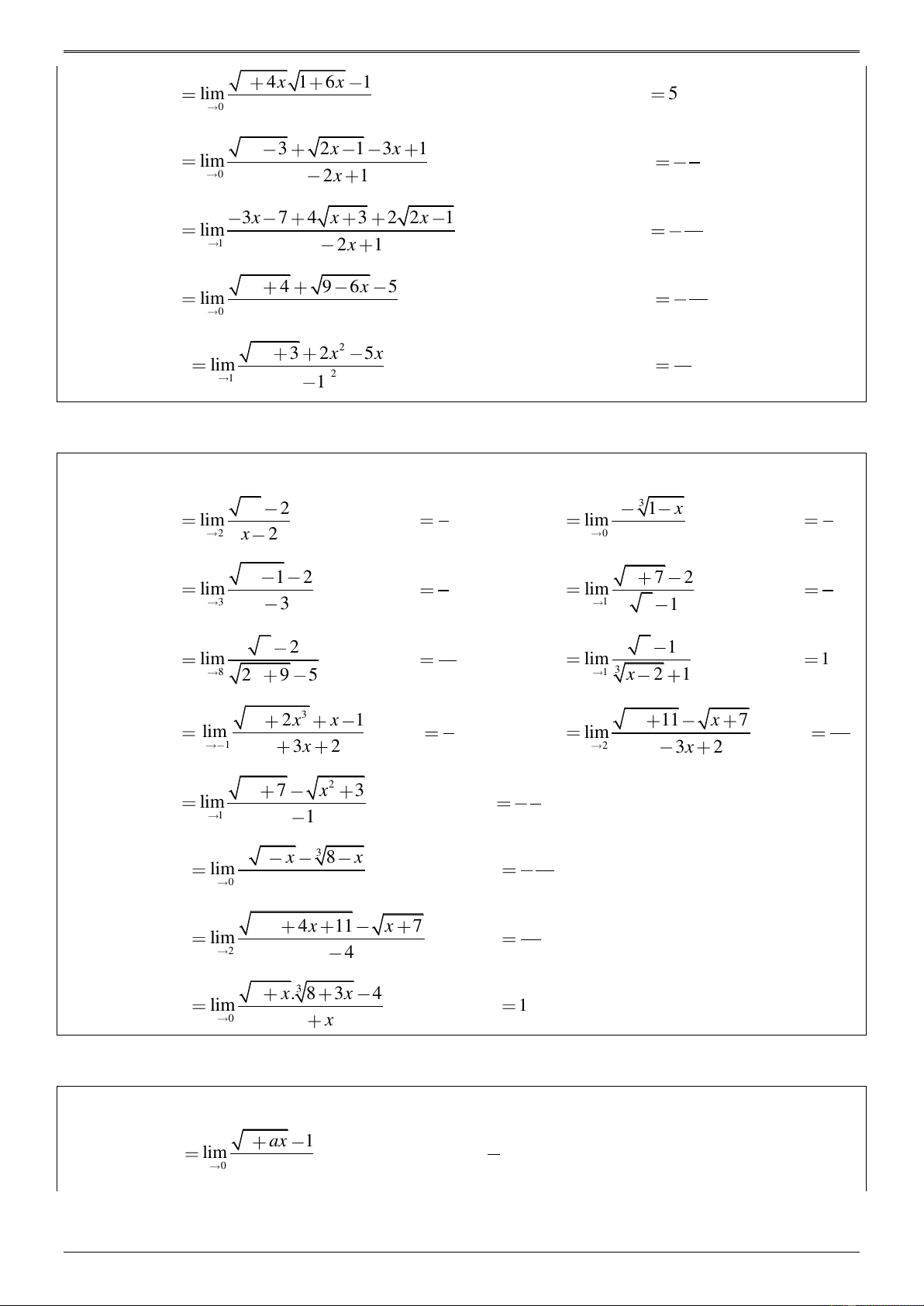
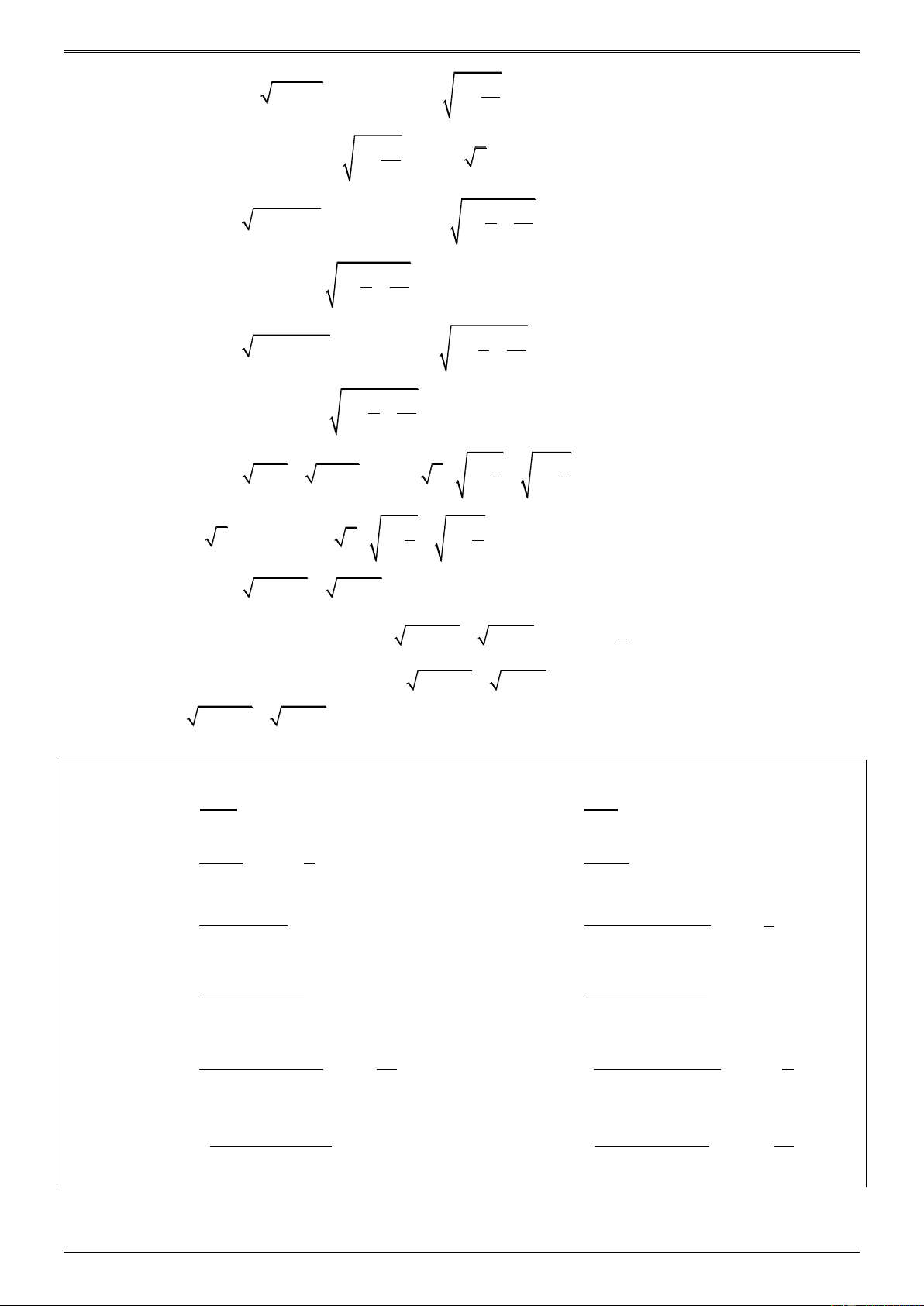
Dạng 2. Tính giới hạn vô định dạng
, trong đó tử thức và mẫu thức có chứa căn thức. 0
Phương pháp giải:
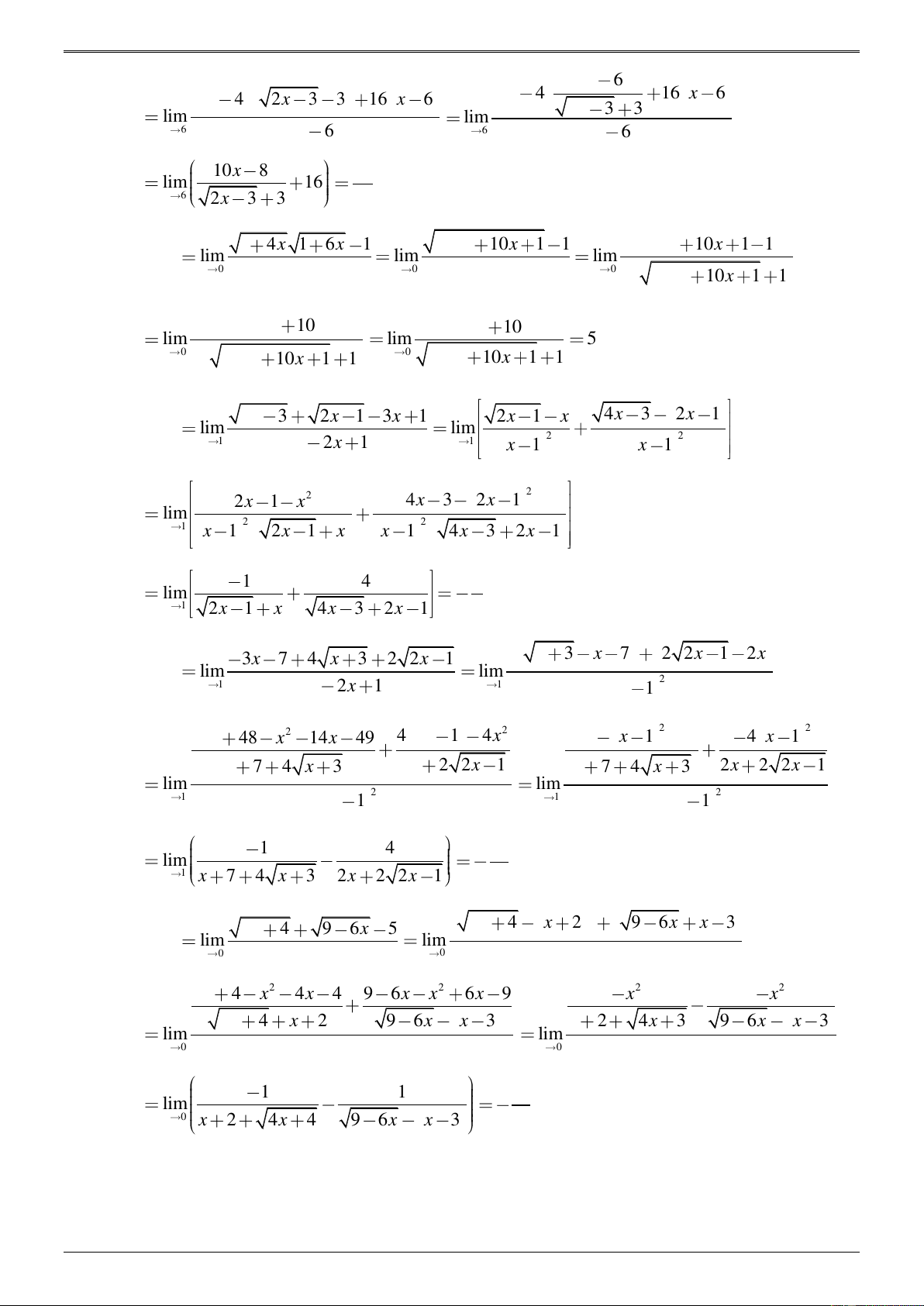
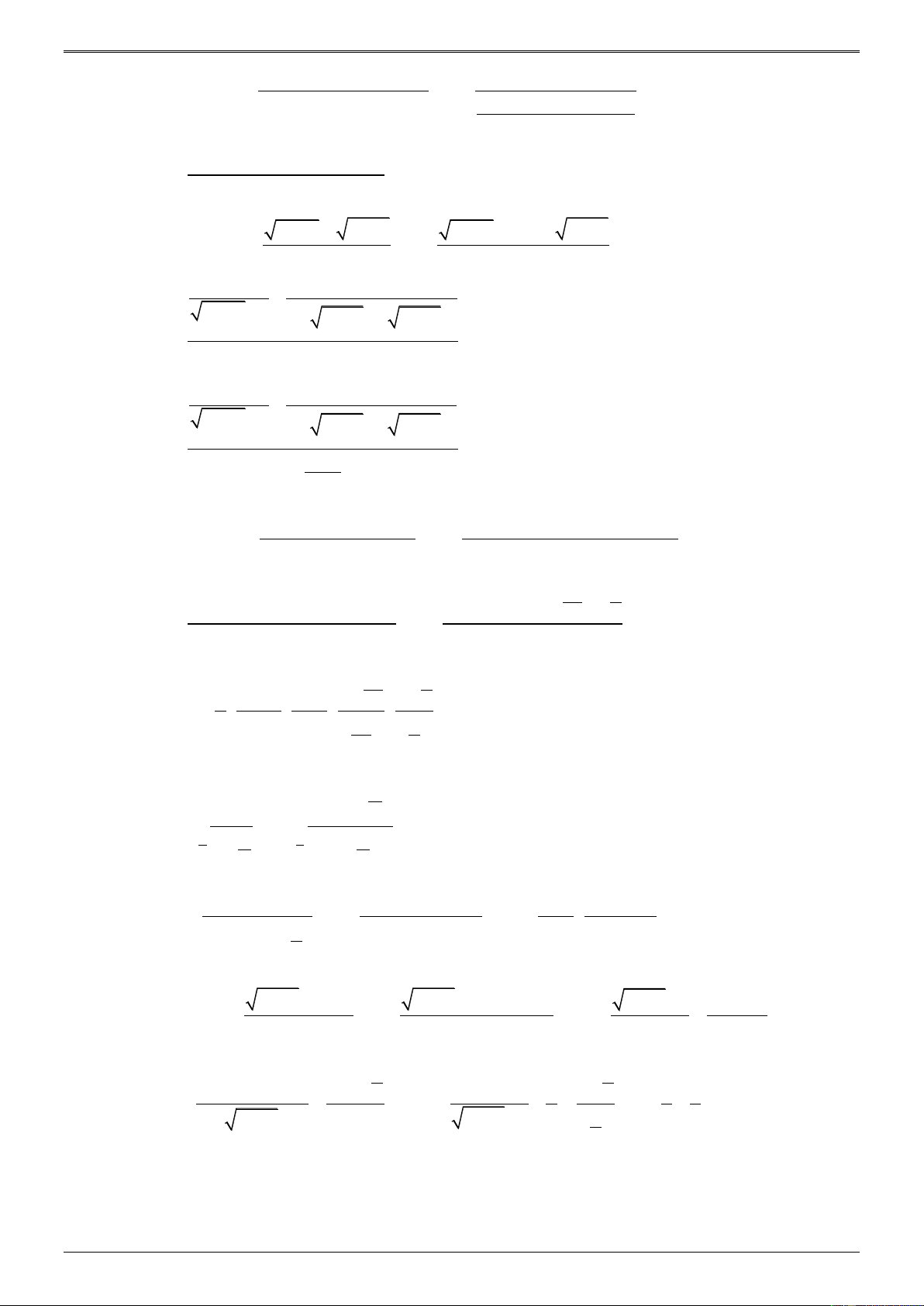
Nhân lượng liên hợp để khử dạng vô định. VÍ DỤ 3 − x + 3 1
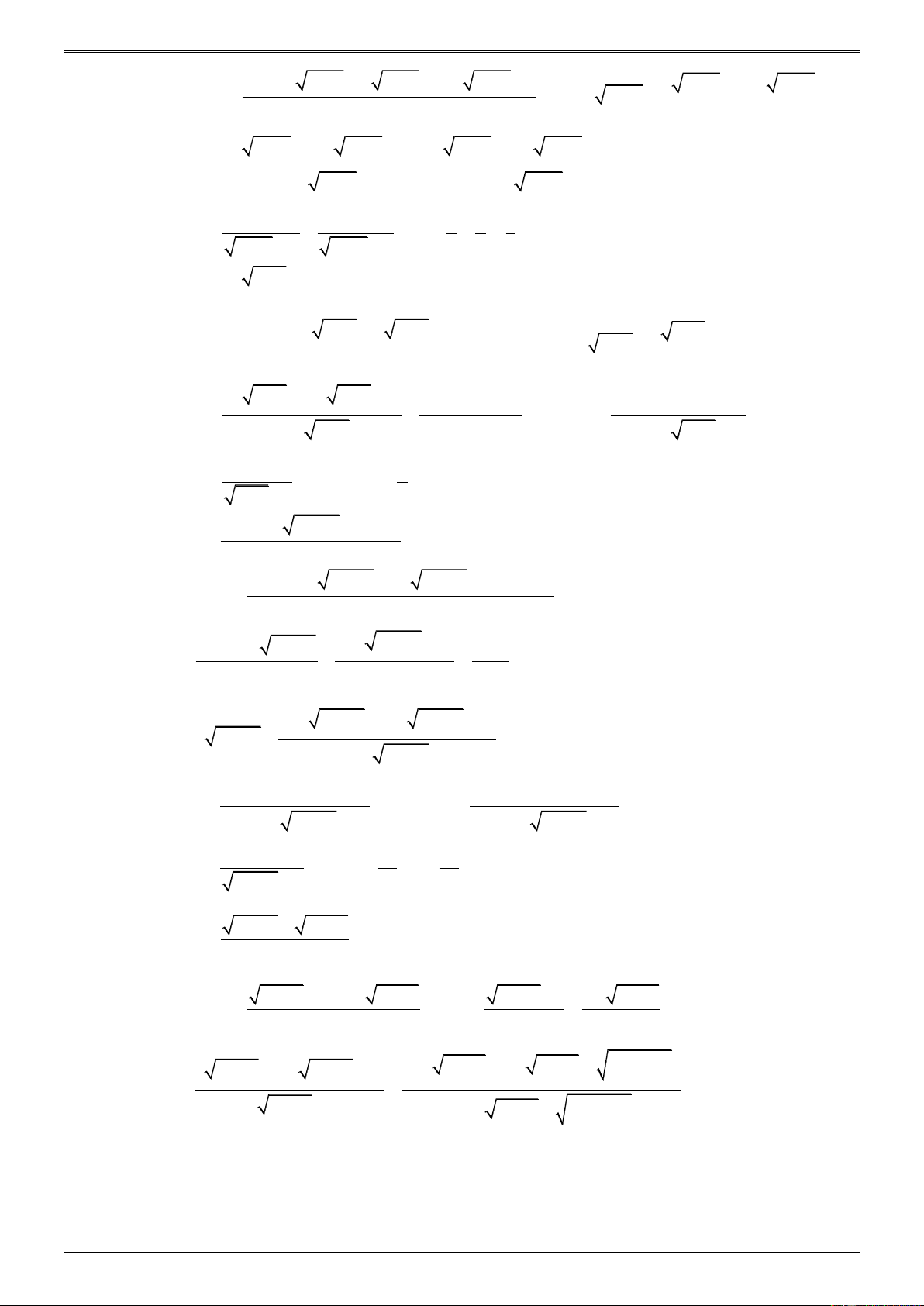
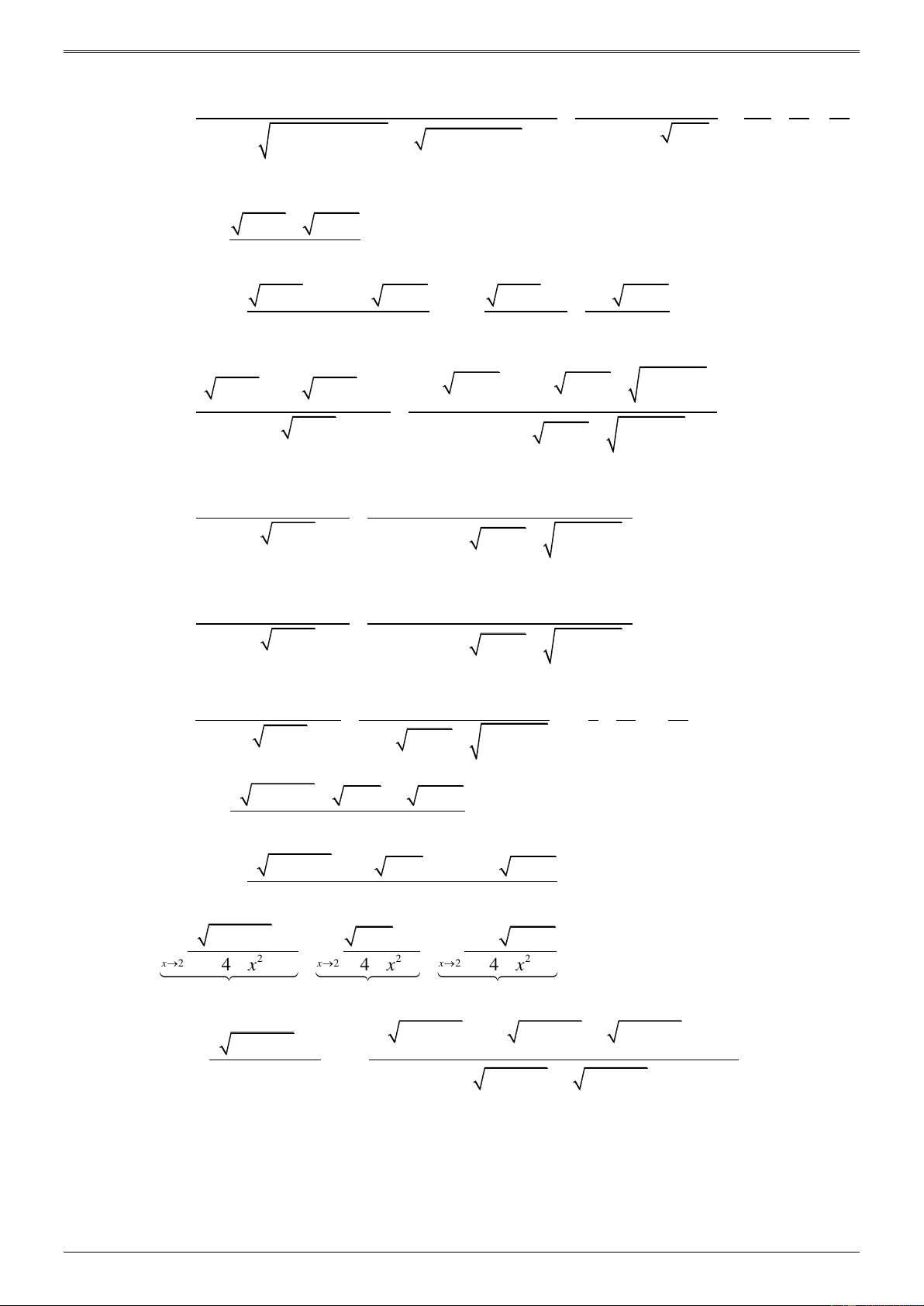
Ví dụ 1. Tính giới hạn B = lim . Đs: B = − . x→6 x − 6 6 Lời giải − + + + − x + (3 x 3)(3 x 3 3 3 ) Ta có: B = lim = lim x→6 x→6 x − 6 (x −6)(3+ x+3) 9 − ( x + 3) 6 − x 1 − 1 − 1 = lim = lim = lim = = −
x→6 ( x − 6)(3+ x + 3) x→6 (x − 6)(3+ x + 3) x→6 3+ x + 3 3+ 6 + 3 6
3 3x + 2 − 5x − 6
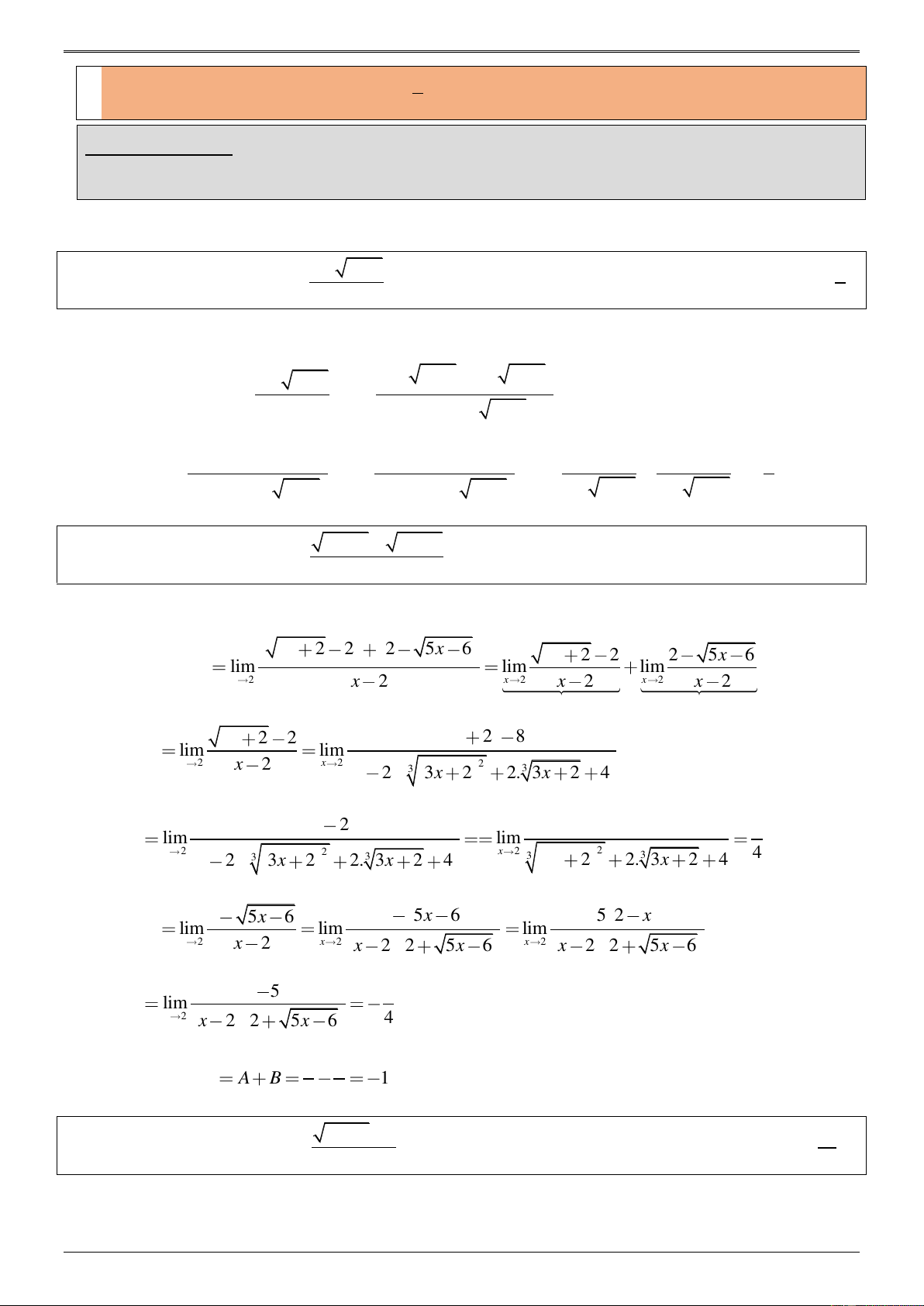
Ví dụ 2. Tính giới hạn E = lim . Đs: E = 1 − . x→2 x − 2 Lời giải 3 3x 2 2 2 5x 6 3 3x 2 2 2 5x 6 Ta có E lim lim lim x 2 x 2 x 2 x 2 x 2 x 2 A B 3 3x 2 2 3x 2 8 A lim lim x 2 x 2 2 x 2 3 3 x 2 3x 2 2. 3x 2 4 3 x 2 3 1 lim lim x 2 2 x 2 2 3 3 3 3 4 x 2 3x 2 2. 3x 2 4 3x 2 2. 3x 2 4 2 5x 6 4 5x 6 5 2 x B lim lim lim x 2 x 2 x 2 x 2 x 2 2 5x 6 x 2 2 5x 6 5 5 lim x 2 x 2 2 5x 6 4 1 5 Suy ra E A B 1 . 4 4 3 5x − 3 + 2
Ví dụ 3. Tính giới hạn L = lim . Đs: 5 L = . x 1 →− x +1 12 Lời giải 8 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 3 5x 3 2 5x 3 8 Ta có: L lim lim x 1 x 1 2 x 1 3 3 x 1 5x 3 2. 5x 3 4 5 x 1 5 5 lim lim . x 1 2 x 1 2 3 3 3 3 12 x 1 5x 3 2. 5x 3 4 5x 3 2. 5x 3 4
3 3x + 2 − 3x − 2 1 −
Ví dụ 4. Tính giới hạn E = lim . Đs: E = . x→2 x − 2 2 Lời giải 3 3x 2 2 3x 2 2 3 3x 2 2 3x 2 2 Ta có E lim lim lim x 2 x 2 x 2 x 2 x 2 x 2 3x 2 8 3x 2 4 lim lim x 2 2 x 2 3 3 x 2 3x 2 2 x 2 3x 2 2. 3x 2 4 3 x 2 3 x 2 lim lim x 2 2 x 2 3 3 x 2 3x 2 2 x 2 3x 2 2. 3x 2 4 3 3 1 3 1 lim lim . x 2 2 x 2 3 3 3x 2 2 4 4 2 3x 2 2. 3x 2 4 3
1+ 2x. 1+ 4x −1 7
Ví dụ 5. Tính giới hạn F = lim . Đs: F = . x→0 x 3 Lời giải 3 3 1 2x. 1 4x 1 1 2x 1 1 2x. 1 4x 1 F lim lim x 0 x 0 x x 3 1 2x. 1 4x 1 1 2x 1 lim lim x 0 x 0 x x 1 2x. 1 4x 1 1 2x 1 lim lim x 0 2 x 0 3 3 x 1 2x 1 x 1 4x 1 4x 1 4. 1 2x 2 4 7 lim lim 1 . x 0 2 x 0 3 3 1 2x 1 3 3 1 4x 1 4x 1
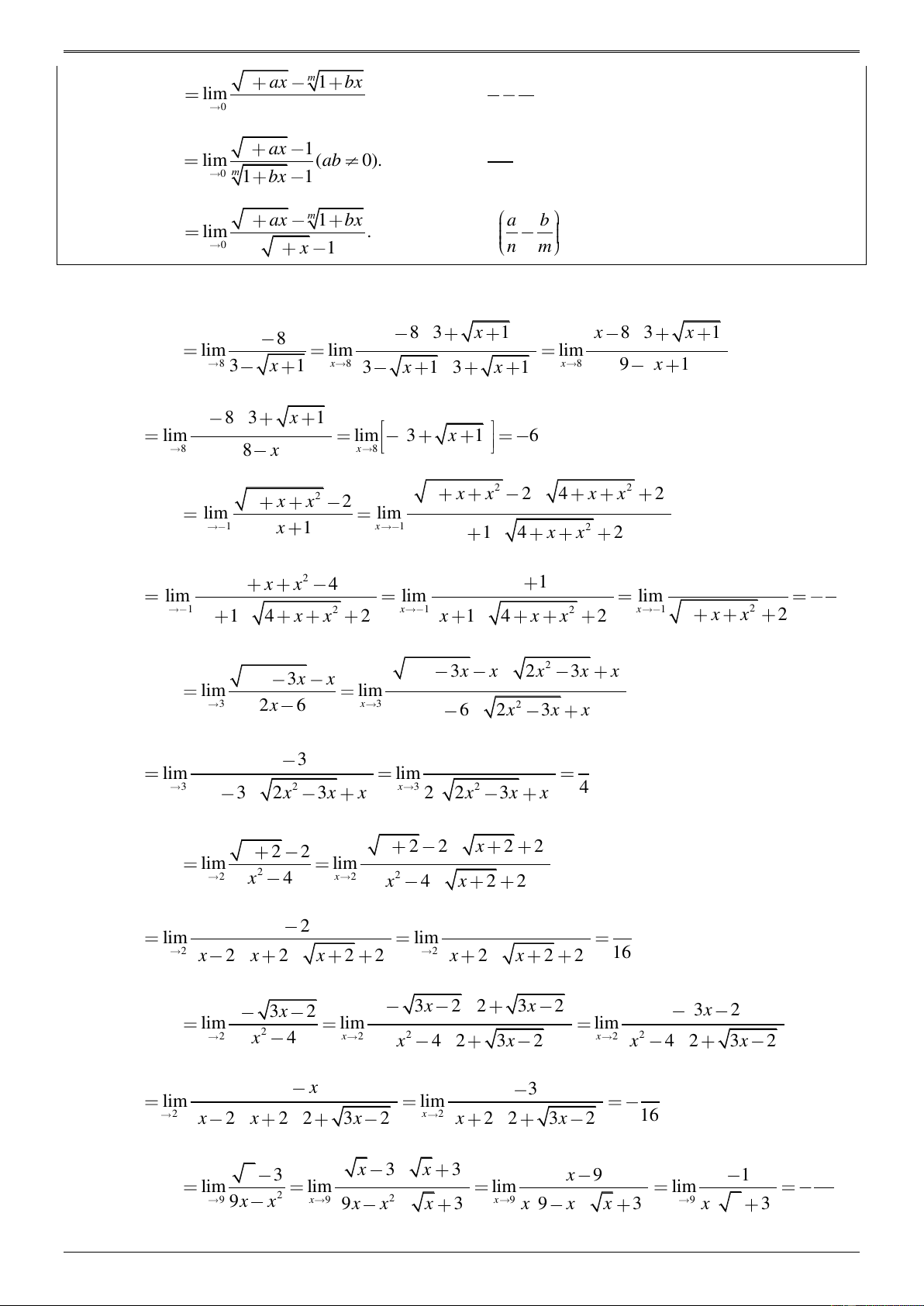
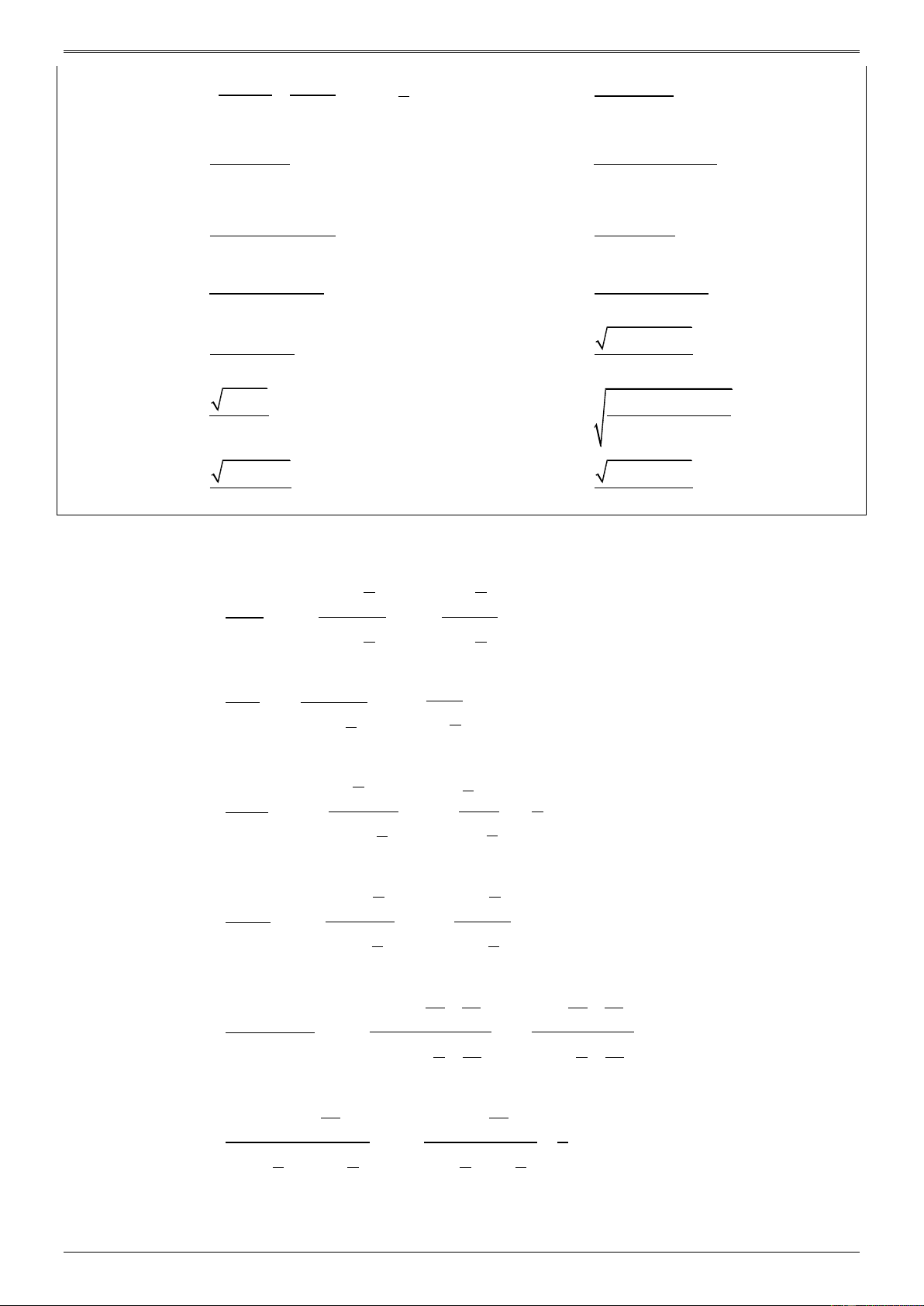
BÀI TẬP ÁP DỤNG Bài 1.
Tính các giới hạn sau: 9 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
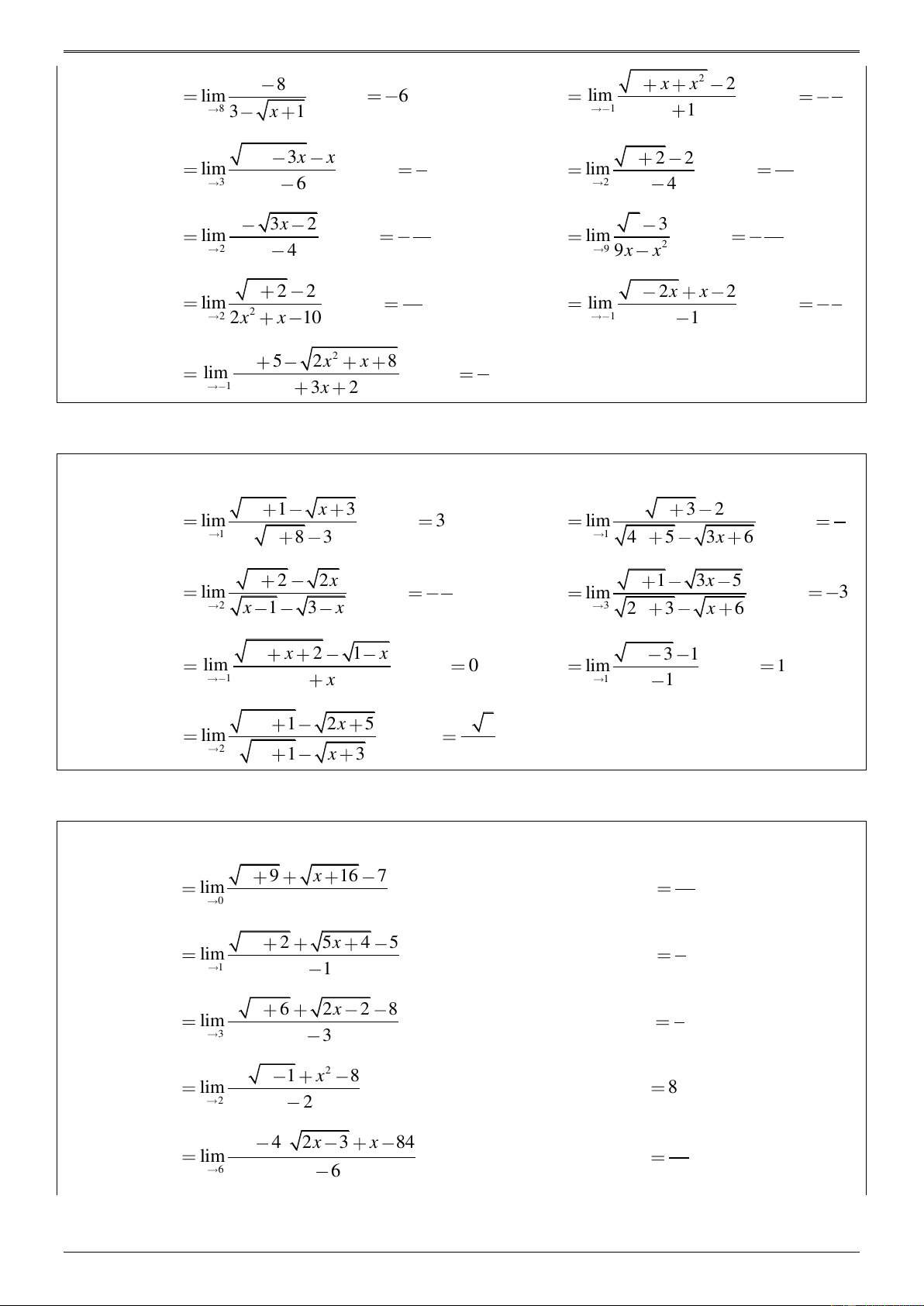
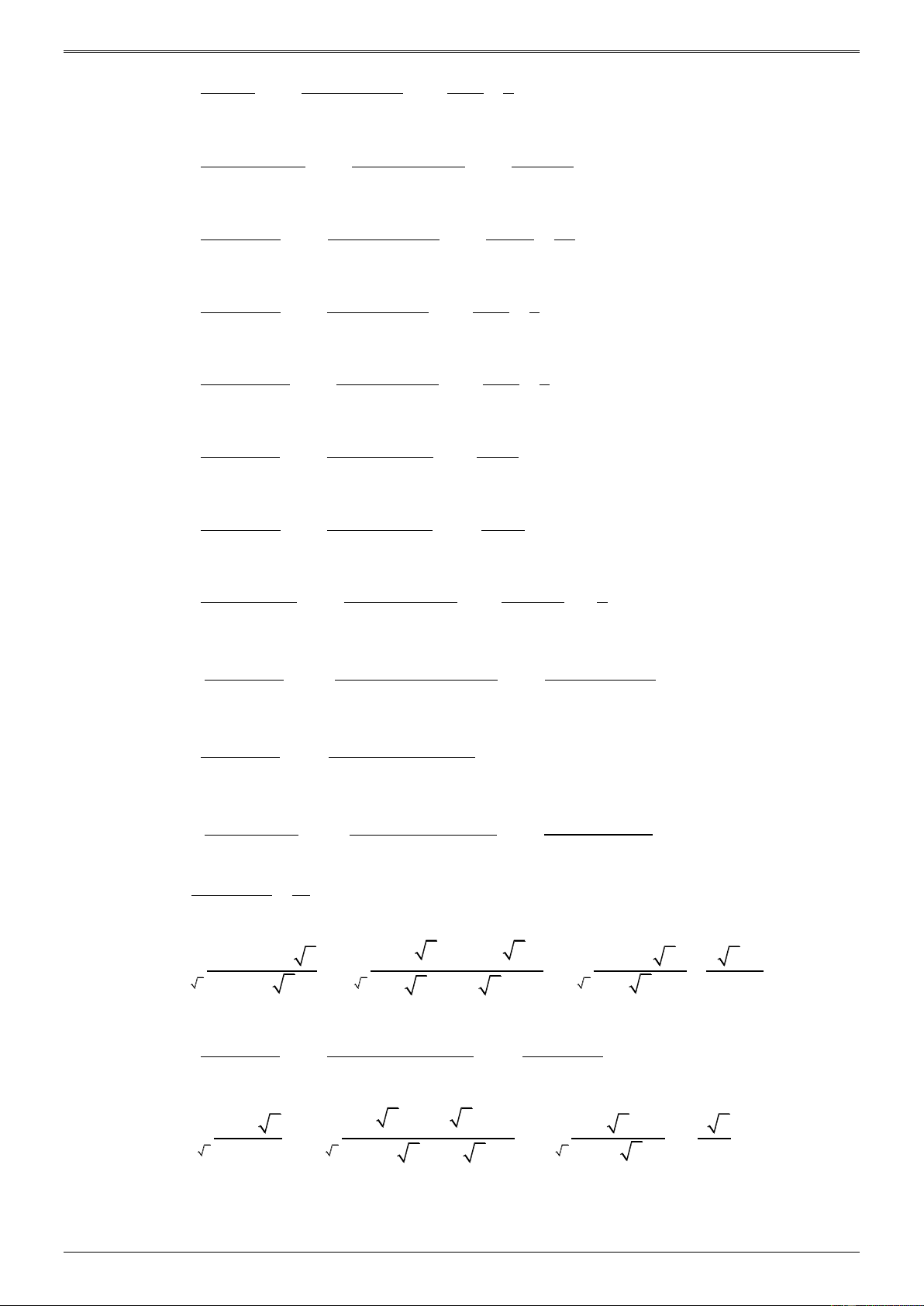
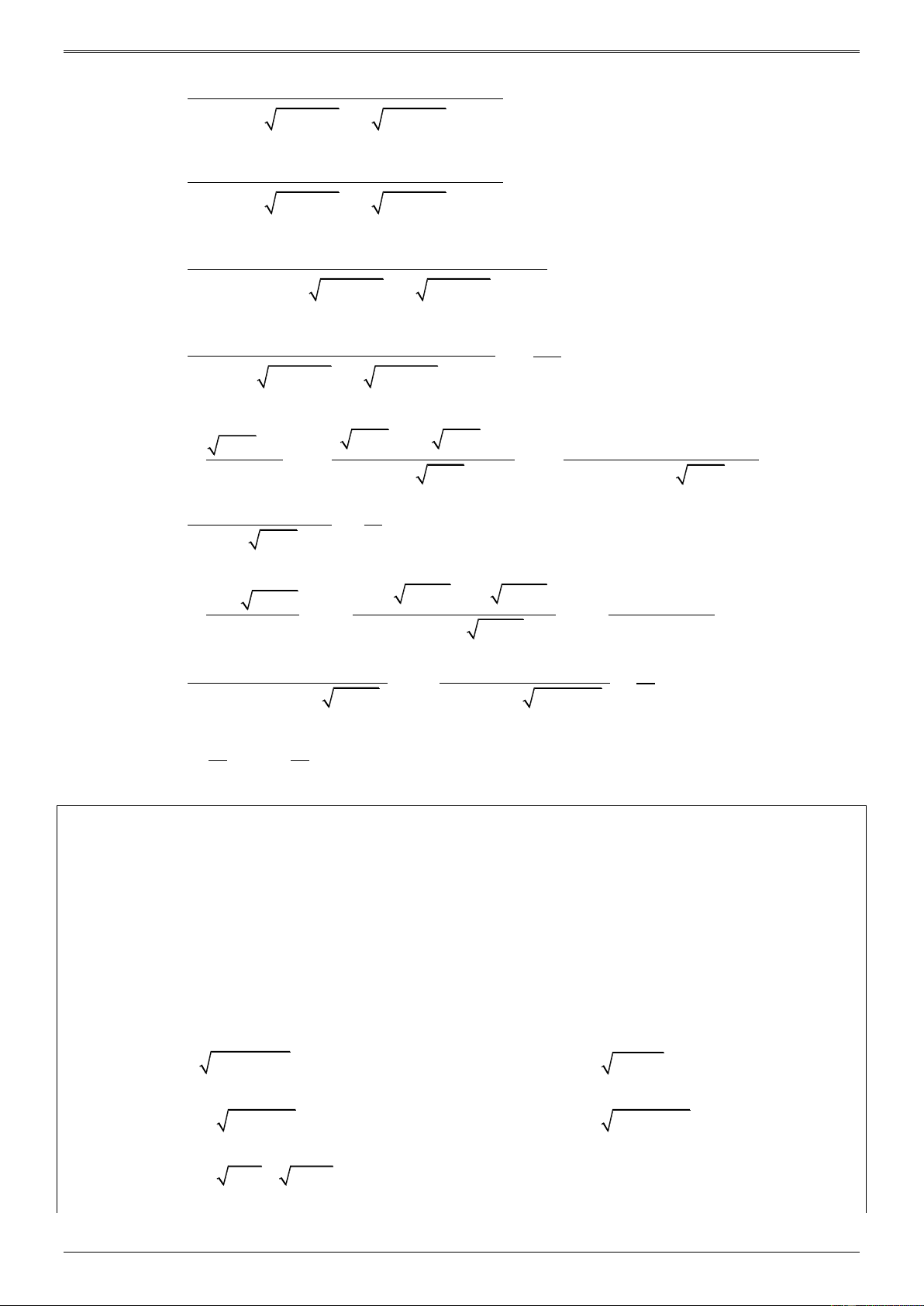
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC x 8 2 4 x x 2 1) B lim . Đs: B 6 2) B lim . Đs: 1 B x 8 3 x 1 x 1 x 1 4 2 2x 3x x x 2 2 3) B lim . Đs: 1 B 4) B lim . Đs: 1 B x 3 2x 6 4 2 x 2 x 4 16 2 3x 2 x 3 5) B lim . Đs: 3 B 6) B lim . Đs: 1 B 2 x 2 x 4 16 2 x 9 9x x 54 x 2 2 7 2x x 2 7) B lim . Đs: 1 B 8) B lim . Đs: 1 B 2 x 2 2x x 10 36 2 x 1 x 1 3 2 2x 5 2x x 8 9) B lim . Đs: 5 B 2 x 1 x 3x 2 2 Bài 2.
Tính các giới hạn sau: 3x 1 x 3 x 3 2 1) B lim . Đs: B 3 2) B lim . Đs: 3 B x 1 x 8 3 x 1 4x 5 3x 6 2 x 2 2x x 1 3x 5 3) B lim . Đs: 1 B 4) B lim . Đs: B 3 x 2 x 1 3 x 4 x 3 2x 3 x 6 2 x x 2 1 x 4 4x 3 1 5) B lim . Đs: B 0 6) B lim . Đs: B 1 4 x 1 x x x 1 x 1 2 2x 1 2x 5 7) B lim . Đs: 2 5 B x 2 2 x 1 x 3 3 Bài 3.
Tính các giới hạn sau: x 9 x 16 7 1) L lim . Đs: 7 B x 0 x 24 2x 2 5x 4 5 2) L lim . Đs: 4 B x 1 x 1 3 2 x 6 2x 2 8 3) L lim . Đs: 5 L x 3 x 3 6 2 2x x 1 x 8 4) L lim . Đs: L 8 x 2 x 2 5x 4 2x 3 x 84 5) L lim . Đs: 74 L x 6 x 6 3 10 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 1 4x 1 6x 1 6) L lim . Đs: L 5 x 0 x 4x 3 2x 1 3x 1 7) L lim . Đs: 5 L 2 x 0 x 2x 1 2 3x 7 4 x 3 2 2x 1 8) L lim . Đs: 17 L 2 x 1 x 2x 1 16 4x 4 9 6x 5 9) L lim . Đs: 5 L 2 x 0 x 12 2 6x 3 2x 5x 10) L lim . Đs: 11 L 2 x 1 x 1 6 Bài 4.
Tính các giới hạn sau: 3 4x 2 3 1 1 x 1) L lim . Đs: 1 L 2) L lim . Đs: 1 L x 2 x 2 3 x 0 x 3 3 2 x 1 2 3 x 7 2 3) L lim . Đs: 1 L 4) L lim . Đs: 1 L x 3 x 3 2 x 1 x 1 6 3 x 2 3 x 1 5) L lim . Đs: 5 L 6) L lim . Đs: L 1 x 8 2x 9 5 12 3 x 1 x 2 1 3 3 10 2x x 1 3 8x 11 x 7 7) L lim . Đs: 3 L 8) L lim . Đs: 7 L 2 x 1 x 3x 2 2 2 x 2 x 3x 2 54 3 3 2 x 7 x 3 9) L lim . Đs: 1 L x 1 x 1 4 3 2 1 x 8 x 11 10) L lim . Đs: L x 0 x 12 3 2 2x 4x 11 x 7 5 11) L lim . Đs: L 2 x 2 x 4 72 3 4 x. 8 3x 4 12) L lim . Đs: L 1 2 x 0 x x Bài 5.
Tính các giới hạn sau: n 1 ax 1 a 1) F lim . Đs: x 0 x n 11 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC n 1 m ax 1 bx a b 2) F lim . Đs: x 0 x n m n 1 ax 1 am 3) F lim (ab 0). Đs: x 0 m 1 bx 1 bn n 1 m ax 1 bx a b 4) F lim . Đs: 2 x 0 1 x 1 n m LỜI GIẢI x 8 3 x 1 x 8 3 x 1 x 8 Bài 1. 1) B lim lim lim x 8 x 8 x 8 3 x 1 3 x 1 3 x 1 9 x 1 x 8 3 x 1 lim lim 3 x 1 6 . x 8 x 8 8 x 2 2 2 4 x x 2 4 x x 2 4 x x 2 2) B lim lim x 1 x 1 2 x 1 x 1 4 x x 2 2 4 x x 4 x x 1 x 1 lim lim lim . x 1 2 x 1 2 x 1 2 4 x 1 4 x x 2 x 1 4 x x 2 4 x x 2 2 2 2 2x 3x x 2x 3 2 3 x x x x x 3) B lim lim x 3 x 3 2 2x 6 2x 6 2x 3x x x x 3 x 1 lim lim . x 3 2 x 3 2 4 2 x 3 2x 3x x 2 2x 3x x x 2 2 x 2 2 x 2 2 4) B lim lim 2 x 2 x 2 2 x 4 x 4 x 2 2 x 2 1 1 lim lim . x 2 x 2 x 2 x 2 2 x 2 x 2 x 2 2 16 2 3x 2 2 3x 2 2 3x 2 4 3x 2 5) B lim lim lim 2 x 2 x 2 2 x 2 2 x 4 x 4 2 3x 2 x 4 2 3x 2 3 2 x 3 3 lim lim . x 2 x 2 x 2 x 2 2 3x 2 x 2 2 3x 2 16 x 3 x 3 x 3 x 9 1 1 6) B lim lim lim lim . 2 x 9 x 9 2 x 9 x 9 12 9x x 9x x x 3 x 9 x x 3 x x 3 54 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC x 2 2 x 2 1 1 7) B lim lim lim . 2 x 2 x 2 2x x 10 x 2 2x 5 x 2 2 x 2 2x 5 x 2 2 36 2 7 2x x 2 7 2x x 2 2 x 2x 3 8) B lim lim lim 2 x 1 x 1 2 x 1 x 1 7 2x 2 x x 1 2 x 1 7 2x 2 x x 1 3 x 3 x 1 lim lim . x 1 x 1 x 1 7 2x 2 x x 1 x 1 7 2x 2 x 3 2 2 2 2x 5 2x x 8 2x 5 2x x 8 9) B lim lim 2 x 1 x 1 2 2 x 3x 2 x 3x 2 2x 5 2x x 8 2 2x 19x 17 x 1 2x 17 lim lim x 1 2 2 x 1 2 x 3x 2 2x 5 2x x 8 x 1 x 2 2x 5 2x x 8 2x 17 5 lim . x 1 2 2 x 2 2x 5 2x x 8 2 x 1 x 8 3 2 x 8 3 3x 1 x 3 Bài 2. 1) B lim lim lim 3 x 1 x 1 x 1 x 8 3 x 1 3x 1 x 3 3x 1 x 3 x 3 2 x 1 4x 5 3x 6 2) B lim lim x 1 4x 5 3x 6 x 1 x 1 x 3 2 4x 5 3x 6 3 lim . x 1 x 3 2 2 x 2 2x 2 x x 1 3 x x 1 3 x 1 3) B lim lim lim . x 2 x 1 3 x x 2 2 x 2 x 2 2x x 2 2 x 2 2x 4 x 1 3x 5 2 3 x 2x 3 x 6 4) B lim lim x 3 2x 3 x 6 x 3 x 3 x 1 3x 5 2 2x 3 x 6 lim 3 . x 3 x 1 3x 5 2 2 x x 2 1 x x x 2 1 x 5) B lim lim 4 x 1 x x x 1 2 2 x x 1 x x 2 x x 2 1 x 2 x 1 x 1 lim lim x 1 2 2 x x 1 x x 2 x x 2 1 x x 1 2 2 x x x 2 x x 2 1 x 0 . 13 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 4 4x 3 1 4 x 1 6) B lim lim x 1 x 1 x 1 3 2 4 4 4 x 1 4x 3 4x 3 4x 3 1 4 lim 1 . x 1 3 2 4 4 4 4x 3 4x 3 4x 3 1 2 2 2 2x 1 2x 5 2x 2x 4 x 1 x 3 7) B lim lim x 2 2 x 1 x 3 x 2 2 2 x x 2 2x 1 2x 5 2 2 x 1 x 3 2 5 lim . x 2 2 2x 1 2x 5 3 x 9 x 16 7 x 9 3 x 9 4 Bài 3. 1) L lim lim x 0 x x 0 x x x x 9 3 x 16 4 1 1 7 lim lim . x 0 x x 0 x 9 3 x 16 4 24 2x 2 5x 4 5 2x 2 2 5x 4 3 2) L lim lim x 1 x 1 x 1 x 2 x 1 5 x 1 2x 2 2 5x 4 3 2 5 4 lim lim . x 1 x 1 x 1 2x 2 2 5x 4 3 3 2 x 6 2x 2 8 2 x 6 6 2x 2 2 3) L lim lim x 3 x 3 x 3 x 3 x 6 9 2x 2 4 x 3 x 3 2 2 2 x 6 3 2x 2 2 x 6 3 2x 2 2 lim lim x 3 x 3 x 3 x 3 2 2 5 lim . x 3 x 6 3 2x 2 2 6 2 2 2 2x x 1 x 8 2x x 1 x 2 x 4 4) L lim lim x 2 x 2 x 2 x 2 2 2 4x 4 x x 2 x 2 x 2 x 2 x 2 x 2 x 2 2 x 1 x 2 x 1 x lim lim x 2 x 2 x 2 x 2 x 2 lim x 2 x 2 8 . x 2 2 x 1 x 5x 4 2x 3 x 84 5x 4 2x 3 3 5x 4 16x 96 14 5) L lim lim x 6 x 6 x 6 x 6 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 2 x 6 5x 4 2x 3 3 16 x 6 5x 4 16 x 6 lim 2x 3 3 lim x 6 x 6 x 6 x 6 10x 8 74 lim 16 . x 6 2x 3 3 3 1 4x 1 6x 1 2 24x 10x 1 1 2 24x 10x 1 1 6) L lim lim lim x 0 x x 0 x x 0 2 x 24x 10x 1 1 x 24x 10 24x 10 lim lim 5 . x 0 2 x 24x 10x 1 1 x 0 2 24x 10x 1 1 4x 3 2x 1 3x 1 2x 1 x 4x 3 2x 1 7) L lim lim 2 2 2 x 1 x 2x 1 x 1 x 1 x 1 2 2 2x 1 x 4x 3 2x 1 lim 2 2 x 1 x 1 2x 1 x x 1 4x 3 2x 1 1 4 5 lim . x 1 2x 1 x 4x 3 2x 1 2 3x 7 4 x 3 2 2x 1 4 x 3 x 7 2 2x 1 2x 8) L lim lim 2 2 x 1 x 2x 1 x 1 x 1 2 2 2 2 16x 48 x 14x 49 4 2x 1 4x x 1 4 x 1 x 7 4 x 3 2x 2 2x 1 x 7 4 x 3 2x 2 2x 1 lim lim 2 x 1 2 x 1 x 1 x 1 1 4 17 lim . x 1 x 7 4 x 3 2x 2 2x 1 16 4x 4 9 6x 5 4x 4 x 2 9 6x x 3 9) L lim lim 2 x 0 x 2 x 0 x 2 2 4x 4 x 4x 4 9 6x x 6x 9 2 2 x x 4x 4 x 2 9 6x x 3 x 2 4x 3 9 6x x 3 lim lim 2 x 0 x 2 x 0 x 1 1 5 lim . x 0 x 2 4x 4 9 6x x 3 12 15 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 2 2 6x 3 2x 5x 2 x 2x 1 6x 3 10) L lim lim 2 2 x 1 x 1 x 1 x 1 2 2 2 6x 3 x 4x 4 2 x 1 2 x 1 2 x 1 6x 3 x 2 6x 3 x 2 lim lim 2 x 1 2 x 1 x 1 x 1 1 11 lim 2 . x 1 6x 3 x 2 6 3 4x 2 4x 8 4 1 Bài 4. 1) L lim lim lim . x 2 x 2 x 2 3 2 3 x 2 16x 2 4x 4 x 2 3 2 3 16x 2 4x 4 3 3 1 1 x 1 1 x 1 1 2) L lim lim lim . x 0 x x 0 2 3 x 0 2 3 x 1 1 x 1 x 3 3 1 1 x 1 x 3 3 2 x 1 2 2 x 9 3) L lim lim x 3 x 3 x 3 2 2 3 2 3 x 3 x 1 2 x 1 4 x 3 1 lim . x 3 2 2 3 2 2 3 x 1 2 x 1 4 3 x 7 2 x 1 4) L lim lim x 1 x 1 x 1 x 1 2 3 3 . x 7 2 x 7 4 x 1 x 1 1 lim . x 1 2 3 3 x 7 2 x 7 4 6 x 8 3 x 2 3 2 3 2x 9 5 5 5) x 2 x 4 L lim lim lim . x 8 2x 9 5 x 8 2x 16 x 8 3 2 3 2 x 2 x 4 12 2x 9 5 x 1 2 3 3 3 x 1 3 2 3 x 2 x 2 1 6) x x 1 L lim lim lim 1 . 3 x 1 x 2 1 x 1 x 1 x 1 3 2 3 x x 1 2 3 3 x 2 x 2 1 3 3 3 3 10 2x x 1 10 2x 2 x 1 7) L lim lim 2 x 1 x 3x 2 x 1 x 1 x 2 16 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 3 2x 2 x 1 2 3 3 3 3 10 2x 2 10 2x 4 lim x 1 x 1 x 2 2 2 x 1 x x 1 x 1 2 3 3 3 3 10 2x 2 10 2x 4 lim x 1 x 1 x 2 2 2 x x 1 1 2 3 3 3 3 10 2x 2 10 2x 4 3 lim . x 1 x 2 2 3 8x 11 x 7 3 8x 11 3 x 7 3 8) L lim lim lim 2 x 2 x 3x 2 2 x 2 x 3x 2 2 x 2 x 3x 2 8x 11 27 x 7 9 lim lim x 2 2 x 2 3 3 x 1 x 2 8x 11 3 8x 11 9 x 1 x 2 x 7 3 8 1 8 1 7 lim lim . x 2 2 x 2 3 3 x 1 8x 11 3 8x 11 9 x 1 x 7 3 27 7 54 3 3 2 3 3 2 x 7 x 3 x 7 2 x 3 2 9) L lim lim lim x 1 x 1 x 1 x 1 x 1 x 1 3 2 x 7 8 x 3 4 lim lim x 1 2 x 1 2 3 3 3 3 x 1 x 3 2 x 1 x 7 2 x 7 4 3 2 x 1 x 1 lim lim x 1 2 x 1 2 3 3 3 3 x 1 x 3 2 x 1 x 7 2 x 7 4 2 x x 1 x 1 1 1 1 lim lim . x 1 2 x 1 2 3 3 3 4 2 4 3 x 3 2 x 7 2 x 7 4 3 3 2 1 x 8 x 2 1 x 2 8 x 2 10) L lim lim lim x 0 x 0 x 0 x x x 4 1 x 4 8 x 8 lim lim x 0 x 0 2 x 2 1 x 2 3 3 x 8 x 2 8 x 4 4 1 1 11 lim lim 1 . x 0 x 0 2 2 1 x 2 3 3 12 12 8 x 2 8 x 4 17 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
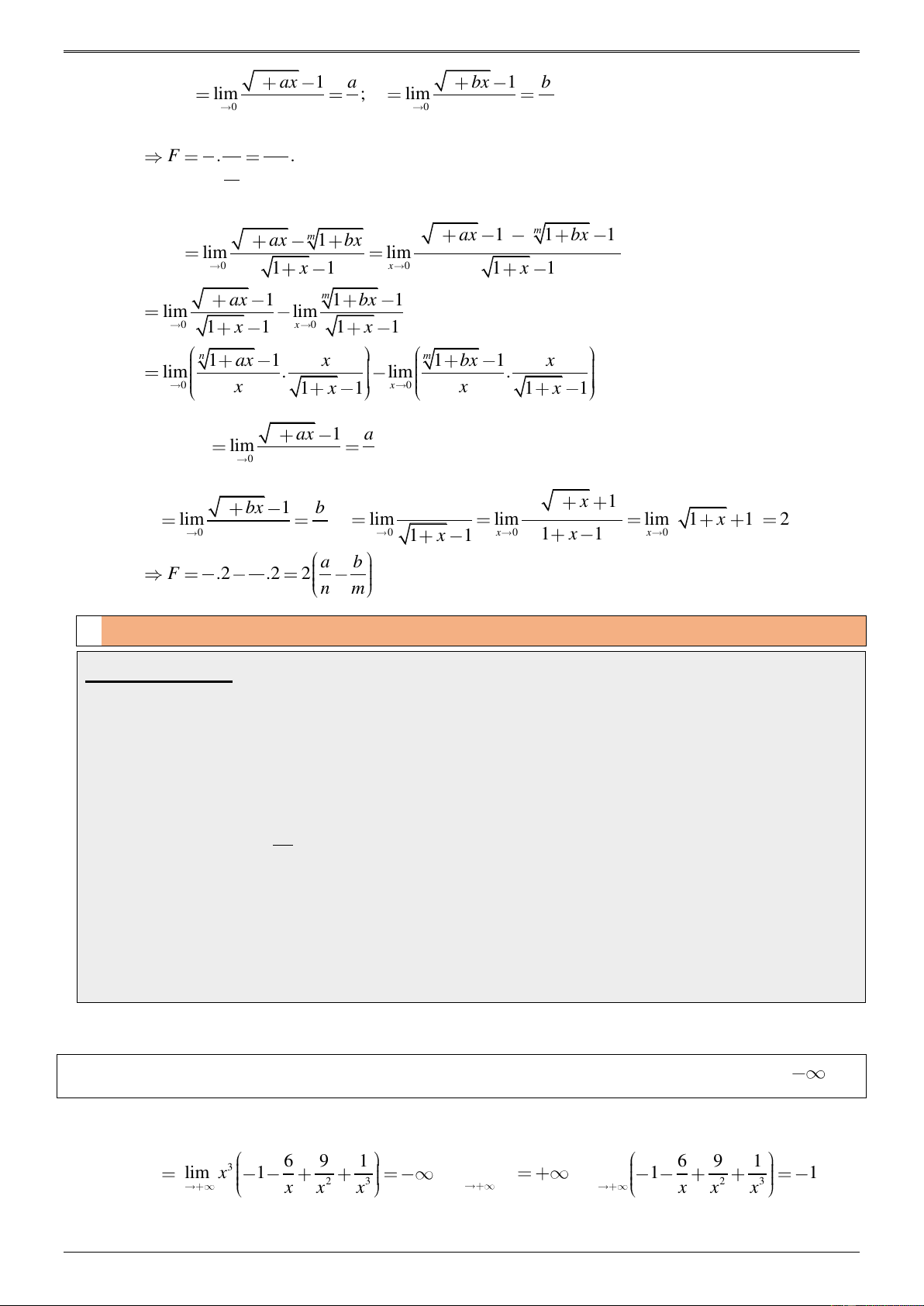
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 3 2 3 2 2x 4x 11 x 7 2x 4x 11 3 x 7 3 11) L lim lim lim 2 2 2 x 2 x 2 x 2 x 4 x 4 x 4 3 2 2x 4x 11 27 x 7 9 lim lim x 2 2 x 2 2 2 2 3 2 3 x 4 x 7 3 x 4 2x 4x 11 3 2x 4x 11 9 2 x 2 x 4 x 2 lim lim x 2 2 x 2 2 2 2 3 2 3 x 4 x 7 3 x 4 2x 4x 11 3 2x 4x 11 9 2 x 4 1 lim lim x 2 2 x 2 2 3 2 3 x 2 x 7 3 x 2 2x 4x 11 3 2x 4x 11 9 1 1 5 9 24 72 3 3 4 x. 8 3x 2 2 4 x 4 4 x. 8 3x 4 12) L lim lim 2 2 x 0 x 0 x x x x 3 4 x. 8 3x 2 2 4 x 4 lim lim 2 2 x 0 x 0 x x x x 4 x. 8 3x 8 2 4 x 4 lim lim x 0 2 x 0 3 3 x x 1 4 x 2 x x 1 8 3x 2 8 3x 4) 4 x.3 2 lim lim x 0 2 x 0 3 3 x 1 4 x 2 x 1 8 3x 2 8 3x 4 1 1 1. 2 2 n 1 ax 1 1 ax 1 Bài 5. 1) F lim lim x 0 x 0 n 1 n 2 x n x 1 n ax 1 ax ... n 1 ax 1 a a lim . x 0 n 1 n 2 n 1 n 1 ... n 1 1 n ax ax ax n 1 ax 1 m n m 1 bx 1 1 ax 1 bx 2) F lim lim x 0 x 0 x x n 1 ax 1 m 1 bx 1 a b lim lim . x 0 x 0 x x n m n 1 ax 1 n 1 ax 1 1 3) F lim lim . x 0 m x 0 1 bx 1 m x 1 bx 1 x 18 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC n 1 ax 1 a m 1 bx 1 b Xét A lim ; B lim x 0 x n x 0 x m a 1 am F . . n b bn m n 1 ax 1 m n m 1 bx 1 1 ax 1 bx 4) F lim lim x 0 x 0 1 x 1 1 x 1 n 1 ax 1 m 1 bx 1 lim lim x 0 x 0 1 x 1 1 x 1 n 1 ax 1 m x 1 bx 1 x lim . lim . x 0 x 0 x 1 x 1 x 1 x 1 n 1 ax 1 a Ta có A lim x 0 x n m 1 bx 1 b x 1 x 1 x B lim C lim lim lim 1 x 1 2 x 0 x m x 0 x 0 x 0 1 x 1 1 x 1 a b a b F .2 .2 2 . n m n m
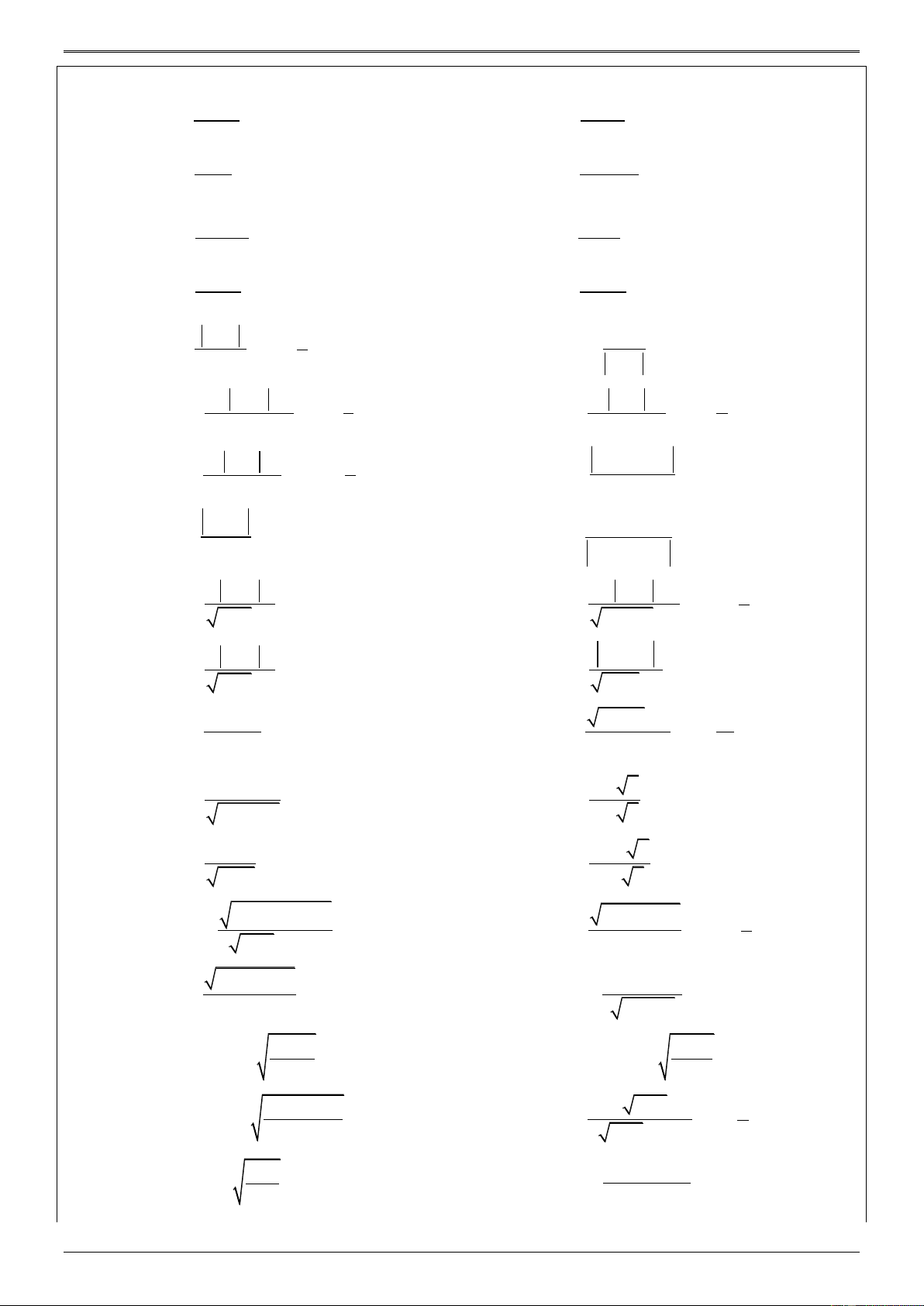
Dạng 3. Giới hạn của hàm số khi x → .
Phương pháp giải:
- Đối với dạng đa thức không căn, ta rút bậc cao và áp dụng công thức khi x → + 1. lim k x = + x→+
 + khi k = 2l 2. lim k x =  x→−
− khi k = 2l +1 c 3. lim = 0 (c hằng số) k x→+ x
- Đối với dạng phân số không căn, ta làm tương tự như giới hạn dãy số, tức rút bậc cao nhất của tử
và mẫu, sau đó áp dụng công thức trên.
- Ngoài việc đưa ra khỏi căn bậc chẵn cần có trị tuyệt đối, học sinh cần phân biệt khi nào đưa ra ngoài
căn, khi nào liên hợp. Phương pháp suy luận cũng tương tự như giới hạn của dãy số, nhưng cần phân
biệt khi x → + hoặc x → −  VÍ DỤ
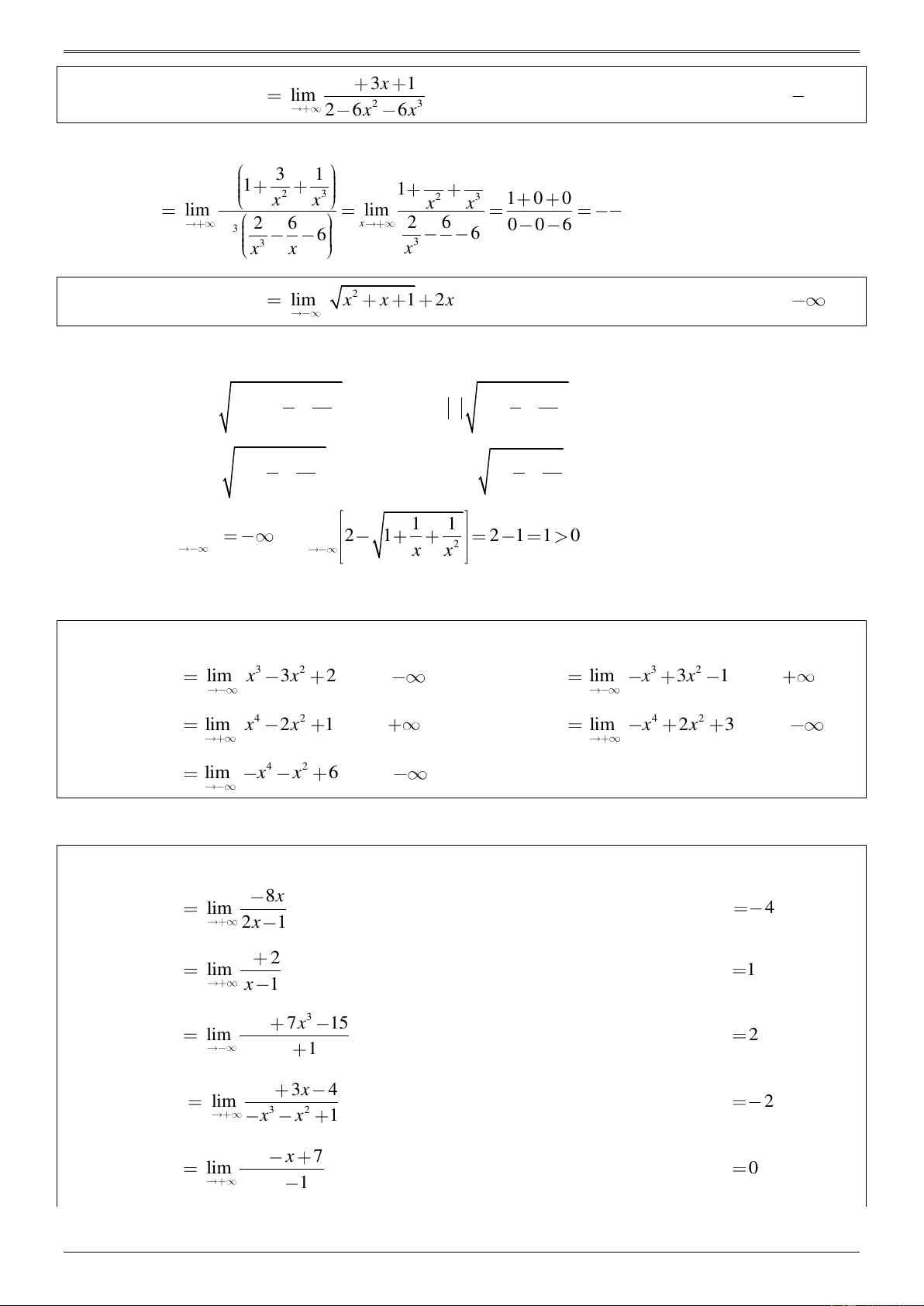
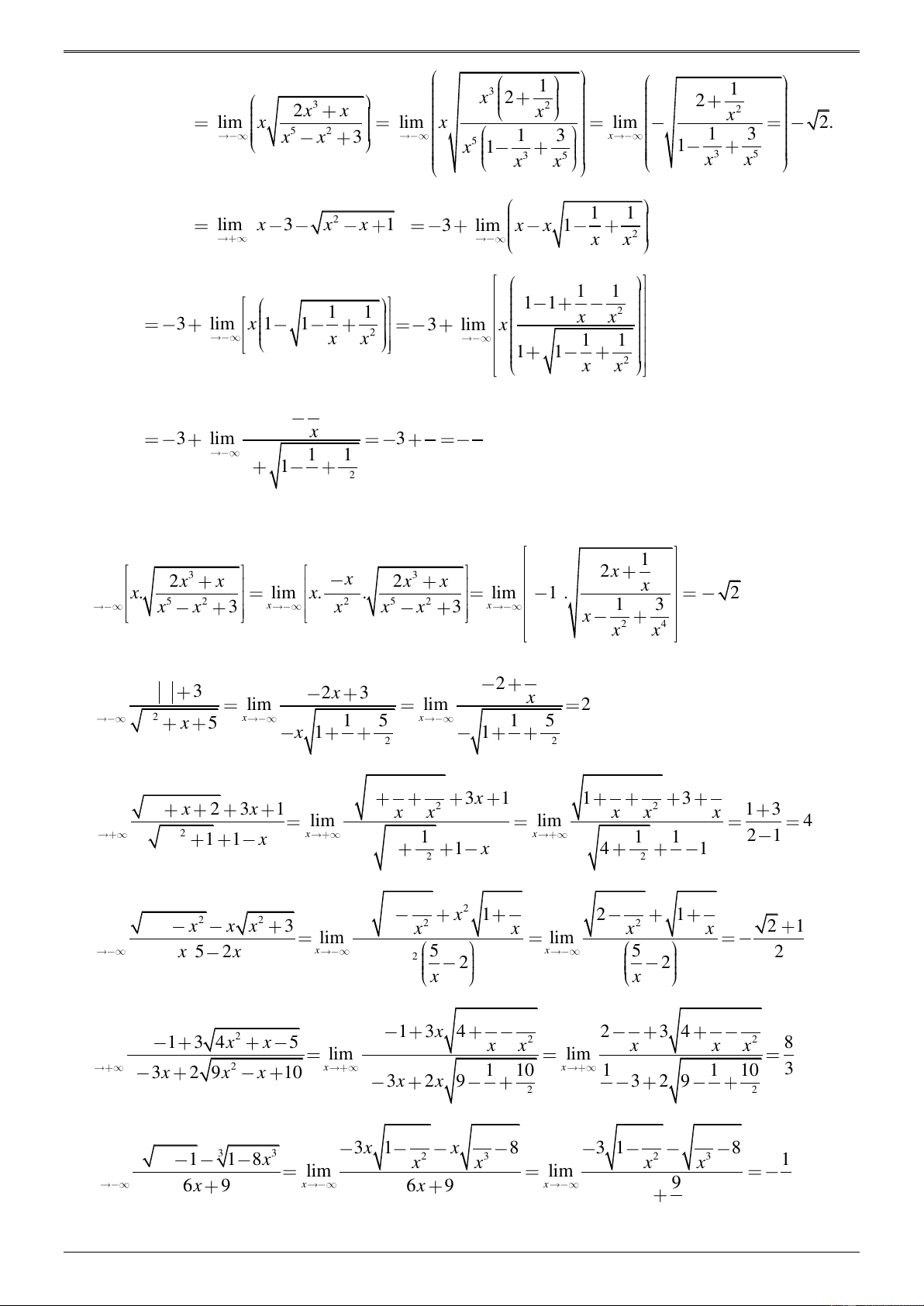
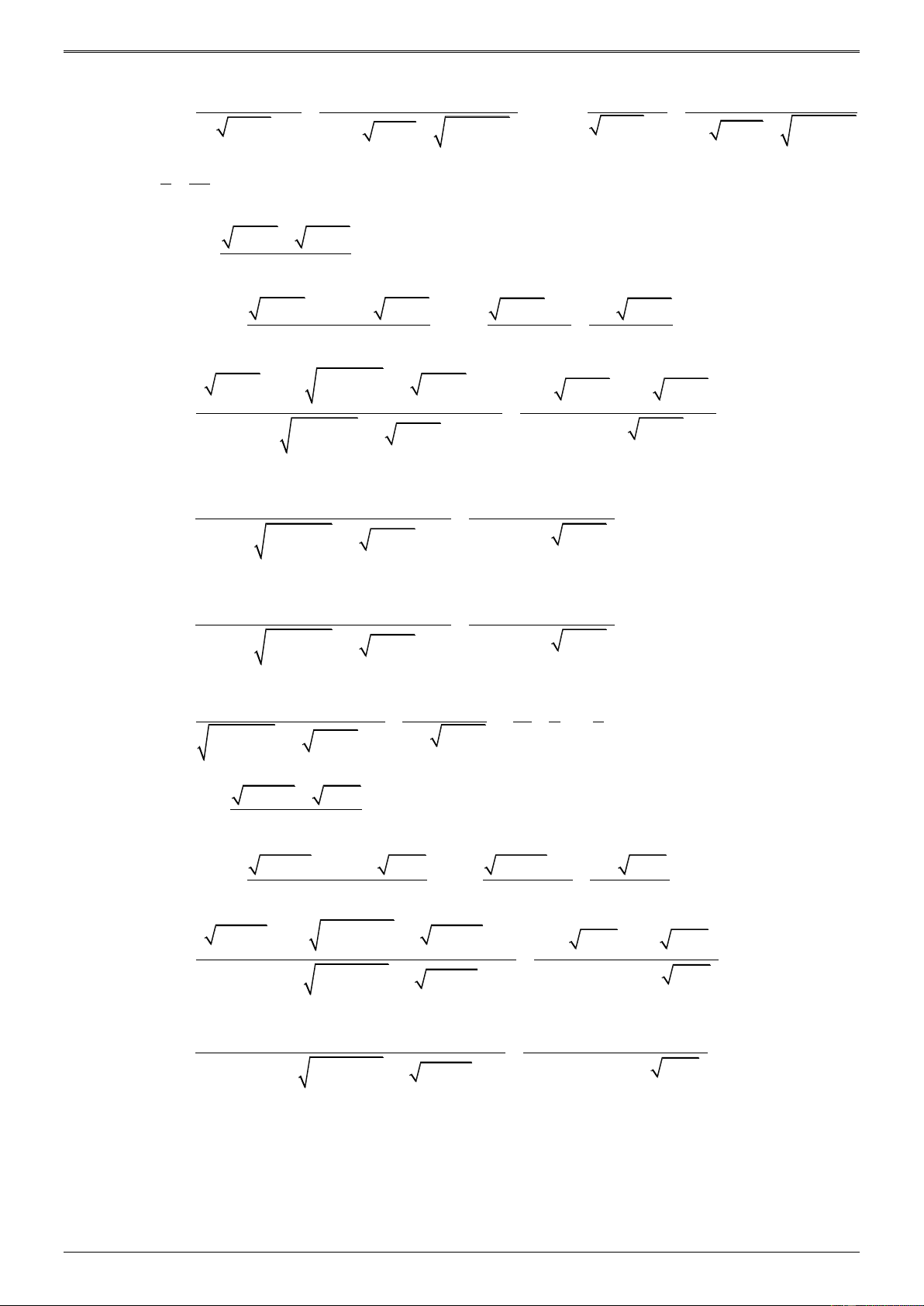
Ví dụ 1. Tính giới hạn A = ( 3 2
lim −x − 6x + 9x + ) 1 . Đs: . x→+ Lời giải 6 9 1 6 9 1 3 A lim x 1 (vì 3 lim x và lim 1 1 ). 2 3 x x x x x 2 3 x x x x 19 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 3 x 3x 1 1
Ví dụ 2. Tính giới hạn B lim . Đs: . 2 3 x 2 6x 6x 6 Lời giải 3 1 3 3 1 x 1 2 3 1 2 3 x x 1 0 0 1 lim lim x x B . x 2 6 x 2 6 3 0 0 6 6 x 6 6 3 3 x x x x
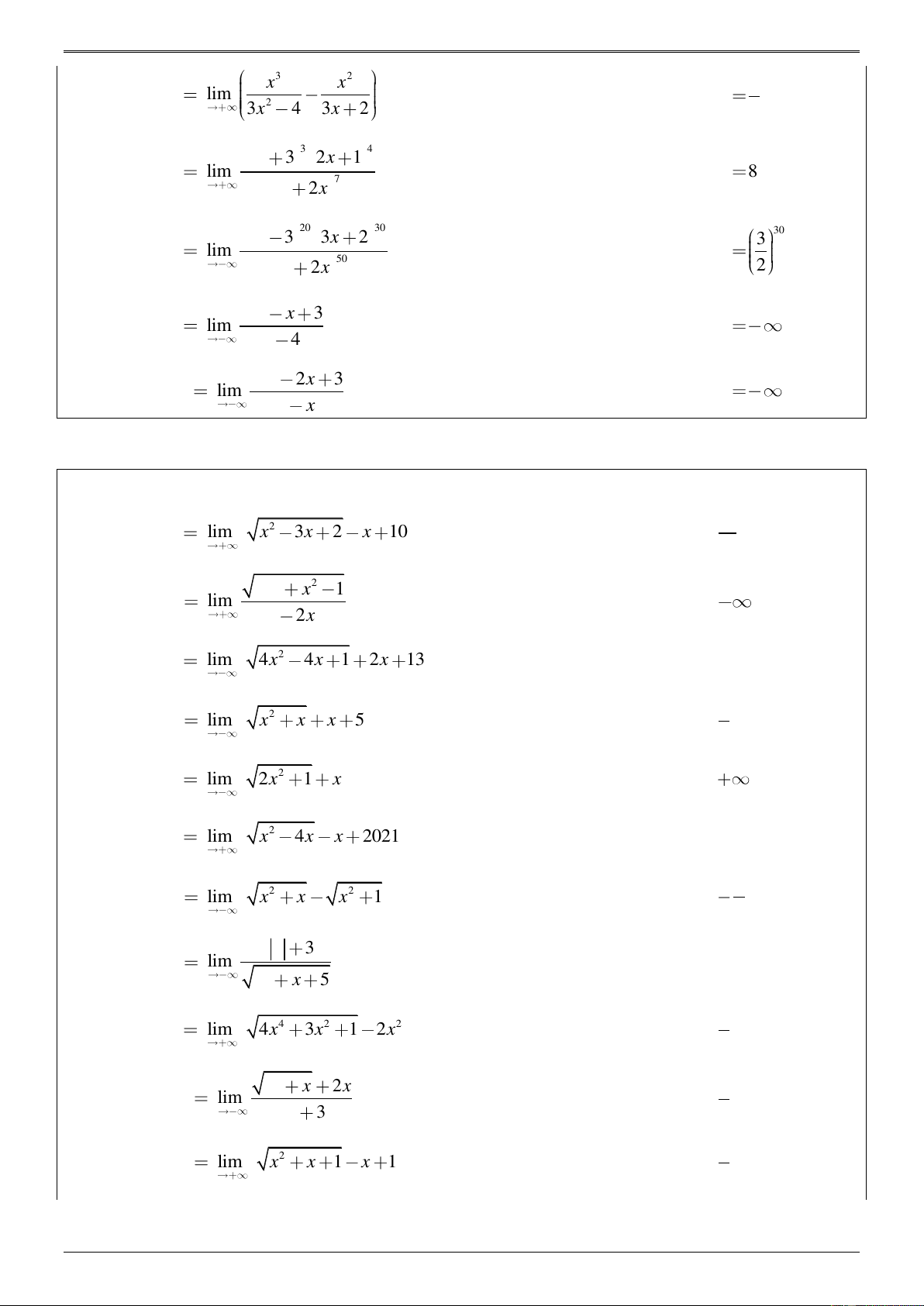
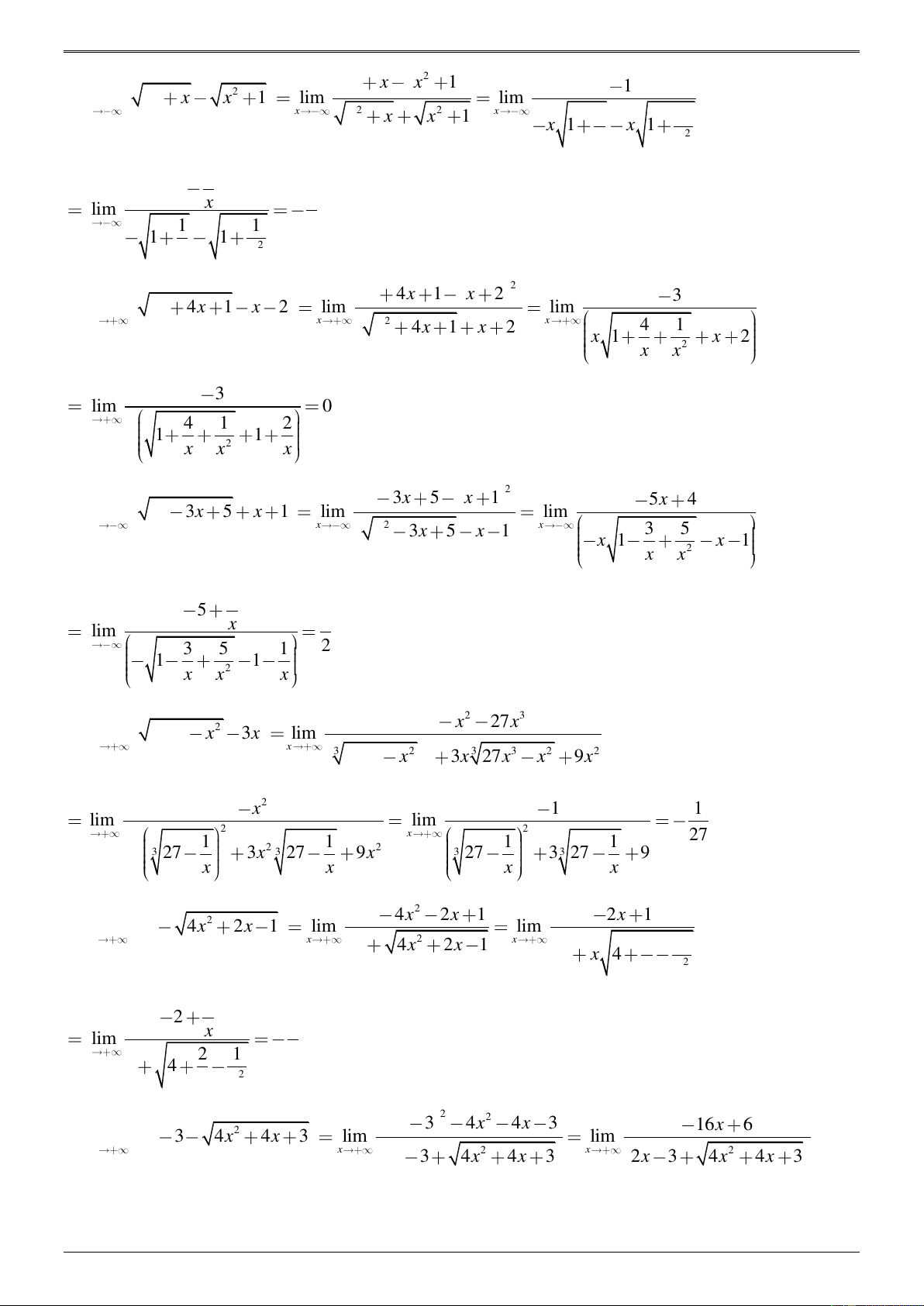
Ví dụ 2. Tính giới hạn 2 C lim x x 1 2x . Đs: . x Lời giải  1 1   1 1      2
C = lim  x 1+ + + 2x    = lim  x 1+ + + 2x    2 2 x→− x   x x →−      x x      1 1     1 1  = lim −x 1+ + + 2x  
 = lim x2 − 1+ +  = − 2  2  x→− x   x x →−     x x    1 1 (Vì lim x và lim 2 1 2 1 1 0 ). x 2 x x x
BÀI TẬP ÁP DỤNG Bài 1. Tính các giới hạn sau: 1) 3 2 A lim x 3x 2 . Đs: . 2) 3 2 A lim x 3x 1 . Đs: . x x 3) 4 2 A lim x 2x 1 . Đs: . 4) 4 2 A lim x 2x 3 . Đs: . x x 5) 4 2 A lim x x 6 . Đs: . x Bài 2. Tính các giới hạn sau: 1 8x 1) B lim . Đs: B 4 . x 2x 1 x 2 2) B lim . Đs: B 1. x x 1 4 3 2x 7x 15 3) B lim . Đs: B 2 . 4 x x 1 3 2x 3x 4 4) B lim . Đs: B 2 . 3 2 x x x 1 2 3x x 7 5) B lim . Đs: B 0 . 3 x 2x 1 20 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 3 2 x x 2 6) B lim . Đs: B . 2 x 3x 4 3x 2 9 3 4 4x 3 2x 1 7) B lim . Đs: B 8 . 7 x 2 2x 20 30 2x 3 3x 2 30 3 8) B lim . Đs: B . 50 x 1 2x 2 2 3x x 3 9) B lim . Đs: B . x x 4 3 2x 2x 3 10) B lim . Đs: B . x 5 x Bài 3. Tính các giới hạn sau: 17 1) 2 C lim x 3x 2 x 10 . Đs: . x 2 4 2 2x x 1 2) C lim . Đs: . x 1 2x 3) 2 C lim 4x 4x 1 2x 13 . Đs: 14. x 9 4) 2 C lim x x x 5 . Đs: . x 2 5) 2 C lim 2x 1 x . Đs: . x 6) 2 C lim x 4x x 2021 . Đs: 2019 . x 1 7) 2 2 C lim x x x 1 . Đs: . x 2 2 x 3 8) C lim . Đs: -2. x 2 x x 5 3 9) 4 2 2 C lim 4x 3x 1 2x . Đs: . x 4 2 x x 2x 1 10) C lim . Đs: . x 2x 3 2 3 11) 2 C lim x x 1 x 1 . Đs: . x 2 21 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 2 x x x 12) C lim . Đs: 2 . x x 10 1 13) 2 2 C lim 4x 9x 21 4x 7x 13 . Đs: x 2 2 2 4x x 3x 7x 1 14) C lim . Đs: 1. 2 x 2 2x 1 . x 3x 15) 2 C lim 4x 4x 1 2x 3 . Đs: 4 x 3 x 16) C lim x 1 . Đs: -1 3 x 5 3x x 43 17) 2 C lim 16x 3x 4x 5 . Đs: x 8 3 2x x 18) C lim x . Đs: 2 5 2 x x x 3 5 19) 2 C lim x 3 x x 1 . Đs: x 2 Bài 4. Tính các giới hạn sau: 3 2x x 1) lim . x . Đs: 2 . 5 2 x x x 3 2 x 3 2) lim . Đs: 2. x 2 x x 5 2 x x 2 3x 1 3) lim . Đs: 4. x 2 4x 1 1 x 4 2 2 2x x x x 3 2 1 4) lim Đs: . x x 5 2x 2 2 2x 1 3 4x x 5 8 5) lim . Đs: . x 2 1 3x 2 9x x 10 3 2 3 3 3 x 1 1 8x 1 6) lim . Đs: . x 6x 9 6 2 2x 1 x 3 2 7) lim . Đs: . 2 x x 5x 5 22 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 2 x 4x 3x 1 1 8) lim . Đs: x 2 9x x 3 5x 3 4 2x 1 9) lim . Đs: 1. x 2 2 x x x x 8x 3 10) lim . Đs: 2. x 2 6x 4x x 3 2 x 1 7x 2 11) lim . Đs: 1. x 2 x 3x 2 5x 3 x 2 1 2x 12) lim . Đs: 1. x 1 x Bài 5. Tính các giới hạn sau: 2 1) lim x x x . Đs: . x 2 2) lim x 4x x . Đs: 2 . x 3) lim x 2 x 2 . Đs: 0. x 1 2 2 4) lim x x x 1 . Đs: . x 2 2 5) lim x 4x 1 x 2 . Đs: 0. x 5 2 6) lim x 3x 5 x 1 . Đs: . x 2 1 3 3 2 7) lim 27x x 3x . Đs: . x 27 1 2 8) lim 2x 4x 2x 1 . Đs: . x 2 2 9) lim 2x 3 4x 4x 3 . Đs: 4 . x 3 4 2 2 10) lim 4x 3x 1 2x . Đs: . x 4 19 2 11) lim 4x 3x 1 2x 4 . Đs: . x 4 2 12) lim 4x 4x 1 2x 3 . Đs: 4 . x 23 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 3 2 3 x 4x x 16 13) lim . Đs: . x 2 2x 4x 3x 9 3 3 14) lim 8x 1 2x 1 . Đs: 1. x LỜI GIẢI 3 2 3 2 Bài 1. 1) 3 A lim x 1 , (vì 3 lim x và lim 1 1 0 ). 3 x x x x 3 x x x 3 1 3 1 2) 3 3 A lim x 1 , ì v lim x à v lim 1 1 0 . 3 3 x x x x x x x 2 1 2 1 3) 4 4 A lim x 1 , ì v lim x à v lim 1 1 0 . 2 4 2 4 x x x x x x x 2 3 2 3 4) 4 4 A lim x 1 , ì v lim x à v lim 1 1 0 . 2 4 2 4 x x x x x x x 1 6 1 6 5) 4 4 A lim x 1 , ì v lim x à v lim 1 1 0 . 2 4 2 4 x x x x x x x 1 1 x 8 8 1 8x x 0 8 Bài 2. 1) lim lim lim x B 4 . x 2x 1 x 1 x 1 2 0 x 2 2 x x 2 2 x 1 1 x 2 x 1 0 2) lim lim lim x B 1. x x 1 x 1 x 1 1 0 x 1 1 x x 7 15 4 7 15 x 2 4 3 4 2 4 2x 7x 15 x x 2 0 0 3) lim lim lim x x B 2 . 4 x x 1 x 1 x 1 4 1 0 x 1 1 4 4 x x 3 4 3 4 3 x 2 2 3 2 3 2 3 2x 3x 4 x x x x 2 0 0 4) B lim lim lim 2 . 3 2 x x x 1 x 1 1 x 1 1 3 1 0 0 x 1 1 3 3 x x x x 3 1 7 3 1 7 3 x 2 3x x 7 2 3 2 3 x x x x x x 0 0 0 5) B lim lim lim 0 . 3 x 2x 1 x 1 x 1 3 2 0 x 2 2 3 3 x x 3 2 x x 2 x 2x 4 6) B lim lim 24 2 x 3x 4 3x 2 2 x 3x 4 3x 2 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 4 4 3 x 2 2 x x 2 0 2 lim lim x 4 2 x 4 2 3 3 0 3 0 9 x 3 3 3 3 2 2 x x x x 3 4 3 1 3 4 4 2 3 4 4x 3 2x 1 x x 4 0 2 0 7) B lim lim 8 . 7 x 7 7 2 2x x 3 2 0 2 x 20 30 3 2 20 30 2 3 20 30 2x 3 3x 2 30 x x 2 0 3 0 3 8) B lim lim . 50 x 50 50 1 2x x 1 2 0 2 2 x 1 3 2 1 3 x 3 2 3 3x x 3 2 2 x x 9) B lim lim lim . x x x , x x 4 x 4 x 4 x 1 1 x x 1 3 3 2 ì lim à lim x x v x v 3 . x x 4 1 x 2 3 3 2 3 x 2 3 2 2x 2x 3 2 3 2 3 x x 10) B lim 2 lim lim . x x x , x 5 x x 5 x 5 x 1 1 x x 2 3 2 2 3 2 ì lim à lim x x v x v 2 . x x 5 1 x Bài 3. 1) 2 C lim x 3x 2 x 10 2 10 lim x 3x 2 x x x 3x 2 3x 2 10 lim 10 lim x 2 x 3x 2 x x 2 x 3x 2 x 2 3 3 17 10 lim x 10 . x 3 2 2 2 1 1 2 x x 25 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 1 1 1 1 2 4 2 x 2 2 2x x 1 2 4 2 4 2) x x x x C lim lim lim x , x 1 2x x 1 x 1 x 2 2 x x 1 1 2 2 4 x x 2 ì v lim x à v lim 0 x x 1 2 2 x 3) 2 C lim 4x 4x 1 2x 13 2 13 lim 4x 4x 1 2x x x 2 2 4x 4x 1 4x 4x 1 13 lim 13 lim x 2 4x 4x 1 2x x 4 1 2 x 4 2x 2 x x 1 1 x 4 x 4 x x 13 lim 13 lim x 4 1 x 4 1 x 4 2x x 4 2x 2 x x 2 x x 1 4 13 lim x 14 x 4 1 4 2 2 x x 1 1 4) 2 C lim x x x 5 5 lim x 1 x 5 lim x 1 1 x x x x x 1 1 1 1 9 5 lim x x 5 lim . x 1 x 1 2 1 1 1 1 x x 1 1 5) 2 C lim 2x 1 x lim x 2 x lim x 1 2 , x 2 2 x x x x 1 ì v lim 1 2 1 2 0 à v lim x . 2 x x x 4 4 6) 2 C lim x 4x x 2021 2021 lim x 1 x 2021 lim x 1 1 x x x x x 4 1 1 4 4 x 2021 lim x 2021 lim 2021 2019 . x 4 x 4 2 1 1 1 1 26 x x Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC x 1 7) 2 2 C lim x x x 1 lim x x 2 2 x x x 1 x 1 x 1 lim lim x 1 1 x 1 1 x 1 x 1 x 1 x 1 2 2 x x x x 1 1 1 lim x x 1 1 2 1 1 2 x x 2 x 3 2 x 3 2 x 3 2 0 8) C lim lim lim x x 2 . x 2 x x 5 x 1 5 x 1 5 1 0 0 x 1 1 2 2 x x x x 3 1 9) 4 2 2 C lim 4x 3x 1 2x 2 2 lim x 4 2x x 2 4 x x x 3 1 1 4 4 3 2 4 2 3 2 lim x x lim x x . x 3 1 x 3 1 4 4 2 4 2 2 4 2 4 x x x x 1 2 x 1 2x x x 2x 10) C lim x lim x 2x 3 x 2x 3 1 1 x 1 2 1 2 x x 1 2 1 lim lim . x 3 x 3 2 2 x 2 2 x x 1 1 11) 2 C lim x x 1 x 1 1 lim x 1 x x 2 x x x 1 1 1 1 1 1 2 1 lim x 1 1 1 lim x x x 2 x x x x 1 1 1 1 2 x x 1 1 1 3 1 lim x 1 . x 1 1 2 2 1 1 2 x x 27 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 1 x 1 2 x x 1 1 x x x x x x 1 1 12) C lim lim lim 2 . x x 10 x x 10 x 10 1 1 x 9 21 7 13 13) 2 2 C lim 4x 9x 21 4x 7x 13 lim x 4 x 4 x 2 2 x x x x x 9 21 7 13 34 4 4 2 2 2 2 1 lim x x x x x lim x . x 9 21 7 13 x 9 21 7 13 2 2 2 4 4 4 4 2 2 x x x x 2 2 x x x x 2 2 4x x 3x 7x 1 14) C lim 2 x 2 2x 1 . x 3x 4 x 3 7 1 4 x 3 7 1 3 x 2 3 2 3 x x x x x x x x 4 lim lim 1 2 2 2 x 1 3 x 1 3 2 .1 2 x 2 . x 1 2 . 1 x x x x 4 1 15) 2 C lim 4x 4x 1 2x 3 3 lim x 4 2x x 2 x x x 4 1 1 4 4 4 2 4 3 lim x x x 3 lim x 3 4 . x 4 1 x 4 1 4 4 2 4 2 2 x x 2 x x 3 x 16) C lim x 1 3 x 5 3x x 3 3 1 1 1 1 0 1 lim 1 x lim 1 x x 1. 1. x x 5 3 x x 5 3 2 0 0 1 x 1 1 3 2 2 x x x x 17) 2 C lim 16x 3x 4x 5 x 3 3 5 lim x 16 4x 5 lim x 16 4 x x x x 3 16 16 3 3 3 43 5 lim x x 5 lim 5 5 . x x 3 3 4 4 8 8 16 4 16 4 x x 28 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 1 3 1 x 2 3 2 2x x 2 2 x 18) C lim x lim lim x x 2. 5 2 x x x 3 x 1 3 x 1 3 5 x 1 1 3 5 3 5 x x x x 1 1 19) 2 C lim x 3 x x 1 3 lim x x 1 x 2 x x x 1 1 1 1 1 1 2 3 lim x 1 1 3 lim x x x 2 x x x x 1 1 1 1 2 x x 1 1 1 5 3 lim x 3 . x 1 1 2 2 1 1 2 x x Bài 4. 1 3 3 2x 2x x x 2x x 1) lim . lim . . lim 1 . x x x 2 . 5 2 2 5 2 x x x 3 x x x x 3 x 1 3 x 2 4 x x 3 2 2 x 3 2x 3 2) lim lim lim x 2 . x 2 x x 5 x 1 5 x 1 5 x 1 1 2 2 x x x x 1 2 1 2 1 2 x 1 3x 1 1 3 2 2 x x 2 3x 1 x x x x x 1 3 3) lim lim lim 4 . x 2 4x 1 1 x x 1 x 1 1 2 1 x 4 1 x 4 1 2 2 x x x 1 3 1 3 2 2 4 2 2 x 2 x 1 2 1 2 2 2x x x x 3 x x x x 2 1 4) lim lim lim . x x 5 2 x x 5 x 5 2 2 x 2 2 x x 1 5 1 1 5 2 2x 1 3x 4 2 3 4 2 2 2x 1 3 4x x 5 x x x x x 8 5) lim lim lim . x 2 1 3x 2 9x x 10 x 1 10 x 1 1 10 3 1 3x 2x 9 3 2 9 2 2 x x x x x 1 1 1 1 2 3 3 3x 1 x 8 3 1 8 2 3 2 3 3 x 1 1 8x x x x x 1 6) lim lim lim . x 6x 9 x 6x 9 x 9 6 6 29 x Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 3 1 3 2x 1 x 1 2 1 1 2 2x 1 x 3 x x x 2 7) lim lim lim . 2 2 x x 5 x x x 5 x x 1 5 5 x 3 1 3 1 2 x x 4 1 4 2 2 x 4x 3x 1 x x x x 1 8) lim lim lim . x 2 9x x 3 5x 3 x 1 3 x 1 3 3 4 x 9 5x 3 9 5 2 2 x x x x x 1 2 2x 1 2x 1 2 9) lim lim lim x 1 . x 2 2 x x x x x 1 1 x 1 1 2 x 1 x 1 1 1 x x x x 3 8 8x 3 8x 3 10) lim lim lim x 2 . x 2 6x 4x x 3 x 1 3 x 1 3 6x x 4 6 4 2 2 x x x x 1 1 2 2 x 1 7x 2 1 7 2 2 x 1 7x 2 x x x 11) lim lim lim 1. x 2 x 3x 2 5x 3 x 3 2 x 3 2 3 x 1 5x 3 x 1 5 2 2 x x x x x 1 2 1 2 x 2x 1 2 2 2 x 2 1 2x x x x x 12) lim lim lim 1. x 1 x x 1 x x 1 1 x Bài 5. 1 1 2 1) lim x x x lim x 1 x lim x 1 1 . x x x x x 1 ì V lim x à v lim 1 1 2. x x x 2 2 x 4x x 4x 2 2) lim x 4x x lim lim 2 . x x 2 x 4 x x x 4 x 1 1 x x 2 x 2 4 3) lim x 2 x 2 lim lim 0 . x x x 2 x 2 x 2 2 x 1 1 x x 4 1 1 ì V lim 0 à v lim . x x x 2 2 2 1 1 30 x x Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 2 2 x x x 1 x 1 2 2 4) lim x x x 1 lim lim x x 2 2 x x x 1 x 1 1 x 1 x 1 2 x x 1 1 1 lim x . x 1 1 2 1 1 2 x x 2 2 x 4x 1 x 2 3 2 5) lim x 4x 1 x 2 lim lim x x 2 x 4x 1 x 2 x 4 1 x 1 x 2 2 x x 3 lim 0 . x 4 1 2 x 1 1 2 x x x 2 2 x 3x 5 x 1 5x 4 2 6) lim x 3x 5 x 1 lim lim x x 2 x 3x 5 x 1 x 3 5 x 1 x 1 2 x x 4 5 5 lim x . x 3 5 1 2 1 1 2 x x x 3 2 3 27x x 27x 3 3 2 7) lim 27x x 3x lim 2 x x 3 3 2 3 3 2 2 27x x 3x 27x x 9x 2 x 1 1 lim lim . 2 2 x x 27 1 1 1 1 2 2 2 3 3 3 3 x 27 3x 27 9x 27 3 27 9 x x x x 2 2 4x 4x 2x 1 2x 1 2 8) lim 2x 4x 2x 1 lim lim x x 2 2x 4x 2x 1 x 2 1 2x x 4 2 x x 1 2 1 lim x . x 2 1 2 2 4 2 x x 2 2 2x 3 4x 4x 3 16x 6 2 9) lim 2x 3 4x 4x 3 lim lim x x 2 x 2 2x 3 4x 4x 3 2x 3 4x 4x 3 31 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 6 16 16x 6 lim lim x 4 x 4 3 x 3 4 3 2x 3 x 4 2 4 2 2 x x x x x 4 2 4 2 4x 3x 1 4x 3x 1 4 2 2 10) lim 4x 3x 1 2x lim lim x x 4 2 2 4x 3x 1 2 x x 3 1 2 2 x 4 2x 2 4 x x 1 3 2 3 lim x . x 3 1 4 4 2 2 4 x x 2 2 4x 3x 1 2x 4 19x 15 2 11) lim 4x 3x 1 2x 4 lim lim x x 2 4x 3x 1 2x 4 x 3 1 x 4 2x 4 2 x x 15 19 19 lim x . x 3 1 4 4 4 2 2 x x x 2 2 4x 4x 1 2x 3 16x 8 2 12) lim 4x 4x 1 2x 3 lim lim x x 2 4x 4x 1 2x 3 x 4 1 x 4 2x 3 2 x x 8 16 lim x 4 . x 4 1 3 4 2 2 x x x 3 2 3 3 2 3 2 x 4x x x 4x x 2x 4x 3x 13) lim lim . 2 2 2 x 2 x 4x 4x 3x 2 3 2 3 3 2 3 2x 4x 3x x x 4x x 4x x 3 2 4 4 x 16 lim . . 2 x 3 9 4 4 3 3 1 1 1 x x 3 3 8x 1 2x 1 14) 3 3 lim 8x 1 2x 1 lim 2 x x 2 3 3 3 3 8x 1 2x 1 8x 1 2x 1 32 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 6 2 2 12 12x 6x 2 2 lim lim x x 1. 2 2 x 2 x 2 3 3 3 3 8x 1 2x 1 8x 1 2x 1 1 1 1 1 3 3 8 2 8 2 3 3 x x x x
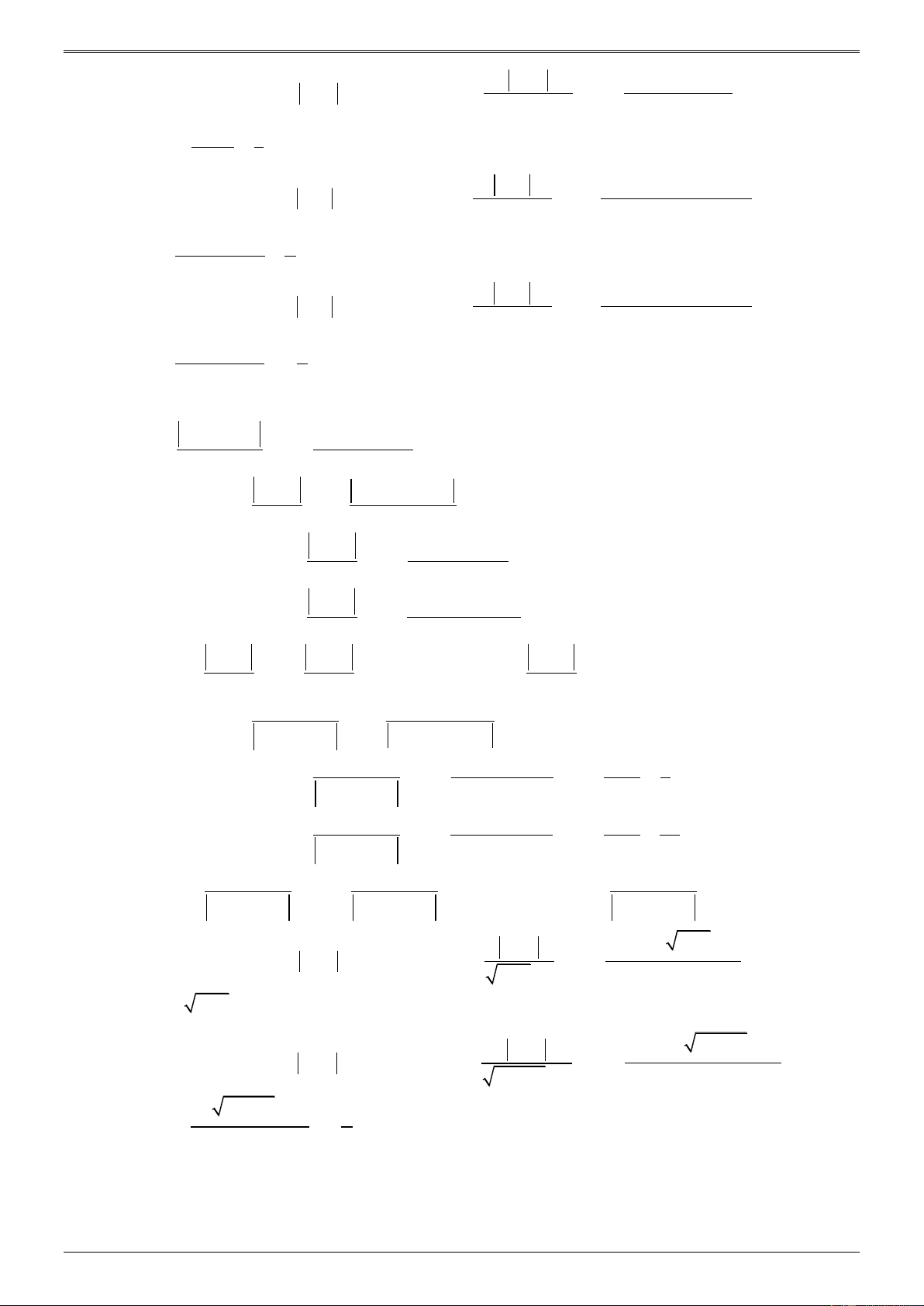
Dạng 4. Giới hạn một bên x x+ → hoặc x x− → . 0 0
Phương pháp giải:
- Sử dụng các định lý về giới hạn hàm số
Chú ý: x → x+  x x x − x  0 0 0 0
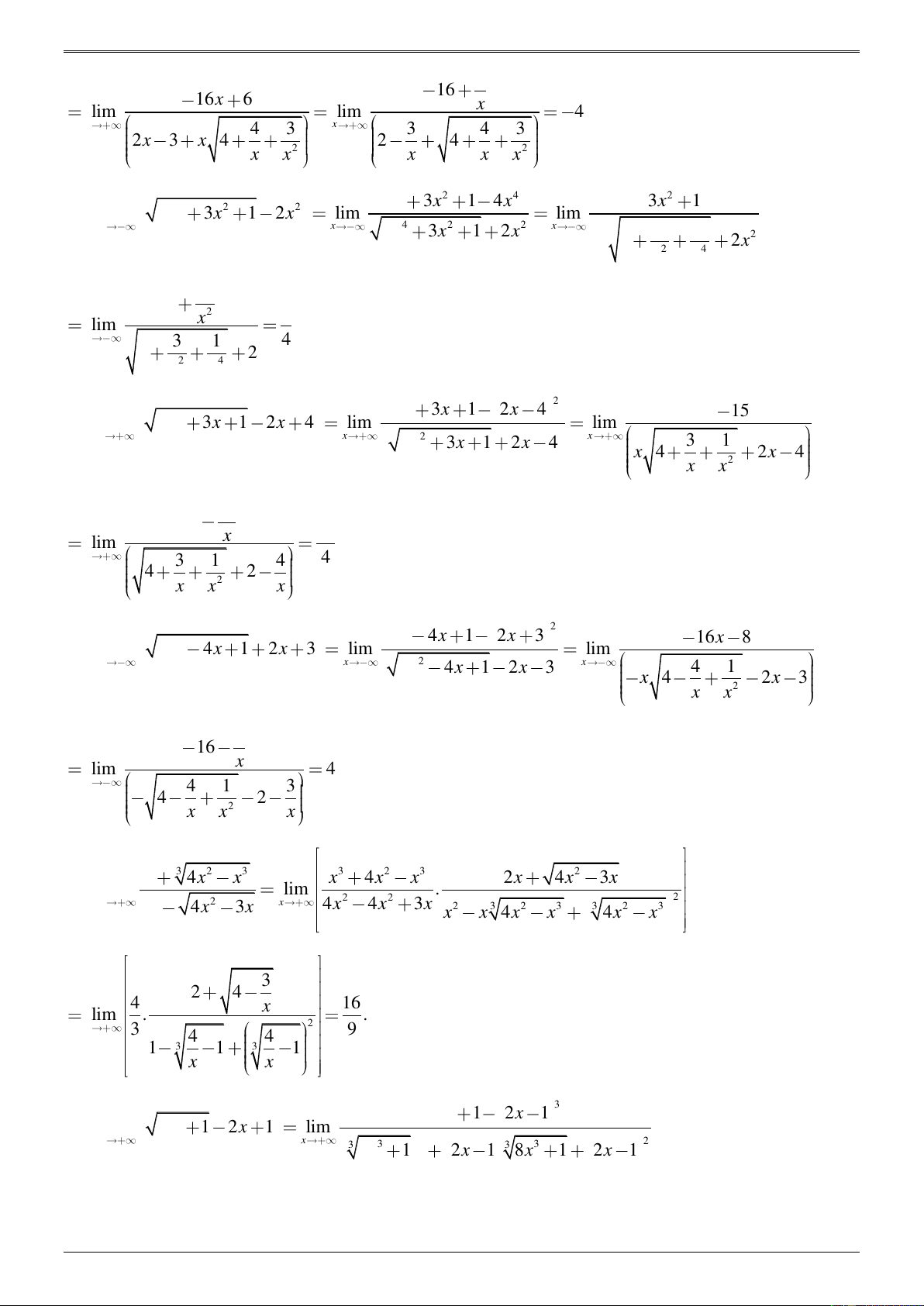
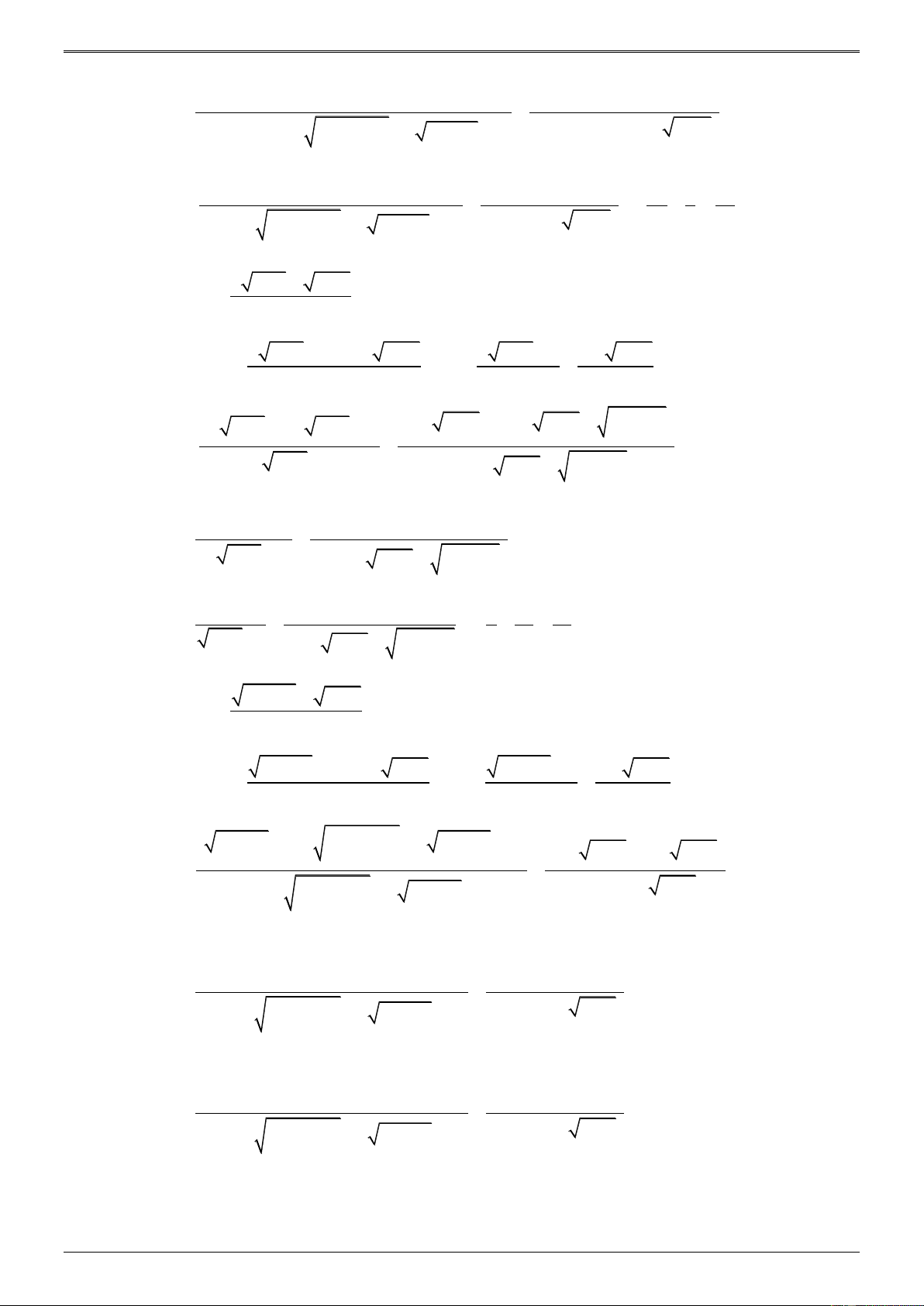
x → x−  x x x − x  0 0 0 0  VÍ DỤ − Ví dụ 1. 2x 3
Tính giới hạn A = lim . Đs: . − + x 1 → x −1 Lời giải lim 2x 3 1 0 x 1 2x 3 Vì lim x 1 0 A lim . x 1 x 1 x 1 x 1 x 1 x 1 0 − Ví dụ 2. x 15
Tính giới hạn A = lim . Đs: . − + x→2 x − 2 Lời giải lim x 15 13 0 x 2 x 15 Vì lim x 2 0 A lim . x 2 x 2 x 2 x 2 x 2 x 2 0 − Ví dụ 3. 2 x
Tính giới hạn A = lim . Đs: . − − x 3 → 3 − x Lời giải
lim (2 − x) = −1 0 − x→3  2 − x
Vì lim (3 − x) = 0  A = lim = − . − − x→3 x→3 3 − x
x → 3−  x  3  3− x  0  + Ví dụ 4. x 1
Tính giới hạn A = lim . Đs: . + + x→2 2x − 4 Lời giải 33 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC lim (x + ) 1 = 3  0 + x→2  x +1
Vì  lim (2x − 4) = 0  A = lim = + . + + x→2 x→2 2x − 4 
x → 2+  x  2  2x − 4  0  − Ví dụ 5. x 5
Tính giới hạn A = lim Đs: . − − x→ (x −4) .2 4 Lời giải lim (x −5) = 1 −  0 − x→4   − 2 x 5
Vì  lim ( x − 4) = 0  A = lim = − . − − x→ x→  (x − 4)2 4 4 x → 4−   (x − 4)2  0 − Ví dụ 6. 3x 8
Tính giới hạn A = lim Đs: . + − x→ (3− x) . 2 3 Lời giải
lim (3x −8) =1 0 − x→3   − 2 3x 8
Vì lim (3 − x) = 0  A = lim = + . − − x→ x→  (3− x)2 3 3 x → 3−   (3− x)2  0 2 + − Ví dụ 7. 2x 5x 3
Tính giới hạn A = lim . Đs: . − + x ( → − ) (x +3)2 3 Lời giải 2 2x + 5x − 3 (2x − ) 1 ( x + 3) 2x −1 Ta có lim = lim = lim + + + x ( → − ) (x +3)2 x→(− ) (x +3)2 3 3 x→( 3 − ) x + 3  lim (2x − ) 1 = 7 −  0 + x→( 3−) 2  2x + 5x − 3
Vì  lim ( x + 3) = 0  A = lim = − . + + x→(− ) x→(− )  (x +3)2 3 3 + x →  ( 3 − )  x  3 −  x + 3  0   Ví dụ 8. 1 1
Tính giới hạn A = lim − .   Đs: . − − 2
x→2  x − 2 x − 4  Lời giải  1 1  x +1 Ta có: A = lim − = lim   − 2 − x→2  − − x→2 x 2 x 4  (x − 2)(x + 2) 34 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC lim (x + ) 1 = 3  0 − x→2   1 1 
Vì lim ( x − 2)( x + 2) = 0  A = lim − = −    . − − 2 x→2 x→2 
x − 2 x − 4 
x → 2−  x  2  
(x − 2)(x + 2)  0 2 − x Ví dụ 9. 1
Tính giới hạn B = lim . Đs: − . − 2 x→2 2x − 5x + 2 3 Lời giải Vì x 2− →
x  2  2 − x = 2 − x − − Do đó 2 x 1 1 B = lim = lim = − . − −
x→2 ( x − 2)(2x − ) x→2 1 2x −1 3 x − 3 Ví dụ 10. 1
Tính giới hạn B = lim . Đs: . + x 3 → 5x −15 5 Lời giải Vì x 3+ →
x  3  x − 3 = x − 3 − Do đó x 3 1 1 B = lim = lim = . − − x 3 → 5( x − 3) x 3 → 5 5
BÀI TẬP ÁP DỤNG
Bài 1. Tính các giới hạn sau: x −1 1 1) A = lim . Đs: − . − 3 x 1 → 2x + x − 3 7 x − 2 2) B = lim .
Đs: Không tồn tại. x→2 x − 2 2 x − 9 3) C = lim .
Đs: Không tồn tại. x→3 x − 3 Bài 2. Tính các giới hạn sau: 2
2x − 2x + x −1 x + 3 7 1) C = lim . Đs: . − 2 x 1 → x − 2x +1 4 x − 2 2) C = lim . Đs: 2. − − x→2 x −1 −1 2 x − 7x +12 1 3) D = lim . Đs: . − → 2 x 3 9 − x 6 2 x − 5x + 6 1 35 4) D = lim . Đs: . − → 2 x 2 4 − x 2 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 1− x + x −1 5) D = lim . Đs: 1. − → 2 3 x 1 x − x x + 5
6) D = lim (1− x) . Đs: 0. + 3 2 x 1 → x + 2x − 3 3 x − 3x + 2 3 7) D = lim . Đs: . − 2 x 1 → x − 5x + 4 3 4 2 5
 x −6x − x khi x 1 Bài 3.
1) Tính giới hạn C = lim f ( x) với f ( x) =  . Đs: −2 x 1 → 3 x −3x khi x  1 x − 3 khi x  1 
2) Tính giới hạn C = lim f ( x) với f ( x) =  . Đs: −2 . x 1 → 2 1
 − 7x + 2 khi x 1 3x − 2  khi x  2 −
3) Tính giới hạn C = lim f ( x) với f ( x) =  x +1 . Đs:8 . x 2 →−
x +10 khi x  2 − 3  x +1  khi x  1 − Bài 4.
Tìm m để hàm số f ( x) =  x +1
có giới hạn tại x = 1. −  2 2
mx − x + m khi x  1 −
Đs: m =1 hoặc m = 2 − .  LỜI GIẢI x −1 Bài 1. 1) A = lim . − 3 x 1 → 2x + x − 3 Vì x 1−
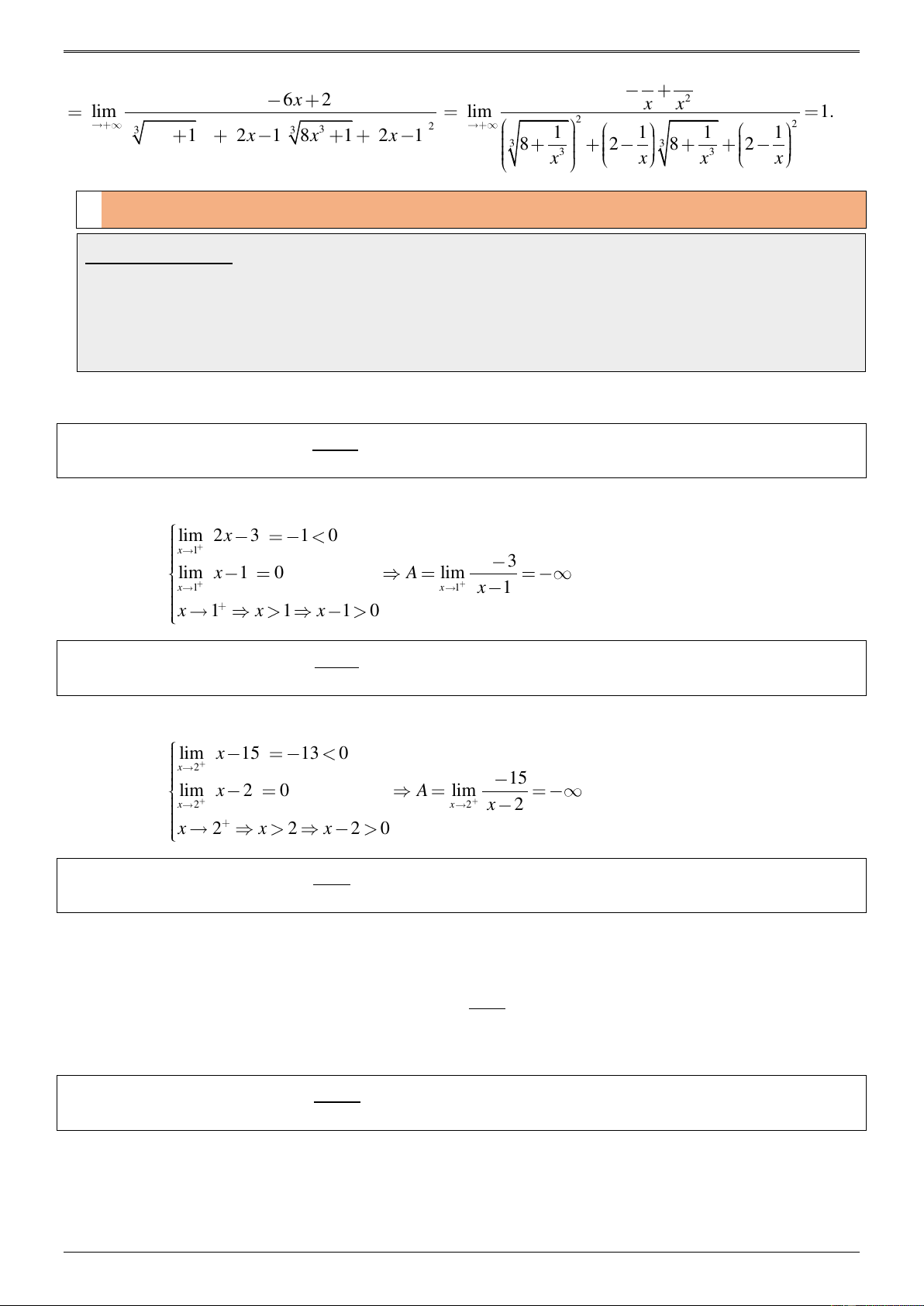
→  x 1 x −1 = −(x − ) 1 . −(x − ) − Do đó 1 1 1 A = lim = = − − − x→ (x − ) 1 ( lim . 2 1 2x + 2x + 3) 2 x 1 → 2x + 2x + 3 7 x − 2 2) B = lim . x→2 x − 2 −(x − 2) +) Vì x 2− →
x  2  x − 2 = −(x − 2) nên lim = lim (− ) 1 = 1 − . − − x→2 − x→2 x 2 x − 2 +) Vì x 2+ →
x  2  x − 2 = x − 2 nên lim = lim1=1. − − x→2 − x→2 x 2 x − 2 x − 2 x − 2 Suy ra lim  lim
nên không tồn tại giới hạn của B = lim . − + x→2 − x→2 x→2 36 x 2 x − 2 x − 2 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 2 x − 9 3) C = lim . x→3 x − 3 x − 3 . x + 3 Ta có C = lim . Do đó: x 3 → x − 3 2 x − 9 (x −3). x +3 +) lim = lim = lim x + 3 = 6. + + + x→3 − x→3 − x→3 x 3 x 3 2 x − 9
−(x −3). x + 3 +) lim = lim = lim − + = − − − − ( x 3 ) 6. x→3 − x→3 − x→3 x 3 x 3 2 x − 9
Suy ra giới hạn của C = lim không tồn tại. x→3 x − 3 2
2x − 2x + x −1 x + 3 Bài 2. 1) C = lim . − 2 x 1 → x − 2x +1 Vì x 1−
→  x −1 0  x −1 = −(x − ) 1 . Do đó 2x ( x − ) 1 − ( x − ) 2 1 x + 3 2x − x + 3 4x − x − 3 C = lim = = − − − x→ (x − ) lim lim 2 1 x 1 → − x 1 1 x 1 → (x − ) 1 (2x + x + 3) (x − ) 1 (4x + 3) 4x + 3 7 = lim = lim = . − − x 1 → (x − )
1 (2x + x + 3) x 1 → 2x + x + 3 4 x − 2 2) C = lim . − x→2 x −1 −1 Vì x 2− →
x − 2  0  x − 2 = −(x − 2) . Do đó:
−(x − 2)( x −1+ ) 1 C = lim = lim − − +  = − . − − − −  ( x 1 )1 2  x→2 (x ) x→2 1 1 2 x − 7x +12 3) D = lim . − → 2 x 3 9 − x (x −3)(x −4) 3 − x. 4 − x 4 − x 1 Ta có D = lim = lim = lim = . − − − x 3 →
(3− x)(3+ x) x 3 → x 3 3 − x. 3 + x → 3 + x 6 2 x − 5x + 6 4) D = lim . − → 2 x 2 4 − x (x −2)(x −3) 2 − x. 3 − x 3 − x 1 Ta có D = lim = lim = lim = . − − − x→2
(2− x)(2+ x) x→2 x→2 2 − x. 2 + x 2 + x 2 37 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 1− x + x −1 5) D = lim . − → 2 3 x 1 x − x
1− x − (1− x)
1− x − (1− x)2 1− 1− x Ta có D = lim = lim = lim =1. − → 2 − − x 1 x (1− x) x 1 → x 1 x 1− x → x x + 5
6) D = lim (1− x) . + 3 2 x 1 → x + 2x − 3  (  x − )2 1 ( x + 5)  (x − ) 1 ( x + 5)  Ta có D = lim −  = −  = + + x→  (x − ) 1 ( lim 0. 2 1 x + 3x + 3) 2  x 1 →  x + 3x + 3      3 x − 3x + 2 7) D = lim . − 2 x 1 → x − 5x + 4 (x − )2 1 ( x + 2) (1− x) x + 2 x + 2 3 Ta có D = lim = lim = lim = . − − − x 1 → (x − ) 1 ( x − 4) x 1 → (x − ) 1 ( x − 4) x 1 → 4 − x 3 Bài 3. 1) Ta có:
+) lim f ( x) = lim ( 3 x − 3x = − − − ) 2. x 1 → x 1 →
+) lim f ( x) = lim ( 4 2
5x − 6x − x = − − = − + + ) 5 6 1 2. x 1 → x 1 →
+) Vì lim f ( x) = lim f ( x) = 2
− nên hàm số f (x) có giới hạn tại x =1 và − + x 1 → x 1 → lim f ( x) = 2 − . x 1 → 2) Ta có:
+) lim f ( x) = lim ( x − 3) = 2 − . − − x 1 → x 1 →
+) lim f ( x) = lim − x + = − + + → x→ ( 2 1 7 2 2. x 1 1 )
+) Vì lim f ( x) = lim f ( x) = 2
− nên C = lim f (x) = 2 − . − + x 1 → x 1 → x 1 → 3) Ta có: 3x − 2
+) lim f ( x) = lim = 8. − − x ( → 2 − ) x ( → 2 − ) x +1
+) lim f ( x) = lim ( x +10) = 8. + + x ( → 2 − ) x ( → 2 − )
+)Vì lim f ( x) = lim f ( x) = 8 nên C = lim f ( x) = 8. − + x ( → 2 − ) x ( → 2 − ) x 2 →− Bài 4. Ta có: 38 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 3 x +1
+) lim f ( x) = lim = lim − + = − − − ( 2 x x )1 3. x→(− ) 1 x→(− ) 1 x +1 x→(− ) 1
+) lim f ( x) = lim − + = + + + + ( 2 2 mx x m ) 2 m m 1. x ( → − ) 1 x→(− ) 1
+) Để hàm số có giới hạn tại x = 1 − thì m =1 2 2
3 = m + m +1  m + m − 2 = 0  .  m = 2 −
Dạng 5. Giới hạn của hàm số lượng giác
Phương pháp giải:
- Sử dụng các định lý về giới hạn hàm số
- Sử dụng các công thức biến đổi lượng giác sin x - Lưu ý: lim 1 x 0 x VÍ DỤ − Ví dụ 1. 2sin x 1 1
Tính giới hạn A = lim . Đs: A = − . 2  − x→ 4 cos x 3 2 6 Lời giải 2sin x −1 2sin x −1 2sin x −1 1 − 1 Ta có: A = lim = lim = lim = lim = − . 2     − − − − + x→ 4 cos x 3 x→ 4( 2 1 sin x) 2 3 x→ 1 4sin x x→ 1 2sin x 2 6 6 6 6 − Ví dụ 2. 2 sin x 1 1
Tính giới hạn A = lim . Đs: A = − . 2  − x→ 2 cos x 1 2 4 Lời giải 2 sin x −1 2 sin x −1 2 sin x −1 1 − 1 Ta có: A = lim = lim = lim = lim = − . 2     − − − − + x→ 2 cos x 1 x→ 2( 2 1 sin x) 2 1 x→ 1 2sin x x→ 1 2 sin x 2 4 4 4 4 − Ví dụ 3. cos 4x 1
Tính giới hạn A = lim . Đs: A = 0. x 0 → sin 4x Lời giải 2 2 2 2 cos 4x −1
cos 2x − sin 2x − cos 2x − sin 2x Ta có: A = lim = lim x 0 → x 0 sin 4x → 2sin 2x cos 2x 2 2 − sin 2x −sin 2x = lim = lim = 0. x 0 → x 0 2sin 2x cos 2x → cos 2x − − Ví dụ 4. 1 sin 2x cos 2x
Tính giới hạn A = lim . Đs: A = 1. − x 0
→ 1+ sin 2x − cos 2x Lời giải 39 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC − −
1− 2sin x cos x − ( 2 2 cos x − sin 1 sin 2 cos 2 x x x ) Ta có: A = lim = lim
x→ 1+ sin 2x − cos 2 x x
→ 1+ 2sin x cos x − ( . 2 2 0 0 cos x − sin x) 2
2sin x − 2sin x cos x
2sin x (sin x − cos x) sin x − cos x = lim = lim = lim = 1 − . 2 x→0 x→0
2sin x + 2sin x cos x
2sin x (sin x + cos x)
x→0 sin x + cos x
BÀI TẬP ÁP DỤNG Bài 1. Tính các giới hạn sau:
1+ sin 2x − cos 2x sin 2x 1) A = lim . Đs: A = 1. − 2) A = lim . Đs: A = 1. − x 0
→ 1− sin 2x − cos 2x x 0
→ 1− sin 2x − cos 2x
sin 7x − sin 5x
sin 5x − sin 3x 3) A = lim . Đs: A = 2. 4) A = lim . Đs: A = 2. x 0 → sin x x 0 → sin x 1− cos x 5) A = lim . Đs: A = 0. x 0 → sin x
cos 3x + 2 cos 2x + 2 2 3 6) A = lim . Đs: A = .  x→ sin 3x 3 3
1+ sin 2x + cos 2x 7) A = lim . Đs: A = 2.  x→ cos x 2 Bài 2. Tính các giới hạn sau: 2 1− cos axa  sin 5x 1) B = lim . Đs: B = .   2) B = lim . Đs: B = 5. x 0 → 1− cosbxb x 0 → x sin 5 . x sin 3 . x sin x 1 1− cos x 1 3) B = lim . Đs: B = . 4) B = lim . Đs: B = . 3 x 0 → 45x 3 2 x 0 → x 2 1− cos 5x 25 1− cosa x 2 a 5) B = lim . Đs: B = . 6) B = lim . Đs: B = . x 0 → 1− cos3x 9 2 x 0 → x 2 2 1− cos 2x sin x − tan x 1 7) B = lim . Đs: B = 4 . 8) B = lim
. Đs: B = − . x 0 → x sin x 3 x 0 → x 2 tan x − sin x 1 3 1− cos x 3 9) B = lim . Đs: B = . 10) B = lim . Đs: B = . 3 x 0 → sin x 2 x→0 x sin x 2 Bài 3. Tính các giới hạn sau: ( cos8x − ) 2 1 sin 3x 1− 2x +1 1 − 1) B = lim . Đs: B = 48 − . 2) B = lim . Đs: B = . 4 x→0 3.x x→0 sin 2x 2 1− cos cos 2x 3 3 1− cos x 1 40 3) B = lim . Đs: B = . 4) B = lim . Đs: B = . 2 x→0 x 2 2 x→0 tan x 6 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 3 tanx −1 1 5) B = lim . Đs: B = . 2  − x→ 2sin x 1 3 4
1+ tan x − 1+ sin x 1 1− cos x 6) B = lim . Đs: B = . 7) B = lim . Đs: B = 2 . 3 x→0 x 4 x→ (1− 1−x)2 0 2 1+ x − cos x
1− 2x +1 + sin x 8) B = lim . Đs: B = 1. 9) B = lim . Đs: B = 0 . 2 x→0 x x→0
3x + 4 − 2 − x 3 2 2x +1 − x +1 10) B = lim . Đs: 1. x→0 sin x
Bài 4. Tính các giới hạn sau:     1
1) C = lim tan 2x tan − x .    Đs: C =   x→   4  2 4 1+ cos x 1 2) C = lim Đs: C = x  → ( x − ) . 2 2 sin ( x − ) 1 1 − 3) C = lim . Đs: C = . 2 x  → x − 4x + 3 2 sin x − sin a 4) C = lim . Đs: C = cos . a x→a x − a LỜI GIẢI + −
1+ 2sin x cos x − ( 2 2 cos x − sin 1 sin 2 cos 2 x x x ) Bài 1. 1) A = lim = lim
x→ 1− sin 2x − cos 2 x x
→ 1− 2sin x cos x − ( . 2 2 0 0 cos x − sin x) 2
2sin x + 2sin x cos x
2sin x (sin x + cos x) sin x + cos x = lim = lim = lim = 1 − . 2 x→0 x→0
2sin x − 2sin x cos x
2sin x (sin x − cos x)
x→0 sin x − cos x sin 2x 2sin x cos x 2) A = lim = lim
x→ 1− sin 2x − cos 2 x x
→ 1− 2sin x cos x − ( . 2 2 0 0 cos x − sin x) 2sin x cos x 2sin x cos x cos x = lim = lim = lim = 1 − . 2 x→0 x→0
2sin x − 2sin x cos x
2sin x (sin x − cos x)
x→0 sin x − cos x
sin 7x − sin 5x 2cos 6 . x sin x 3) A = lim = lim = lim2cos6x = 2 . x 0 → x 0 → x 0 sin x sin x →
sin 5x − sin 3x 2cos 4 . x sin x 4) A = lim = lim = lim2cos 4x = 2. x 0 → x 0 → x 0 sin x sin x → 41 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC x x 2 2sin sin 1− cos x 5) 2 2 A = lim = lim = lim = 0. x→0 x→0 x x x x→0 sin x 2sin .cos cos 2 2 2 3 + +
4 cos x − 3cos x + 2( 2 2 cos x − sin x x x )+2 cos 3 2 cos 2 2 6) A = lim = lim 3   − x→ sin 3x x→ 3sin x 4sin x 3 3 − + cos x ( 2 3 2 4 cos x − 3 + 4 cos 4 cos 3cos 4 cos x x x x ) = lim =   −  − −  x→ sin x ( lim 2 3 4sin x) x→ sin x 3 4  ( 2 1 cos x 3 3 )
cos x (2cos x + )2 1 − 4 cos x  
(2cos x +3)(2cos x − ) 1
cos x (2cos x + 3) 2 3 = lim = lim = lim = .  2    −  − + + x→ sin x 4 cos x 1 x→ sin x (2cos x ) 1 (2cos x ) 1 x→ sin x (2cos x ) 1 3   3 3 3 2
1+ sin 2x + cos 2x
2 cos x + 2sin x cos x 7) A = lim = lim   x→ cos x x→ cos x 2 2
2 cos x (cos x + sin x) = lim
= lim 2(cos x + sin x) = 2.   x→ cos x x→ 2 2 2 axax bx  2 2 2sin sin 1− cos axa   a Bài 2. 1) 2 2 2 A = lim = lim = lim . .  = .   x→0 x→0 − bx bx x→0 1 cos b ax bx 2  b  2sin  sin  2  2 2  ax bx sin (Vì 2 lim =1 và 2 lim =1). x→0 ax x→0 bx sin 2 2 sin 5x  sin 5x  sin 5x 2) B = lim = lim 5. = 5   . (Vì lim =1). x→0 x→0 x  5x x 0 → 5x sin 5 . x sin 3 . x sin x
 sin 5x sin 3x sin x 1  1 3) B = lim = lim . . . =   3 x→0 x→0 45x  5x 3x x 3  3 sin 5x sin 3x sin x (Vì lim =1, lim =1, lim =1). x 0 → 5x x 0 → 3x x 0 → x x x 2 2sin 2 sin 1− cos x 1 4) 2 B = lim = lim = , (vì 2 lim =1. 2 2 2 x→0 x→0 xx  2 x→0  x  .4      2   2  2  5x  3  5 x x  2 2 sin . 2sin    1− cos 5x 2 = = =   2  25 25 5) 2 B lim lim lim .  = 2 x→0 x→0 1− cos 3x 3x x→0   2  5x  3x 9 9 2 42 2sin  .sin    2  2  2  Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 2 5x  3x  2 sin    2  (Vì 2 lim =1 và lim =1). 2 x→0  5x x→0 3x 2   sin  2  2   ax ax 2 2sin  2 2 2 sin 1− cosa x a a 6) = =  2 B lim lim .  = , (vì 2 lim =1). 2 2 2 x→0 x→0 xax 4    2 x→0  ax          2    2  2 2 2 sin 2x 4sin . x cos x  sin x  sinx 7) 2 B = lim = lim = lim .4 cos x = 4   , (vì lim =1). x→0 x→0 x→0 . x sin x . x sin xxx 0 → x sin x sin x − sin x − tan x sin .
x cos x − sin x 8) cos = lim = lim x B = lim 3 3 3 x→0 x→0 x→0 x x x cos x   x   − x ( − x) 2 sin sin 1 cos 2 − sin x 2 − 1 = =  2 − lim lim . .  = . 3 2 x→0 x→0 x cos xx x 4 cos x    2       2    x  2 sin   sinx  2  (vì lim =1và lim =1). 2 x 0 → x x→0  x     2  sin x −sin x tan x − sin x sin x − sin . x cos x 9) cos = lim = lim x B = lim 3 3 3 x→0 x→0 x→0 sin x sin x sin x cos x x 2 2sin 1− cos x 1 1 2 = lim = lim = lim = 2 x→0 x→0 x x x x→0 sin x .cos x 2 2 2 2 4.sin .cos .cos x cos .cos x 2 2 2 ( − )( + + ) x 2 2sin ( 2 2 1+ cos x + cos 1 cos 1 cos cos x x x x ) 10) 2 B = lim = lim x→0 x→0 x sin x x x 2 . x sin .cos 2 2  xx sin 2  sin
1+ cos x + cos x  3 2 = lim .  = , (vì 2 lim =1). x→0 x x 2  x→0 x 2 cos   2 2  2 ( cos8x − ) 2 1 sin 3x (cos8x − ) 2 2 2 1 sin 3x 2 − sin 4xsin 3x Bài 3. 1) B = lim = lim = lim 4 43 x→0 x→0 4 3x 3x ( cos8x + ) x→0 4 1 3x ( cos8x + ) 1 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 2 2
 sin 4x   sin3x  9 − 6  = lim  . .      = 4 − 8
x→0  4x   3x  cos8x +1   1− 2x +1  2x 1 −  1 2) B = lim = lim . = −   x→0 x→0 sin 2x
sin 2x 1+ 2x +1 2 2 − − 1− cos ( 2 2 1− 2 sin 1 cos cos 2 1 cos cos 2 x x x x x ) 3) B = lim = lim = lim 2 x→0 x→0 2 x
x (1+ cos x cos 2x ) x→0 2
x (1+ cos x cos 2x ) 2 2 2
sin x + cos x − cos x ( 2 1− 2sin x) 2 2 2
sin x + 2sin x cos x = lim = lim x→0 2
x (1+ cos x cos 2x ) x→0 2
x (1+ cos x cos 2x ) 2 2  sinx  1+ 2 cos x  3 = lim  .    = .
x→0  x  1+ cos x cos 2x   2  3 1− cos x 1− cos x 4) B = lim = lim 2 2 x→0 x→0 tan x
sin x 1+ cos x + cos x 2 ( 3 3 2 ) cos x x 2 2 4sin cos x 2 cos x 1 2 = lim = lim = . x→0 x x → x 2 2 ( x 3 2 + x + x ) 0 3 2 ( 3 3 2 + x + x ) 6 2sin cos 1 cos cos 2 cos 1 cos cos x 2 2 3 tanx −1 tan x −1 5) B = lim = lim 2   − x→ 2 sin x 1 x→ ( 2 2
sin x − cos x)( 3 2 3 tan x + tan x +1 4 4 ) sin x − cos x 1 1 cos = lim x = =   x→ ( lim . 2 2 x − x)( 3 2 3 x + x + ) x→ x ( x + x)( 3 2 3 3 sin cos tan tan 1 cos sin cos tan x + tan x +1 4 4 )
1+ tan x − 1+ sin x tan x − sin x 6) B = lim = lim 3 x→0 x→0 3 x
x ( 1+ tan x + 1+ sin x ) x 2 2 sin x sin
sin x − sin x cos x 2 = lim = lim x→0 3
x cos x ( 1+ tan x + 1+ sin x ) x→0 3
x cos x ( 1+ tan x + 1+ sin x ) 2  x     sin    sin x 2 1 2 = lim  .  .  = x→0  x x
 4( 1+ tan x + 1+ sin x ) 4   2     44 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC xx    2 sin − (1+ 1−x) 2 2  sin   2(1+ 1−  1 cos x x )2 2 7) 2 2 B = lim = =    = . x→ ( → → − − x ) lim lim . 2 2 2 0 x 0 x 0 x x  4 1 1     2     2 2 2 2 2 1+ x − cos x 1+ x − cos x x + sin x 8) B = lim = lim = lim 2 x→0 x→0 2 x → x ( 2
1+ x + cos x) x 0 2 x ( 2 1+ x + cos x) 2  sin x 1 1  1 1 = lim  . +  = + =1. 2 x→0 2 2 x  + + + + 2 2 1 x cos x 1 x cos x
1− 2x +1 + sin x 1− 2x +1 sin x 9) B = lim = lim + lim x→0 x→0 x→0
3x + 4 − 2 − x
3x + 4 − 2 − x
3x + 4 − 2 − x 2
− x( 3x + 4 + 2+ x)
sin x ( 3x + 4 + 2 + x) = lim + lim x→0 ( 2
−x − x)(1+ 2x +1) x→0 −x(x + ) 1 2
− ( 3x + 4 + 2+ x)
 sin x 3x + 4 + 2 + x  = lim + lim .   
x→0 (−x − )
1 (1+ 2x +1) x→0 x −x −1   = 4 − 4 = 0. 3 2 3 2 3 2 2x +1 − x +1
2x +1 −1+1− x +1 2x +1 −1 1− x +1 10) B = lim = lim = lim + lim x→0 x→0 x→0 x→0 sin x sin x sin x sin x 2 2x −x = lim + lim =1.
x→0 sin x ( 2x +1 + ) x→0 1  
sin x 1+ x +1 + ( x +  )2 3 2 2 3 1        Bài 4.
1) C = lim tan 2x tan − x      x→   4  4  
Đặt t = x − , vì x →  t → 0. Khi đó: 4 4      C = t + − t =   ( t t ) cos 2t 1 lim tan 2 ( 1) tan lim cot 2 tan = lim =   . 2 t →0 t →0 t →0   2   2 cos t 2 1+ cos x 2) C = lim x  → (x − )2
Đặt t = x − , vì x →  t → 0. Khi đó: t 2 2 sin 1− cos t 1 2 C = lim = lim = . 2 2 t →0 t →0 t t 2 sin ( x − ) 1 3) C = lim 2  → 45 x x − 4x + 3 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
Đặt t = x − , vì x →1 t → 0. Khi đó: sin ( x − ) 1 sin ( x − ) 1 sint 1 C = lim = lim = lim = − . 2 x  → x − 4x + 3 x  → ( x − ) 1 ( x − 3)
t→0 t (t − 2) 2 sin x − sin a 4) C = lim x→a x − a
Đặt t = x − a . vì x → a t → 0. Khi đó: t + 2a t (t + a) 2 cos .sin sin − sin a 2 2 C = lim = lim = cos a . t→0 t→0 t t 2. 2
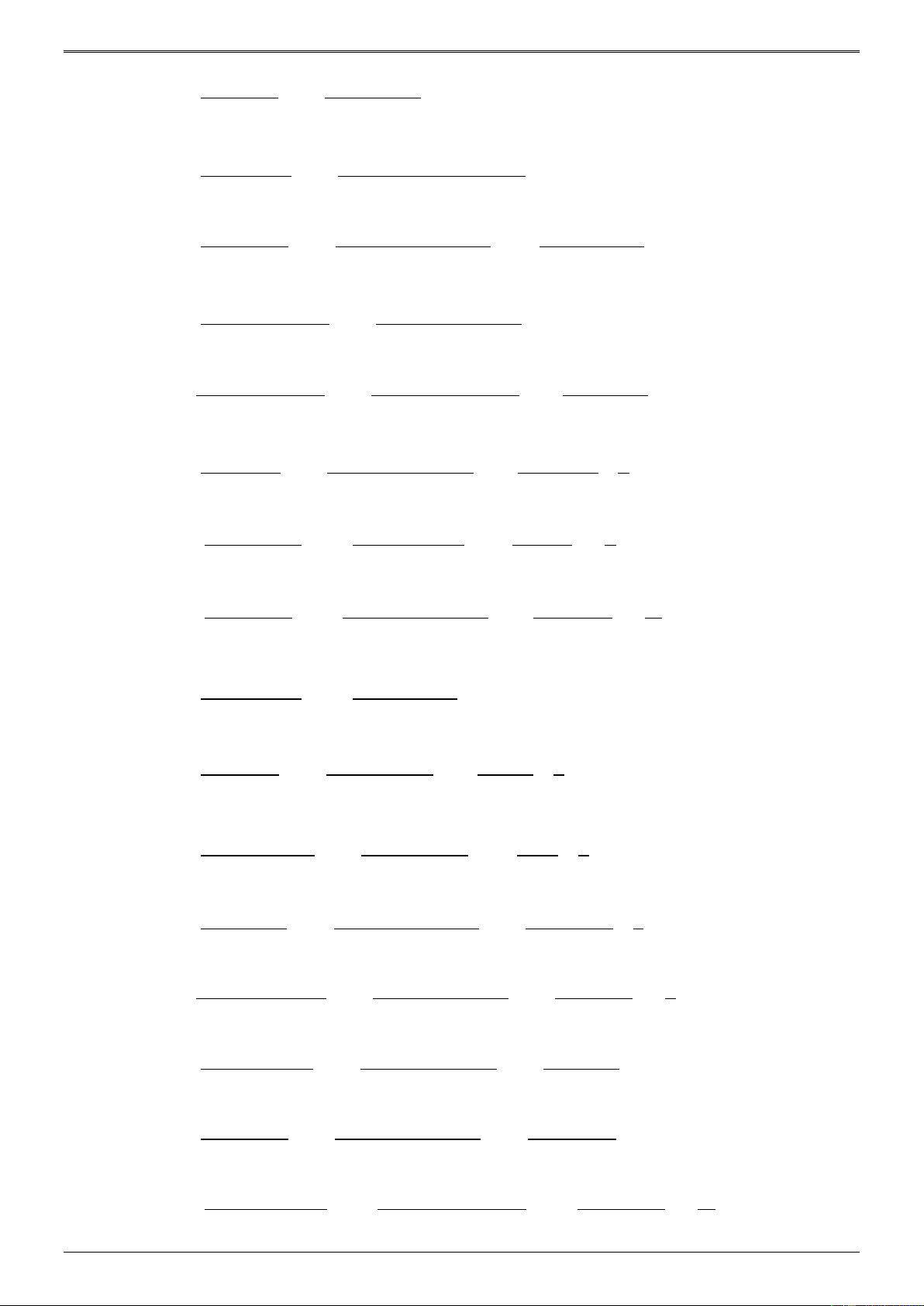
C. BÀI TẬP RÈN LUYỆN Bài 1. Tính các giới hạn sau: x − 3 2 x + 2x −15 1. lim . ĐS: 1 2. lim . ĐS : 8 2 x 3
→ x − x − 6 5 x 3 → x − 3 x + 3 2 x − 3x + 2 3. lim ĐS: 1 4. lim . ĐS: 1 . 2 x 3
→− x + 2x − 3 4 2 x→2 x − 4 4 2 x + 3x + 2 − 2 x − 7x +12 5. lim . ĐS: 1 6. lim . ĐS: 1 − . 2 x 2 →− 4 − x 4 2 x 3 → x − 9 6 2 x −1 2 x + x − 6 7. lim . ĐS: 2 8. lim . ĐS: 5 . 2 x 1 → x + 3x − 4 5 2 x→2 x − 4 4 2 2x + 3x −14 2 x − 9 9. lim . ĐS: 11. 10. l im . ĐS: 3 2 x→2 x − 4 4 2 x 3 → x − 4x + 3 2 3x − x −10 2 x − 5x 11. lim . ĐS: 11 . 12. lim . ĐS: 1 . 2
x→2 4x + x −18 7 2 x 5 → x − 25 2 2 4 − x 2 4 − x − 13. lim . ĐS: 2 14. lim . ĐS: 4 . 2
x→2 2x −10x +12 2
x→2 2x − x − 6 7 2 x − 5x + 6 2 x − 9x + 20 15. lim . ĐS: 1 . 16. lim . ĐS: 1 . 2 x 3 → x − 3x 3 2 x 5 → x − 5x 5 2 3x −10x + 3 2 x + 2x − 3 17. lim . ĐS: 8 18. lim . ĐS: 4 2 x 3 → x − 5x + 6 2 x 3
→ 2x − x −1 3 2
x − 5x + 6x 4 x −16 19. lim . ĐS: 1 − . 20. lim . ĐS: 16. − 2 x 3 → 9 − x 2 2 x 2
→− x + 6x + 8 3 8 − x 3 8 + x 21. lim . ĐS: 12 22. lim . ĐS: 12 . 2
x→2 x − 5x + 6 2 x 2
→− x +11x +18 7 46 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 2
x − x − 2 + 2 − 3 x − 8 23. lim . ĐS: 2 2 1. 24. lim . ĐS: 12. 3 2 x→ 2 x − 2 2 6
x→2 x − 3x + 2 3 x + 2 2 (x + )3 1 −1 25. lim . ĐS: 3 2 − . 26. lim . ĐS: 3. 2 x→− 2 x − 2 2 x→0 x (x + )3 1 − 27 4 x − 27x 27. lim . ĐS: 27. 28. lim . ĐS: 9. x→0 x 2 x 3
→ 2x − 3x − 9 3 2
x − 5x +10x − 8 3 2
2x − 5x + 2x +1 29. lim . ĐS: 2. 30. lim . ĐS: 1. − x→2 x − 2 2 x 1 → x −1 3 x − 2x − 4 3 2
x + 3x + 2x 31. lim . ĐS: 5 . 32. lim . ĐS: 2 − . 2 x→2 x − 4 2 2 x 2 →− x − x − 6 5 2 2x − x −10 3 2
x − x − x +1 33. lim . ĐS: 9 − . 34. lim . ĐS: 2. 3 x 2 →− x − x + 6 11 2 x 1 → x − 2x +1 2 x − 4 3 2
x − 2x − x + 2 35. lim . ĐS: 4 . 36. lim . ĐS: 3 . 3
x→2 x − 3x − 2 9 2 x→2 x − 4 4 2 x + 3x − 4 3 2
3x − 4x − 2x + 3 37. lim . ĐS: 5 38. lim . ĐS: 1 − 3 2 x 1 → 2x + x − 3 8 2 x 1 → 3x − 2x −1 4 3 2
x + x − 5x − 2 3 2x − 5x + 3 39. lim ĐS: 11 40. lim ĐS: -1 2 x→2 x − 3x + 2 2 x 1 → x − 3x + 2 2 x − 2x − 8 3 1− x 41. lim ĐS: 6 − . 42. lim ĐS: 3 . 3 2 x 2
→− 3x + 4x − x + 6 19 4 2 x 1 → x − 4x + 3 4 3 2
x − 5x + 3x + 9 3 2
6x − 5x + 4x −1 43. lim ĐS: 0 . 44. lim ĐS: 2 . 4 2 x 3 → x − 8x − 9 4 2 1 + − x→ 9x 8x 1 5 3 x + 2 x − 3 3 x − 3x + 2 45. lim ĐS: 4 − . 46. lim ĐS: 1 . x 1
→ x − 5 x + 4 3 4 x 1 → x − 4x + 3 2 5 4
x − 2x + x − 2 4 3
x − x − x +1 47. lim ĐS: 17 . 48. lim ĐS: 3 − . 2 x→2 x − 4 4 3 2 x 1
→ x − 5x + 7x − 3 2 3 2
2x − 5x − 2x − 3 3 2
2x + 5x + 4x +1 49. lim ĐS: 11 . 50. lim ĐS: 1 . 3 2 x 3
→ 4x −13x + 4x − 3 17 3 2 x 1 →−
x + x − x −1 2 3 2
2x − 5x − 2x − 3 3 2 3 x − 2 x +1 51. lim ĐS: 11 . 52. lim ĐS: 1 . 3 2 x 3
→ 4x −12x + 4x −12 20 2 x 1 → (x −1) 9 4 3 2
2x + 8x + 7x − 4x − 4 3 2
2x − 3x + x + 9 + 7 3 53. lim ĐS: 7 − . 54. lim ĐS: 7 3 3 2 x 2 →−
3x +14x + 20x + 8 4 2 x→− 3 3 − x 6 4 3 2
x − 5x + 9x − 7x + 2 5 4 3 2
x + x + x + x + x − 5 55. lim ĐS: 0 . 56. lim ĐS: 15 . 4 3 2 x 1 →
x − 3x + x + 3x − 2 2 x 1 → x −1 2 47 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC  1 2   1 12  57. lim −   ĐS: 1 . 58. lim −   ĐS: 1 . 2 x 1
→  x −1 x −1 2 3
x→2  x − 2 x − 8  2  1 1 
 2x − 3 x − 26  59. lim +   ĐS: −2 . 60. lim −   ĐS: 7 . 2 2
x→2  x − 3x + 2 x − 5x + 6  2 x 2 →−  x + 2 4 − x  2  1 1 
(1+ x)(1+ 2x)(1+ 3x) −1 61. lim −   ĐS: 2 . 62. lim ĐS: 6 . 2 3 x 1
→  x + x − 2 x −1 9 x 0 → x n x −1 n
x − nx + n −1 n − n − 63. lim ĐS: n . 64. lim ĐS: ( 2)( 1) . 1 m x→ x −1 m 2 x 1 → (x −1) 2 100 x − 2x +1 2 x + x + ... n + x − n n n + 65. lim ĐS: 2 . 66. lim ĐS: ( 1) . 50 x 1 → x − 2x +1 x 1 → x −1 2 Lời giải x − 3 x − 3 1 1 1. lim = lim = lim = . 2 x 3 → x 3 x − x − 6
→ ( x + 2)( x − 3) x 3 → x + 2 5 2 x + 2x −15 (x −3)(x +5) 2. lim = lim = lim( x + 5) = 8 x 3 → x 3 → x 3 x − 3 x − 3 → x + 3 x + 3 3. lim = lim 2 x 3
→− x + 2x − 3 x 3
→− ( x + 3)( x − ) 1 2 x − 3x + 2 (x − ) 1 ( x − 2) 1 1 x −1 1 4. lim = lim = lim = . = lim = . 2 x→2 x − 4
x→2 ( x − 2)( x + 2) x 3 →− x −1 4 x→2 x + 2 4 2 x + 3x + 2 (x + ) 1 ( x + 2) x +1 1 5. lim = lim = lim = − . 2 x→ 2 − x→ 2 4 − x
− (2 − x)(2 + x) x→ 2 − 2 − x 4 2 x − 7x +12 (x −3)(x −4) x − 4 1 6. lim = lim = lim = − . 2 x 3 → x 3 x − 9
→ ( x − 3)( x + 3) x 3 → x + 3 6 2 x −1 (x − ) 1 ( x + ) 1 x +1 2 7. lim = lim = lim = 2 x 1 → x 1 x + 3x − 4 → ( x − ) 1 ( x + 4) x 1 → x + 4 5 2 x + x − 6 (x −2)(x +3) x + 3 5 8. lim = lim = lim = . 2 x→2 x→2 x − 4
(x −2)(x + 2) x→2 x + 2 4 2 2x + 3x −14 (x −2)(2x +7) 2x + 7 11 9. lim = lim = lim = . 2 x→2 x→2 x − 4
(x −2)(x + 2) x→2 x + 2 4 2 x − 9 (x −3)(x +3) x + 3 10. l im = lim = lim = 3. 2 x 3 → x 3 x − 4x + 3
→ ( x − 3)( x − ) x 3 1 → x −1 2 3x − x −10 (x −2)(3x +5) 3x + 5 11 11. lim = lim = lim = . 2 x→2 x→2 4x + x −18
(x −2)(4x +9) x→2 4x +9 17 48 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 2 x − 5x x ( x − 5) x 1 12. lim = lim = lim = . 2 x 5 → x 5 x − 25
→ ( x − 5)( x + 5) x 5 → x + 5 2 2 4 − x (2− x)(2+ x) −x − 2 13. lim = lim = lim = 2. 2 x→2 x→2 2x −10x +12
2( x − 2)( x − 3) x→2 2 ( x − 3) 2 4 − x (2− x)(2+ x) −x − 2 4 − 14. lim = lim = lim = . 2 x→2 x→2 2x − x − 6
(x − 2)(2x +3) x→2 2x +3 7 2 x − 5x + 6 (x −2)(x −3) x − 2 1 15. lim = lim = lim = . 2 x 3 → x 3 x − 3x → x ( x − 2) x 3 → 2 3 2 x − 9x + 20 (x −4)(x −5) x − 4 1 16. lim = lim = lim = . 2 x 5 → x 5 x − 5x → x ( x − 5) x 5 → x 5 2 x − 5x + 6
(x −3)(3x − ) 1 3x −1 17. lim = lim = lim = 8. 2 x 3 → x 3 x − 3x
→ ( x − 2)( x − 3) x 3 → x − 2 2 x + 2x − 3 (x − ) 1 ( x + 3) x + 3 18. lim = lim = lim = 4. 2 x 3 → x 3 2x − x −1 → ( x − ) 1 (2x − ) x 3 1 → 2x −1 3 2
x − 5x + 6x
x ( x − 2)( x − 3) x ( x − 2) 1 19. lim = lim = lim = − 2 x 3 → x 3 9 − x →
(3− x)(3+ x) x 3 → −x − 3 2 x −
( 2x +4)(x−2)(x+2) ( 2 4 x + 4)( x − 2 16 ) 20. lim = lim = lim = 1 − 6 2 x→ 2 − x→ 2 x + 6x + 8 − (x + 2)(4+ x) x→ 2 − x + 4 3 2 8 − x
(2− x)(4+2x+ x ) 21. lim = lim 2
x→2 x − 5x + 6 x→2 (x −2)(x −3) + −( 2 x + 2x + 4) x (x + 2)( 2 3 x − 2x + 4 8 ) 22. lim = lim = lim = 12. 2 x→ 2 − x→ 2 x +11x +18 − (x + 2)(x +9) x→2 x − 3 2 x − 2x + 4 12 = lim = . x 2 →− x + 9 7 2 − − + x − x − + (x 2)(x 1 2 2 2 ) x −1+ 2 2 2 −1 23. lim = lim = lim = . 3 x→ x − 2 2 x→ (x− 2)( 2 2 2 x + 2x + 2) 2 x→ 2 x + 2x + 2 6 x − (x −2)( 2 3 x + 2x + 4 8 ) 2 x + 2x + 4 24. lim = lim = lim =12. 2 x→2 x→2 x − 3x + 2 (x − ) 1 ( x − 2) x→2 x −1 − − + x + (x 2)( 2 3 x 2x 2 2 2 ) 2 x − 2x + 2 3 2 25. lim = lim = lim = − . 2 x→− 2 x→− 2 x − 2
(x− 2)(x+ 2) x→− 2 x− 2 2 49 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC (x + )3 3 2 1 −1
x + 3x + 3x 26. lim = lim = lim( 2
x + 3x + 3) = 3. x→0 x→0 x→0 x x (  + + + +  x + ) x − (x 3)2 3 3( x 3) 9 1 27   2 27. lim = lim
= lim ( x + 3) + 3( x + 3) + 9 = 27.   x→0 x→0 x→0 x x − x x x
(x −3)( 2x +3x+9) x ( 2 4 x + 3x + 9 27 ) 28. lim = lim = lim = 9. 2 x 3 → x 3 2x − 3x − 9 → (x −3)(2x +3) x 3 → 2x + 3 x − x + x − (x − 2)( 2 3 2 x − 3x + 4 5 10 8 ) 29. lim = lim = lim ( 2
x − 3x + 4) = 2. x→2 x→2 x→2 x − 2 x − 2 − + + (x − ) 1 ( 2 3 2 2x − 3x x x x − ) 2 1 2 5 2 1 2x − 3x −1 30 lim = lim = lim = 1 − . 2 x 1 → x 1 x −1 → (x − ) 1 ( x + ) x 1 1 → x +1 x − x − (x −2)( 2 3 x + 2x + 2 2 4 ) 2 x + 2x + 2 5 31. lim = lim = lim = . 2 x→2 x→2 x − 4 (x −2)(x + 2) x→2 x + 2 2 3 2
x + 3x + 2x x ( x + ) 1 ( x + 2) x ( x + ) 1 2 32. lim = lim = lim = − . 2 x→ 2 − x→ 2 x − x − 6 −
(x + 2)(x −3) x→ 2− x −3 5 2 2x − x −10 (x + 2)(2x −5) 2x + 5 9 33. lim = lim = lim = − . 3 x→− x − x + 6
x→− ( x + 2)( 2 2 2 x − 2x + 3) 2 x→ 2 − x − 2x + 3 11
x − x − x +1 (x − )2 3 2 1 ( x + ) 1 34. lim . = lim = lim x +1 = 2. 2 2 ( ) x 1 → x 1 x − 2x +1 → (x − ) x 1 1 → 2 x − 4 (x −2)(x + 2) x + 2 4 35. lim = lim = lim = . 3 x→2 x→2 x − 3x − 2
(x −2)(x + )2 x→2 1 (x + )2 1 9 − − + (x −2)( 2 3 2 x x x x − ) 2 1 2 2 x −1 3 36. lim = lim = lim = . 2 x→2 x→2 x − 4
(x −2)(x + 2) x→2 x + 2 4 2 x + 3x − 4 (x −1)(x + 4) x + 4 5 37. lim = lim = lim = 3 2 2 2 x 1 → x 1 → x 1 2x + x − 3
(x −1)(2x + 3x + 3) → 2x + 3x + 3 8 3 2 2 2
3x − 4x − 2x + 3
(x −1)(3x − x − 3) 3x − x − 3 1 38 lim = lim = lim = − 2 x 1 → x 1 → x 1 3x − 2x −1 (x −1)(3x +1) → 3x +1 4 3 2 2 2
x + x − 5x − 2
(x − 2)(x + 3x +1) x + 3x +1 39. lim = lim = lim =11. 2 x→2 x→2 x→2 x − 3x + 2
(x − 2)(x −1) x −1 3 2 2 2x − 5x + 3
(x −1)(2x + 2x − 3) 2x + 2x − 3 40. lim = lim = lim = 1 − . 2 x 1 → x 1 → x 1 x − 3x + 2
(x − 2)(x −1) → x − 2 2 x − 2x − 8 (x + 2)(x − 4) x − 4 6 50 41. lim = lim = lim = − . 3 2 2 2 x→ 2 − x→ 2 − x→ 2
3x + 4x − x + 6
(x + 2)(3x − 2x + 3)
− 3x − 2x + 3 19 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 3 2 2 1− x
(x −1)(−x − x −1) −x − x −1 3 42. lim = lim = lim = . 4 2 3 2 3 2 x 1 → x 1 → x 1 x − 4x + 3
(x −1)(x + x − 3x − 3)
→ x + x − 3x − 3 4 3 2 2 2
x − 5x + 3x + 9
(x − 3)(x − 2x − 3) x − 2x − 3 43. lim = lim = lim = 0 . 4 2 3 2 3 2 x 3 → x 3 → x 3 x − 8x − 9
(x − 3)(x + 3x + x + 3)
→ x + 3x + x + 3 3 2 2 2
6x − 5x + 4x −1
(3x −1)(2x − x +1) 2x − x +1 2 44. lim = lim = lim = . 4 2 3 2 3 2 1 1 1 + − − + + + + + + x→ 9x 8x 1 x→ (3x 1)(3x x 3x 1) x→ 3x x 3x 1 5 3 3 3 x + 2 x − 3
( x −1)( x + 3) x + 3 4 45. lim = lim = lim = − . x 1 → x 1 → x 1 x − 5 x + 4
( x −1)( x − 4) → x − 4 3 3 2 x − 3x + 2
(x + 2)(x − 2x +1) x + 2 1 46. lim = lim = lim = . 4 2 2 2 x 1 → x 1 → x 1 x − 4x + 3
(x − 2x +1)(x + 2x + 3) → x + 2x + 3 2 5 4 4 4
x − 2x + x − 2 (x − 2)(x +1) x +1 17 47. lim = lim = lim = . 2 x→2 x→2 x→2 x − 4 (x − 2)(x + 2) x + 2 4 4 3 2 2 2
x − x − x +1
(x − 2x +1)(x + x +1) x + x +1 3 48. lim = lim = lim = − . 3 2 2 x 1 → x 1 → x 1
x − 5x + 7x − 3
(x − 2x +1)(x − 3) → x − 3 2 3 2 2 2
2x − 5x − 2x − 3
(x − 3)(2x + x +1) 2x + x +1 11 49. lim = lim = lim = . 3 2 2 2 x 3 → x 3 → x 3
4x −13x + 4x − 3
(x − 3)(4x − x +1)
→ 4x − x +1 17 3 2 2
2x + 5x + 4x +1
(2x +1)(x + 2x +1) 2x +1 1 50. lim = lim = lim = . 3 2 2 x→ 1 − x→ 1 − x→ 1
x + x − x −1
(x −1)(x + 2x +1) − x −1 2 3 2 2 2
2x − 5x − 2x − 3
(x − 3)(2x + x +1) 2x + x +1 11 51. lim = lim = lim = . 3 2 2 2 x 3 → x 3 → x 3
4x −12x + 4x −12 4(x − 3)(x +1) → 4(x +1) 20 3 2 3 3 2 x − 2 x +1 ( x −1) 1 1 52. lim = lim = lim = . 2 x 1 → x 1 → 3 2 3 2 3 2 x 1 → 3 2 3 2 (x −1) − + + + + 9 ( x 1) ( x x 1) ( x x 1) 4 3 2 2 2 2
2x + 8x + 7x − 4x − 4
(2x −1)(x + 4x + 4) 2x −1 7 53. lim = lim = lim = − . 3 2 2 x→ 2 − x→ 2 − x→ 2
3x +14x + 20x + 8
(3x + 2)(x + 4x + 4) − 3x + 2 4 3 2 2
2x − 3x + x + 9 + 7 3
(x + 3)(2x − (3 − 2 3)x + 7 − 3 3) 54. lim = lim 2 x→− 3 x→− 3 3 − x
( 3 − x)( 3 + x) 2
2x − (3 − 2 3)x + 7 − 3 3 7 3 = lim = x→− 3 3 − x 6 4 3 2 3
x − 5x + 9x − 7x + 2
(x −1) (x − 2) x −1 55. lim = lim = lim = 0. 4 3 2 2 x 1 → x 1 → x 1
x − 3x + x + 3x − 2
(x −1) (x − 2)(x +1) → x +1 51 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 5 4 3 2 4 3 2
x + x + x + x + x − 5
(x −1)(x + 2x + 3x + 4x + 5) 56. lim = lim 2 x 1 → x 1 x −1 → (x −1)(x +1) 4 3 2
x + 2x + 3x + 4x + 5 15 = lim = . x 1 → x +1 2  1 2  x −1 1 1 57. lim − = lim = lim =   . 2 x 1 → x 1 → x 1
x −1 x −1 (x −1)(x +1) → x +1 2  1 12  (x − 2)(x + 4) x + 4 1 58. lim − = lim = lim =   . 3 2 2 x→2 x→2 x→2
x − 2 x −8 
(x − 2)(x + 2x + 4) x + 2x + 4 2  1 1  2(x − 2) 2 59. lim + = lim = lim = 2 −   . 2 2 x→2 x→2 x→2
x − 3x + 2 x − 5x + 6 
(x − 2)(x − 3)(x −1)
(x − 3)(x −1)
 2x − 3 x − 26 
2(x − 5)(x + 2) 2(x − 5) 7 60. lim − = lim = lim =   . 2 x→ 2 − x→ 2 − x→ 2  x + 2 4 − x  (x − 2)(x + 2) − x − 2 2  1 1  (x −1)(x +1) x +1 2 61. lim − = lim = lim =   . 2 3 2 2 x 1 → x 1 → x 1
x + x − 2 x −1
(x −1)(x + 2)(x + x +1)
→ (x + 2)(x + x +1) 9 2
(1+ x)(1+ 2x)(1+ 3x) −1
x(6x +11x + 6) 62. lim = lim = lim( 2
6x +11x + 6) = 6 . x 0 → x 0 → x 0 x x → n n 1 − n−2 n 1 − n−2 x −1 (x −1)(x + x +...+ x +1) x + x +...+ x +1 n 63. lim = lim = lim = . m m 1 − m−2 m 1 − m−2 x 1 → x 1 → x 1 x −1 (x −1)(x + x +...+ x +1) → x + x +...+ x +1 m n n 1 − n−2
x − nx + n −1 (x −1)(x + x
+...+ x +1) − n(x −1) 64. lim = lim 2 2 x 1 → x 1 (x −1) → (x −1) n 1 − n−2 2 (x −1) + (x
−1) +...+ (x −1) + (x −1) = lim x 1 → x −1 = lim( n−2 n−3 n−3 n−4 (x
+ x +...+ x +1) + (x + x +...+ x +1) +...+ ) 1 x 1 →
(n − 2)(n −1)
= (n − 2) + (n −3) +...+ 2 +1= 2 100 x − 2x +1 99 98
(x −1)(x + x + ... + x +1) − (x −1) 99 98
x + x + ... + x 49 65. lim . = lim = lim = 50 x 1 → x − 2x +1 49 48 x 1
→ (x −1)(x + x + ... + x +1) − (x −1) 49 48 x 1
→ x + x + ...+ x 24 2 n 2
x + x + ... + x − n
(x −1) + (x −1) + ...+ ( n x −1) 66. lim = lim x 1 → x 1 x −1 → x −1 n 1 − n−2
(x −1) + (x −1)(x +1) + ... + (x −1)(x + x +...+ x +1) = lim x 1 → x −1 n n + n 1 − n−2
= lim(1+ (x +1) +...+ (x + x +...+ x + ( 1) 1)) = 1+ 2 + 3 + ... + n = x 1 → 2 Bài 2.
Tính các giới hạn sau: x + 3 − 2 x + 2 1. lim . 2. lim ĐS: 2 52 x 1 → x − ĐS: 1 1 4 x 2 →− x + 3 −1 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 3 − x + 3 x − 8 3. lim − . 4. lim ĐS: 6 − . x→6 x − ĐS: 1 6 6 x 8 → 3 − x +1 2 4 + x + x − 2 2
x − 3x − x 5. lim ĐS: 1 − . 6. lim ĐS: 1 . x 1 →− x +1 4 x 3 → 2x − 6 4 x + 2 − 2 2 − 3x − 2 7. lim . 8. lim − . 2 x→2 x − ĐS: 1 4 16 2 x→2 x − ĐS: 3 4 16 2 x − 9 x − 3 9. lim ĐS: 24. 10. lim − . 2 x 3 → x +1 − 2 x→9 9x − ĐS: 1 x 54 2 x − 49 2x − x + 3 11. lim ĐS: 56 − . 12. lim . 2
x→7 2 − x − 3 x 1 → x − ĐS: 7 1 8 x − 3 + 2x 2 x − x 13. lim . 14. lim ĐS:  . 2 x→3 x − ĐS: 2 3x 9 x 1 → 2 2x − x −1 4x +1 − 3 3x − 3 − 3 15. lim . 16. lim . 2 x→2 x − ĐS: 1 2x 3 2 x→4 x − ĐS: 1 4x 8 x + 2 − 2 2 x − 3x + 2 17. lim . 18. lim ĐS: 2 . 2
x→2 2x + x − ĐS: 1 10 4 x→2 x −1 −1 2 x − 3x − 4 3x +1 − 2 19. lim ĐS: 30 . 20. lim . 2 x→4 x + 5 − 3 x 1 → x + x − ĐS: 1 2 4 x −1 2 3x − 3(x +1) 21. lim . 22. lim ĐS: 12 − . 2 x 1 → x − ĐS: 1 1 4 x→2 3 − 4x +1 3 x +1 −1 x + 2 23. lim ĐS: 0 . 24. lim ĐS: 1 − . 2 x→0 x + x x 2 →− 3 1− x − 3 2 2 2x − x −1 2x + 5 + x − 5 25. lim ĐS: 0 . 26. lim . 2 2 x 1 → x − x x→2 x − ĐS: 2 2x 3 2 x − x
x − 2 + 7 − 2x 27. lim ĐS: 3 . 28. lim . 2 x 1 → 2x + 7 + x − 4 4 x 1 →− x − ĐS: 1 1 6 2
2x + 5 − 2x + x + 8
5x − 6 − x + 2 29. lim ĐS: 5 . 30. lim 2 x 1 →− x + 3x + 2 → − ĐS: 1. 2 x 2 x 2 3x + 3 2 2
x − 2x + 6 − x + 2x − 6 31. lim ĐS: 6 . 32. lim − . x 1 →− 3 + 2x − x + 2 2 x 3 → x − 4x + ĐS: 1 3 3 2
x + x + 2 − 1− x 2 − x + 2 33. lim lim ĐS: 3 − . 4 x 1 →− x + ĐS: 0 . 34. 4 x→2 x + 7 − 3 2 53 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 3 − x 3x +1 − x + 3 35. lim ĐS: 2 − . 36. lim ĐS: 3 . x→9 x − 5 − 2 3 x 1 → x + 8 − 3 x + 2 − 2x x + 3 − 2 37. lim ĐS: 1 − . 38. lim ĐS: 3 . x→2
x −1 − 3 − x 4 x 1 →
4x + 5 − 3x + 6 2
x +1 − 3x − 5 2 2x +1 − 2x + 5 39. lim ĐS: 3 − . 40. lim ĐS: 2 5 . x→3 2x + 3 − x + 6 x→2 2 x +1 − x + 3 3 x −1 4 4x + 3 −1 41. lim ĐS: 4 − . 42. lim 2 x 1 →
x + 3 + x − 3x 3 x 1 → x − ĐS: 1. 1 4 3 2
x −1 + x − 3x + x + 3 43. lim ĐS: 1. x→2 2x − 2 Lời giải x + 3 − 2
( x + 3 − 2)( x + 3 + 2) 1 1 1. Ta có lim = lim = lim = . x 1 → x 1 → x 1 x −1
(x −1)( x + 3 + 2) → x + 3 + 2 4 x + 2
(x + 2)( x + 3 +1) 2. Ta có lim = lim = lim( x + 3 +1) = 2 . x→ 2 − x→ 2 − x→ 2 x + 3 −1
( x + 3 −1)( x + 3 +1) − 3 − x + 3 (3 − x + 3)(3 + x + 3) 1 − 1 3. Ta có lim = lim = lim = − x→6 x→6 x→6 x − 6 (x − 6)(3 + x + 3) 3 + x + 3 6 x − 8
(x − 8)(3 + x +1) 3 + x +1 4. Ta có lim = lim = lim = 6 − . x 8 → x 8 → x 8 3 − x +1 (3 − x +1)(3 + x +1) → 1 − 2 4 + x + x − 2 x(x +1) x 1 5. Ta có lim = lim = lim = − . x→ 1 − x→ 1 − 2 x→ 1 − 2 x +1 + + + − + + − 4 (x 1)( 4 x x 2) 4 x x 2 2 2 2
2x − 3x − x
( 2x − 3x − x)( 2x − 3x + x) 6. Ta có lim = lim x 3 → x 3 → 2 2x − 6
(2x − 6)( 2x − 3x + x) x(x − 3) x 1 = lim = lim = . x→3 2 x→3 2 − − + − + 4 2(x 3)( 2x 3x x) 2( 2x 3x x) 2 + x − 2 x − 2 1 1 7. Ta có lim = lim = lim = . 2 x→2 x→2 x→2 x − 4
(x − 2)(x + 2)( 2 + x − 2)
(x + 2)( 2 + x − 2) 16 2 − 3x − 2 3(2 − x) 3 3 8. Ta có lim = lim = lim = − . 2 x→2 x→2 x→2 x − 4
(x − 2)(x + 2)(2 + 3x − 2)
(x + 2)(2 + 3x − 2) 16 2 x − 9
(x + 3)(x − 3)( x +1 + 2) 9. Ta có lim = lim
= lim (x + 3)( x +1 + 2) = 24   . x 3 → x 3 → x 3 x +1 − 2
( x +1 − 2)( x +1 + 2) → 54 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC x − 3 x − 9 1 − 1 10. Ta có lim = lim = lim = − . 2 x 9 → x 9 → x 9 9x − x
x(9 − x)( x + 3) → x( x + 3) 54 2 x − 49
(x − 7)(x + 7)(2 + x − 3) 11. Ta có lim = lim x→7 x→7 2 − x − 3 (2 − x − 3)(2 + x − 3)
(x − 7)(x + 7)(2 + x − 3) = lim
= −lim(x + 7)(2 + x − 3) = 5 − 6 x→7 x→7 7 − x 2 2x − x + 3 4x − x − 3 4x + 3 7 12. Ta có lim = lim = lim = . 2 x 1 → x 1 → x 1 x −1
(x −1)(x +1)(2x + x + 3)
→ (x +1)(2x + x + 3) 8 2 x − 3 + 2x x − 2x − 3 x +1 2 13. Ta có lim = lim = lim = . 2 x 3 → x 3 → x 3 x − 3x
x(x − 3)(x + 3 + 2x )
→ x(x + 3 + 2x) 9 2 2 2 x − x
x(x −1)( 2x − x +1)
x( 2x − x +1) 14. Ta có lim = lim = lim =  . 2 x 1 → 2 x 1 → x 1 − − −x + 2x −1 → −(x −1) 2x x 1 4x +1 − 3 4(x − 2) 4 1 15. Ta có lim = lim = lim = . 2 x→2 x→2 x→2 x − 2x
x(x − 2)( 4x +1 + 3) x( 4x +1 + 3) 3 3x − 3 − 3 3(x − 4) 3 1 16. Ta có lim = lim = lim = . 2 x→4 x→4 x→4 x − 4x
x(x − 4)( 3x − 3 + 3) x( 3x − 3 + 3) 8 x + 2 − 2
( x + 2 − 2)( x + 2 + 2) x − 2 17. Ta có lim = lim = lim 2 x→2 x→2 x→2 2x + x −10
(x − 2)(2x − 5)( x + 2 + 2)
(2x − 5)(x − 2)( x + 2 + 2) 1 1 = lim = −
x→2 (2x − 5)( x + 2 + 2) 4 2 x − 3x + 2
(x −1)(x − 2)( x −1 +1) 18. Ta có lim = lim
= lim(x −1)( x −1 +1) = 2 . x→2 x→2 x→2 x −1 −1
( x −1 −1)( x −1 +1) 2 x − 3x − 4
(x +1)(x − 4)( x + 5 + 3) 19. Ta có lim = lim
= lim(x +1)( x + 5 + 3) = 30 . x→4 x→4 x→4 x + 5 − 3 x − 4 3x +1 − 2 3(x −1) 3 1 20. Ta có lim = lim = lim = . 2 x 1 → x 1 → x 1 x + x − 2
(x −1)(x + 2)( 3x +1 + 2)
→ (x + 2)( 3x +1 + 2) 4 x −1 x −1 1 1 21. Ta có lim = lim = lim = . 2 x 1 → x 1 → x 1 x −1
(x +1)(x −1)( x +1)
→ (x +1)( x +1) 4 2 3x − 4(x +1)
(x − 2)(3x + 2)(3 + 4x +1)
(x − 2)(3x + 2)(3 + 4x +1) 22. Ta có lim = lim = lim x→2 x→2 3 − 4x +1
(3 − 4x +1)(3 + 4x +1) x→2 4(2 − x)
(3x + 2)(3 + 4x +1) = lim = 1 − 2 . x→2 4 55 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 3 3 2 x +1 −1 x x 23. Ta có lim = lim = lim = 0 2 x→0 x→0 3 x→0 3 x + x
x(x +1)( x +1 +1) (x +1)( x +1 +1) . 3 3 x + 2
(x + 2)( 1− x + 3) 1− x + 3 1 24. Ta có lim = lim = lim = . 2 2 x→ 2 − 3 x→ 2 − x→−2 − −
−(x + 2)(x − 2x + 4)
−(x − 2x + 4) 2 1 x 3 2 2 2x − x −1 ( − x −1) −(x −1) 25. Ta có lim = lim = lim = 0 2 x 1 → x 1 → 2 x 1 → 2 x − x
x(x −1)( 2x − x +1)
x( 2x − x +1) 2 2x + 5 + x − 5 −x +12x − 20 26. Ta có lim = lim 2 x→2 x→2 x − 2x
x(x − 2)( 2x + 5 − (x + 5))
−(x − 2)(x −10) −(x −10) 2 = lim = lim = x→2 x→2
x(x − 2)( 2x + 5 − (x + 5))
x( 2x + 5 − (x + 5)) 3 2 x − x
x(x −1)( 2x + 7 − (x − 4)
x( 2x + 7 − (x − 4) 3 27. Ta có lim = lim = lim = 2 x 1 → x 1 → x 1 2x + 7 + x − 4 −x +10x − 9 → −(x − 9) 4 2
x − 2 + 7 − 2x x − 2x − 3 28. Ta có lim = lim 2 x→ 1 − x→ 1 x −1
− (x −1)(x +1)((x − 2) − 7 − 2x) x + 3 1 = lim = x 1
→− (x −1)((x − 2) − 7 − 2x) 6 2
2x + 5 − 2x + x + 8 2x +17 5 29. Ta có lim = lim = 2 x→ 1 − x→ 1 − 2 x + 3x + 2 + + + + + 2 (x 2)((2x 5) 2x x 8)
5x − 6 − x + 2 4(x − 2) 4 30. Ta có lim = lim = lim =1. x→2 x→2 x→2 x − 2
(x − 2)( 5x − 6 + x + 2) ( 5x − 6 + x + 2) 3x + 3
3(x +1)( 3 + 2x + x + 2) 31. Ta có lim = lim
= lim 3( 3+ 2x + x + 2) = 6. x→ 1 − x→ 1 3 + 2x − x + 2 − x +1 x 1 →− 2 2
x − 2x + 6 − x + 2x − 6 4 − 1 32. Ta có lim = lim = . 2 x 3 → x 3 → 2 2 x − 4x + 3 − − + + + − 3 (x 1)( x 2x 6 x 2x 6) 2
x + x + 2 − 1− x x +1 33. Ta có lim = lim = 0. 4 x→ 1 − x→ 1 − 2 2 x + x
x(x − x +1)( x + x + 2 + 1− x ) 2 − x + 2 x + 7 + 3 3 34. Ta có lim = −lim = − . x→2 x→2 x + 7 − 3 2 + x + 2 2 3 − x x − 5 + 2 2 35. Ta có lim = −lim = − . x→9 x→9 x − 5 − 2 3 + x 3 3x +1 − x + 3 2( x + 8 + 3) 36. Ta có lim = lim = 3. x 1 → x 1 x + 8 − 3 → 3x +1 + x + 3 56 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC x + 2 − 2x x −1 + 3 − x 1 37. Ta có lim = lim = − . x→2 x→2
x −1 − 3 − x x + 2 + 2x 4 x + 3 − 2 4x + 5 + 3x + 6 3 38. Ta có lim = lim = . x 1 → x 1
4x + 5 − 3x + 6 → x + 3 + 2 2 x +1 − 3x + 5 2x + 3 + x + 6 39. Ta có lim = 2 − lim = 3 − . x→3 x→3 2x + 3 − x + 6 x +1 + 3x + 5 2 2 2x +1 − 2x + 5 x +1 + x + 3 2 5 40. Ta có lim = lim 2 = . x→2 2 x→2 2 + − + + + + 3 x 1 x 3 2x 1 2x 5 2 x −1
x + 3 − (x − 3x) 4 41. Ta có lim = lim = − . 2 3 2 x 1 → x 1
x + 3 + x − 3x
→ −x + 5x − 4x − 3 3 4 4x − 3 −1 4 42. Ta có lim = lim =1. x 1 → x 1 → 3 2 − 4 4 4 x 1
(4x − 3) + (4x − 3) + 4x − 3 +1 4 3 2
x −1 + x − 3x + x + 3 43. Ta có lim =1. x→2 2x − 2 Bài 3.
Tính các giới hạn sau: 3 4x − 2 3 5x − 3 + 2 1. lim . 2. lim . x→2 x − . ĐS: 1 2 3 x 1 →− x + ĐS: 5 1 12 3 1− 1− x 3 2 − 5x + 3 3. lim . 4. lim − . 2 x→0 x + ĐS: 1 x 3 x 1 → x − ĐS: 5 1 12 x − 3 x −1 5. lim ĐS: 2 . 6. lim ĐS: 3 . x→3 3 2 x −1 − 2 → 3 x 1 1+ x − 2 3 5x − 4 − x x −1 7. lim . 8. lim ĐS: 3 . 2 x 1 → 2x − x − ĐS: 2 1 9 → 3 x 1 x −1 3 x − 27 3 x + 5 − 2 9. lim ĐS: 54 . 10. lim . x→3 3 2 3 x +1− 4x + 28
x→3 x + x − ĐS: 1 30 336 3 3 10 + 2x + x −1 3 x −1 11. lim . ĐS: 3 . 12. lim ĐS: 2 . 2 x 1 →− x + 3x + 2 2 x 1 → 2 x + 3 + − 2 3 x −1 2 x + 3 − 2 13. lim . ĐS: 6 . 14. lim ĐS: 3 − . → 3 x 1 x + 7 − 2 →− 3 x 1 x +1 2 3 3 2x −1 − x 3 x −1 15. lim . ĐS: 2 . 16. lim ĐS: 1. x 1 → x −1 3 x 1 → 2 x − 2 +1 57 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 3 x −1 3 3 x + 2 + x 17. lim . ĐS: 1. 18. lim − . → 3 x 1 4x + 4 − 2 2 x 1 →− x − ĐS: 1 1 3 3 3 x + 9 + 2x − 6 3 3 19 − x + 2 19. lim . 20. lim ĐS: 27 − . 3 x 1 → x + . ĐS: 1 1 12 x 3 → 4x − 3 − 3 8 3 3 1+ x − 1− x 3 2x −1 −1 21. lim − . 22. lim . 2 x→0 x − . ĐS: 1 4x 6 3 x 1 → x − ĐS: 2 1 9 3 3x + 2 − x 3 x +1 −1 23. lim . ĐS: 1 − . 24. lim ĐS: 2 . x→2 3x − 2 − 2 → 4 x 0 2x +1 −1 3 Lời giải 3 4x − 2 4 1 1) Ta có lim = lim = . x→2 x→2 3 2 3 x − 2 + + 3 16x 2 4x 4 3 5x − 3 + 2 5 5 2) Ta có lim = lim = . x→ 1 − x→ 1 x +1 − 3 ( x − )2 3 12 5 3 − 2 5x − 3 + 4 3 1− 1− x 1 1 3) Ta có lim = lim = . 2 x→0 x→0 x + x
(x + )( + −x + ( −x)2 3 3 ) 3 1 1 1 1 3 2 − 5x + 3 5 − 5 4) Ta có lim = lim = − . x 1 → x 1 x −1 → 3 3 + x + + ( x + )2 12 4 2 5 3 5 3 − + − + x − ( 2x )2 3 2 3 1 2 x 1 4 3 5) Ta có lim = lim = 2. x→3 3 2 x→3 − − x + 3 x 1 2 x −1 2 6) Ta có lim = lim 1− x − 2 + x − 2 = 3. → 3 x 1 x 1 → + − ( 3 3 ( ) ) 1 x 2 3 2 5x − 4 − x −x − x + 4 2 7) Ta có lim = lim = . 2 x 1 → x 1 2x − x −1
→ ( x + )(3 ( x− )2 3 + x − + ) 9 2 1 5 4 5 4 4 x −1 8) Ta có lim = lim x + x + = x→ x→ ( 3 2 3 1 3. 3 1 1 ) x −1 58 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 3 x − 27 9) Ta có lim x→3 3 2 x +1− 4x + 28 (   x − 3)( 2
x + 3x + 9) ( x + ) 1 + ( x + ) 2 1 4x + 28 +  ( 2 3 4x + 28)2 2 3    = lim x→ (x −3)( 2 3 x + 2x + 9) (   2
x + 3x + 9) ( x + ) 1 + ( x + ) 2 1 4x + 28 +  ( 2 3 4x + 28)2 2 3    = lim = 72. 2 x→3 x + 2x + 9 3 x + 5 − 2 1 1 10) Ta có lim = lim = . 3 x 3 → x 3 x + x − 30 → (   x + x + ) 3 (x+ )2 2 3 336 3 10 5 + x + 5 + 4   3 3 10 + 2x + x −1 3 2
3x − 3x + 3x + 9 11) Ta có lim = lim 2 x 1 →− x + 3x + 2 x 1 →− (   x + ) 1 ( x + 2)  ( 3
10 + 2x )2 + ( x − ) 3 3 1
10 + 2x + ( x − )2 3 1    2 3x − 6x + 9 3 = lim = . x 1 →− (   x + 2)  ( 3
10 + 2x )2 + ( x − ) 3 2 3 1
10 + 2x + ( x − )2 3 1    3 2 x −1 x + 3 + 2 2 12) Ta có lim = lim = . x 1 → 2 x 1 x → + − (x + )(3 2 3 x + x + ) 3 3 2 1 1 3 x −1 (x + 7)2 3 + 2 x + 7 + 4 13) Ta có lim = lim = 6. → 3 x 1 x 1 x + 7 − 2 → x +1 (x − ) 1 + − (3 2 3 2 x − x + x )1 3 2 3 14) Ta có lim = lim = − . →− 3 x 1 x→ 1 − 2 x +1 + + 2 x 3 2 3 3 2x −1 − x x +1 2 15) Ta có lim = lim = . x 1 → x 1 x −1 → (2x − )2 1 + x(2x − ) 3 2 3 3 3 1 + x 3 3 x −1 (x −2)2 3 − x − 2 +1 16) Ta có lim = lim =1. → 3 x 1 x 1 → 3 2 3 x − 2 +1 x + x +1 3 3 x −1 (4x + 4)2 3 + 2 4x + 4 + 4 17) Ta có lim = lim =1. → 3 x 1 x 1 4x + 4 − 2 → 4 ( 3 2 3 x + x + ) 1 3 3 x + 2 + x 2 1 18) Ta có lim = lim = − . 2 x→ 1 − x→ 1 x −1 − (   x − ) 1
(x + 2)2 − x(x + 2) 3 2 3 3 3 + x   59 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 3 3 x + 9 + 2x − 6 19) Ta có lim 3 x 1 →− x +1 3 1 = lim = . x 1 →− (   x − x + ) 1 (x +9)2 2 3
− (x + 9)(2x − 6) + (2x + 6)2 2 3 3   − + − + − x + ( 2 3 3 9 3x x )( 4x 3 3 19 2 ) 27 20) Ta có lim = lim = − . x→3 x→3 4x − 3 − 3   4 −  ( 3 19 − x )2 3 3 8 3 − 2 19 − x + 4   3 3 1+ x − 1− x 2 1 21) Ta có lim = lim = − . 2 x→0 x→0 x − 4x (   x − )
( + x)2 + − x + ( − x)2 3 2 3 3 6 4 1 1 1   3 2x −1 −1 2 2 22) Ta có lim = lim = . 3 x 1 → x 1 x −1 → (  
x + x + ) 3 ( x − )2 2 3 9 1 2 1 + 2x −1 +1   − + − + x + − x (x )2 3 1 ( 3x 2 2 3 2 ) 23) Ta có lim = lim = 1 − . x→2 x→2 3x − 2 − 2   3 3 (3x + 2)2 3 2
+ x 3x + 2 + x   + + + + x + − (4 3 2x 1 )1( 2x 1 )1 1 1 2 24) Ta có lim = lim = . → 4 x 0 x→0 2x +1 −1   3 2 (x + )2 3 3 1 + x +1 +1   Bài 4.
Tính các giới hạn sau: x + 9 + x +16 − 7
2x + 2 + 5x + 4 − 5 1) lim . ĐS: 7 2) lim . ĐS: 4 x→0 x 24 x 1 → x −1 3
2 x + 6 + 2x − 2 − 8 2 x +1 + x + 4 − 4 3) lim . ĐS: 5 4) lim . ĐS: 5 x→3 x − 3 6 x→0 x 4 x x + 2 + x + 7 − 7 2
2x x −1 + x − 8 5) lim . ĐS: 8 6) lim . ĐS: 8 x→2 x − 2 3 x→2 x − 2
(5x −4) 2x −3 + x −84 3 1+ 2x − 1+ 3x 7) lim . ĐS: 74 8) lim . ĐS: 0 x→6 x − 6 3 x→0 x 3 3 2 x + 7 − x + 3
3 8x +11 − x + 7 9) lim . − 10) lim . ĐS: 7 x 1 → x − ĐS: 1 1 4 2 x→2 x − 3x + 2 54 3
2 1+ x − 8 − x 3 2 3x + 5 − x + 3 11) lim . ĐS: 13 12) lim . x→0 x 12 x 1 → x − ĐS: 1 1 4 3 2 3
x + 7 − 5 − x
3x + 2 − 3x − 2 13) lim . ĐS: 7 14) lim . ĐS: 1 − x 1 → x −1 12 x→2 x − 2 2 60 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
3 3x + 2 − 5x − 6 3 2
2x + 4x +11 − x + 7 15) lim . ĐS: 1 − 16) lim . ĐS: 5 . x→2 x − 2 2 x→2 x − 4 72 3 3 2
5 − x − x + 7 3 3
3 4x − 24 + x + 2 − 8 2x − 3 17) lim . ĐS: 11 − 18) lim . ĐS: 17 − 2 x 1 → x −1 24 2 x→2 4 − x 16 3 2
3x − 2 − 4x − x − 2 3
x 2x −1 + 3x − 2 − 2 19) lim . ĐS: 5 20) lim . ĐS: 3 2 x 1 → x − 3x + 2 6 2 x 1 → x −1 2 3 2 4 1+ x − 1− 2x 3 4 x + 6 − 7x + 2 21) lim . ĐS: 1 22) lim . ĐS: 13 − 2 x→0 x + x 2 x→2 x − 2 96
1+ 4x. 1+ 6x −1 3
1+ 2x. 1+ 4x −1 23) lim . ĐS: 5 24) lim . ĐS: 7 x→0 x x→0 x 3 3
3x +1. 2 − x − 2 3
4 + x. 8 + 3x − 4 25) lim . ĐS: 1 26) lim . ĐS:1 x 1 → x −1 12 2 x→0 x + x
4x + 4 + 9 − 6x − 5 3 1+ 2x − 1+ 3x 27) lim . ĐS: 5 − 28) lim . ĐS: 1 2 x→0 x 12 2 x→0 x 2 2
6x + 3 + 2x − 5x
4x − 3 + 2x −1 − 3x +1 29) lim ĐS: 11 30) lim . ĐS: 5 − . 2 x→ (x − ) . 2 1 1 6 x 1 → x − 2x +1 2 3
− x − 7 + 4 x + 3 + 2 2x −1 2 x − 4x + 4 31) lim . ĐS: 17 − 32) lim . ĐS: 8 2 x 1 → x − 2x +1 16 x→2 2
2x + 8 − 2 2x − 3 + x − 4 9 3 2 6x + 2 − 2 x 2 3 2
2x − 6x + 5 − 3x − 9x + 7 33) lim . ĐS: 1 34) lim ĐS: 1 3 2 2 x 1 →
x − x − x +1 8 x→2 (x − 2) 2 3 1+ 2x − 1+ 3x 3 1+ 4x − 1+ 6x 35) lim . ĐS: 1 36) lim . ĐS: 2 2 x→0 x 2 2 x→0 x Lời giải x + 9 + x +16 − 7 1) I = lim . x→0 xx + 9 −3 x +16 − 4  Ta có I = lim +    x→0 x x  
( x+9 −3)( x+9 +3) ( x+16 −4)( x+16 + 4) = lim  +  x→0  x  ( x+9 +3) x ( x +16 + 4)       x + 9 − 9 x +16 −16 x x = lim  +  = lim  +  x→0  x
 ( x + 9 + 3) x( x +16 + 4) x→0   x
 ( x + 9 + 3) x ( x +16 + 4)  1 1  1 1 7 = lim + = + = .   x→0 61  x + 9 + 3 x +16 + 4  6 8 24 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
2x + 2 + 5x + 4 − 5 2) I = lim . x 1 → x −1  2x + 2 − 2 5x + 4 − 3  Ta có I = lim +    x 1 → x −1 x −1  
( 2x+ 2 −2)( 2x+2 +2) ( 5x+4 −3)( 5x+4 +3) = lim  +  x 1 →  (x − )1  ( 2x+2 +2) (x − ) 1 ( 5x + 4 + 3)     2x + 2 − 4 5x + 4 − 9 = lim  +  x 1 → (x − ) 1 
( 2x+2 +2) (x− )1( 5x+4 +3)   2 ( x − ) 1 5( x − ) 1 = lim  +  x 1 → (x − ) 1 
( 2x+2 +2) (x− )1( 5x+4 +3)  2 5  2 5 4 = lim + = + = .   x 1 →  2x + 2 + 2 5x + 4 + 3  4 6 3
2 x + 6 + 2x − 2 − 8 3) I = lim . x→3 x − 3  2 x + 6 −6 2x − 2 − 2  Ta có I = lim +    x 3 → x − 3 x − 3  
2( x+6 −3)( x+6 +3) ( 2x−2 −2)( 2x−2 +2) = lim  +  x→3  (x −3)  ( x+6 +3)
(x −3)( 2x−2 +2)    2( x + 6 − 9) 2x − 2 − 4 = lim  + 
x→3  ( x − 3) 
( x+6 +3) (x−3)( 2x−2 +2)   2( x − 3) 2 ( x − 3) = lim  + 
x→3  ( x − 3) 
( x+6 +3) (x−3)( 2x−2 +2)  2 2  2 2 5 = lim + = + = .  
x→3  x + 6 + 3 2x − 2 + 2  6 4 6 2 x +1 + x + 4 − 4 4) I = lim . x→0 x  2 x +1 − 2 x + 4 − 2  Ta có I = lim +    x→0 x x  
2( x+1− )1( x+1+ )1 ( x+ 4 −2)( x+ 4 + 2) = lim  +  x→0  x  ( x+1+ )1 x ( x + 4 + 2)     2 ( x +1− ) 1 x + 4 − 4 =  2 1  2 1 5 lim  +  = lim + = + =   x→0  x x→0  + + + +  ( x +1 + ) 1
x ( x + 4 + 2) x 1 1 x 4 2  2 4 4 x x + 2 + x + 7 − 7 5) I = lim . x→2 x − 2 62 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
(x −2) x + 2 + 2 x + 2 −4+ x +7 −3  2 x + 2 − 4 x + 7 − 3  Ta có I = lim = lim x + 2 + +    x→2 x − 2 x→2 x − 2 x − 2  
2( x+ 2 −2)( x+2 +2) ( x+7 −3)( x+7 +3) = 2 + lim  +  x→2  (x − 2)  ( x+2 +2)
(x − 2)( x+7 +3)   2 1  2 1 8 = 2 + lim + = 2 + + = .  
x→2  x + 2 + 2 x + 7 + 3  4 6 3 2
2x x −1 + x − 8 6) I = lim . x→2 x − 2 2( x − 2) 2
x −1 + 4 x −1 − 4 + x − 4 2  4 x −1 − 4 x − 4  Ta có I = lim = lim 2 x −1 + +    x→2 x − 2 x→2 x − 2 x − 2  
4( x−1− )1( x−1+ )1 (    x − 2)( x + 2) 4 ( x −1− ) 1 = 2 + lim  +  = 2 + lim  + x + 2 x→2 
(x − 2)( x+1+ ) x→2 1 x − 2 
(x − 2)( x+1+    )1    4  4 = 2 + lim + x + 2 = 2 + + 4 = 8.  
x→2  x −1 +1  2
(5x −4) 2x −3 + x −84 7) I = lim . x→6 x − 6
(5x −30) 2x −3 + 26 2x −3 −78+ x −6 Ta có I = lim x→6 x − 6  ( − − − −  x ) 26 x ( 2x 3 3 5 6 2 3 ) x−6 = lim  + +  x→6  x − 6 x − 6 x − 6    
26 ( 2x − 3 − 3)( 2x − 3 + 3)  = lim 5 2x − 3 + +1 x→6  (x −6)  ( 2x−3+3)   26(2x − 3 − 9) 26.2( x − 6) =15 + lim +1=15 + lim +1
x→6 ( x − 6)( 2x −3 + 3)
x→6 ( x − 6)( 2x −3 + 3) 52 52 74 =15+ lim +1=15+ +1= . x 6 → 2x − 3 + 3 6 3 3 1+ 2x − 1+ 3x 8) I = lim . x→0 x 3 3
1+ 2x −1+1− 1+ 3x
 1+ 2x −1 1− 1+ 3x  Ta có I = lim = lim +    x→0 x→0 x x x   ( + − )( + +  ) ( 
1− 1+ 3x )(1+ 1+3x + (1+3 1 2 1 1 2 1 x x x )2 3 3 3 ) = lim +   x→0 x  ( 1+2x + )1
x (1+ 1+3x + (1+3x)2 3 3 )    63 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC      1+ 2x −1 1− (1+ 3x)  2 3 −   = lim + = lim +   x→0 x  ( 1+ 2x + ) 1 →  + +  x ( 3 x 3
1+ 1+ 3x + (1+ 3x)2 ) 0 3 1 2x 1 3 
1+ 1+ 3x + (1+ 3x)2     2 3 − = + = 0. 2 3 3 3 2 x + 7 − x + 3 9) I = lim . x 1 → x −1 3 3 2
x + 7 − 2 + 2 − x + 3 3 3 2  x 7 2 2 x 3  + − − + Ta có I = lim = lim +  x 1 → x −1 x 1 →  x −1 x −1     (    3 x + 7 − 2) ( 3 x + 7)2 3 3 3 3 + 2 x + 7 + 4 ( 2 2 − x + 3 )( 2 2 + x + 3 )   = lim  +  x 1 →  (   − + +  x − ) ( 3 x + )2 3 3 + x + + (x ) 1  ( 2 3 2 x 3 1 7 2 7 4 )          + − 4 − +  x ( 2 3 x 3 7 8 ) = lim  +  x 1 → (   − + +  x − ) ( 3 x + )2 3 3 + x + + (x )1  ( 2 3 2 x 3 1 7 2 7 4 )          3 2  x −1 1− x = lim  +  x 1 → (   − + +  x − ) ( 3 x + )2 3 3 + x + + (x )1  ( 2 3 2 x 3 1 7 2 7 4 )         2  x + x +1 x +1  3 2 1 = lim − = − = − .   x 1 →  ( 3x + )2 2 3 3 + + 12 4 4 3 2 x 3 7 + 2 x + 7 + 4   
3 8x +11 − x + 7 10) I = lim . 2 x→2 x − 3x + 2 3 3
8x +11 − 3 + 3 − x + 7
 8x +11 −3 3− x + 7  Ta có I = lim = lim +  2  2 2  x→2 x→2 x − 3x + 2 x − 3x + 2 x − 3x + 2    ( 
8x +11 − 3)( 3 (8x +1 )2 3 3 1
+ 3 8x +11 + 9) (3− x+7)(3+ x+7)  = lim +  
x→2  (x − x + )(3 ( x+ )2 2 3 + x +
+ ) ( 2x −3x+2)(3+ x+7 3 2 8 11 3 8 11 9 )      8x +11− 27 9 − ( x + 7)  = lim +  
x→2 (x − x + )(3 ( x+ )2 2 3 + x +
+ ) ( 2x −3x+2)(3+ x+7 3 2 8 11 3 8 11 9 )   64 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC    8( x − 2) 2 − x  = lim +  
x→2 (x − )(x − )(3 ( x+ )2 3 + x +
+ ) (x− )1(x−2)(3+ x+7 1 2 8 11 3 8 11 9 )      8 1  8 1 7 = lim − = − = .  
x→2 (x − )(3 ( x+ )2 3 + x +
+ ) (x− )1(3+ x+7 1 8 11 3 8 11 9 ) 27 6 54    3
2 1+ x − 8 − x 11) I = lim . x→0 x 3 3
2 1+ x − 2 + 2 − 8 − x
 2 1+ x − 2 2 − 8− x  Ta có I = lim = lim +    x→0 x→0 x x x    ( + − )( + +  ) ( 
2 − 8 − x )(4+ 2 8− x + (8 2 1 1 1 1 − x x x )2 3 3 3 ) = lim +   x→0 x  ( 1+ x + )1
x (4+ 2 8− x + (8− x)2 3 3 )       2(1+ x − ) 1 8 − (8 − x)  = lim +   x→0 x  ( 1+ x + ) 1
x (4+ 2 8− x + (8− x)2 3 3 )     2 1 2 1 13 lim   = + = + = . x→0  + +  3 1 x 1 3
4 + 2 8 − x + (8 − x)2 2 12 12   3 2 3x + 5 − x + 3 12) I = lim . x 1 → x −1 3 2 3 2 3x 5 2 2 x 3  3x 5 2 2 x 3  + − + − + + − − + Ta có I = lim = lim +  x 1 → x 1 x −1 →  x −1 x −1     (    2 3x + 5 − 2) ( 2 3x + 5)2 3 3 2 3 + 2 3x + 5 + 4 
 (2 − x + 3)(2 + x + 3) = lim  +  x 1 →  (   − + +  x − ) ( 2 x + )2 3 2 3 (x ) 1 + x + + (2 x 3 1 3 5 2 3 5 4 )           2  3x + 5 − 8 4 − ( x + 3) = lim  +  x 1 → (   − + +  x − ) ( 2 x + )2 3 2 3 (x )1 + x + + (2 x 3 1 3 5 2 3 5 4 )           3( 2 x − ) 1  1− x = lim  +  x 1 → (   − + +  x − ) ( 2 x + )2 3 2 3 (x )1 + x + + (2 x 3 1 3 5 2 3 5 4 )        65 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC    3( x + ) 1 1  6 1 1 = lim − = − = .   x 1 →  ( 2 + + 3x + 5)2 3 2 2 x 3 12 4 4 3 + 2 3x + 5 + 4    3 2
x + 7 − 5 − x 13) I = lim . x 1 → x −1 3 2  3 2 x 7 2 2 5 x x 7 2 2 5 x  + − + − − + − − − Ta có I = lim = lim +  x 1 → x 1 x −1 →  x −1 x −1     ( 
x + 7 − 2)( 3 (x + 7)2 3 3 + 2 x + 7 + 4) ( 2 2 − 5 − x )( 2 2 + 5 − x ) = lim +   x 1 →  (x − )
1 ( 3 (x + 7)2 + 2 x + 7 + 4) (x − ) 1 ( 2 3 2 + 5 − x )       + − 4 − ( 2 5 7 8 − x x )  = lim +   x 1 → (x − )
1 ( 3 (x + 7)2 + 2 x + 7 + 4) (x − ) 1 ( 2 3 2 + 5 − x )     2  x −1 x −1  = lim +   x 1 → (x − )
1 ( 3 (x + 7)2 + 2 x + 7 + 4) (x − ) 1 ( 2 3 2 + 5 − x )     1 x +1 1 2 7 lim   = + = + = . x 1 →   3 ( x + 7)2 2 3 + + + + − 12 4 12 2 x 7 4 2 5 x  
3 3x + 2 − 3x − 2 14) I = lim . x→2 x − 2 3 3
3x + 2 − 2 + 2 − 3x − 2
 3x + 2 − 2 2 − 3x − 2  Ta có I = lim = lim +    x→2 x→2 x − 2 x − 2 x − 2    ( 
3x + 2 − 2)( 3 (3x + 2)2 3 3
+ 2 3x + 2 + 4) (2− 3x−2)(2+ 3x−2) = lim +   x→2 
(x − )(3 ( x+ )2 3 + x + + )
(x − 2)(2+ 3x−2 2 3 2 2 3 2 4 )       3x + 2 − 8 4 − (3x − 2)  = lim +  
x→2 (x − )(3 ( x+ )2 3 + x +
+ ) (x−2)(2+ 3x−2 2 3 2 2 3 2 4 )      3( x − 2) 6 − 3x  = lim +  
x→2 (x − )(3 ( x+ )2 3 + x +
+ ) (x−2)(2+ 3x−2 2 3 2 2 3 2 4 )     3 3 3 3 − 1 lim   = − = + = − . x→2  + −  3 (3x + 2)2 3 2 3x 2 12 4 2 + 2 3x + 2 + 4   66 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
3 3x + 2 − 5x − 6 15) I = lim . x→2 x − 2 3 3
3x + 2 − 2 + 2 − 5x − 6
 3x + 2 − 2 2 − 5x −6  Ta có I = lim = lim +    x→2 x→2 x − 2 x − 2 x − 2    ( 
3x + 2 − 2)( 3 (3x + 2)2 3 3
+ 2 3x + 2 + 4) (2− 5x−6)(2+ 5x−6) = lim +   x→2 
(x − )(3 ( x+ )2 3 + x + + )
(x − 2)(2+ 5x−6 2 3 2 2 3 2 4 )       3x + 2 − 8 4 − (5x − 6)  = lim +  
x→2 (x − )(3 ( x+ )2 3 + x +
+ ) (x−2)(2+ 5x−6 2 3 2 2 3 2 4 )      3( x − 2) 10 − 5x  = lim +  
x→2 (x − )(3 ( x+ )2 3 + x +
+ ) (x−2)(2+ 5x−6 2 3 2 2 3 2 4 )     3 5 3 5 − lim   = − = + = −1. x→2  + −  3 (3x + 2)2 3 2 5x 6 12 4 + 2 3x + 2 + 4   3 2
2x + 4x +11 − x + 7 16) I = lim . 2 x→2 x − 4 3 2 3 2 2x 4x 11 3 3 x 7  2x 4x 11 3 3 x 7  + + − + − + + + − − + Ta có I = lim = lim +  2 2 2 x→2 x→2 x − 4  x − 4 x − 4     (    2
2x + 4x +11 − 3) ( 2 2x + 4x + )2 3 3 2 3 11
+ 3 2x + 4x +11 + 9 
 (3− x + 7 )(3+ x + 7 ) = lim  +  x→2  (   2 − + +  x − ) ( 2 x + x + )2 3 2 3 + x + x + + ( 2x 4)(3 x 7 4 2 4 11 3 2 4 11 9 )           2 
2x + 4x +11− 27 9 − ( x + 7) = lim  +  x→2 (   2 − + +  x − ) ( 2 x + x + )2 3 2 3 + x + x + + ( 2x 4)(3 x 7 4 2 4 11 3 2 4 11 9 )           2  2x + 4x −16 2 − x = lim  +  x→2 (   2 − + +  x − ) ( 2 x + x + )2 3 2 3 + x + x + + ( 2x 4)(3 x 7 4 2 4 11 3 2 4 11 9 )           2 ( x 2)( x 4)  − + 2 − x = lim  +  x→2 (   2 − + +  x − ) ( 2 x + x + )2 3 2 3 + x + x + + ( 2x 4)(3 x 7 4 2 4 11 3 2 4 11 9 )        67 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC    2 ( x 4)  + 1 12 1 − 5 = lim  −  = + = . x→2 (   + + +  x + 2) ( 2 2x + 4x + )2 3 2 x 2 3 x 7 108 24 72 3 ( ) 11
+ 3 2x + 4x +11 + 9 ( )        3 3 2
5 − x − x + 7 17) I = lim . 2 x 1 → x −1 3 3 2 3 3 2 5 x 2 2 x 7  5 x 2 2 x 7  − − + − + − − − + Ta có I = lim = lim +  2 2 2 x 1 → x 1 x −1 →  x −1 x −1     (  
− x − )( − x + ) ( 2 2 − x + 7 ) 2 4 + 2 x + 7 +  ( 2 3 3 3 x + 7 5 2 5 2 )2 3 3    = lim  +  x 1 →  ( 2x − )1( 3 5 − x + 2) (   2  x − ) 3 2 1 4 + 2 x + 7 +  ( 2 3 x + 7)2           − − 8 − +  x ( 2 3 x 7 5 4 ) = lim  +  x 1 → ( 2 x − ) 1 ( 3 5 − x + 2) (   2  x − ) 3 2 1 4 + 2 x + 7 +  ( 2 3 x + 7)2           3 2  1− x 1− x = lim  +  x 1 → ( 2 x − ) 1 ( 3 5 − x + 2) (   2  x − ) 3 2 1 4 + 2 x + 7 +  ( 2 3 x + 7)2         −  (  2 x + x + ) 1 1 −  3 1 − 11 = lim + = − + = − .   x 1 → (x + )( 3 − x + ) 3 2 4 + 2 x + 7 + ( 2 8 12 24 1 5 2 3 x + 7)2    3 3
3 4x − 24 + x + 2 − 8 2x − 3 18) I = lim . 2 x→2 4 − x 3 3
3 4x − 24 − 6 + x + 2 − 2 + 8 − 8 2x − 3 Ta có I = lim 2 x→2 4 − x 3 3 3 4x − 24 − 6 x + 2 − 2 8 − 8 2x − 3 = lim + lim + lim 2 2 2 x→2 x→2 x→2 4 − x 4 − x 4 − x 1 I I2 I3   3( 3 4x − 24 − 2) 2 3 3 3 3 3 2
 4x − 24 + 4x − 24.2 + 2  3 3 3 4x − 24 − 6   I = lim = lim 1 2 x→2 4 − x x→ (   2 4 − x ) 2 2 3 3 3 3 2
 4x − 24 + 4x − 24.2 + 2    68 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 3( 3 4x − 24 − 8) = lim x→ (   2 4 − x )( 3 4x − 24 )2 2 3 3 3 2 + 4x − 24.2 + 2    3.4( 3 x − 8) = lim x→ (   2 4 − x )( 3 4x − 24 )2 2 3 3 3 2 + 4x − 24.2 + 2    12( x − 2)( 2 x + 2x + 4) = lim x→ (  
2 − x)(2 + x)( 3 4x − 24 )2 2 3 3 3 2 + 4x − 24.2 + 2    1 − 2( 2 x + 2x + 4) = 144 lim = − = 3 − . x→ (   48 2 + x)( 3 4x − 24 )2 2 3 3 3 2 + 4x − 24.2 + 2   
( x+2 −2)( x+2 +2) x + 2 − 2 (x + 2−4) I = lim = lim = lim 2 2 x→2 4 − x x→2 ( 2
4 − x )( x + 2 + 2)
x→2 (2 − x)(2 + x)( x + 2 + 2) 1 − 1 = lim = − x→
(2+ x)( x+2 +2) 16
8(1− 2x − 3)(1+ 2x − 3) 8 − 8 2x − 3 8(1− (2x − 3)) I = lim = lim = lim 3 2 x→2 4 − x x→2 ( 2
4 − x )(1+ 2x − 3)
x→2 (2 + x)(2 − x) 8.2(2 − x) = 16 16 lim = lim = = 2
x→2 (2 − x)(2 + x)(1+ 2x + 3)
x→2 (2 + x)(1+ 1+ 2x −3) 8 1 17 I = 3 − − + 2 = − . 16 16 Bài 5.
Tính các giới hạn sau: 1 ( 3
lim 2x − 3x) ĐS: + 2. ( 3 2
lim x − 3x + 2) ĐS: − x→+ x→− 3. ( 3 2
lim −x − 6x + 9x + ) 1 ĐS: − 4. ( 3
lim −x + 3x − ) 1 ĐS: + x→+ x→− 5. ( 4 2 lim x − 2x + ) 1 ĐS: + 6. ( 4 2
lim x − 8x +10) ĐS: + x→+ x→− 7. ( 4 2
lim −x + 2x + ) 3 ĐS: − 8. ( 4 2
lim −x − x + 6) ĐS: − x→+ x→− 9. 2 lim
x − 3x + 4 ĐS: + 10. + + ĐS: + →− ( 2 lim 2x 1 x x ) x→ 11. + + + ĐS: − 12. + + − ĐS: + →+ ( 2 lim 4x x 1 x x ) →− ( 2 lim x x 1 2x x ) 13. lim + − + ĐS: − →+ ( x 1 9x 1) x 69 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 14. lim + + +
ĐS: không tồn tại giới hạn →− ( 16x 7 9x 3 ) x Lời giải 1. I = ( 3 lim 2x − 3x) x→+  3   3  Ta có I = ( 3 lim 2x − 3x) 3 = lim x 2 − = +   . (vì 3
lim x = + và lim 2 − = 2  0   ) x→+ 2 x→+  x x→+ 2 x→+  x  2. I = ( 3 2
lim x − 3x + 2) . x→−  3 2  Ta có I = ( 3 2
lim x − 3x + 2) 3 = lim x 1− + = −   . (vì 3 lim x = − và x→− 3 x→−  x x x→−  3 2  lim 1− + =1   ). 3 x→−  x x  3. I = ( 3 2
lim −x − 6x + 9x + ) 1 . x→+  6 9 1  Ta có 3 I = lim x 1 − − + + = −   . 2 3 x→+  x x x   6 9 1  (vì 3 lim x = + và lim 1 − − + + = 1 −  0   ). x→+ 2 3 x→+  x x x  4. I = ( 3
lim −x + 3x − ) 1 x→−  3 1   3 1  Ta có 3 I = lim x 1 − + − = +   . (vì 3 lim x = − và lim 1 − + − = 1 −  0   ). 2 3 x→−  x x x→− 2 3 x→−  x x  5. I = ( 4 2 lim x − 2x + ) 1 x→+  2 1   2 1  Ta có 4 I = lim x 1− + = +   . (vì 4
lim x = + và lim 1− + =1  0   ). 2 4 x→+  x x x→+ 2 4 x→+  x x  6. I = ( 4 2
lim x − 8x +10) x→−  8 10   8 10  Ta có 4 I = lim x 1− + =1  0   (vì 4
lim x = + và lim 1− + =1  0   ) 2 4 x→−  x x x→− 2 4 x→−  x x  7. I = ( 4 2
lim −x + 2x + ) 3 x→+  2 3   2 3  Ta có 4 I = lim x 1 − + + = −   . ( vì 4 lim x = + và lim 1 − + + = 1 −  0   ). 2 4 x→+  x x x→+ 2 4 x→+  x x  8. I = ( 4 2
lim −x − x + 6) x→−  1 6   1 6  Ta có 4 I = lim x 1 − − + = −   . (vì 4 lim x = + và lim 1 − − + = 1 −   ) 2 4 x→−  x x x→− 2 4 x→−  x x  9. 2 I = lim x − 3x + 4 . x→  3 4   3 4  Ta có 2 I = lim x 1− +   = lim x 1− + = +   . 2 x→  x x  2 x→  x x   3 4 
(vì lim x = + và lim 1− + = 1  0   ). x→ 2 x→  x x  10. I = + + →− ( 2 lim 2x 1 x x ) 70 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC  1  Ta có I = + + = lim x− 2 + +1 = + . →− ( 2 lim 2x 1 x   x ) 2 x→− x    1 
(vì lim x = − và lim  − 2 + +1 = − 2 +1 0   ). x→− 2 x→− x    1 1  11. I = + + + = lim x− 1+ + + 2 = − . →− ( 2 lim x x 1 2x   x ) 2 x→− x x    1 1 
(vì lim x = − , lim  − 1+ + + 2 =1 0   ). x→− 2 x→− x x    1 1  12. I = + + − = lim x 4 + + −1 = + →+ ( 2 lim 4x x 1 x   x ) 2 x→+ x x    1 1 
(vì lim x = + , = lim  4 + + −1 =1 0   ). x→+ 2 x→+ x x     13. I = lim + − + 1 1
= lim x  1+ − 9 +  = − . →+ ( x 1 9x 1)   x x→+ x x    1 1  (vì lim x = + , = lim x  1+ − 9 +  = −   ). x→+ x→+ x x   14. I = lim + + + →− ( 16x 7 9x 3 ) x  1 
Tập xác định của hàm số f ( x) = 16x + 7 + 9x + 3 là D = − ; +   .  3 
Ta có khi x → − hàm số f ( x) = 16x + 7 + 9x + 3 không xác định. Do đó lim + + + không tồn tại. →− ( 16x 7 9x 3 ) x Bài 6.
Tính các giới hạn sau: x + 2 2x 1. lim . ĐS: 1 2. lim . ĐS: 2 x→+ x −1 x→− x +1 1− x 3x − 2 3. lim .ĐS: 1 − 4. lim . ĐS: 3 x→+ 2x −1 2 x→− x +1 3 3x ( 2 2x − ) 2x + 3x − 4 1 5. lim . ĐS: −2 6. lim . ĐS: 6 3 2
x→+ −x − x +1
x→+ (5x − ) 1 ( 2 x + 2x) 5 4 3 ( 2 4x + ) 2x + 7x −15 1 (7x − ) 1 7. lim . ĐS: 2 8. lim . ĐS: 0 4 x→− x +1 x→+ ( 3 2x − ) 1 ( x + 3) ( 4 3 x − )2 1 (5x + 2)2 (x + ) 1 (1− 2x) 9. lim . ĐS: 25 10. lim .ĐS: 1 − 5 x→− (3x + )4 1 81
x→− (2x + 2) ( 2 x + 3) 4 (x +2)2 2 (x + 2)
(x + 2)3 (1− x)4 11. lim . ĐS: − 12. lim . ĐS: 1 − x→− ( 5 2x + ) 1 (1− x)2 2 x→− (1−2x) 2x 32 71 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 3 2  x x  2 3x − x + 7 13. lim  −  . ĐS: 2 14. lim .ĐS: 0 2
x→−  3x − 4 3x + 2  9 3 x→− 2x −1 3 ( 2 4x + ) x + 2x + 2 1 (7x − ) 1 15. lim ĐS: 0 16. lim ĐS: 0 4
x→+ 2x + x + 3 x→+ ( 3 2x − ) 1 ( x + 3) ( 2 4x + ) 1 (2x + 3) 3 x + 2x + 2 17. lim ĐS: − 18. lim ĐS: + 2 x→− x − 6x +1 2
x→+ 2x + x + 3 4 3
x + 2x + x + 2 4 3
x + 2x + x + 2 19. lim ĐS: − 20. lim ĐS: − 3 x→− 2x + x + 3 2 3 x→+ 2x − x 4 3 x − x +11 4 2 2x + x −1 21. lim ĐS: + 22. lim ĐS: + x→+ 2x − 7 x→+ 1− 2x 4 x − x 5 3 2x + x −1 23. lim ĐS: + 24. lim 3 ĐS: 1 x→+ 1− 2x x→+ ( 2 2x − ) 1 ( 3 x + x) 3 3 x + x +1 4 2 2x + x −1 25. lim ĐS: 1 26. lim ĐS: − x→+ 2x +1 x→+ 1− 2x Lời giải  2   2  x 1+   1+   x + 2  x   x  1. I = lim = lim = lim =1. x→+ x −1 x→+  1  x→+  1  x 1−   1−    x   x  2x 2x 2 2. I = lim lim = lim = 2 .
x→− x +1 x→−  1  x→− 1 x 1+   1+  x x  1  x −1   1 −1 1− xx  1 3. I = lim = lim = lim x = − . x→+ 2x −1 x→+  1  x→+ 1 2 x 2 −   2 −  x x  2   2  x 3 −   3 −   3x − 2  x   x  4. I = lim = lim = lim = 3. x→− x +1 x→−  1  x→−  1  x 1+   1+    x   x   3 4   3 4  3 x 2 + −   2 + −   3 2x + 3x − 4 2 3  x x  2 3  x x  5. I = lim = lim lim = 2 − . 3 2
x→+ −x − x +1 x→+  1 1  x→+  1 1  3 x 1 − − +   1 − − +   3  x x  3  x x   1   1  2 3 . x x 2 −   3 2 −   2  x  2  x  6 6. I = lim = lim = . x→+  1   2  x→+  1   2  2 − + 5 − + x 5 x 1     5 1     x   x   x  x  72 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC  7 15   7 15  4 x 2 + −   2 + −   4 3 2x + 7x −15 4  x x  4  x x  7. I = lim = lim = lim = 2 . 4 x→− x +1 x→−  1  x→−  1  4 x 1+   1+   4  x  4  x  (  1   1   1   1  2 + − + − 2 x 4 x 7 4 x 7 4x + ) 1 (7x − ) 1         2  x   x  2  x   x  8. I = lim = lim = lim = 0. x→+ ( 3 2x − ) 1 ( x + 3) x→+  1   3  x→+  1   3  3 x 2 − x 1+     2 − x 1+     3  x   x  3  x   x  2 2  2 2 1   2   1   2  ( 2 2 x 1− x 5 +     1− 5 +     x − )2 1 (5x + 2)2  x   x   x   x  25 9. I = lim = lim = lim = . x→− ( 4 4 3x + )4 1 x→−  1  x→−  1  81 4 x 3 +   3 +    x   x  4 3  4 3 1   1   1   1  ( 4 3 x 1+ x − 2     1+ − 2     x + )4 1 (1− 2x)3  x   x   x   x  1 10. I = lim = lim = lim = − . x→− ( 5 5 2x + 2)5 ( 2 x + 3) x→−  2   3  x→−  2   3  4 5 2 x 2 + x 1+     2 + 1+     2  x   x  2  x   x  2 2 (  2   2   2   2  4 + + + + x + 2)2 2 (x + 2) x 1 x 1     . x 1 1     2  x   x  2  x   x  11. I = lim = lim = lim = − x→− ( 2 2 2x + ) 1 (1− x)2 2 x→−  1   1  x→−  1  1  2 2 x 2 + x −1     2 + −1    2  x   x  2  x  x  2  2   2  1+ 1+     2  x   x  1
(vì lim x = − , lim =  0 ). x→− 2 x→−  1  1  2 2 + −1    2  x  x  3 4  3 4 2   1   2   1  ( 3 4 x 1+ x −1     1+ −1    
x + 2)3 (1− x)4  x   x   x   x  1 12. I = lim = lim = lim = − . x→− ( 5 5 1− 2x)5 2 x x→−  1  x→−  1  32 5 2 x − 2 .x   − 2    x   x  3 2  x x  13. I = lim  −  . 2
x→−  3x − 4 3x + 2  3 2  3 2 2 x x
x (3x + 2) − x (3x − 4) 3 2 2x + 4x Ta có I = lim  −  = lim = lim 2
x→−  3x − 4 3x + 2  x→− ( 2
3x − 4)(3x + 2) x→− ( 2
3x − 4)(3x + 2)  4   4  3 x 2 +   2 +    x  =  x  2 lim = lim = . x→−  4   2  x→−  4  2  2 9 x 3 − x 3 +     3 − 3 +     x   x   x  x   3 1 7   3 1 7  2 x − +   − +   2 3x − x + 7 2  x x x  2 =  x x x  = 14. I = lim lim lim 0 . 3 x→− 2x −1 x→−  1  x→−  1  3 x 2 −   2 −   3 3 73  x   x  Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC Bài 7.
Tính các giới hạn sau: 2x − 3 x −15 1. lim . ĐS: − 2. lim . ĐS: − + + x 1 → x −1 x→2 x − 2 2 − x x − 5 3. lim . ĐS: + 4. lim . ĐS: − − − x 3 → 3 − x x→ (x − 4)2 4 3 − x +1 3x −1 5. lim . ĐS: + 6. lim . ĐS: − − − x→2 x − 2 x 1 → x −1 6 − 5x x +1 7. lim . ĐS: − 8. lim . ĐS: + + + x→2 4x − 8 x→2 2x − 4 x − 3 7x −1 9. lim . ĐS: 1 10. lim .ĐS: − + − x 3 → 5x −15 5 x ( → − ) 3 x + 3 2 − x x −1 11. lim . ĐS: 1 12. lim . ĐS: 1 − 2 + 3 x→2 2x − 5x + 2 3 x 1 → 2x + x − 3 7 2 x −1 x − 3x + 2 13. lim . ĐS: 1 − 14. lim .ĐS: 1 − 3 + x 1 → 2x + x − 3 7 x→2 x − 2 2 x − 9 x − 4 15. lim lim ĐS: không tồn tại x→3 x − ĐS: không tồn tại 16. 3 2
x→4 x + x − 20 x − 2 x − 3 17. lim ĐS: 2 18. lim ĐS: 4 − − − x→2 x −1 −1 x 3 → 5x −11 − 2 5 2 x − 2 x − 25 19. lim ĐS: 3 − 20. lim ĐS: 30 − − 3 − x→2 x −1 −1 3 x→5 x − 4 −1 3x − 8 3 x + 25 − 3 21. lim ĐS: + 22. lim ĐS: 1 + 2 x→ (3− x)2 3 x→2 x − x − 2 81 3x + 2 x + x 23. lim ĐS: + 24. lim ĐS: 1 − + → 2 + x 2 4x −16 x→0 x − x 2 4 − x x + 2 x 25. lim ĐS: 0 26. lim ĐS: −2 + + x→2 2 − x x→0 x − x (x + 2)(x + ) 1 2 x − 6x + 9 27. lim ĐS: 1 28. lim ĐS: 1 − + − 2 x→(− ) 1 x +1 − x −1 x 3 → x − 9 6 2 x − 4x + 3 2 x + 3x + 2 29. lim ĐS: − 30. lim ĐS: 0 − 2 + x 1 → −x + 6x − 5 x→(− ) 5 4 1 x + x x x 31. lim ( x − 2) lim + + ( 3 x )1 + 2 2 x→2 x − ĐS: 0 32. 4 x ( → − ) 1 x − ĐS: 0 1 x + 5 x 1− x 33. lim (1− x) lim ĐS: 1 + 2 − x 1 → x + 2x − ĐS: 0 34. 3 x 1 → 2 1− x +1− x 2 2  1− x  2x + 5x − 3 35. lim  2x  ĐS: 0 36. lim ĐS: − +   + 2 x→0 x x ( → 3 − ) (x +3) 74   Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC  1 1  3 x − 3x + 2 37. lim −   ĐS: − 38. lim ĐS: 3 − − 2 − 2
x→2  x − 2 x − 4  x 1 → x − 5x + 4 5 Lời giải lim (2x −3) = 1 − + x 1 →  2x − 3  1 lim
= − vì lim (x − ) 1 = 0 . + + x 1 → x −1 x 1 → 
x −1  0, x  →1+  lim (x −15) = 13 − + x→2  x −15  2. lim
= − vì lim (x − 2) = 0 . + + x→2 x − 2 x→2 
x − 2  0, x  → 2+  lim (2 − x) = −1 − x→3  2 − x  3. lim
= + vì lim (3− x) = 0 . − − x 3 → 3 − x x→3  3
 − x  0, x  → 3−  lim (x −5) = 1 − − x→4  x − 5  2 4. lim
= − vì lim (x − 4) = 0 . − − x→ (x −4)2 4 x→4 (x−4  )2  0, x  → 4− lim ( 3 − x + ) 1 = 5 − − x→2  3 − x +1  5. lim
= + vì lim (x − 2) = 0 . − − x→2 x − 2 x→2 
x − 2  0, x  → 2−  lim (3x − ) 1 = 2 − x 1 →  3x −1  6. lim
= − vì lim (x − ) 1 = 0 . − − x 1 → x −1 x 1 → 
x −1 0, x  →1−  lim (6 − 5x) = 4 − + x→2  6 − 5x  7. lim
= − vì lim (4x −8) = 0 . + + x→2 4x − 8 x→2 
4x − 8  0, x  → 2+   lim (x + ) 1 = 3 + x→2  x +1  8. lim
= + vì lim (2x − 4) = 0 . + + x→2 2x − 4 x→2 
2x − 4  0, x  → 2+  x − 3 x − 3 1 1 9. Do x 3+
→ nên x − 3 = x − 3 suy ra lim = lim = lim = . + + + x 3 → − x 3 5x 15 → 5x −15 x 3 → 5 5  lim (7x − ) 1 = 22 − − x→( 3−) 7x −1  10. lim = −  lim x + 3 = 0 . − − x ( → − ) 3 x + vì 3 x→( 3 − )  −
x +3  0, x  →  (−3) 75 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 2 − x 2 − x 11. Do x 2−
→ nên 2 − x = 2 − x suy ra lim = lim − 2 − x→2 − + x→2 2x 5x 2 (2x − ) 1 ( x − 2) 1 1 = lim = . − x→2 2x −1 3 x −1 x −1 12. Do x 1+
→ nên x −1 = x −1 suy ra lim = lim + 3 + x→ 2x + x − 3 x→ (x − ) 1 ( 2 1 1 2x + 2x + 3) 1 1 lim = . + 2 x 1 → 2x + 2x + 3 7 x −1 1− x 13. Do x 1−
→ nên x −1 =1− x suy ra lim = lim − 3 − x→ 2x + x − 3 x→ (x − ) 1 ( 2 1 1 2x + 2x + 3) 1 − 1 lim = − . − 2 x 1 → 2x + 2x + 3 7 14. Ta có 2
x − 3x + 2 = ( x − )
1 ( x − 2) , do x 2+ → nên 2
x − 3x + 2  0 , suy ra 2 x − 3x + 2 (x − ) 1 ( x − 2) lim = lim = lim (x − ) 1 = 1 + + + x→2 − x→2 − . x→2 x 2 x 2 2 x − 9 (x +3)(x −3) 15. Ta có lim = lim x→3 x→3 x − 3 x − 3 2 x − 9 (x +3)(x −3) TH1: x  3 ta có lim = lim = lim (x + 3) = 6 + + + x→3 − x→3 − . x→3 x 3 x 3 2 x − 9
−(x + 3)(x − 3) TH2: x  3 ta có lim = lim = lim (−x −3) = 6 − − − − x→3 − x→3 − . x→3 x 3 x 3 2 2 x − 9 x − 9 2 x − 9 Do lim  lim lim + − x→3 − x→3 x 3 x − nên không tồn tại 3 x→3 x − . 3 x − 4 x − 4 16. Ta có lim = lim 2 x→4 x→4 x + x − 20 (x − 4)(x +5) x − 4 x − 4 1 1
TH1: x  4 , ta có lim = lim = lim = + 2 + + x→4 x→4 x + x − 20
(x −4)(x +5) x→4 x +5 9 x − 4 x − 4 1 − 1 −
TH2: x  4 , ta có lim = lim = lim = + 2 − − x→4 x→4 x + x − 20
(4− x)(x +5) x→4 x +5 9 x − 4 x − 4 x − 4 Do lim  lim nên không tồn tại lim . + 2 − x→4 x + x − 20 2 x→4 x + x − 20 2
x→4 x + x − 20 x − 2
(x − 2)( x−1+ )1 17. Do x 2−
→ nên x − 2 = 2 − x suy ra lim = lim − − x→2 x −1 −1 x→2 x −1−1 lim − + = . − ( x 1 )1 2 x→2 x − 3
(3− x)( 5x−11+2) 18. Do x 3−
→ nên x − 2 = 3− x suy ra lim = lim − − x 3 → 5x −11 − 2 x 3 → 5x −11− 4 −( 5x −11+ 2) 4 = lim = − . − x 3 → 5 5 76 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC (2− x)( 2 3 3 x −1 + x −1 + ) x − 2 1 19. Do x 2−
→ nên x − 2 = 2 − x suy ra lim = lim − 3 − x→2 x −1 −1 x→2 x −1−1 = lim − − + − + = − . − → ( ( 2 3 3 x 1 x 1 1 3 x 2 ) 2 x − 25 20. Ta có 2
x − 25 = ( x − 5)( x + 5) , do x 5− → nên 2
x − 25  0 , suy ra lim− 3 x→5 x − 4 −1 (25− x )( 2 2 3 3 x − 4 + x − 4 + ) 1 = 2 lim = lim − + − + − + = − . − ( (5 x)) → (3 3 x 4 x 4 1 30 x 5 ) − x→5 x − 4 −1 lim (3x −8) =1 + x→3  3x − 8  2 21. lim
= + , vì lim (3− x) = 0 . + + x→ (3− x)2 3 x→3 (3−x  )2  0, x  → 3+ 3 x + 25 − 3 x + 25 − 27 22. Ta có lim = lim 2 x→2 x − x − 2 x→ (x −2)(x + ) 1 ( 2 2 3 3
x + 25 + 3 x + 25 + 9) 1 1 = lim = . x→ (x + )( 2 2 3 3 x + + x + + ) 81 1 25 3 25 9 lim (3x + 2) = 8 + x→2  3x + 2  23. lim = + , vì 2 lim 4x −16 = 0 . + → 2 + x 2 4x −16 x→2  2
 4x −16  0, x  → 2+  x ( x + ) x + x 1 x +1 24. lim = lim = lim = 1 − . + + + x→0 x − x x→0
x ( x − ) x→0 1 x −1 2 4 − x (2− x)(2+ x) 25. lim = lim
= lim 2 − x (2 + x) = 0 . + + + x→2 2 − x x→2 x→2 2 − x x ( x + 2) x + 2 x x + 2 26. lim = lim = lim = 2 − . + + + x→0 x − x x→0
x ( x − ) x→0 1 x −1 (x + 2)(x + ) 1 x + 2 x +1 x + 2 27. Ta có lim = lim = lim =1. + + + x→(− ) 1 x +1 − x −1 x→(− ) 1
x +1 (1− x +1) x→(− )1 1− x +1 28. Ta có − x − x + = (x − )2 2 6 9 3
= x −3 , do x → 3 nên 2
x − 6x + 9 = 3 − x , suy ra 2 x − 6x + 9 2 x − 6x + 9 3 − x 1 − 1 lim = lim = lim = lim = − − 2 − 2 − − x 3 → x − 9 x 3 → − x 3 x 9 →
(x −3)(x +3) x 3 → x + . 3 6 29. Do x 1−
→ nên x −1 0 , từ đó ta có 2 x − 4x + 3
(x − )1(x −3) 1− x 3 − x 3 − x lim = lim = lim = lim − 2 − − − x 1 → −x + 6x − 5 x 1 → −(x − ) 1 ( x − 5) x 1 → −(x − ) 1 ( x − 5) x 1 →
1− x ( x − 5)  1 3 − x  = lim  .  = − . −   x 1 → 1− x x − 5   77 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 3 − x 2  1  vì lim = − và lim = +   . − − x 1 → x − 5 4 x 1 →  1− x  2 x + 3x + 2 (x + )1(x + 2) x +1 ( x + 2) 30. lim = lim = lim = 0 . + + 2 + 2 x→(− ) 5 4 1 x + x x ( → − ) 1 x x +1 x→(− ) 1 x x x x ( x − 2) 31. lim ( x − 2) = lim (x − 2) = lim = 0 + 2 + + x→2 x − 4 x→2
(x − 2)(x + 2) x→2 x + . 2 x x 32. Ta có lim + = lim (x + ) 1 − + + ( 2x x )1 + ( 3 x )1 x ( → − ) 2 1 x −1 x ( → − ) 1 (x − ) 1 ( x + ) 1 x x + = lim − + = . + ( 1 2 x x ) ( ) 1 0 x ( → − ) 1 x −1 33. Do x 1+
→ nên 1− x  0 , vì thế ta có 2    + −  ( + (x +5)(x − ) (x 5)(x ) − 1 1 x) x 5 lim 1 = lim   = lim   = 0 . + 2 + + x 1 → x + 2x − 3 x 1 →  (x − ) 1 ( x + 3)  →  +    x 1 x 3   x 1− x x 1− x x 1 34. lim = lim = lim = . − − − x 1 → x 1 2 1− x +1− x →
1− x (2 + 1− x ) x 1 → 2 + 1− x 2  2   1− x x (1− x) 35. lim  2x  = lim  2
 = lim 2 x(1− x) = 0. +   + + x→0 x   x→0  x    x→0 2 2x + 5x − 3 (2x − ) 1 ( x + 3) 2x −1 36. lim = lim = lim = − . + + 2 + x ( → − ) (x +3)2 3 x ( → 3 − ) (x +3) x ( → 3 − ) x + 3  1 1   x + 2 −1   x +1 1  37. lim −   = lim   = lim . = −   . − 2 −   −
x→2  x − 2 x − 4 
x→2  ( x − 2)( x + 2) 
x→2  x + 2 x − 2  38. Do x 1−
→ nên x −1 0 , suy ra (x − )2 1
= x −1 =1− x nên ta có 2 3 x − 3x + 2 (x + 2)(x − ) 1 (1− x) x + 2 − x + 2 3 lim = lim = lim = lim = − . − 2 − − − x 1 → x − 5x + 4 x 1 → (x − ) 1 ( x + 4) x 1 → ( x − ) 1 ( x + 4) x 1 → x + 4 5 Bài 8.
Tính các giới hạn sau: sin 5x tan 2x 1) lim . ĐS: 5 2) lim . ĐS: 2 x 0 → x x 0 → 3x 3 1− cos x sin 5 . x sin 3 . x sin x 3) lim . ĐS: 1 4) lim . ĐS: 1 2 x 0 → x 2 3 x 0 → 45x 3 1− cos 5x 2 1− cos 2x 5) lim 6) lim . ĐS: 4 x 0 → 1− cos3x x 0 → . x sin x x sin ax 1− cos ax 2 a 7) lim (a  0). ĐS: 2 8) lim . ĐS: x 0 → 1− cos ax a x 0 → 1− cosbx 2 b 1− cos x 2 a sin x − tan x 9) lim ; a  0 ĐS: 10) lim ĐS: 1 − 2 ( ) x 0 → x 2 3 x 0 → x 2 78 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC tan x − sin x sin x − sin a 11) lim . ĐS: 1 12) lim . ĐS: cos a 3 x 0 → sin x 2 x→a x − a cos x − cos b 1− 2x +1 − 13) lim ĐS: −sin b 14) lim ĐS: 1 x b → x − b x→0 sin 2x 2
cos (a + x) − cos(a − x) tan x − tan c 1 15) lim ĐS: 2 − sin a 16) lim ĐS: x 0 → x x c → x − c 2 cos c 3 1− cos x 2 2 sin x − sin a a 17) lim ĐS: 3 18) lim ĐS: sin 2 x→0 x sin x 2 2 2 x→a x − a 2a
cos x − cos  x 2 2  − 3 x + 8 19) lim ĐS: 20) lim ĐS:12 2 x 0 → x 2 x 2 →− tan ( x + 2)
1− cos x cos 2x cos 3x
sin (a + 2x) − 2sin (a + x) + sin a 21) lim . ĐS:1422) lim . ĐS: − sin ( ) x 0 → 1− cos x 2 x 0 → x sin ax + tan bx
cos 3x − cos 5x cos 7x 23) lim
;(a + b  0) ĐS: 1 24) lim ĐS: 33 − x→0 (a + b)x 2 x 0 → x 2
cos ax − cos bx cos cx 2 2 2
b − a − c
sin (a + x) − sin (a − x) 25) lim . ĐS: 26) lim ĐS: 3 cos a x 0 → 1− cos x 2
x→0 tan(a + x) − tan(a − x) 3 2 2x +1 − x +1 2
sin 2x − sin x sin 4x 27) lim . ĐS: 1 28) lim ĐS: 6 x→0 sin x 4 x 0 → x cos x sin x − sin 2x 29) lim . ĐS: 1 30) lim ĐS: -1  →−  →   x + x 0 x 2 2 x x 1− sin   2  2  2 1+ x − cos x
1+ tan x − 1+ sin x 31) lim . ĐS: 1 32) lim ĐS: 1 2 x→0 x 3 x→0 x 4
1− cos 5x cos 7x x + 3 − 2 33) lim . ĐS: 37 34) lim 2 x 0 → sin 11x 121 x 1 → tan(x − ĐS: 1 1) 4 1+ cos x sin(x −1) 35) lim ĐS: 1 36) lim ĐS: 1 − x  → ( 2 x −  ) . 2 2 x 1 → x − 4x + 3 2 2 x +1 − cos 2x
1− cos x cos 2x 37) lim . ĐS: 5 38) lim ĐS: 3 2 x→0 x 2 2 x→0 x 2 Lời giải. sin 5x  sin 5x  1) lim = lim 5 = 5   . x→0 x→0 x  5x  tan 2x  2 tan 2x  2 2) lim = lim  = .   x→0 x→0 79 3x  3 2x  3 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC 2   x     2   2 sin x      sin  1− cos x  2  1   1 3) 2 lim = lim  = lim 2    = . 2 2 x→0 x→0 x→0 xx   4 x  2        2     
sin 5x sin 3x sin x
 1 sin 5x sin 3x sin x  1 4) lim = lim    =   . 3 x→0 x→0 45x  3 5x 3x x  3 2 2 5x  5x 3x      2 2sin  sin  1− cos 5x 25   4   25 5) 2 2 2 lim = lim = lim        = . x→0 x→0 1− cos 3x 3x x→0  4 5x 9 3x 2  9 2sin    sin    2  2   2    2 2 2 1− cos 2x sin 2x 4sin x cos x  sin x  6) 2 lim = lim = lim = lim 4cos x = 4   . x→0 x→0 x→0 x→0 x sin x x sin x xx  2  ax      x sin ax x sin ax 1 sin ax 4   2 7) 2 lim = lim = lim  a       = . 2 x→0 x→0 − ax ax x→0 1 cos  ax a ax 2 2  a 2sin  sin    2  2    2 2 axax bx      2 2sin  2 sin  2 1− cos ax a   4   a 8) 2 2 2 lim = lim = lim        = . 2 2 x→0 x→0 − bx bx x→0 1 cos  ax b bx 2 4  b 2sin    sin    2  2   2    2 axax    2 2sin  2 sin  2 1− cos ax a   a 9) 2 2 lim = lim = lim 2     = . 2 2 x→0 x→0 x→0 x x  4 ax  2      2    sin x − tan x
sin x (cos x − ) 1 10) Ta có lim = lim 3 3 x 0 → x 0 x → x cos x 2 xx    2 2 − sin xsin  sin  2 sin x 1   1 2 2 = lim = lim −       = − . 3 x→0 x→0 x cos x  cos x x 4 x  2      2    tan x − sin x
sin x (1− cos x)  1  1 11) lim = lim = lim  = . 3   x→ sin x x
→ cos x sin x ( 2 0 0 1− cos x) x→0 cos x  (1+ cos x) 2  x + a x − ax − a  2 cos sin sin sin x − sin ax + a  12) Ta có 2 2 lim = lim 2 = limcos   = cos . a x→a − − → x − a x→a x a x a x a 2    2  80 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC x + b x − bx − b  2 − sin sin sin cos x − cos bx + b  13) Ta có 2 2 lim = lim 2 = lim−sin   = −sin . b x→b x→b x − b x − b x→b 2 x − b    2  1− 2x +1 1− 2x −1  1 2x  1 14) Ta có lim = lim = lim −  = − .   x→0 x→0 sin 2x
sin 2x (1+ 2x +1)
x→0  1+ 2x +1 sin 2x  2
a + x + a − x
a + x − a + x 2 − sin sin
cos(a + x) − cos(a − x) 15) Ta có 2 2 lim = lim x→0 x→0 x x  sin x  = lim 2 − sin a  = 2 − sin . a   x→0  x  tan x − tan c sin ( x − c)
 sin (x − c) 1  1 16) lim = lim =    = x→c x→c x − c (x −c) lim 2 cos x cos x→c c x − c cos x cos c cos . c   − (1−cos x)( 2 3 1+ cos x + cos 1 cos x x ) 17) Ta có lim = lim x→0 x→0 x sin x x sin x xx  2 2sin ( 2
1+ cos x + cos x) 2 sin
1+ cos x + cos x 1  3 2 2 = lim = lim    = . x→0 x x x→0 x 2 x 2 2x sin cos  cos  2 2  2 2  1− cos 2x 1− cos 2a − 2 2 sin x − sin a 18) Ta có 2 2 lim = lim 2 2 x→a x→a x − a
(x −a)(x + a)
cos 2a − cos 2x 2
− sin (a + x)sin(a − x) = lim = lim
x→a 2(x − a)(x + a) x→a
2(x − a)(x + a)
 sin(a + x) sin(a − x)  sin 2a = lim  = .   x→a x + a a − x  2a
( +  ) x ( −  ) x 2 − sin sin
cos x − cos  x 19) Ta có 2 2 lim = lim 2 2 x→0 x→0 x x  ( +  ) x ( −  ) x   sin sin 2 2    + 2  −  2  − = lim 2 −      = . x→0  2 ( +  ) x 2 ( −  ) x  2    2 2  x + 8 (x + 2)( 2 3 x − 2x + 4)  x + 2  20) Ta có lim = lim = lim ( 2 x − 2x + 4) ( )   =12. x→ 2
− tan ( x + 2) x→ 2 − x→ 2 tan(x + 2) − tan(x + 2)  
1− cos x cos 2x cos 3x
1− cos x + cos x(1− cos 2 )
x + cos x cos 2 ( x 1− cos 3 ) x 21) Ta có lim = lim 81 x 0 → x 0 1− cos x → 1− cos x Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC  3x  2 2 2sin  2sin x  2 = lim1+ cos x + cos x cos 2xx→0 x x 2 2  2sin 2sin   2 2  2 2 2  x 3x x        2  sin   sin x        2 2 2 = lim1+ 4cos x  
 + 9cos x cos 2x      =1+ 4 + 9 =14. x→0   x x 3x x    sin   sin   sin     2   2   2   
sin (a + 2x) − 2sin(a + x) + sin a
2sin (a + x)cos x − 2sin (a + x) 22) Ta có lim = lim 2 2 x 0 → x 0 x → x x 4
− sin (a + x) 2 sin
2 sin(a + x)(cos x −1) 2 = lim = lim 2 2 x→0 x→0 x x 2  x     sin  = − (    a + x) 1 2 lim 4 sin      = −sin . a x→0  4 x       2    sin ax tan bx sin ax tan bx ax  + bxa  b sin ax + tan bx a + b 23) Ta có lim = lim ax bx = lim ax bx = =1. x→0 x→0 x→0 (a + b)x (a + b)x a + b a + b
cos 3x − cos 5x cos 7x
cos 3x − cos 5x + cos 5x − cos 5x cos 7x 24) Ta có lim = lim 2 2 x 0 → x 0 x → x 7x 2
2 sin 4x sin x − 2 cos 5x sin
2 sin 4x sin x + cos 5x(1− cos 7x) 2 = lim = lim 2 2 x→0 x→0 x x 2  7x     sin  sin 4x sin x 49   49 33 2 = lim8  − 2 cos5x     = 8 − = − . x→0  4x x 4 7x  2 2      2   
cos ax − cos bx cos cx
cos ax − cos bx + cos bx − cos bx cos cx 25) Ta có lim = lim 2 2 x 0 → x 0 x → x (a + b)x (b−a)x (a + b)x (b−a)x cx 2 2sin sin
+ cosbx(1− coscx) 2sin sin − 2cosbxsin 2 2 2 2 2 = lim = lim 2 2 x→0 x→0 x x  (a + b)x (b − a) 2 xcx    2 2 sin sin 2 sin  2 2 2 2 2 2 b − a   2 2 c 2 b − a c
b − a − c = lim 2   − 2 cosbx     = − = . x→0  4 (a + b)x
(b − a) x 4 cx  2 2 2     2  2  2   82 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
sin(a + x) − sin(a − x) 2 cos a sin x 26) Ta có lim = lim
x→0 tan (a + x) − tan (a − x) x→0 sin 2x
cos (a + x) cos (a − x)
cos a cos (a + x)cos(a − x) 3 = lim = cos a x 0 → cos x 3 2 3 2 2x +1 − x +1
2x +1 −1+1− x +1 27) Ta có lim = lim x→0 x→0 cos x sin x 2 2x −x + 2x +1 +1 2 1+ x +1 + ( 2 x +1)2 3 3 = lim x→0 sin x 2x x − 2x +1 +1 2 1+ x +1 + ( 2 x +1)2 3 3 = lim =1. x→0 sin x x 2
sin 2x − sin x sin 4x
sin 2x (sin 2x − 2sin x cos 2x) 28) Ta có lim = lim 4 4 x 0 → x 0 x → x 3x x x x ( x − x)
4 sin 2x sin x sin sin 2 sin 2 sin cos cos 2 2 2 = lim = lim 4 4 x→0 x→0 x x  3x x  sin sin
 3 sin 2x sin x  2 2 = lim4      = 6. x→0 2 2x x 3x x    2 2     sin x +   cos x  2  29) lim = lim =1.  →−   →−  x x + + 2 x 2 x 2 2 sin x − sin 2x
sin x (1− 2cos x)
 sin x 1− 2cos x  30) lim = lim = lim  = 1 −   . x→0 x→0 x→0  x  2 x cos xx cos xx 1− 2sin    2  2 2 1+ x − cos x
1+ x −1+1− cos x 2
 1 x 1 1 cos x  + − − 31) Ta có lim = lim = lim +  2 2 x→0 x→0 x x 2 2 x→0  x x     2 x   x    2 2 2sin   sin  1+ x −1    = 1 1 1 1  +  2 = lim +     = + = 1 . x→  ( → 1+ + ) 2 lim 2 0 2 2 1 x x xx 0 2  + + 2 x  2 x 1 2 1        2    83 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC
1+ tan x − 1+ sin x
1+ tan x −1− sin x 32) lim = lim 3 x→0 x→0 3 x
x ( 1+ tan x + 1+ sin x ) x 2
sin x (1− cos x) 2sin x sin = lim 2 = lim x→0 3
x cos x ( 1+ tan x + 1+ sin x ) x→0 3
x cos x ( 1+ tan x + 1+ sin x ) 2  x     sin  2 sin x 1   1 2 = lim       = .
x→0  cos x ( 1+ tan x + 1+ sin x ) x 4 x  4      2     5x 7x  2 2 2sin 2 cos 5x sin
1− cos 5x cos 7x
1− cos 5x + cos 5x (1− cos 7x)   33) lim = lim 2 2 = lim +  2 2 2 2 x 0 → x 0 sin 11x → sin 11x x→0 sin 11x sin 11x     2 2  5x 7x      2 2  sin sin  25    11x  49    11 2 2 x  = 25 49 37 lim    + cos 5x         = + = . x→0  242 5x  sin11x  242 7x  sin11x       242 242 121    2   2    x + 3 − 2 x + 3 − 4  1 x −1  1 34) lim = lim = lim   =   . x 1 → tan ( x − ) x 1 1 → tan ( x − ) 1 ( x + 3 + 2) x 1 → x + 3 + 2 tan  (x − ) 1 4  2   x   − x     − x   2 2 2 cos 2sin    sin      1+ cos x  2  1   2  1  35) 2 lim = = lim = lim   = . 2 x  → ( → →  −   −  x − ) lim 2 x   → (x − )2 x  (  ) x  2 x x  2      2      sin ( x − ) 1 sin ( x − ) 1  sin (x − ) 1 1  1 36) lim = lim = lim .  = − . 2 x 1 → x 1 x − 4x + 3 → ( x − ) 1 ( x − 3) x 1 → x −1 x − 3 2   2 2 x +1 − cos 2x
x +1 −1+1− cos 2x 37) lim = lim 2 2 x→0 x→0 x x   2 2 2
x 1 1 1 cos2x  + − −  x +1−1 2sin x  = lim +  = lim + 2 2     x→ x x x →     ( +1+ ) 2 0 0 2 2 1 x x x   2  1 sin x    1 5 = lim + 2   = + 2 =   . x→0 2  + +  x  2 2 x 1 1 
1− cos x + cos x − (1− cos2 1 cos cos 2 x x x ) 38) lim = lim 2 2 x→0 x→0 x x 84 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC x   −  1− cos x x ( x ) 2 2sin cos 1 cos 2 
cos x (1− cos 2x)    2 = lim + = lim +  2 2 2 x→0   x→0 2 x x x
x (1+ cos 2x )      2   x    x   2 sin   sin  1   1   sin x   1 3 2 2 = lim   = lim       = +1 = x→0 2 2 
x (1+ cos 2x ) x→0  2
1+ cos 2x x   2 2              Bài 9.
Tính các giới hạn sau: cos 3x − cos x 1− 2sin x 1) lim ĐS: 1 2) lim ĐS: 1 x 0
→ cos5x − cos x 3 2  − x→ 4 cos x 3 2 6
1+ sin 2x + cos 2x
sin 7x − sin 5x 3) lim ĐS: 24) lim ĐS: 2  x 0 → x→ cos x sin x 2 2 − 2 cos x 3 1− cos x 5) lim ĐS: 2 lim ĐS: 1  6) →    → x x 0 sin x 6 4 sin x −    4  3 sin x − cos x     7) lim ĐS: 2 − 8) lim tan 2 . x tan − x    ĐS: 1    x→ sin 3x 3 x→   4  3 4
cos 3x + 2 cos 2x + 2 3 tan x −1 9) lim ĐS: 2 3 10) lim ĐS: 1 −  2  − x→ sin 3x 3 x→ 2sin x 1 12 3 3 Lời giải sin x cos 3x − cos x 2 − sin 2xsin x sin x 1 1) lim = lim = lim = lim x = . x→0 x→0 x→0 x→0 cos 5x − cos x 2 − sin 3xsin 2x sin 3x sin 3x 3 3 3x 1− 2sin x 1− 2sin x 1 1 2) lim = lim = lim = . 2 2    − − + x→ 4 cos x 3 x→ 1 4sin x x→ 1 2sin x 2 6 6 6 2
1+ sin 2x + cos 2x 2 cos x + sin 2x 3) lim = lim
= lim (2cos x + 2sin x) = 2 .    x→ cos x x→ cos x x→ 2 2 2
sin 7x − sin 5x 2cos 6x sin x 4) lim = lim = lim2cos6x = 2 . x 0 → x 0 → x 0 sin x sin x → 85 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC  2     2 − cos x 2 cos − cos x   2 − 2 cos x 2    4  5) lim = lim = lim    →    →    →    x x x 4 sin x − 4 sin x − 4 sin x −        4   4   4    x    x    x  4 − sin + sin −     2sin +    8 2   8 2  =  8 2  lim = lim = 2   →       →    x x x x x 4 2 sin − cos −     4 cos −    2 8   2 8   2 8  1− cos x (1−cos x) 2 3 cos x 6) lim = lim 2 x→0 x→0 tan x   2 3 3 2
sin x  1+ cos x + cos x    2 cos x 1 = lim = x→0 (   + x) 3 3 2 6 1 cos
1+ cos x + cos x        2sin 3 x −      3         2sin x − sin 3 x −     
sin x − 3 cos x  3    3  2 7) lim = lim = = = − .      − +     → →   → − − − →     x x x ( x ) lim lim sin 3 sin 3 x x x 3 sin 3    3 3 3 3   3 3  sin 3 x −      3  3 −     3 x −    3     
 2 tan x 1− tan x  2 tan x 8) lim tan 2x  tan − x = lim  = lim =1     . 2         − + x→ 4 x→
1 tan x 1 tan x x→ (1+ tan x)2 4 4 4 3 2
cos 3x + 2 cos 2x + 2
4 cos x − 3cos x + 4 cos x 9) lim = lim 3   − x→ sin 3x x→ 3sin x 4sin x 3 3    ( cos x − cos 
(2cos x + 3)cos x 2 cos x − )
1 (2cos x + 3)cos x =  3  lim = lim   x→ 2sin x + 3
2sin x − 3 sin x x→ 3 3 ( )( ) 3  x   −sin + 
(2cos x + 3)cos x  2 6  2 3 = lim =  →    x x 3 3 cos + 
(2sin x + 3)sin x  2 6  tan x −1 (tan x − ) 2 3 1 cos x 10) lim = lim 2   −   x→ 2sin x 1 x→
(1−tan x).( tanx)2 2 3 3 + + 4 4 tan x 1   2 −cos x = 1 lim = − .  2   x→ (1+ tan x) 12 ( 3 tan x ) 3 + + 4 tan x 1   86 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !
Tài liệu tự học dành cho HS Lớp 11 GIỚI HẠN VÀ LIÊN TỤC Bài 10.
Tính các giới hạn sau: cos 4x −1
1+ sin 2x − cos 2x 1) lim ĐS: 0 2) lim ĐS: 1 − x 0 → sin 4x x 0
→ 1− sin 2x − cos 2x sin 2x 1− cos x 3) lim ĐS: 1 − 4) lim ĐS: 0 x 0
→ 1− sin 2x − cos 2x x 0 → sin x
sin 5x − sin 3x  1 1  5) lim ĐS: 2 6) lim −   ĐS: 0 x 0 → sin x x→0  sin x tan x     sin − x   2 sin x −1 2  6  2 3 7) lim ĐS: − 8) lim ĐS: 2  −  − x→ 2 cos x 1 4 x→ 1 2sin x 3 4 6    sin − x    4   2  9) lim ĐS: 2 lim − cot x   ĐS: 0  − 10) x→0 x→ 1 2 sin x  sin 2x  4 87 Page
Fb: ThayTrongDGL Tài liệu biên soạn và sưu tầm Chúc các em học tốt !