THE INTERNATIONAL UNIVERSITY (IU) Course: Stochastic Model
Department of Industrial System Engineering
FINAL EXAMINATION STOCHASTIC MODEL Duration: 120 minutes Head of Department of Student ID: Date: Industrial & Systems Lecturer: 3 Apr, 2018 Engineering Name: Assoc Prof. Ho Thanh Phong Phan Nguyen Ky Phuc INSTRUCTIONS:
1. This is an open book examination. No laptops, PDA.
2. Use of calculator is allowed; discussion and material transfer are strictly prohibited. Total
pages: 3 (including this page) PART I (100 %) Question 1: (20 pts)
There are 15 tennis balls in a box, of which 9 have not previously been used. Three of the balls are
randomly chosen, played with, and then returned to the box. Later, another 3 balls are randomly chosen from the box.
a) Find the probability that in the 1st pick, the number of balls that has never been used is 0,1,2,3.
b) Find the probability that none of these balls in the 2nd pick has ever been used. Question 2: (15 pts)
a) A gambler has a fair coin and a two-headed coin in his pocket. He selects one of the coins at random;
when he flips it, it shows heads. What is the probability that it is the fair coin? (b)
Suppose that he flips the same coin a second time and, again, it shows heads. Now what is the
probability that it is the fair coin? Stochastic Model – 3, Apr, 2018 1
THE INTERNATIONAL UNIVERSITY (IU) Course: Stochastic Model
Department of Industrial System Engineering (c)
Suppose that he flips the same coin a third time and it shows tails. Now what is the probability that it is the fair coin? Question 3: (15 pts)
A coin having probability .8 of landing on heads is flipped. A observes the result—either heads or tails—
and rushes off to tell B. However, with probability .4, A will have forgotten the result by the time he
reaches B. If A has forgotten, then, rather than admitting this to B, he is equally likely to tell B that the coin
landed on heads or that it landed tails. (If he does remember, then he tells B the correct result.
a) What is the probability that B is told that the coin landed on heads?
b) What is the probability that B is told the correct result?
c) Given that B is told that the coin landed on heads, what is the probability that it did in fact land on heads?
Question 4: (10 pts)
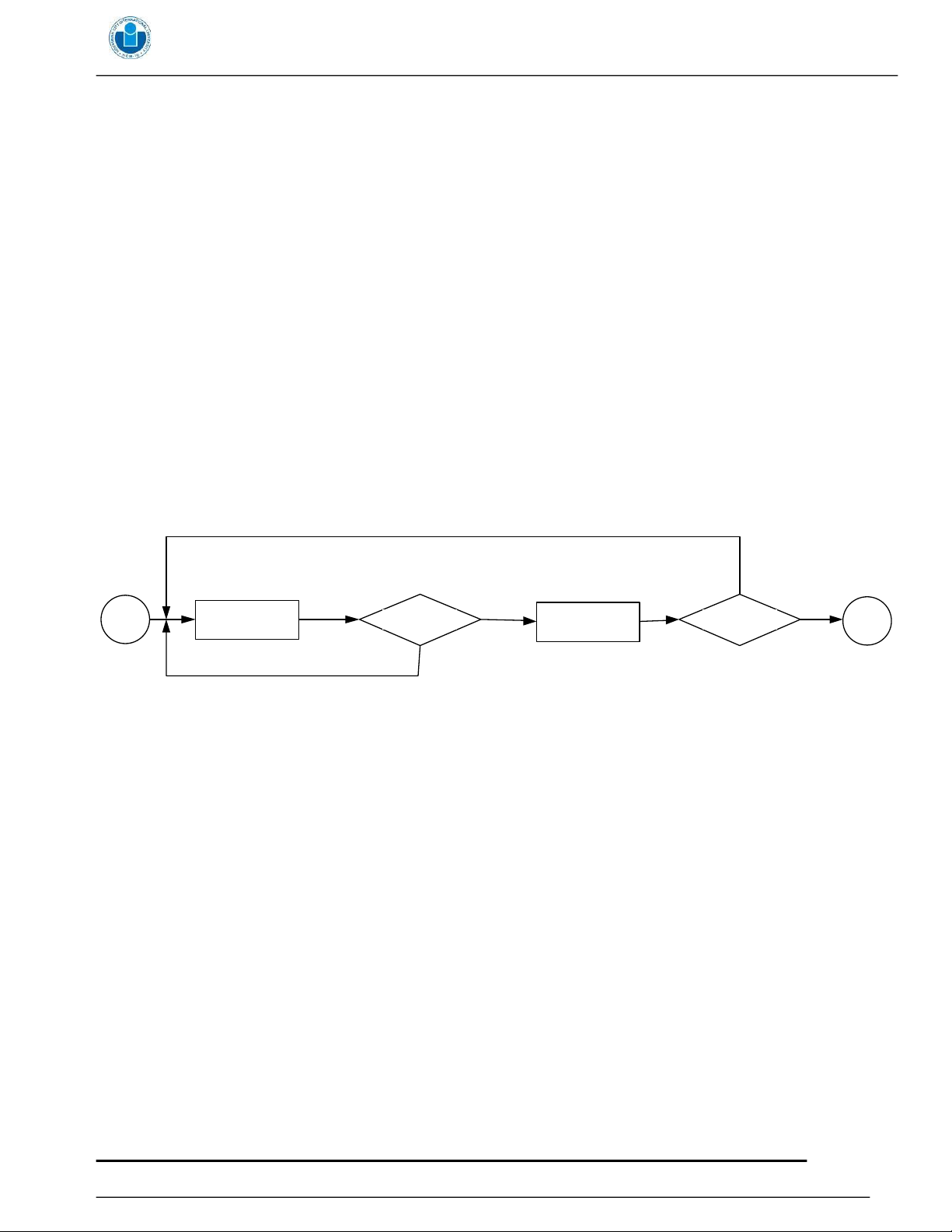
Find the expected processing time for finishing this project P(Fail)=0.4 P(Pass)=0.3 P(Pass)=0.6 Start Take 3 days Inspector 1 Take 7 days Inspector 2 End P(Fail)=0.7 Figure 1 Question 5: (20pts)
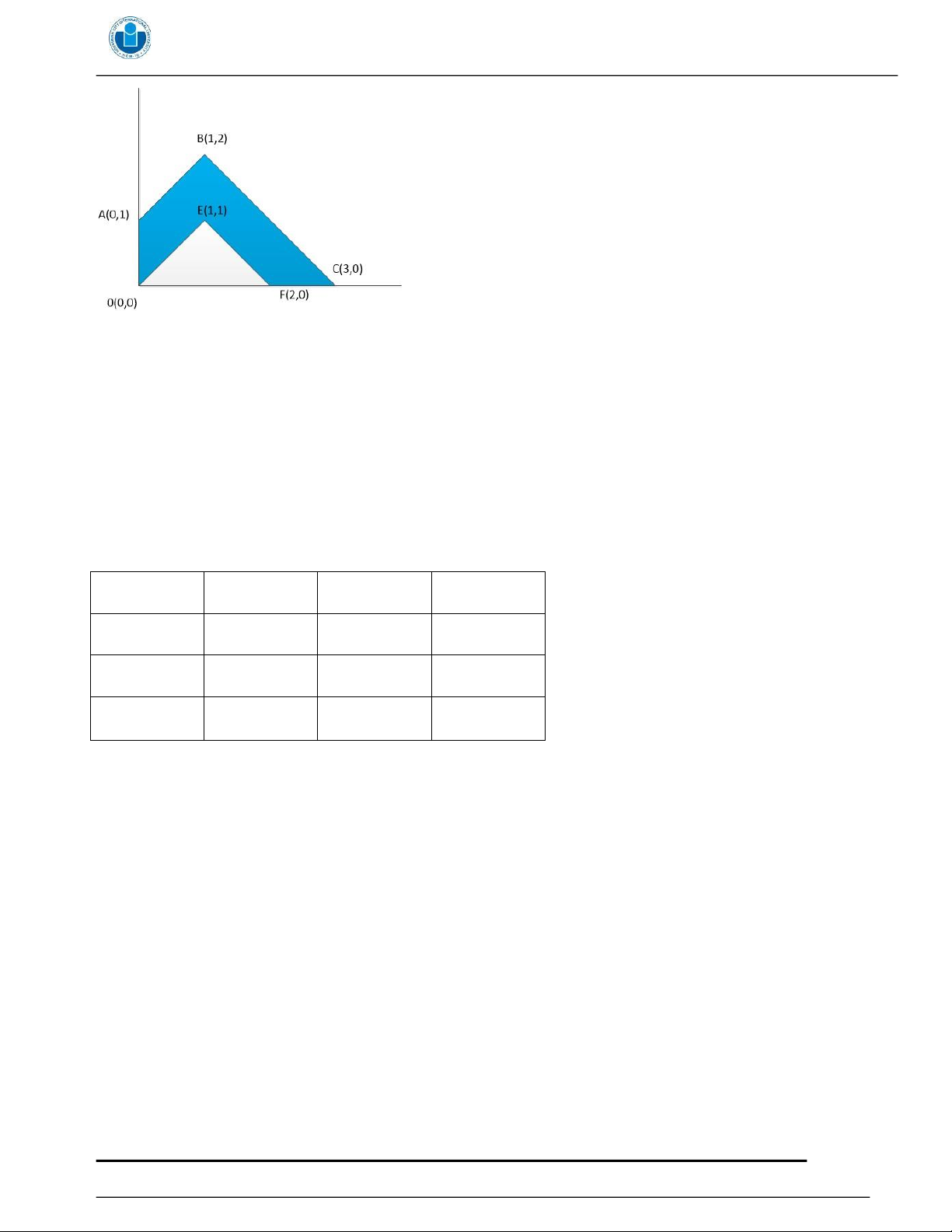
f(x,y) = {0Cy, otherwise on ROI Given that
a) Find C so that f(x,y) is a probability density function (7 pts)
b) Determine the conditional distribution function fX|Y(x|y) (7 pts)
c) Find the probability on the region ABCFEO (7 pts) Stochastic Model – 3, Apr, 2018 2
THE INTERNATIONAL UNIVERSITY (IU) Course: Stochastic Model
Department of Industrial System Engineering Final Exam Question 6: (20 pts)
The customer demands for a specific product on a market can be classified as: high, medium and low.
When customer demand is high, it follows Poisson distribution with rate 7 thousands per year. When
demand is medium or low, it follows Poisson distribution with rate 4 thousands and 1 thousands per year,
respectively. Following matrix gives the transition probability between each type of demands. High Medium Low High 0.5 0.3 0.2 Medium 0.3 0.4 0.3 Low 0.2 0.4 0.4
a) Find the transition probability matrix after 2 years (5pts)
b) Assumed we are at year 0 and the customer demand is high. Find the expected of total demand after 2 years. (10pts)
c) Based on above data company will decide whether they should invest on a project. This project
will last for 2 years. According to the plan, this project requires the minimum of 7.8 thousand
demands so as to not suffer loss. Assumed that we are currently at year 0 and the demand of the
market is not determined. Which case of demand in year 0 should we invest on this project? (10 pts) GOOD LUCKS! Stochastic Model – 3, Apr, 2018 3