lOMoAR cPSD| 45903860 APPLIED STATISTICS COURSE CODE: ENEE1006IU Lecture 7:
Chapter 4: Probability and Distribution
(3 credits: 2 is for lecture, 1 is for lab-work) 1 lOMoAR cPSD| 45903860
4.3. CONTINUOUS PROBABILITY DISTRIBUTIONS
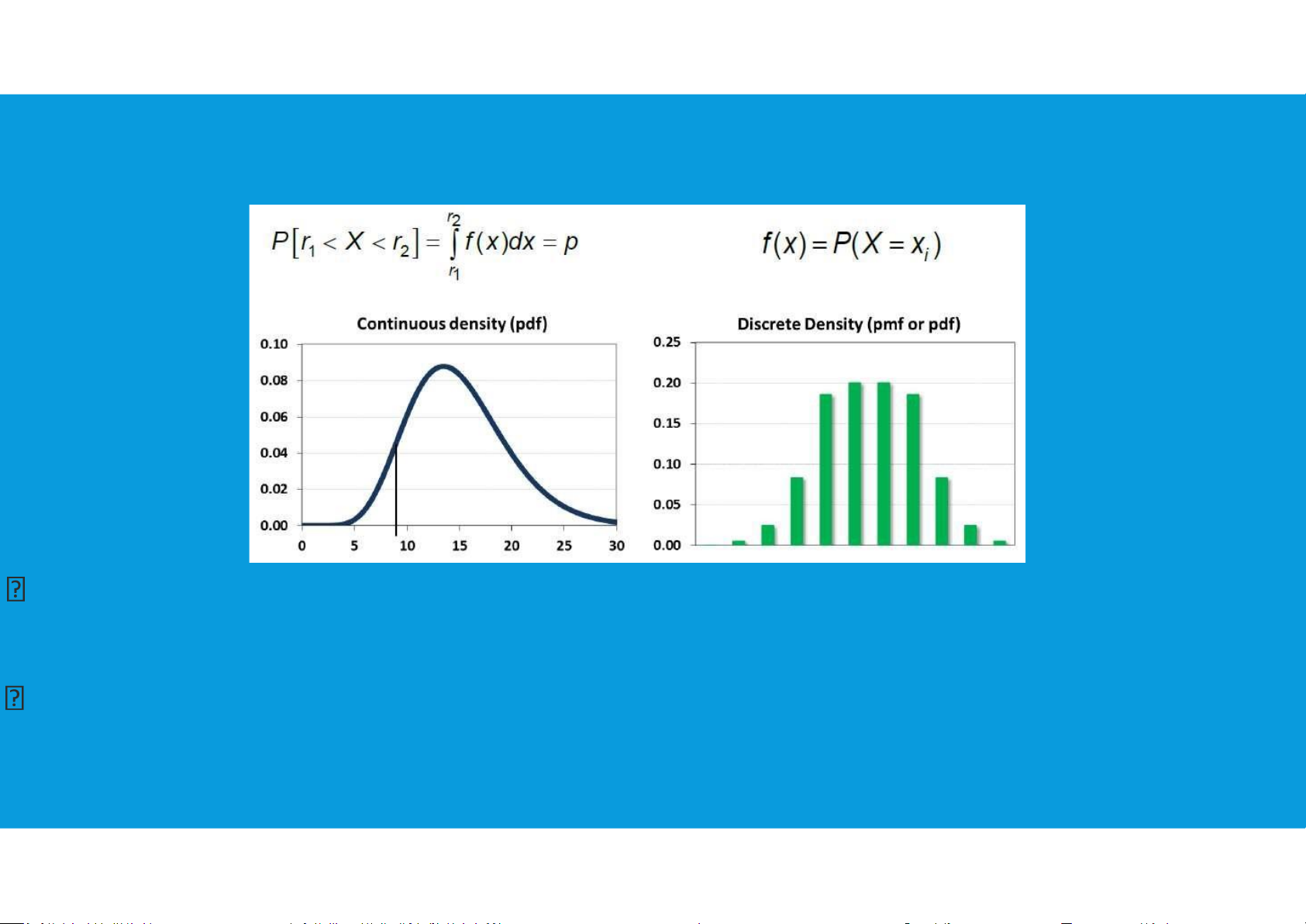
•A fundamental difference separates discrete and continuous random variables in
terms of how probabilities are computed.
-For a discrete random variable, the probability function f (x) provides the
probability that the random variable assumes a particular value.
-With continuous random variables, the counterpart of the probability function is
the probability density function, also denoted by f (x).
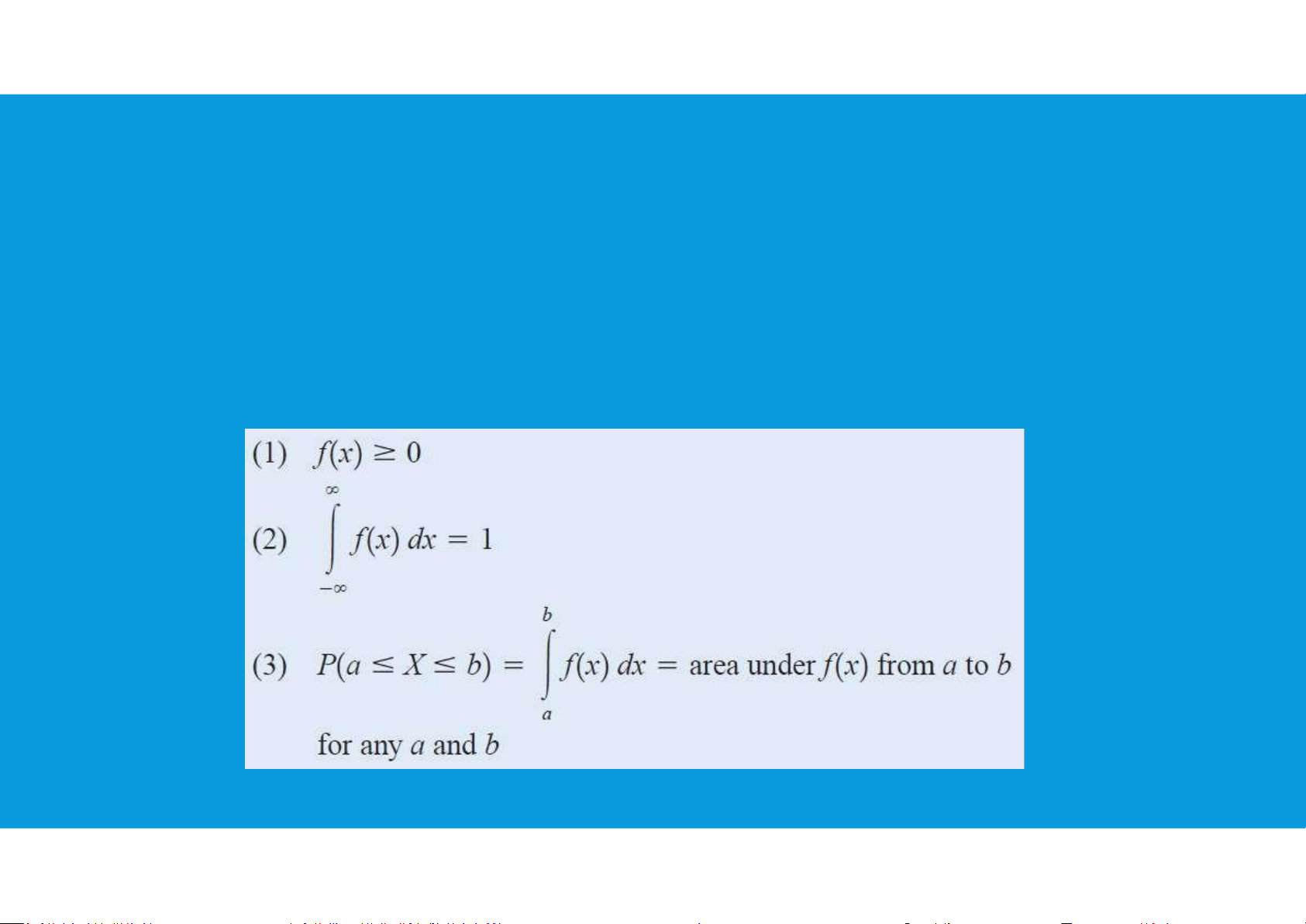
The difference is that the probability density function does not directly provide probabilities.
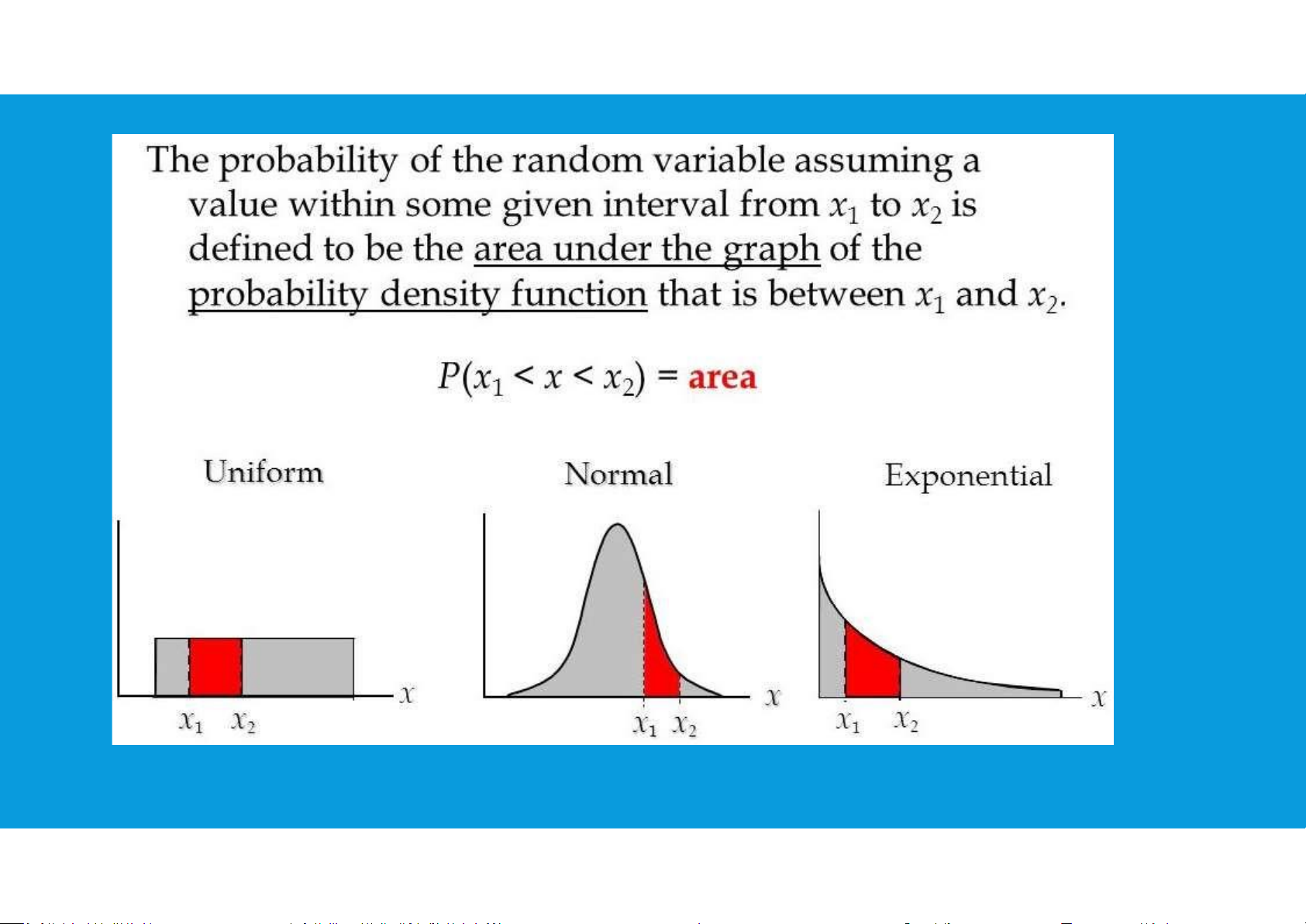
The area under the graph of f (x) corresponding to a given interval does provide
the probability that the continuous random variable x assumes a value in that interval. lOMoAR cPSD| 45903860
4.3. CONTINUOUS PROBABILITY DISTRIBUTIONS
•Continuous probability distribution: A probability distribution in which
the random variable x can take on any value (is continuous).
•Because there are infinite values that x could assume, the
probability of x taking on any one specific value is zero.
4.3. CONTINUOUS PROBABILITY DISTRIBUTIONS ) lOMoAR cPSD| 45903860
Uniform probability distribution
Normal probability distribution
Normal approximation of binomial probabilities
Exponential probability distribution
4.3. CONTINUOUS PROBABILITY DISTRIBUTIONS lOMoAR cPSD| 45903860 ) lOMoAR cPSD| 45903860
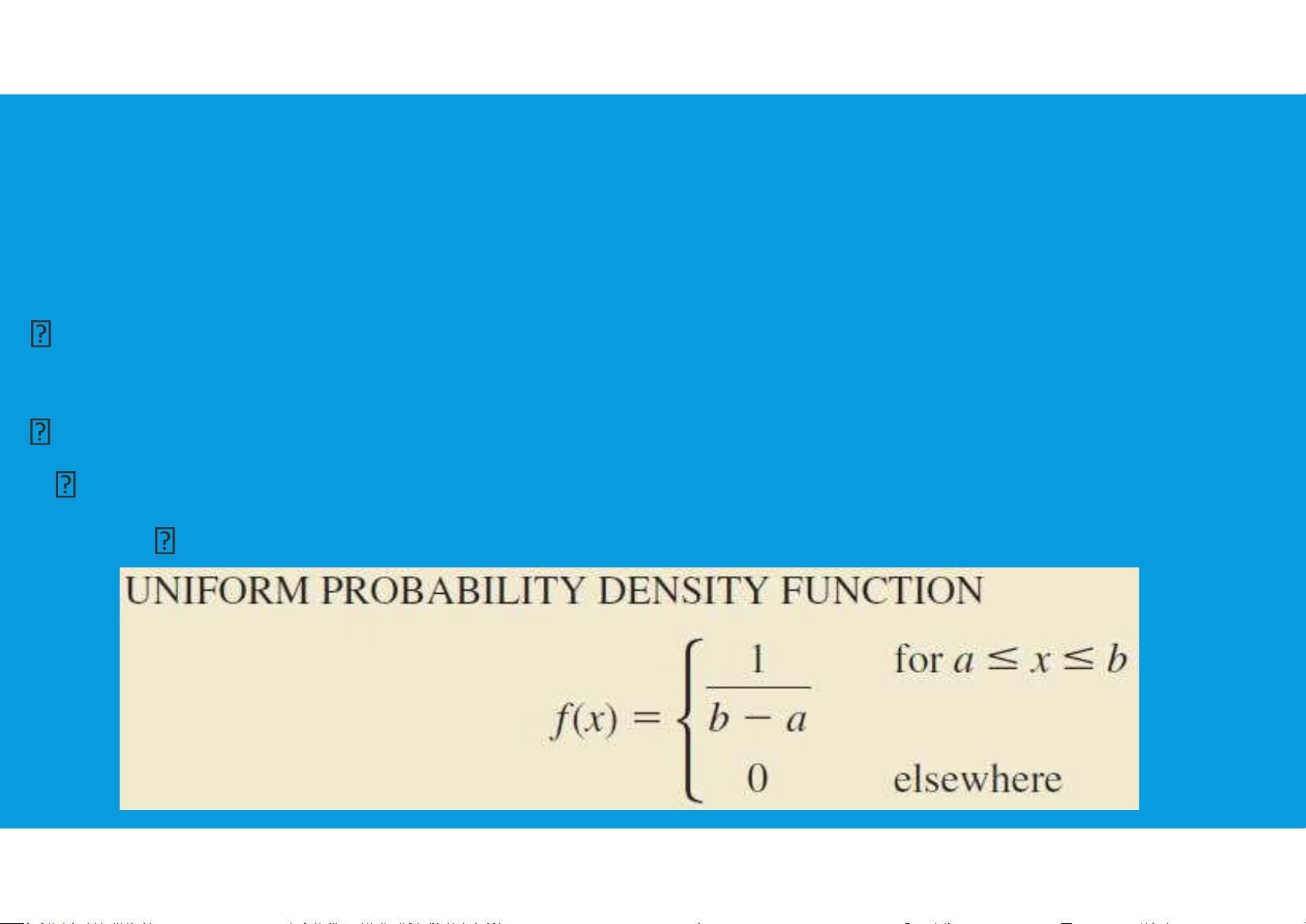
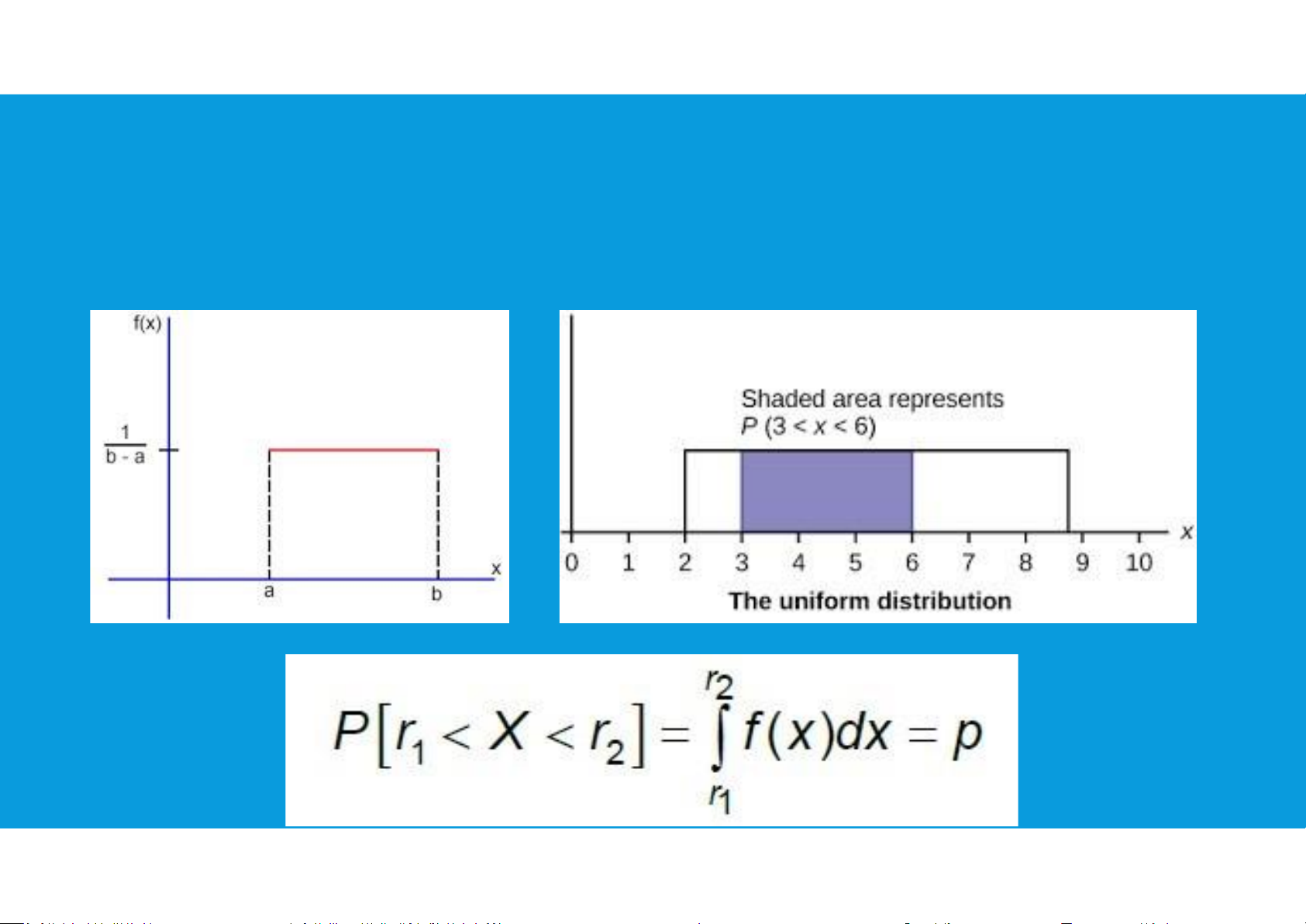
4.3. CONTINUOUS PROBABILITY DISTRIBUTIONS •Uniform probability distribution:
Uniform distributions are probability distributions with equally likely outcomes.
There are two types of uniform distributions: discrete and continuous.
In a discrete distribution, each outcome is discrete.
In a continuous distribution, outcomes are continuous and infinite. lOMoAR cPSD| 45903860
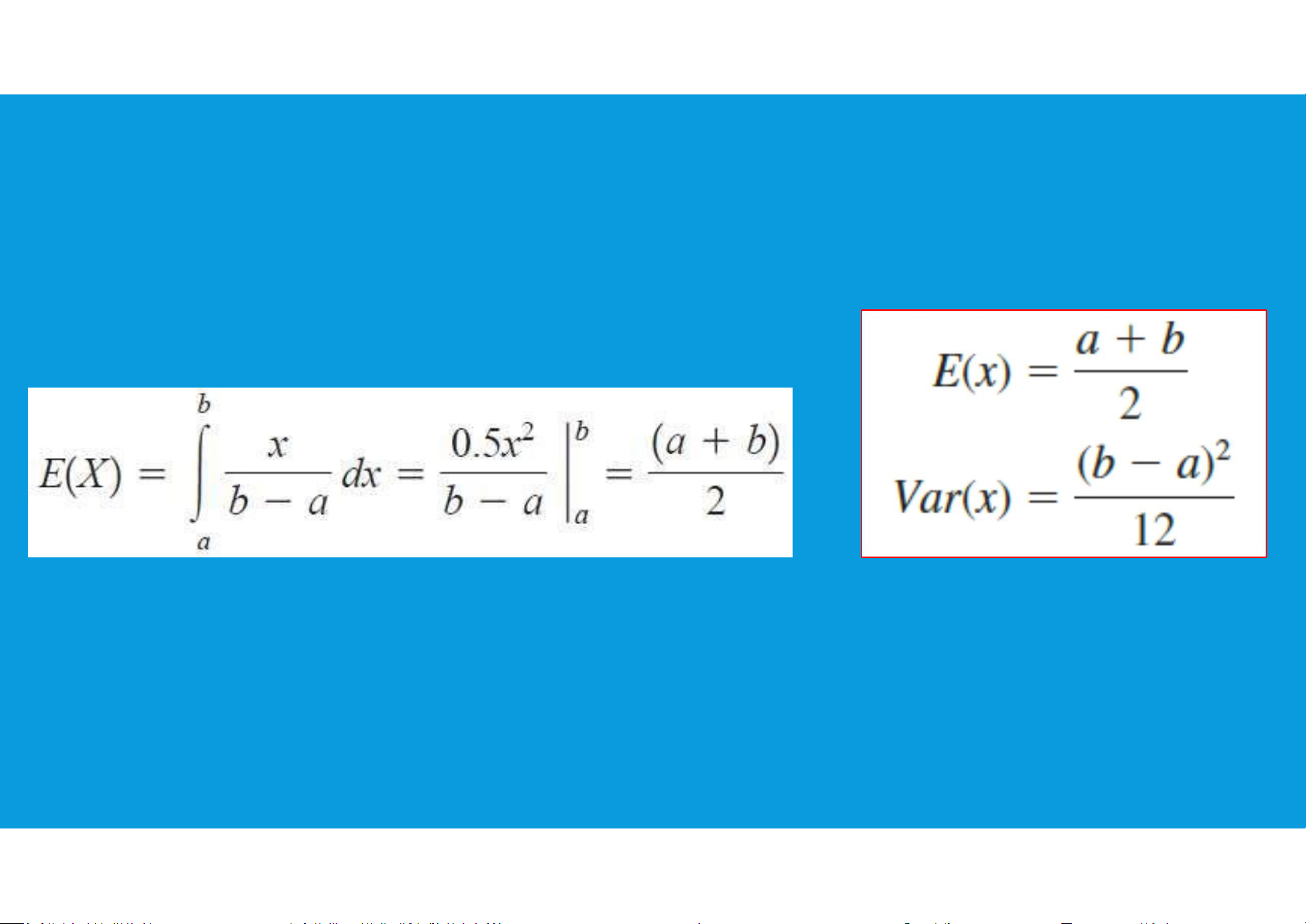
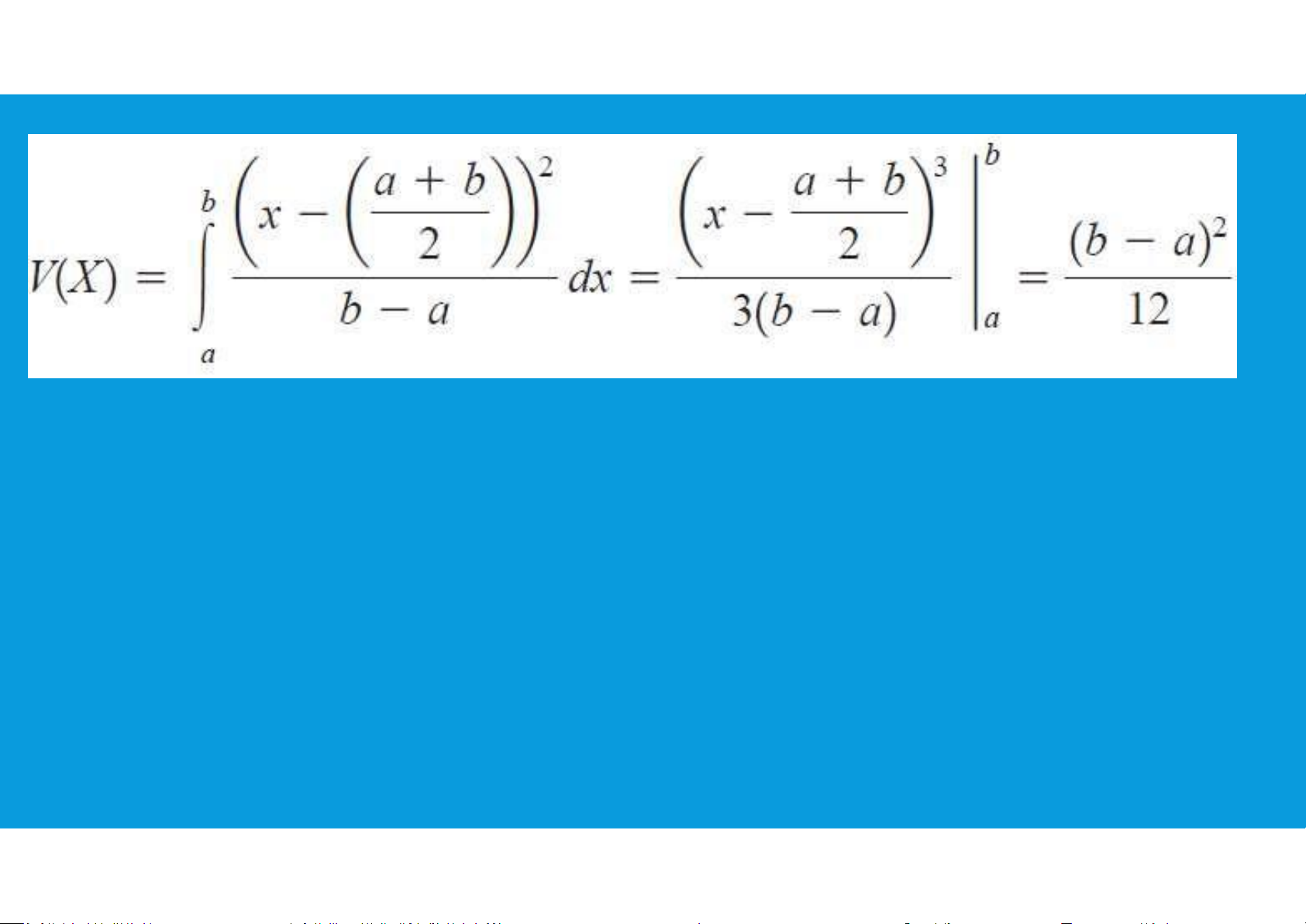
4.3. CONTINUOUS PROBABILITY DISTRIBUTIONS •Uniform probability distribution: ) lOMoAR cPSD| 45903860
4.3. CONTINUOUS PROBABILITY DISTRIBUTIONS •Uniform probability distribution: lOMoAR cPSD| 45903860
4.3. CONTINUOUS PROBABILITY DISTRIBUTIONS
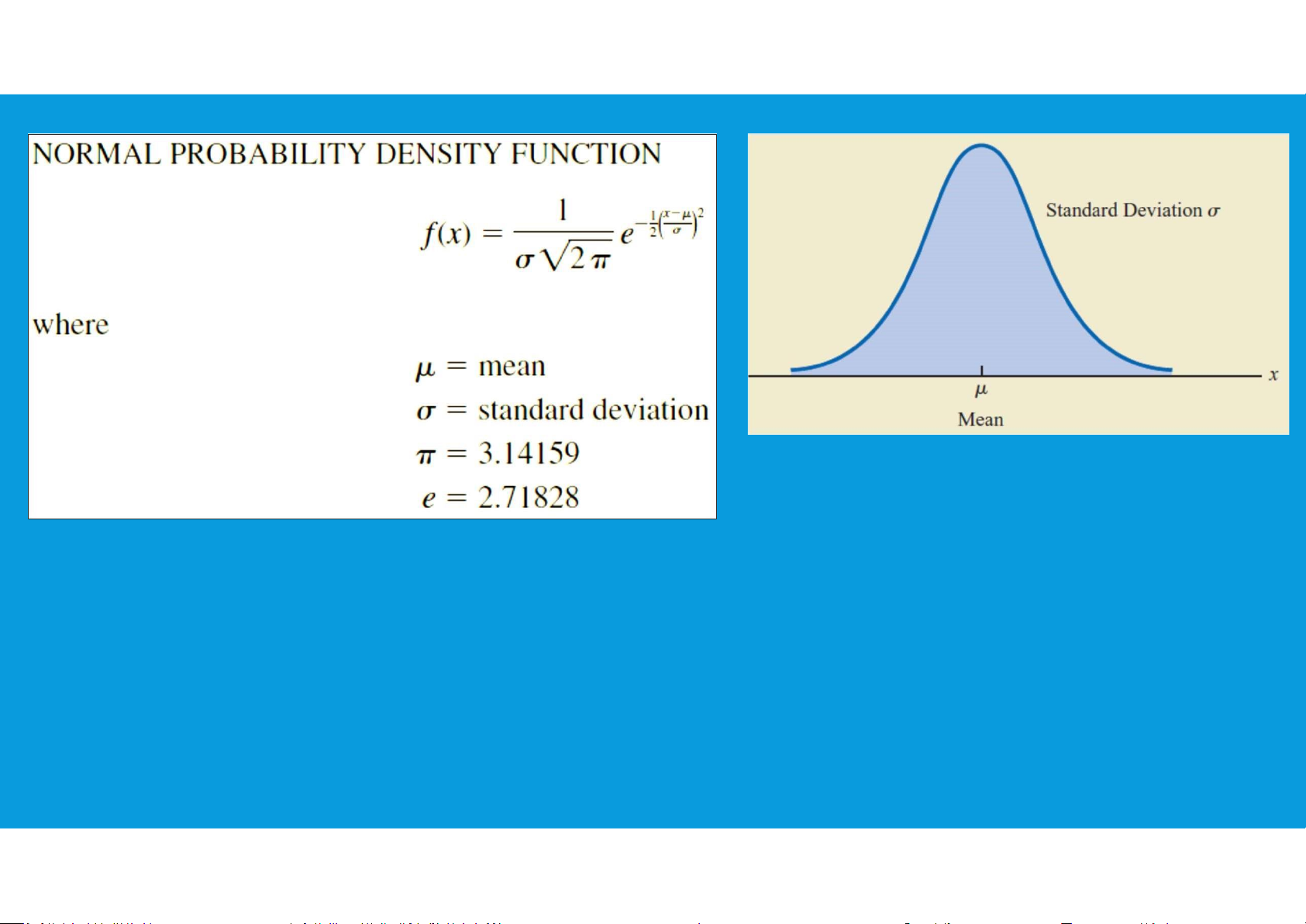
•Normal probability distribution: The most important probability
distribution for describing a continuous random variable.
•Normal Curve: The form, or shape, of the normal distribution is illustrated by the bell-shaped normal curve ) lOMoAR cPSD| 45903860
4.3. CONTINUOUS PROBABILITY DISTRIBUTIONS
•Normal probability distribution: characteristics
•The entire family of normal distributions is differentiated by two parameters: the
mean µ and the standard deviation σ. lOMoAR cPSD| 45903860
•The highest point on the normal curve is at the mean, which is also the median and mode of the distribution.
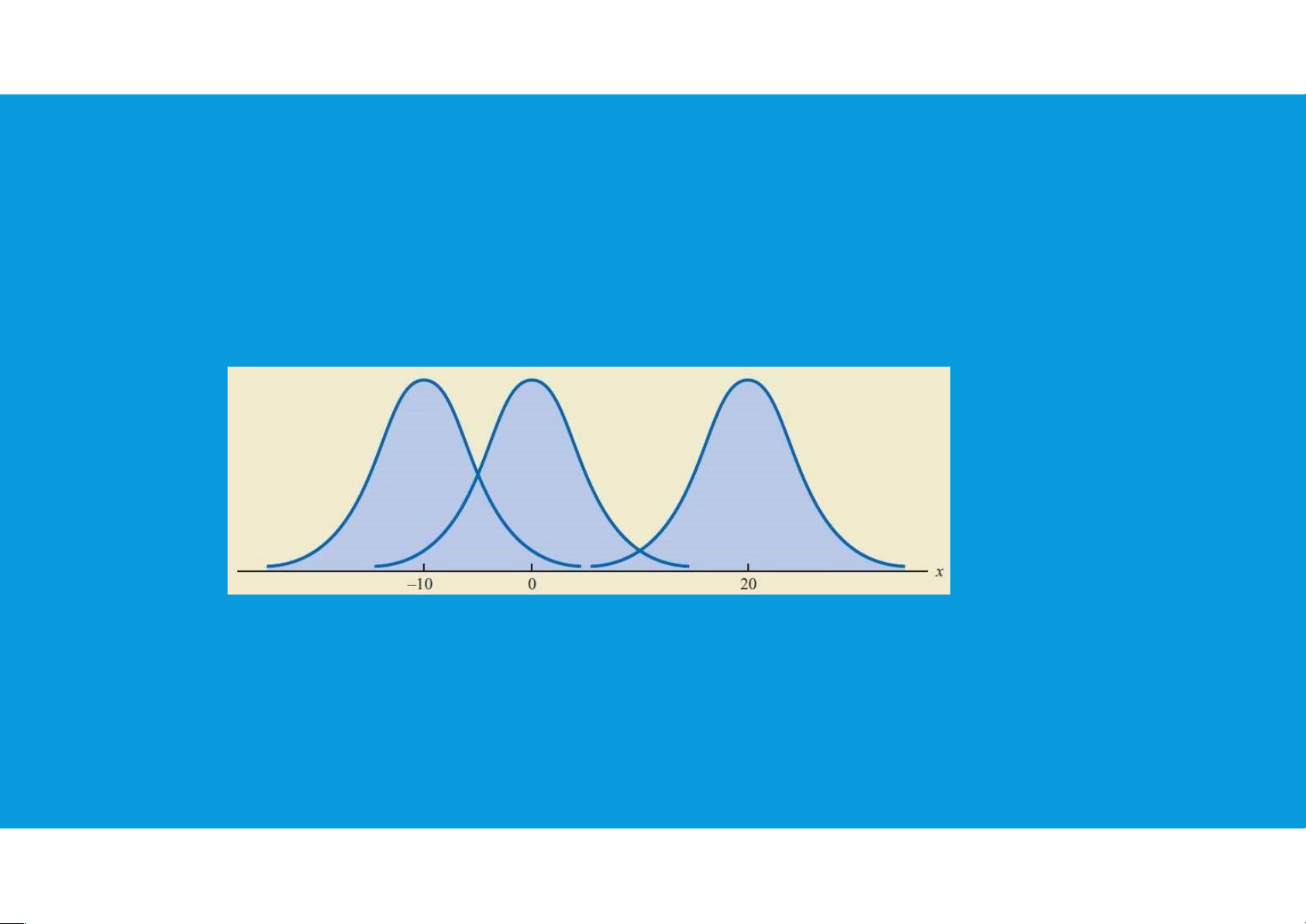
•The mean of the distribution can be any numerical value: negative, zero, or
positive. Three normal distributions with the same standard deviation but three
different means (−10, 0, and 20) are shown here.
4.3. CONTINUOUS PROBABILITY DISTRIBUTIONS
•Normal probability distribution: characteristics
• The normal distribution is symmetric, with the shape of the normal curve to the left of
the mean a mirror image of the shape of the normal curve to the right of the mean. The ) lOMoAR cPSD| 45903860
tails of the normal curve extend to infinity in both directions and theoretically never
touch the horizontal axis. Because it is symmetric, the normal distribution is not
skewed; its skewness measure is zero.
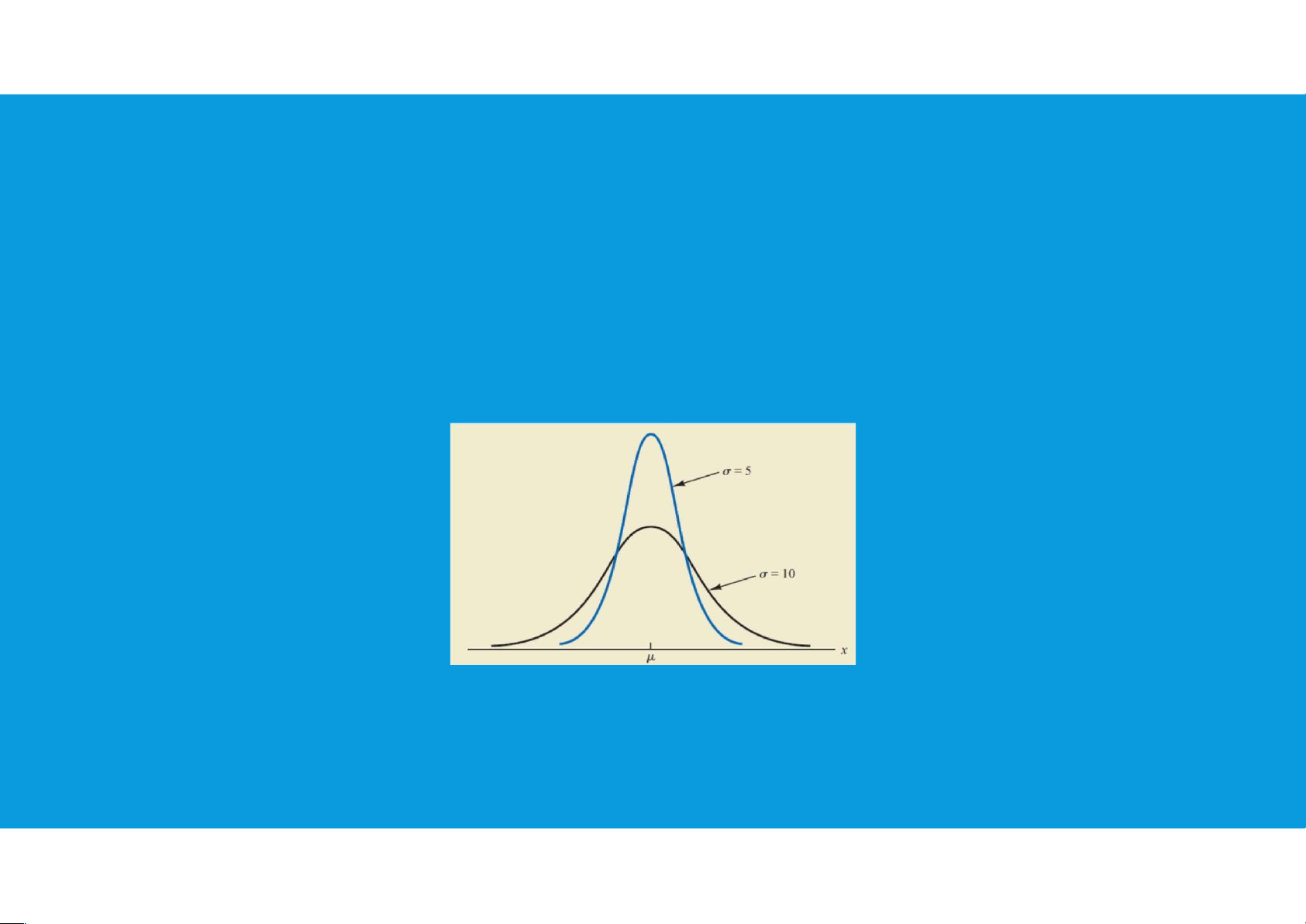
• The standard deviation determines how flat and wide the normal curve is. Larger values
of the standard deviation result in wider, flatter curves, showing more variability in the
data. Two normal distributions with the same mean but with different standard deviations are shown here.
4.3. CONTINUOUS PROBABILITY DISTRIBUTIONS
•Normal probability distribution: characteristics lOMoAR cPSD| 45903860
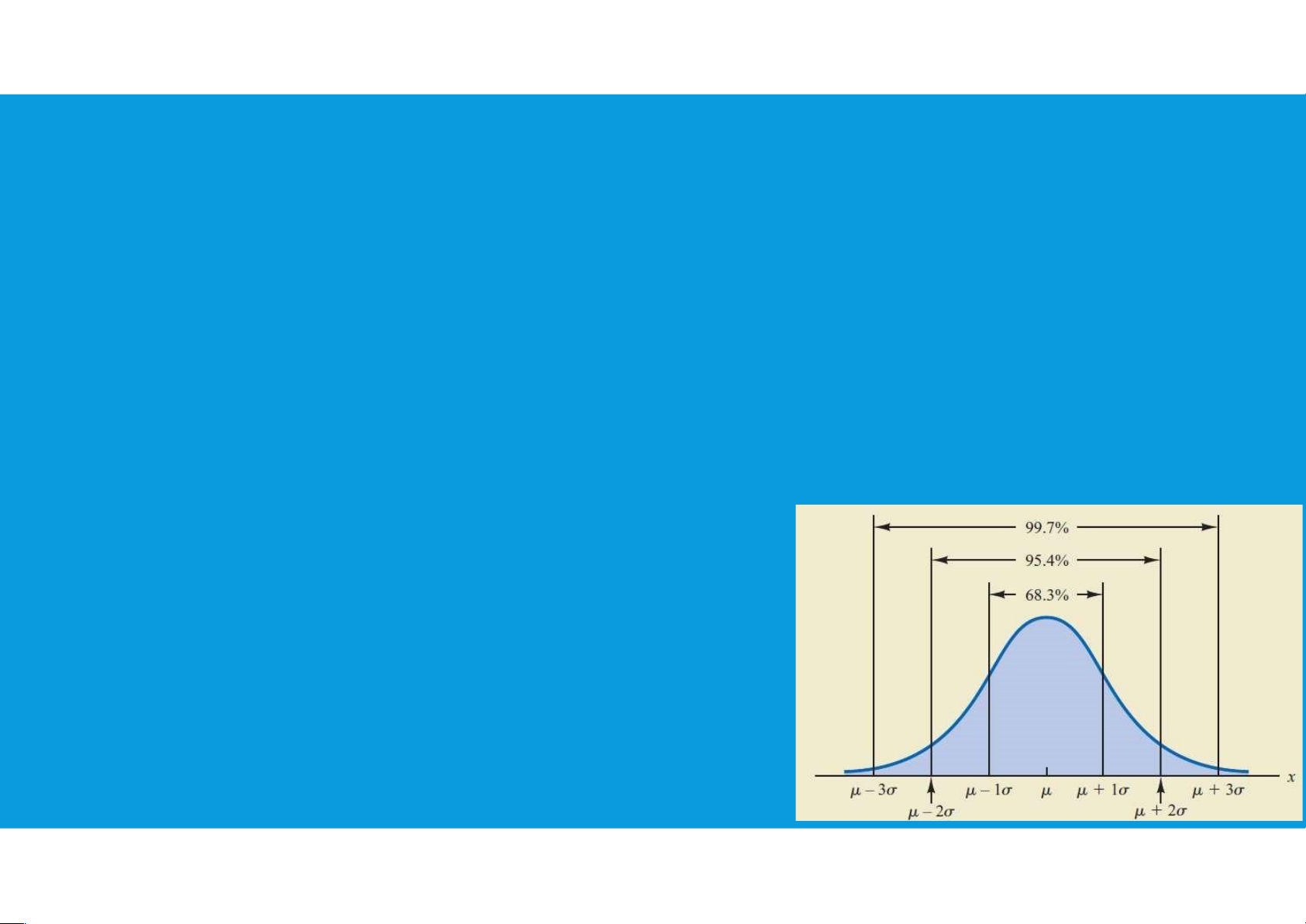
•Probabilities for the normal random variable are given by areas under the normal
curve. The total area under the curve for the normal distribution is 1. Because the
distribution is symmetric, the area under the curve to the left of the mean is 0.5
and the area under the curve to the right of the mean is 0.5.
•The percentage of values in some commonly used intervals are:
a. 68.3% of the values of a normal random variable are within
plus or minus one standard deviation of its mean. b. 95.4% of
the values of a normal random variable are within plus or
minus two standard deviations of its mean. c. 99.7% of the
values of a normal random variable are within plus or minus
three standard deviations of its mean. 4.3. CONTINUOUS PROBABILITY DISTRIBUTIONS ) lOMoAR cPSD| 45903860
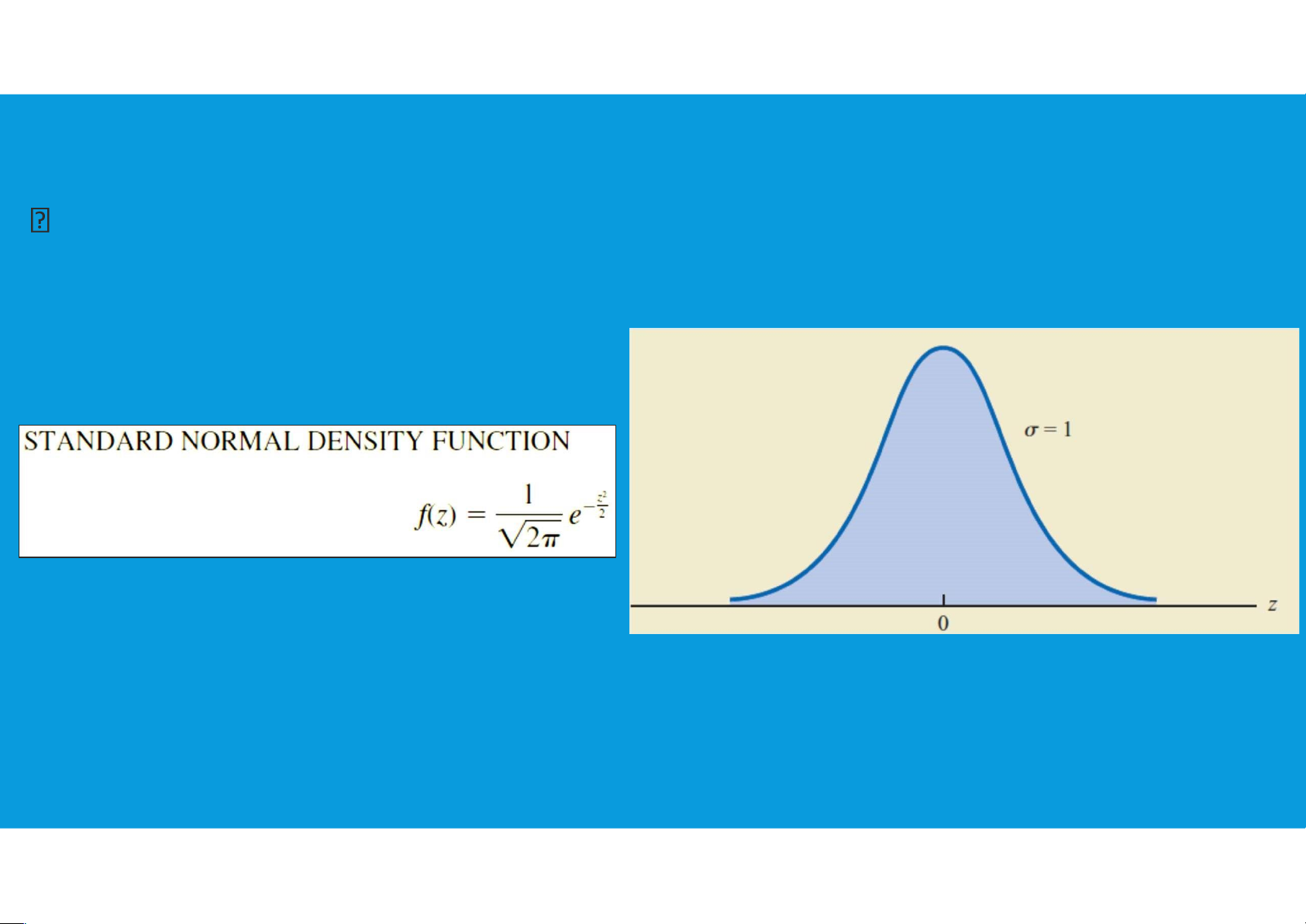
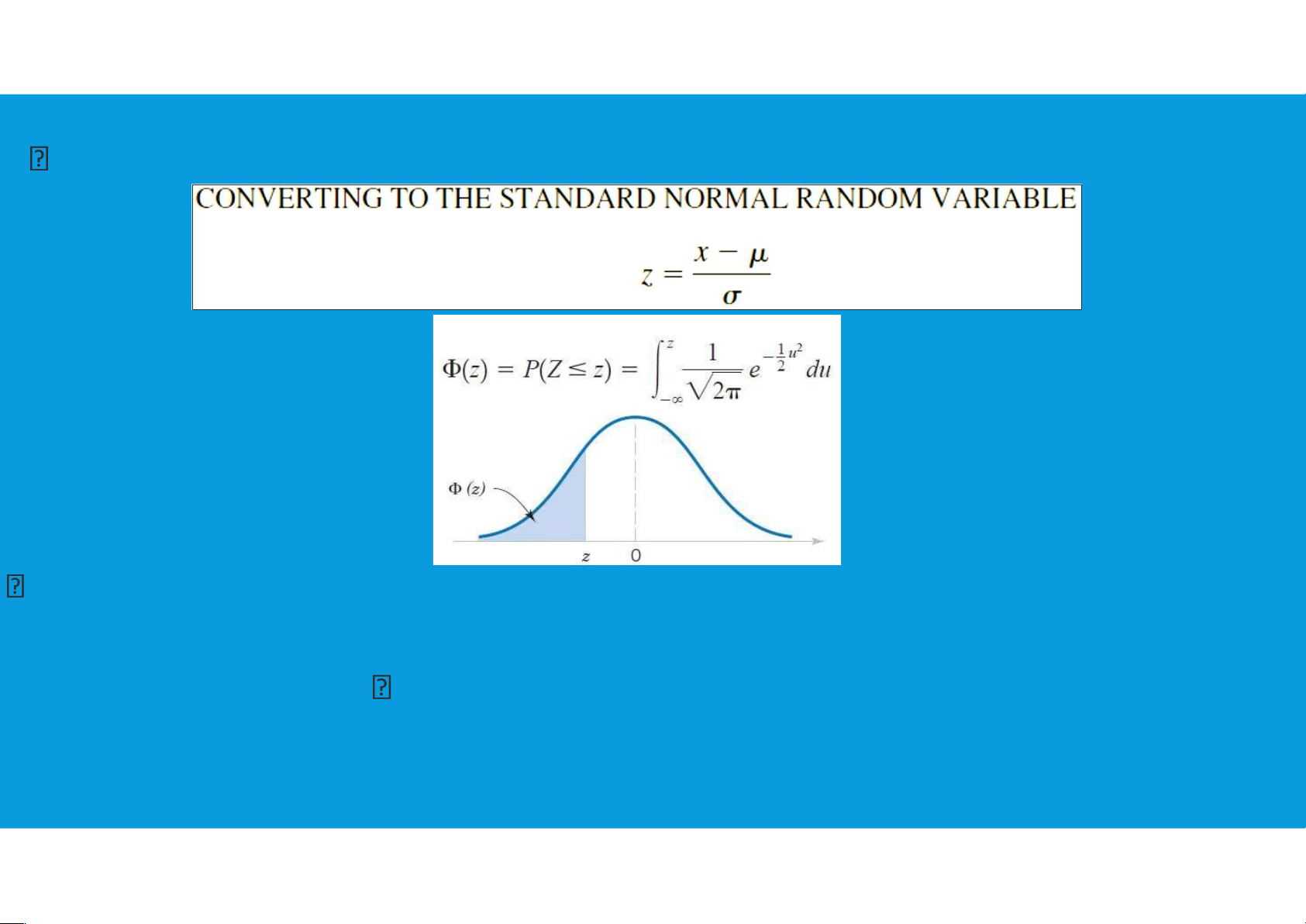
•Normal probability distribution:
Standard Normal Probability Distribution: A random variable that has a normal
distribution with a mean of zero and a standard deviation of one is said to have a
standard normal probability distribution.
4.3. CONTINUOUS PROBABILITY DISTRIBUTIONS
•Normal probability distribution: lOMoAR cPSD| 45903860
Computing Probabilities for Any Normal Probability Distribution:
we can interpret z as the number of standard deviations that the normal random
variable x is from its mean µ.
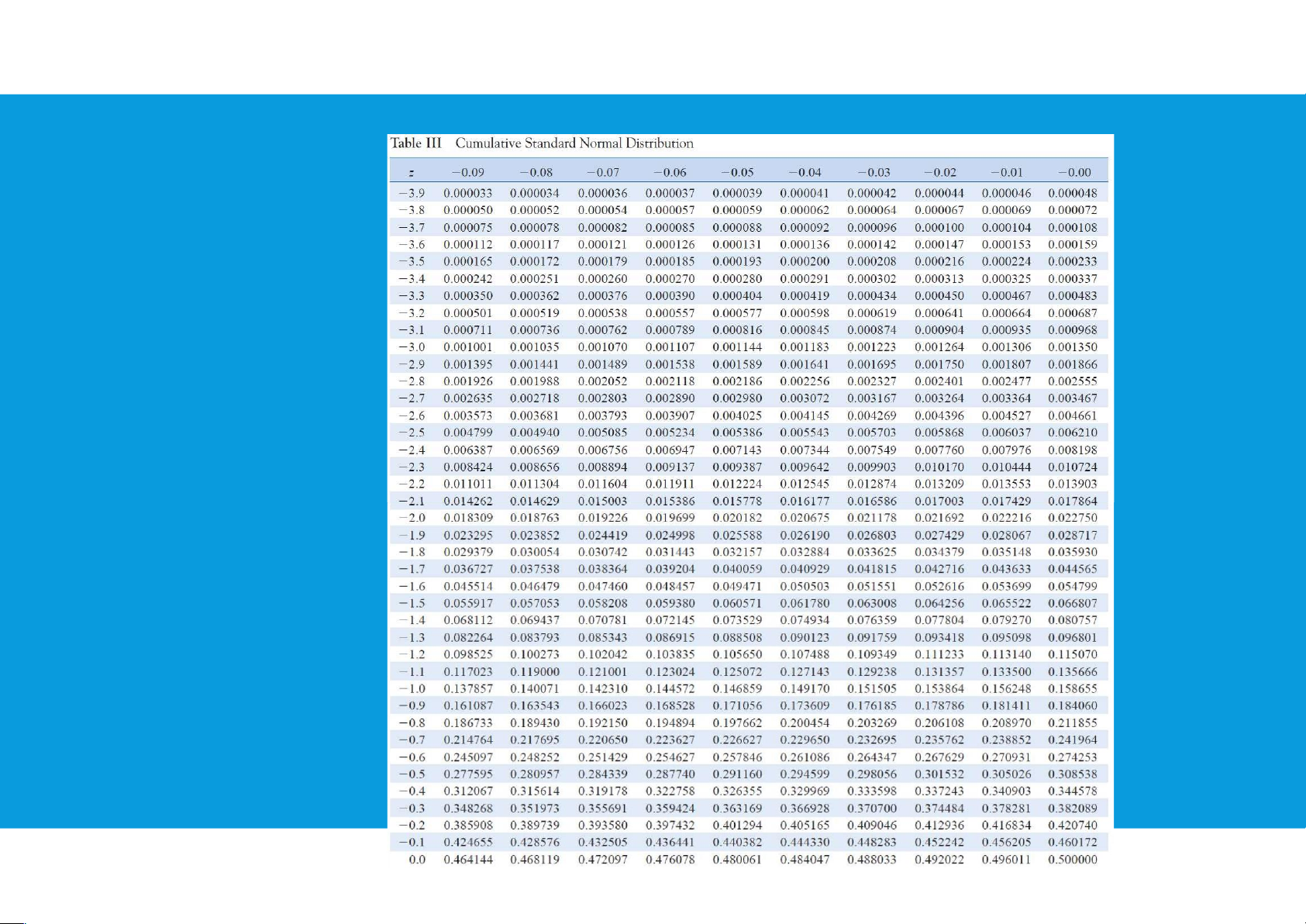
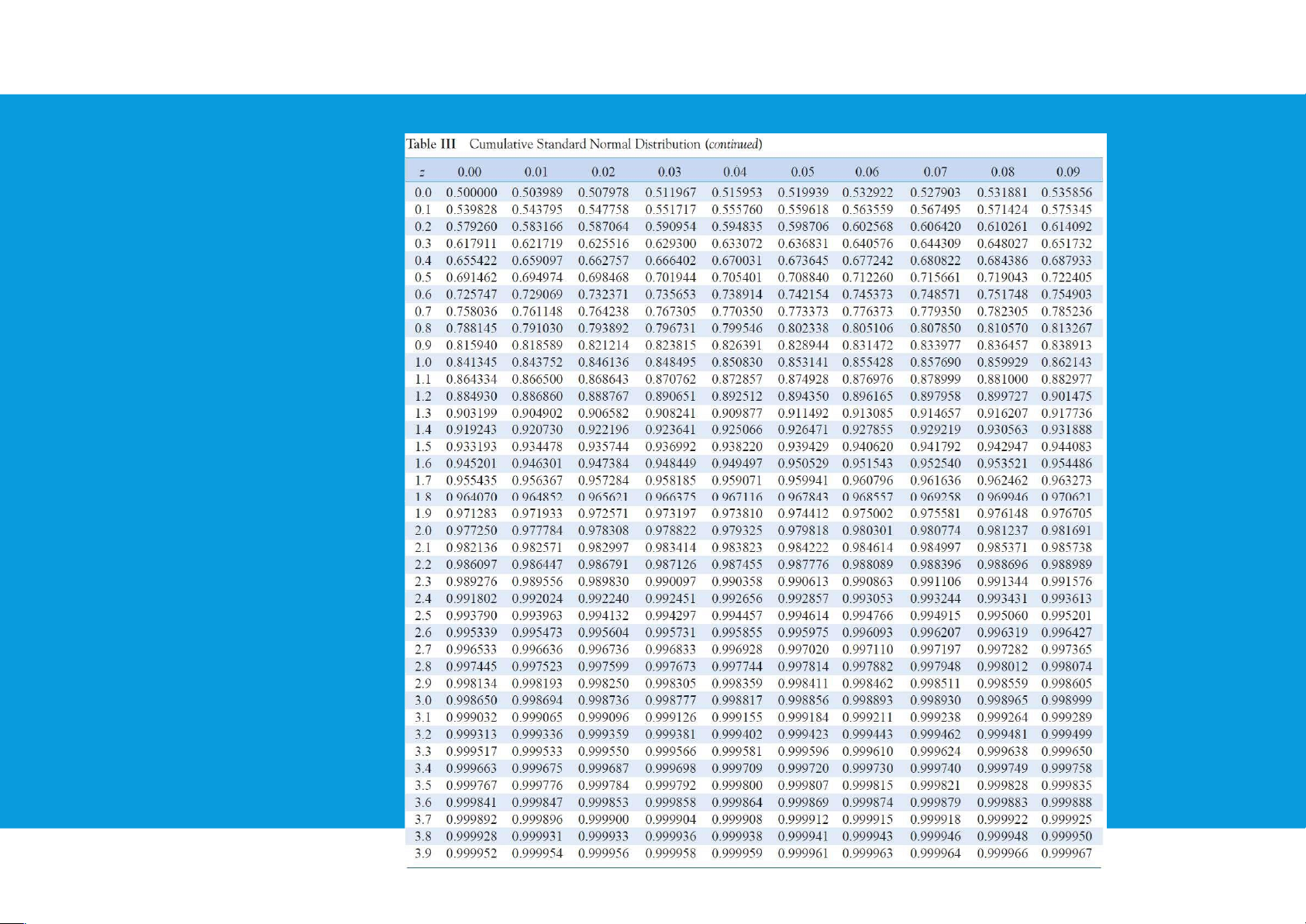
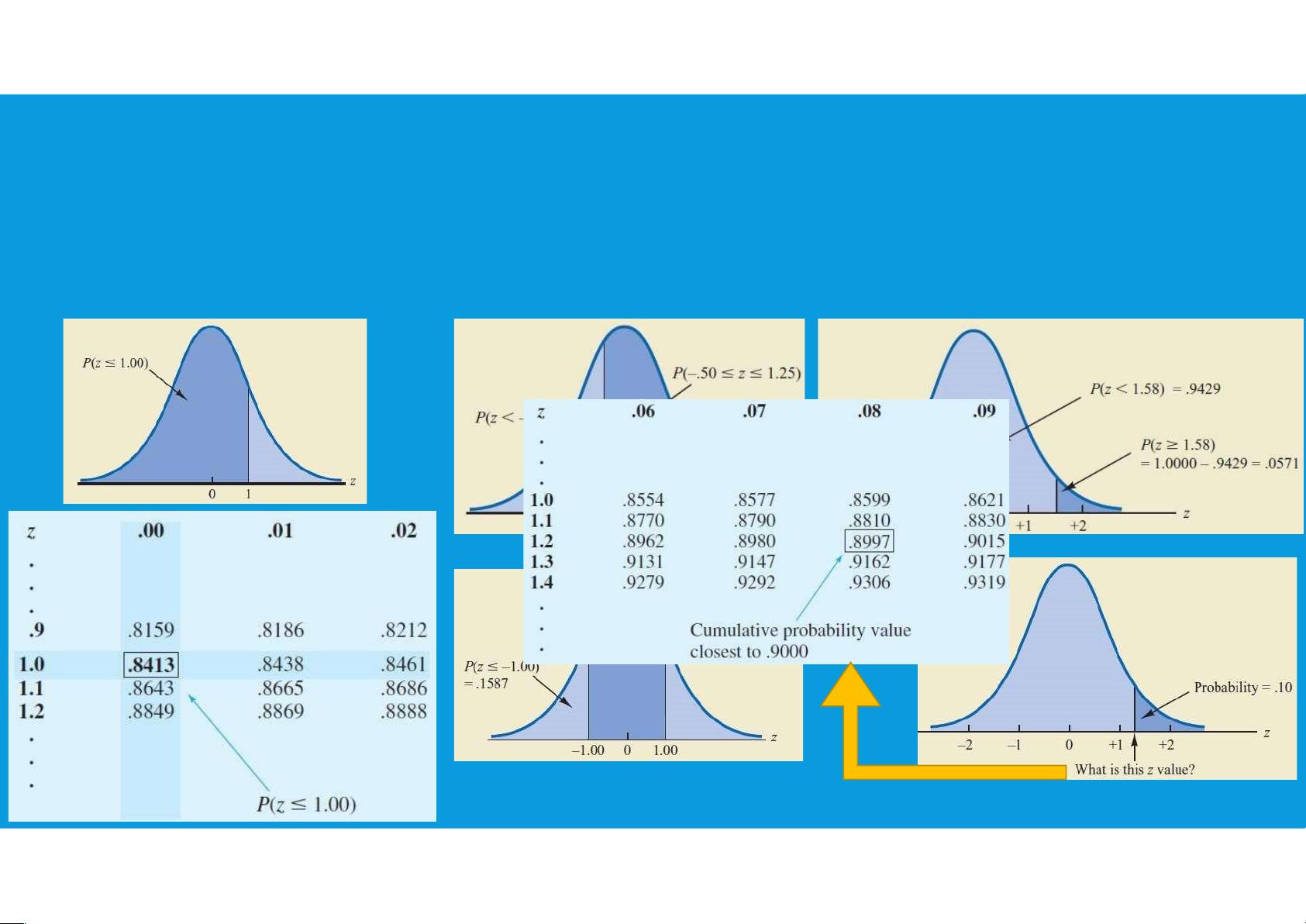
Use the table to check the probability ) lOMoAR cPSD| 45903860 •Normal lOMoAR cPSD| 45903860 probability distribution (z < 0): ) lOMoAR cPSD| 45903860 •Normal lOMoAR cPSD| 45903860 probability distribution (z > 0): ) lOMoAR cPSD| 45903860
4.3. CONTINUOUS PROBABILITY DISTRIBUTIONS
•Normal probability distribution: