Reference - Baì tập chương 7 - Tài liệu tham khảo | Đại học Hoa Sen

REFERENCE. LECTURE.8 QUESTION 1. Calculating beta
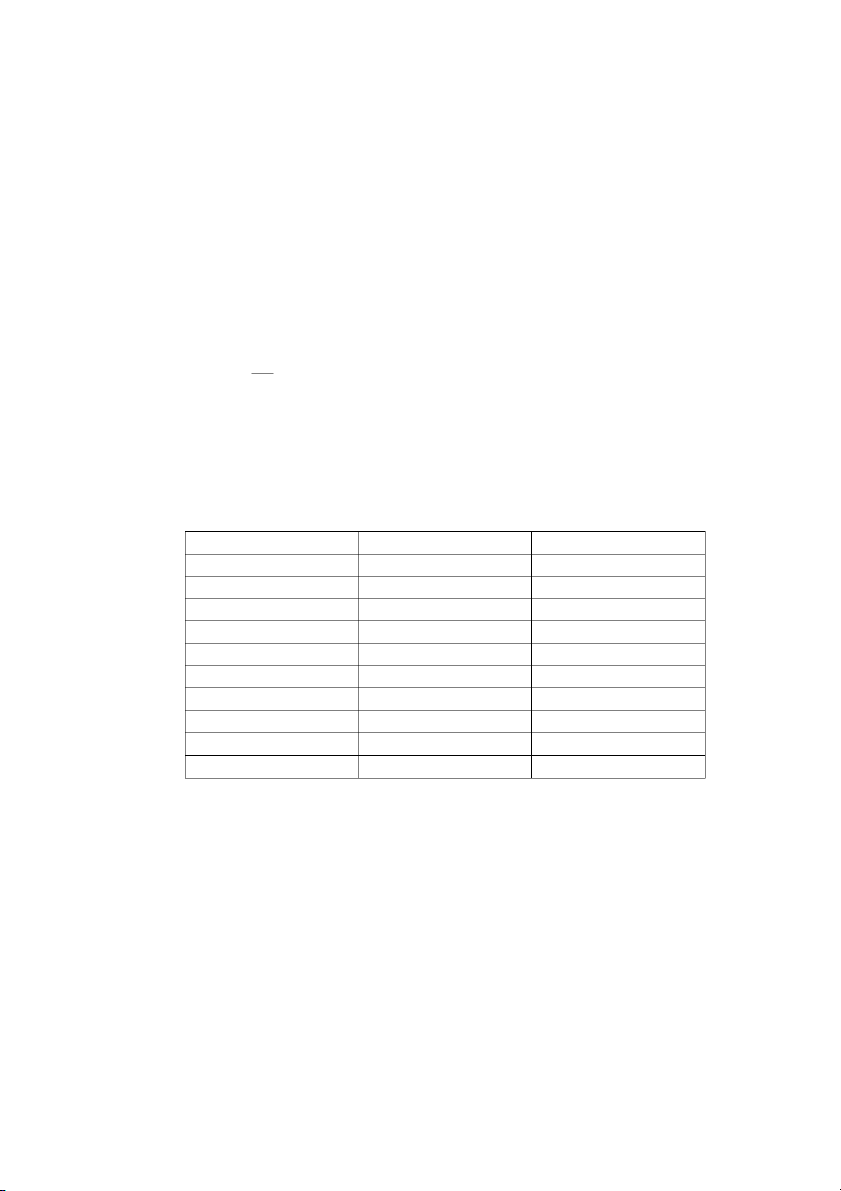
Following are several months’ rate of return for Tumblehome Canoe Company.
Prepare a plot like figure 12-1. What is Tumblehome’s beta?
Beta (β) of a stock is its sensitivity to market movements. It is the percentage change in
the stock for 1% change in the market. ∆ β = ∆
β is the sensitivity of stock return to market return
∆rs is the change in security return
∆rm is the change in market return
- Problem gives the monthly rate of return for Tumblehome Canoe Company and
Market return for several months as shown below: Month Market Return % Company T Return % 1 0 1 2 0 -1 3 -1 -2.5 4 -1 -0.5 5 1 2 6 1 1 7 2 4 8 2 2 9 -2 -2 10 -2 -4
To, get β for Tumblehome Canoe Company, first calculate the average return for
Tumblehome Canoe Company for various values of market return. For example when
market return is 1% in month 5 and 6, Tumblehome Canoe Company average return is
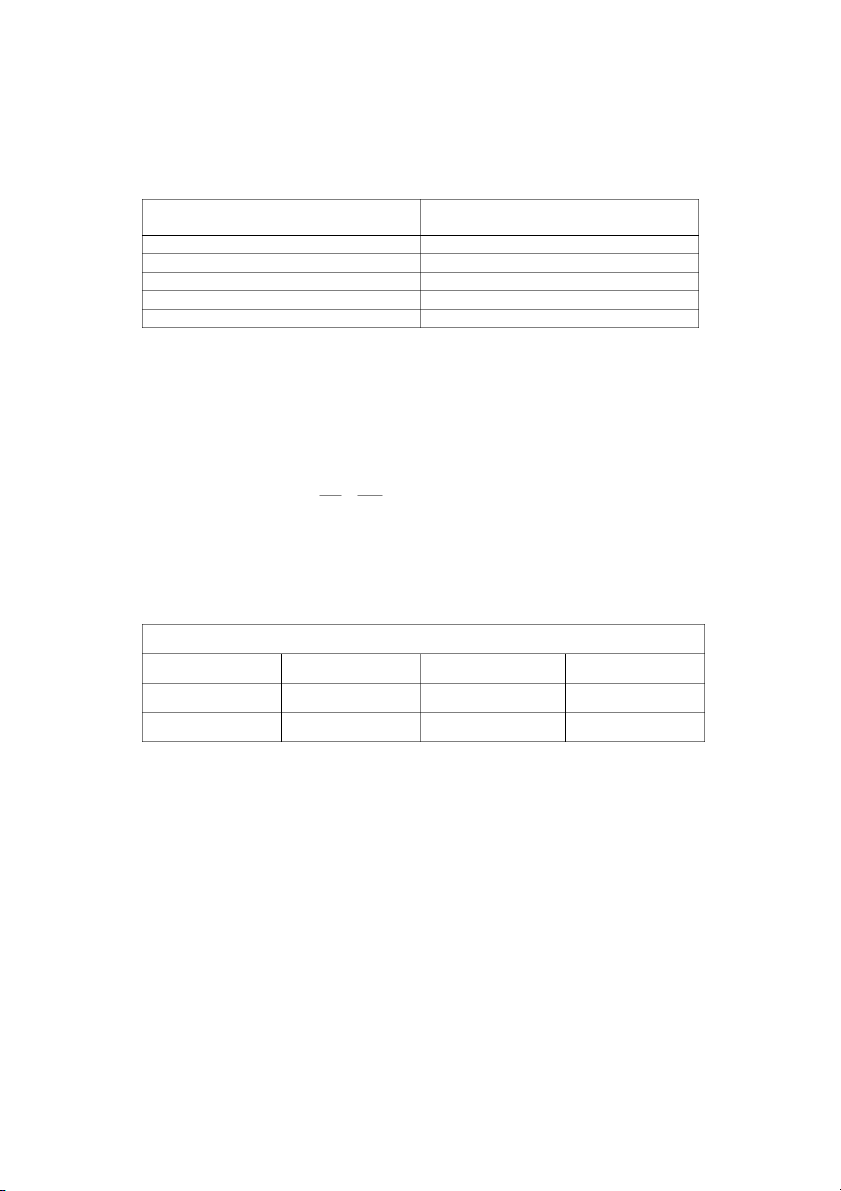
1.5%. Similarly calculate the average return for Tumblehome Canoe Company for each value of market return . Market Return % Avg. Tumblehome Canoe Company Return % 2 3 1 1.5 0 0 -1 -1.5 -2 -3
Table above shows that for every percentage change in market return, Tumblehome
Canoe Company return is 1.5 times the market return; and in the same direction.
Calculate beta for Tumblehome Canoe Company (βT) by putting the values in the formula: ∆ .% βT = = = 1.5 ∆ %
So, the beta for Tumblehome Canoe Company is 1.5. 2. Expected returns.
Consider the following two scenario for the economy and the returns in each
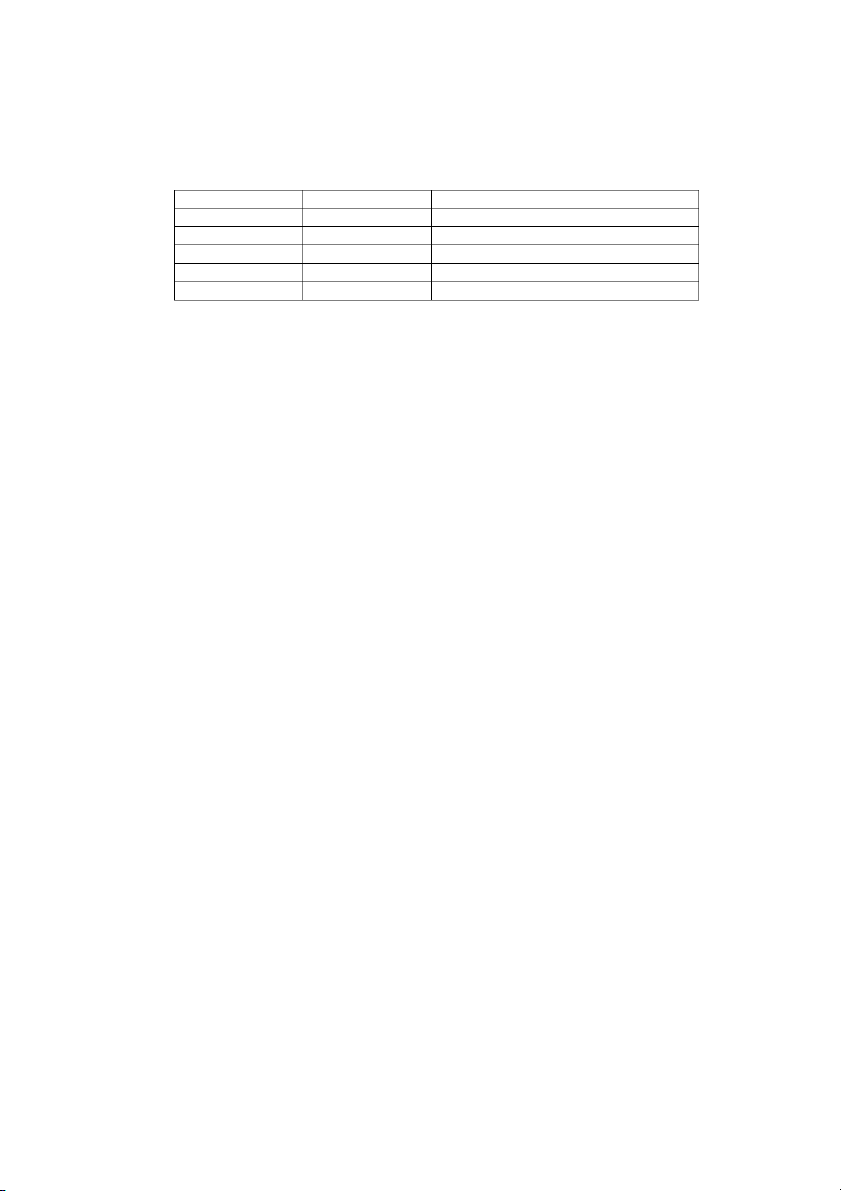
scenario for the market portfolio, an aggressive stock A and a defensive stock D. Rate of Return Scenario Market
Aggressive Stock A Defensive Stock D Bust -8% -10% -6% Boom 32 38 24
a. Find the beta of each stock. In what way is stock D defensive ?
b. If each scenario is equally likely, find the expected rate of return on the
market portfolio and on each stock
c. If the T-bill rate is 4%, what does the CAPM say about the fair expected rate of return on the two stocks?
d. Which stock seems to be a better buy on the basis of your answers to (a) through (c)?
a. Beta of a security is its sensitivity to market movements. It is calculated as the ratio
of change in the security return to change in the market return: ∆ β = ∆ Where,
β is the sensitivity of stock return to market return
∆r is the change in security return
∆rm is the change in market return
- Problem states the rate of return for market and two stocks, aggressive stock A and
defensive stock D, under two scenarios Bust and Boom as given in the following table: Rate of Return Scenario Market Aggressive Stock A Defensive Stock D Bust -8% -10% -6% Boom 32 38 24
Calculate beta for stock A ans stock D by dividing the change in stock return on the bust
and boom scenarios to change in market return under these scenarios: For stock A, ∆
,, %(%) β = = = = 1.2 ∆
,, %(%)
So, the beta for stock A is 1.2 Similarly, for stock D, ∆
,, %(%) β = = = = 0.75 ∆
,, %(%)
So, the beta for stock D is 0.75
 Stock D is defensive because its beta is less than that of stock A. It means that
stock D return is less sensitive to change in market return.
b. Expected rate of return of a stock is it weighted average rate of return, where the
weights being the probabilities of each scenario .
E(R) = w1R1 + w2R2 + … + wnRn Where,
E(R) = expected rate of return on stock
Wi = weight or probability of scenario i
Ri = rate of return on stock under scenario i
For this part problem states that both the scenarios ie. Bust and Boom are equally likely.
So the probability of each scenario is 50% Wboom = 0.5 Wbust = 0.5
Calculate expected rate of return for market, stock A and stock D by putting the value in the formula . For market:
E(Rm) = wboomR m,boom + wbust Rm,bust
= (0.5 x 32%) + (0.5 x -8%) = 12%
So, the expected return is 12% Similarly for stock A:
E(RA) = wboomRA,boom + wbustRA,bust
= (0.5 x 38%) +(o.5 x -10%) = 14%
So, the expected return is 14% Similarly for stock D:
E(RD) = wboomR D,boom + wbustRD,bust
= (0.5 x 24%) + (0.5 x -6%) = 9% So, the expected return is 9%
c. As per CAPM stock’s fair expected or required rate of return is the return that an
investor expects to get in return for taking the risk by investing in that stock. It is calculated by the formula: r = rf + β(rm – rf) Where,
r = expected rate of return on stock rf = risk free rate
rm= expected return on market portfolio
β = sensitivity of stock return to market return
Problem states that the rf is 4%
From part a: β A = 1.2 βD = 0.75 And from part b: rm is 12%
Calculated the fair expected or required rate of return for stock A and stock D as follows:
For stock A: rA = r f + βA(rm – rf) = 4% + 1.2 x (12% - 4%) = 13.6%
So, the required return is 13.6% Similarly, for stock D:
rD = rf + β D(rm – rf) = 4% + 0.75 x (12% - 4%) = 10%
So, the required return is 10%
d. A stock should be bought if its actual expected or internal rate of return truly reflects
its riskiness and is more than its fair expected or required rate of return.
From part b, actual expected or internal rate of return for stock A and stock D are 14% and 9% respectively.
And from part c, fair expected or required rate of return for stock A and stock D are 13.6% and 10% respectively.
Actual expected or internal rate of return for stock A (14%) is more than its fair expected
or required rate or return (13.6%) whereas actual expected or internal rate of return for
stock B (9%) is less than its fair expected or required rate of return (10%).
Thus, stock A is better buy as compared to stock D as its actual expected or internal rate
of return is more than its fair expected or required rate of return. 3. CAPM and Cost of Capital.
Draw the security market line when the Treasury bill rate is 4% and the market
risk premium is 7%. What are the project costs of capital for new ventures with
betas of 0.75 and 1.75? Which of the following capital investment have positive NPVs? Project Beta Internal Rate of Return % P 1.0 14 Q 0 6 R 2.0 18 S 0.4 7 T 1.6 20
Project costs of capital are calculated as follows using the following information: T-bill rate is 4% Market risk premium is 7%
Use CAPM to compute the project cost of capital as follows when beta is 0.75
Expected return = Rf + β x (market risk premium) Where, Rf is the return on T-bill
Market risk premium is the difference between return on market and return on risk-free rate.
β is the measure of systematic risk.
Expected return = Rf + β x (market risk premium) =4% +0.75 x 7% = 9.25%
Therefore, the project cost of capital when beta is 0.75 is computed as 9.25%.
Use CAPM to compute the project cost of capital as follows when beta is 1.75:
Expected return = Rf + β x (market risk premium) =4% +1.75 x 7% = 16.25%
Therefore, the project cost of capital when beta is 1.75 is computed as 16.25%.
- Computation of cost of capital of Project P using CAPM is shown as follows: When beta is 1.0 (Project P):
Cost of capital = Rf + β x (market risk premium) =4% + 1 x 7% = 11% (< 14%) When beta is 0 (Project Q):
Cost of capital= Rf + β x (market risk premium) =4% +0 x 7% = 4% (< 6%) When beta is 2.0 (Project R):
Cost of capital = Rf + β x (market risk premium) =4% + 2.0 x 7% = 18% (= 18%) When beta is 0.4 (Project S):
Cost of capital = Rf + β x (market risk premium) =4% +0.4 x 7% = 6.8% (< 7%) When beta is 1.6 (Project T):
Cost of capital = Rf + β x (market risk premium) =4% + 1.6 x 7% = 15.2% (< 20%)
NPV of project P,Q,S and T are positive because the opportunity costs of capital is less
than the internal rate of return. 4. CAPM and Valuation.
You are a cónultant to a firm evaluating an expansion of its current business. The
CF forecasts for the project are as follows: Years Cash flow 0 -100 1-10 +15
On the basis of the behavior of the firm’s stock, you believe that the beta of the firm
is 1.4. asssuming that the rate of return available on risk-free investments is 4% and
that the expected rate of return on the market portfolio is 12%, what is the net present value of the project?
The appropriate discount rate for the project is
 =  +  − = 4%+1,4 x (12%-4%)=15.2% Therefore:
NPV=-$100 + [$15 x annuity factor (15.2% .10years)]  = -$100 + $15 x[ −  ]= -$25.29 .
.(. 5. CAPM and Cost of Capital
Reconsider the project in the preceding problem. What is the project IRR? What is
the cost of capital for the project? Does the accept-reject decision using IRR agree with the decision using NPV?
Find the discount rate ( r ) at which
S15 x annuity factor (r,10 years) = 100  S15 x ( −  ) = S100    ()
Solving this equation using trial and error or a financial calculator, we find that the
project IRR is 8.14% . The IRR is less than the opportunity cost of capital (15.2%).
Therefore, you should reject the project, just as you found from the NPV rule. 6. CAPM and Valuation.
A share of stock with a beta of 0,75 now sells for $50. Investors expect the stock to
pay a year-end dividend of $2. The T-bill rate is 4%, and the market risk premium
is 7%. If the stock is perceived to be fairly price today, what must be investors’
expectation of the price of the stock at the end of the year?
From the CAPM, the appropriate discount rate is
 =  +  − = 4% + (0.75 x 7%) = 9.25%
   () r= 0.0925= = = P1 =$52,625   7. CAPM and expected return.
Reconsider the stock in the preceding problem. Suppose investors actually believe
the stock will sell for $52 at year-end. Is the stock a good or bad buy? What will
investors do? At what point will the sotck reach an “equilibrium” at which it again
is perceived as fairly priced?
If investors believe the year-end stock price will be $52, then the expected return on the stock is: $2 ($52  $50)   0.08  8.0% $50
This is less than the opportunity cost of capital. Alternatively, the “fair” price of
the stock (that is, the present value of the investor’s expected cash flows) is: ($2 + $52)/1.0925 = $49.43
This is less than the current price. Investors will want to sell the stock, in the process
reducing its price until it reaches $49.43. At that point, the expected return is a “fair” 9.25%: $2 ($52  $49.43)   0.0925 9.25%  $49.43