lOMoARcPSD|50202050 REVISION Lecture 1: Corporate finance? 3 important qs 3 decisions Forms of business
1. TYPE 1: Calculate Cash Flow from Asset
Ex1.1: Bonner Collision has shareholders' equity of $141,800. The firm owes a total of
$126,000 of which 60 percent is payable within the next year. The firm net fixed assets of
$161,900. What is the amount of the net working capital?
Current liabilities = 0.6 * $126,000 = $75,600
Total assets = Liabilities + SE = $126,000 + $141,800 = $267,800
Current assets = Total assets – Fixed assets = $267,800 - $161,900 = $105,900
Net working capital = CA – CL =$105,900 - $75,600 = $30,300
Ex1.2: Crandall Oil has total sales of $1,349,800 and costs of $903,500. Depreciation is
$42,700 and the tax rate is 34 percent. The firm does not have any interest expense. What is the operating cash flow?
EBIT= Sales Revenue – Costs – Other Exp – Depreciation= 1,349,800 – 903,500 – 0 – 42,700 =403,600
Taxes = (EBIT – Interest Exp) * Tax rate = (403,600 – 0) *34%=137,224
OCF= EBIT + depreciation – Taxes = 403,600 + 42,700 – 137,224= 309,076 lOMoARcPSD|50202050
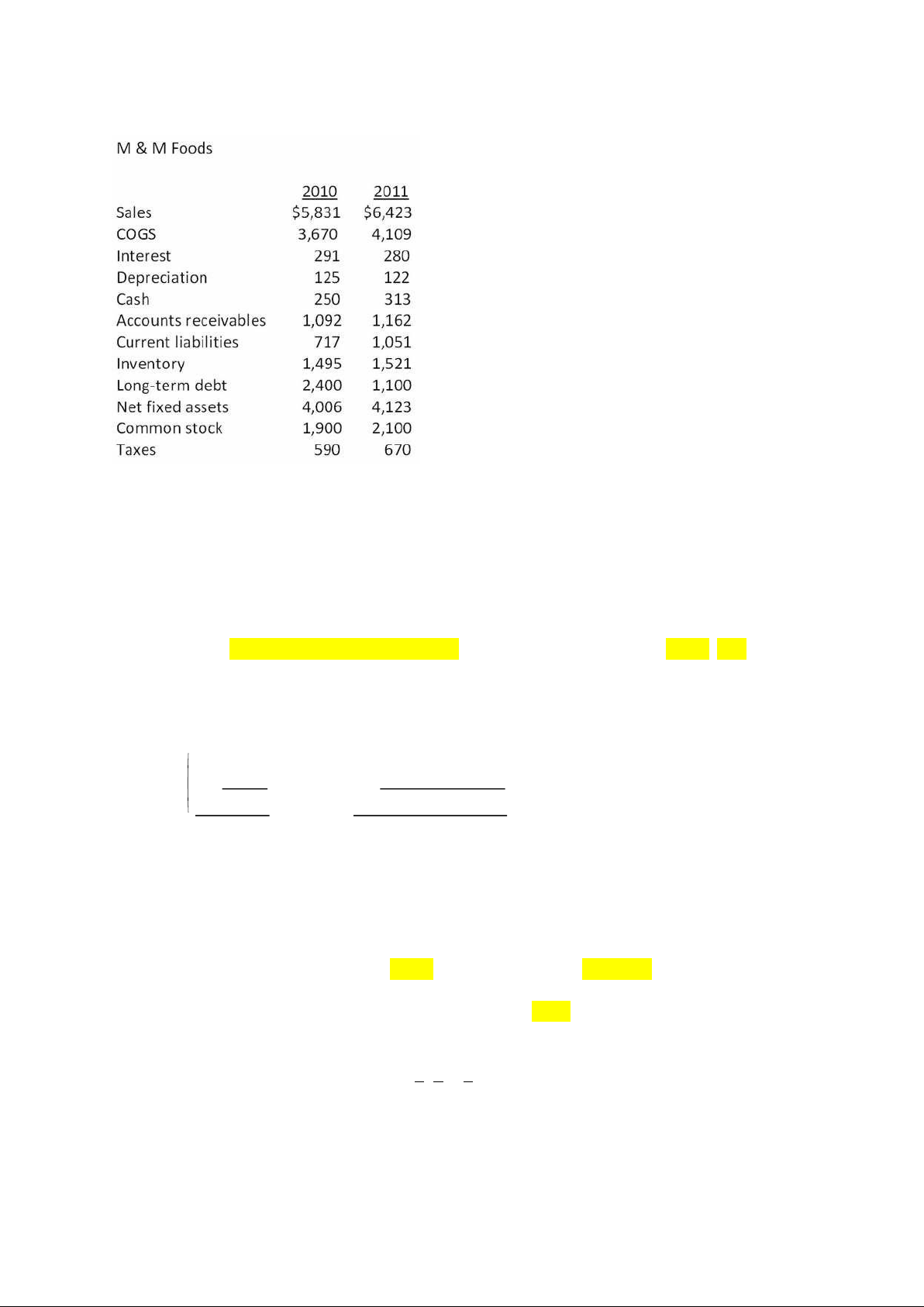
Ex1.3: What is the cash flow from assets for 2011 of M&M Foods
2. TYPE 2: Time value of money (Finding FV, PV of Annuity and Perpetuity)
Ex 2.1: You just won the grand prize in a national writing contest! As your prize, you will
receive $2,000 a month for ten years (t x12). If you can earn 7 percent (year) r/12 on your
money, what is this prize worth to you today? 1 1 1− 1− (1+r)t ) ( ) (1+0.07/12)10×12 172,252.71
PV=C×=2000×=¿ r 0.07/12
Ex 2.2: Alexa plans on saving $3,000 a year and expects to earn an annual rate of 10.25 percent.
How much will she have in her account at the end of 45 years? (1+r )t−1 FV=C×( )=2,333,571.66 r lOMoARcPSD|50202050
Ex2.3: You are planning to save for retirement over the next 35 years. To do this, you will
invest $750 per month in a stock account and $300 per month in a bond account. The return of
the stock account is expected to be 10% per year, and the bond account will pay 6% per year.
When you retire, you will combine your money into an account with a return of 5%.
How much can you withdraw each month from your account assuming a 25-year withdrawal period.
r (stock)= 0.1/12= 0.0083 r (bond)=
0.06/12= 0.005 r (combined account) = 0.05/12= 0.0042
FV (stock)=C×( (1+r)t−1)=750×( (1+0.0083)420−1)=2,818,247.87 r 0.0083
FV (bond )=C×( (1+r)t−1 )=300×((1+0.005 )420−1)=427,413.09 r 0.005
So, the total amount saved at retirement is: 2,818,247.87 + 427,413.09 = 3,245,661
The money you can withdraw each month: 1 ( (1+r)t ) 1− PV=C× r lOMoARcPSD|50202050 1 1− (1+0.0042) ( 3,245,661=C× 25×12 )=¿C=19,049.53 0.0042
Ex2.3: You borrow $165,000 to buy a house. The mortgage rate is 4.5 percent and the loan
period is 20 years. Payments are made monthly. If you pay the mortgage according to the loan
agreement, how much total interest will you pay? 1 ( (1+r)t ) 1− PV=C× r 1 1− 4.5% 20× 12 ( (1+ ) ) 12 165000=C× =¿C=$1,043.9 4.5% lOMoARcPSD|50202050 12
Total interest = $1,043.9*20*12 – 165000= $85,536
3. TYPE 3: Bond Valuation
Ex3.1: Grand Adventure Properties offers a 9.5 percent coupon bond with annual payments.
The yield to maturity is 11.2 percent and the maturity date is 11 years from today. What is the
market price of this bond if the face value is $1,000? Face Value = $1000 C= $1000 x 9.5%= 95 YTM= 11.2% t = 11 years 1 1 1− 1− ( ) ) Market price = ( ) ) 1+r ( ) ( ( t FV t (1+11.2% 11 1000 )= C×+ =95× + r (1+r) 11.2% (1+11.2%)11 $895.43
4. TYPE 4: Stock Valuation
Ex4.1: How much are you willing to pay for one share of Jumbo Trout stock if the company
just paid a $0.70 annual dividend, the dividends increase by 2.5 percent annually, and you
require a 10 percent rate of return? D0(1+g) 0.7(1+2.5%) P0= = =$9.57 R−g 10%−2.5% lOMoARcPSD|50202050
Ex4.2: Free Motion Enterprises paid a $2 per share annual dividend last week. Dividends are
expected to increase by 20 percent in year 1 and 15% in year two. After that dividends are
expected to increase by 3 percent annually. What is one share of this stock worth to you today
if your required rate of return is 13 percent? (3 points) Dt(1+g) Formula:Pt= R−g
D1=D0 (1+g)=2 (1+0.2)=$2.4
D2=D1 (1+g )=2.4 (1+0.15)=$2.76 P2=
DR2(−1+gg)=2.760.2(−1+0.030.03)=$16.72 D1 D2 P2 2.4 PV= 1 + 2 + 2= 1 + + =$17.38 (1+r) (1+r) (1+r) (1+0.13)
Ex4.3: The current dividend yield on Clayton's Metals common stock is 3.2 percent. The
company just paid a $1.48 annual dividend and announced plans to pay $1.54 next year. The
dividend growth rate is expected to remain constant at the current level. What is the required
rate of return on this stock? (tìm r) P0 D (10 g) D1 R - g R -g lOMoARcPSD|50202050 R D (10 g) g D1 g P0 P0
Making the stock price is X with the next dividend at $1,54 1.54
We have: 1,54=0,032×X=¿X= =$48,13 0.032 The growth rate is: 1.54−1.48 g= D1−D0= =0,0405=4.05% D0 1.48 D1
The required rate of return on this stock is: R= +g= +0.0405=0.0725=7.25% P 48.13
5. TYPE 5: Capital Budgeting Techniques
Ex 5.1: You are considering the following two mutually exclusive projects. The required rate
of return is 14.6 percent for project A and 13.8 percent for project B. The management numbers
of payback and discounted payback periods for both projects are 3 years. Which project should
you accept based on NPV/ Payback/ Discounted Payback Analysis. lOMoARcPSD|50202050 NPV: Project A: PV cash¿ ( + ) ( + ) ( + ) =PV NPV
cash∈¿−PV cashout=$63,157.24−$50,000=$13,157.24 >0¿ Project B: PV cash¿ ( + ) ( + ) ( + ) PV NPV=
cash∈¿−PV cashout=$58,256.98−$50,000=$8,256.98>0¿
Based on the Net Present Values of the two projects, Project A should be accepted. Payback period Project A
Year 1: 50,000 – 24,800 = 25,200
Year 2: 25,200 – 36,200 = -11,000 lOMoARcPSD|50202050 PP=1+ =1,97 years Project B
Year 1: 50,000 – 41,000 = 9,000
Year 2: 9,000 -20,000 = -11,000 PP=1+ =1,45 years
Based on the PP of the two projects, project B should be accepted. Because we recover our cost of the project early. Discounted Payback: Project A: FV 24,800 PV1= t = 1=$21,640.49 (1+r) (1+14.6%) FV 36,200 PV2= t = 2=$27,563.82 (1+r) (1+14.6%) FV 21,000 PV3= t = 3=$13,952.93 (1+r) (1+14.6%)
DPP: Year 1: $50,000 – 21,640.49 = $28,359.51
Year 2: $28,359.51 – 27,563.82 = $795.69 lOMoARcPSD|50202050
Year 3: $795.69 – 13,952.93 = -13,157.24 DPP=2+ =2,06 years Project B: FV 41,000 PV1= t = 1=$36,028.12 (1+r) (1+13.8%) FV 20,000 PV2= t = 2=$15,443.49 (1+r) (1+13.8%) FV 10,000 PV3= t = 3=$6,785.36 (1+r) (1+13.8%)
DPP: Year 1: $50,000 – 36,028.12 = 13,971.88
Year 2: $13,971.88 – 15,443.49 = -1,471.61 DPP=1+ =1,90 years
Based on the DPP of the two projects, project B should be accepted. Because we recover our cost of the project early.
Ex 5.2: Day Interiors is considering a project with the following cash flows. What is the IRR of this project? IRR: Internal Rate of Return lOMoARcPSD|50202050
NPV=0 => PV cash∈¿¿= PV cashout
1 + 2 + 3 =114,600=¿R=0,0703=7.03%
(1+R) (1+R ) (1+R)
6. TYPE 6: Compute expected return, SD of portfolio
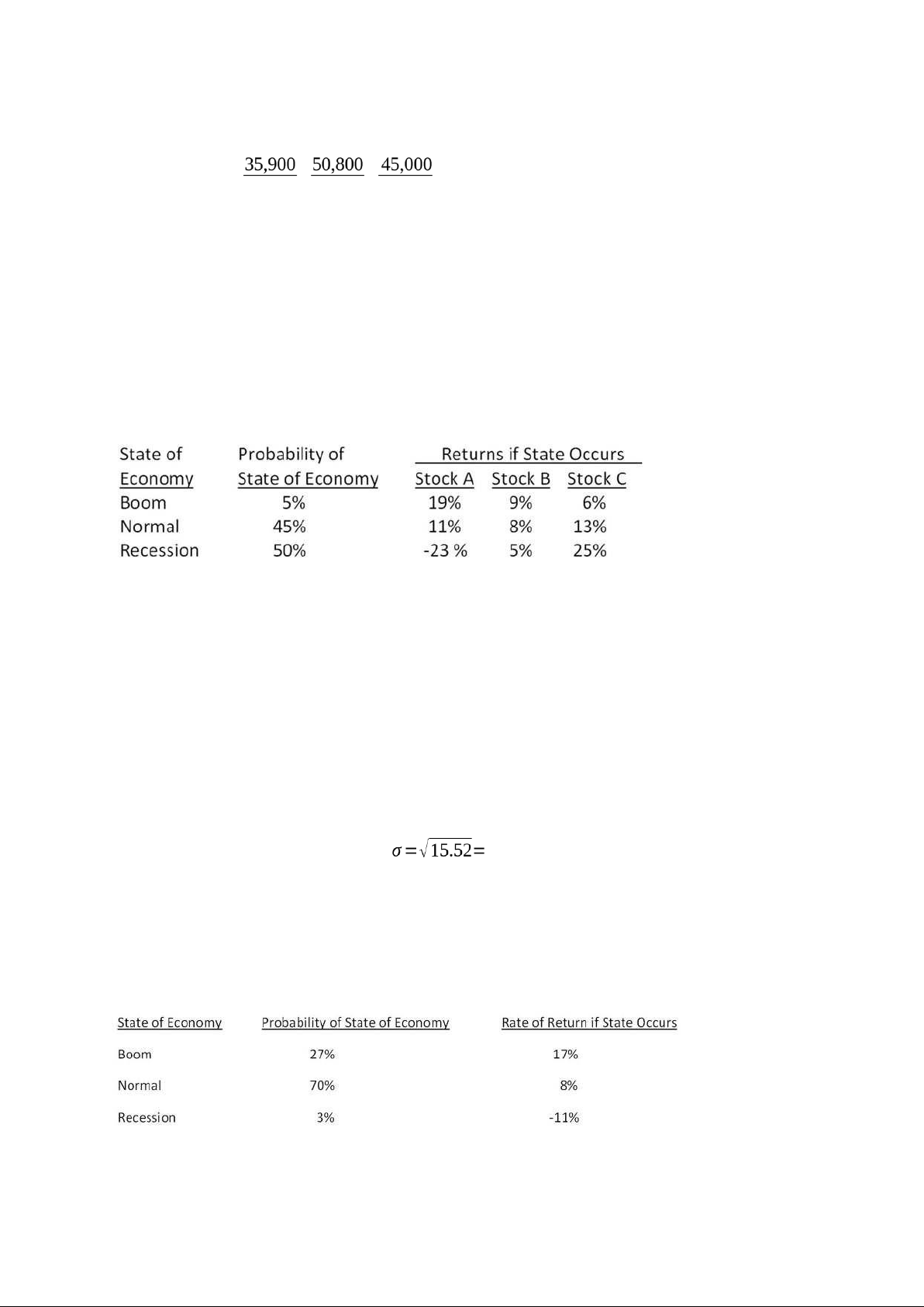
Ex 6.1: What is the expected return and SD on a portfolio which is invested 25 percent in stock
A, 55 percent in stock B, and the remainder in stock C?
Boom: RP=0.25×19+0.55×9+0.2×6=10.9%
Normal: RP=0.25×11+0.55×8+0.2×13=9.75%
Recession: RP=0.25×(−23)+0.55×5+0.2×25=2% E(R )
P ¿0.05×10.9+0.45×9.75+0.5×2=5.93% σ 2
P =0.05× (10.9−5.93)2+0.45× (9.75−5.93)2+0.5× (2−5.93)2=15.52 3.94%
Ex 6.2: You own a portfolio with the following expected returns given the various states of the
economy. What is the overall portfolio expected return and SD?
E(R)¿.27 (17)+.7 (8 )+.03 (−11)=9.86% lOMoARcPSD|50202050
σ2=.27 (17−9.86)2+.7 (8−9.86)2+.03 (−11−9.86 )2=29.24 5.41% 7. TYPE 7: WACC
Ex 7.1: Mangrove Fruit Farms has a $250,000 bond issue outstanding that is selling at 92
percent of face value. The firm also has 1,500 shares of preferred stock and 15,000 shares of
common stock outstanding. The preferred stock has a market price of $35 a share compared to
a price of $24 a share for the common stock. What is the weight of the preferred stock as it
relates to the firm's weighted average cost of capital? V = D + E + PS
This is the % financed with debt: WD = D/V
This is the % financed with equity: WE = E/V
This is the % financed with Preferred stock: Wps=PS/V
Debt: $250,000 x 0.92 = $230,000
Preferred: 1,500 x $35 = $52,500
Common: 15,000 x $24 = $360,000
V= Debt + Common + Preferred stock = $642,500
W(PS)= PS/V = $52,500 ÷ $642,500= 8.17%
Ex 7.2: Boulder Furniture has bonds outstanding that mature in 15 years, have a 6 percent
coupon, and pay interest annually. These bonds have a face value of $1,000 and a current
market price of $1,075. What is the company's after tax cost of debt if its tax rate is 32 percent? lOMoARcPSD|50202050
After-tax cost of debt = RD(1-TC) 1 PV=C× (1r+r)t
)+(1FV+r)t 1− PV= C N= 15 years PV= -1,075 Face value = 1000 C= 0.06*1000=60 1 1− ) (1+r)15 1000 1,075=60×r
+(1+r)15 =¿RD=0,0526=5,26%
After-tax cost of debt = RD(1-TC) = 0,0526(1-0,32) = 0,0358 =3,58%
Payback, discounted payback, NPV, PI, IRR
Chap 13 / E(RA)/ E(R)/ risk: standard deviation (%) / variance: (k co don vi) CAPM