Cal1 Mid S22021 22 with Answer key short
Tài liệu học tập môn Calculus 1 tại Trường Đại học Quốc tế, Đại học Quốc gia Thành phố Hồ Chí Minh. Tài liệu gồm 5 trang giúp bạn ôn tập hiệu quả và đạt điểm cao! Mời bạn đọc đón xem!
Môn: Calculus 1 (MA001IU)
Trường: Trường Đại học Quốc tế, Đại học Quốc gia Thành phố Hồ Chí Minh
Thông tin:
Tác giả:
Thông tin :
Môn:
Trường:
Trường Đại học Quốc tế, Đại học Quốc gia Thành phố Hồ Chí Minh
Tác giả:
Tài liệu liên quan:
Preview text:
lOMoARcPSD|364 906 32 Calculus 1 Answer key for the Midterm Page 1 of 5
PART 1: MULTIPLE CHOICE QUESTIONS
No calculator is allowed in this part. x2023 −1
1. The limit lim is x→1 x−1 (A) ∞ (B) 2023 (C) 2021 (D) 2022 Answer: (B) xn −1
f(x)− f(1)
Generally, the limit lim = lim = f ′(1) = n by the definition of derivative. The value of n can be n →1 − 1 → 1 − 1 = , 2021 = 2023 = 2024 in some exam versions.
2. A bacteria culture initially contains 100 cells and grows at a rate proportional to its size, that is,dP
= kP, where k is a positive constant. After an hour the population has increased to 400. The dt
number of bacteria after t hours is
(A) P(t)= 100e(ln4)t
(C) P(t)= 300tet−1 +100
(B) P(t)= 100+300t
(D) P(t)= 300t2 +100 Answer: (A)
3. If the point (−1,2) lies on the graph of y= f(x) then which point must lie on the graph of y= f −1(x)? 1 1 ( − 1 , ) B ( , − 1 ) 2 2 (C) (1,−2) (D) (2,−1). Answer: (D)
4. Which of the following is the inverse of f(x)= ln(x−2) for x > 2? = 1 ln ( − 2 )
(A) f −1(x)= ex−2 (B) y
(C) f −1(x)= ex +2
(D) f −1(x)= ex −2 Answer: (C)
5. If x6 −2 f f(x) f x2 −2 for −1 f x f 1, then limx→0 f(x) is (A) undefined (B) 2 (C) 0 (D) −2 Answer: (D)
6. Given a curve with equation 2x2 +xy−4y3 = 10. The slope of the tangent line to this curve at the point (2,−1) is 1 7 2 (B) 1 (C) 10 (D) 2 lOMoARcPSD|364 906 32 Calculus 1 Answer key for the Midterm Page 2 of 5 Answer: (C)
7. The displacement s of a particle (in meters) at time t seconds is given by s(t)=t4 −3t3 +4t2 +2t−1.
The acceleration of the particle (in m/s2) at time t = 1 (s) is (A) 2 (B) 3 (C) 4 (D) None of the above Answer: (A)
8. If the side x of a cube (a cubical box) is increased by 3%, then the volume is increased approximately by (A) 0.03x (B) 0.09x3 (C) 0.06x (D) 8% Answer: (B)
(Note: V(x)= x3. Thus, dV =V′(x)dx = 3x2dx = 0.09x3 with dx = 0.03x.)
9. The cost C in dollars of producing x units of a product is C(x)= 4000+20√x+10x+0.01x2. At a production
level of 100 units, the marginal cost is (A) $4000 /unit (B) $12 /unit (C) $4300 /unit (D) $13 /unit Answer: (D)
(the marginal cost is C′(x) at x = 100 units).
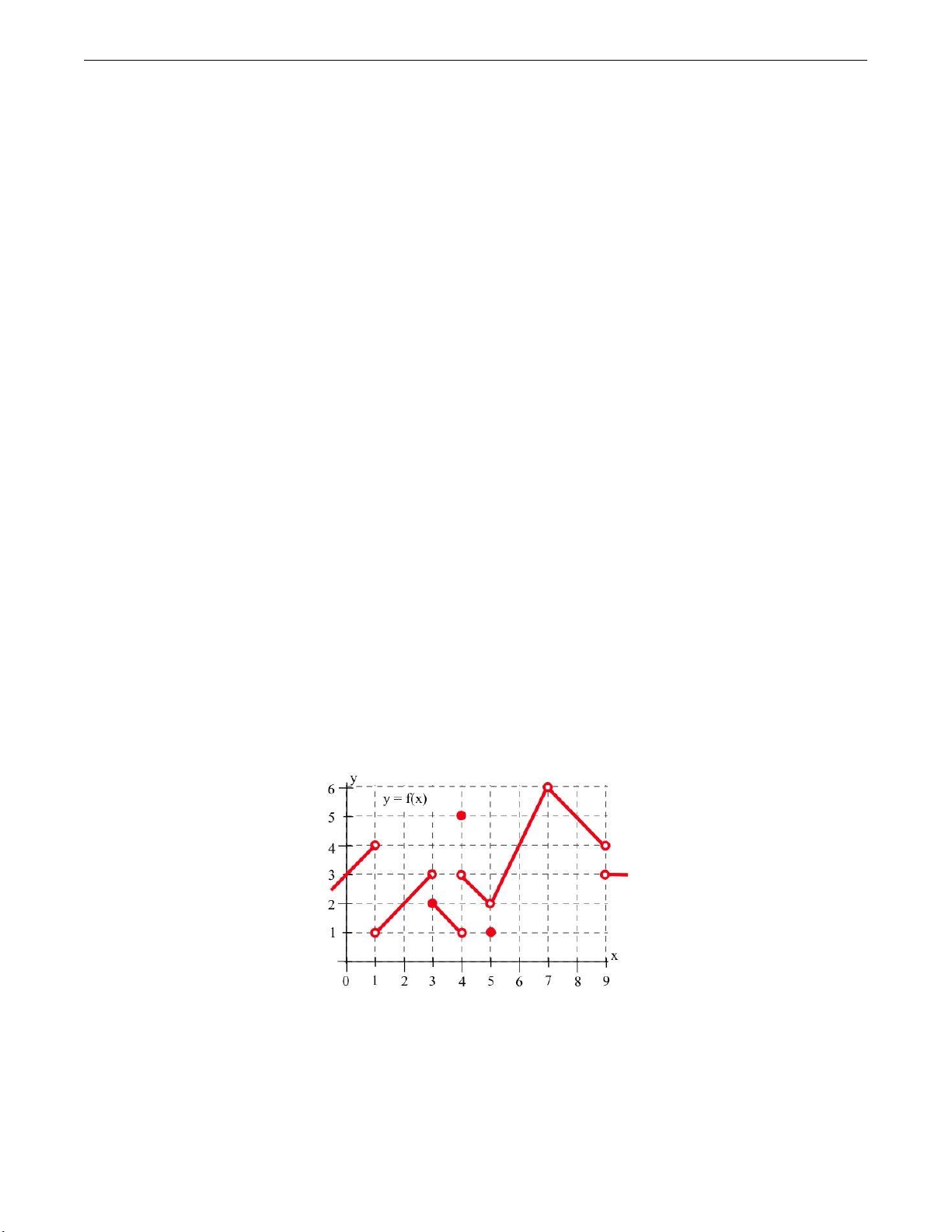
10. For the function f whose graph is given below. Find lim f(x) and lim f(x) x→1 x→5 lOMoARcPSD|364 906 32 Calculu ( s A )1
lim f(x)= 1 and lim f(x)= 2 An
x→1swer key for the (M C i)d ter li m
m f (x) is undefined and lim f(x Pa )= g e 3 of 5 x→5 2 x→1 x→5
(B) lim f(x) is undefined and lim f(x)= 1
(D) lim f(x)= 4 and lim f(x)= 2 x→1 x→5 x→1 x→5 Answer: (C)
11. The derivative of f(x)= x2 sin−1 x is (A) ′ 2 sin−1 x2 (C) ′ 2 sin−1 x2 f (x)= x x+ √ − f (x)= x x+ 1+x2 1 x2 2
(B) f ′(x)= xsin−1 x+ √1 −x x2 (D) None of them Ans: (A)
12. Consider the following piecewise function:
x+−2 if x f 1 f f(x)= x2
2x+4 if 1 < x 2
4−x if x > 2.
(A) f is discontinuous at x = 1 (C) f is continuous at both x = 1 and x = 2
(B) f is discontinuous at x = 2 (D) None of these. Ans: (B)
13. Let g(x)=p6+4f(x),
f(1)= 5 and f ′(1)= 1. Find g′(1). (C) 0 2 1 1 √ 26 (A) √ (B) √ 2 26 2 26 Ans: (D) 4 (An answer in form of √
is also correct. Additionally, (A) and (B) are the same but the answer of 2 26 1 √ 2 26 is incorrect). lOMoARcPSD|364 906 32 Calculus 1 Answer key for the Midterm Page 4 of 5
14. The value of lim | | is x+2 x→−2− x+2 (A) 0 (B) 1 (C) −1 (D) undefined Ans: (C)
(Note: |x+2| = −(x+2) if x < −2. Therefore, |
| = −1 if x < −2.) x+2 x+2
15. A particle moves along a horizontal line with its displacement s(t) given by s = 1+10t −5t2, where s is
measured in meters and t in seconds. At which time below is the particle moving forward? (A) t = 0.5 (B) t = 2 (C) t = 3 (D) t = 4 Ans: (A)
16. Let f(x)= √3 x and g(x)= √x+14. Find (g◦ f)(8). √ (A) 3 (B) 2 (C) 4 (D) 14 Ans: C
17. Let f(x)= 1+x+e3x. Find f −1(2). (A) −1 (B) 2 (C) 4 (D) 0 Ans: (D) (clearly, f(0)= 2.)
18. Suppose that f is continuous on [0,5] and the only solutions of the equation f(x)= 5 are x = 1 and x = 2. If f(3)= 4 then (A) f(4) < 5 (B) f(4) > 6 (C) f(4) > 5 (D) f(4) > 7 Answer: (A) − 1 ′ ( 4 )
19. Let f(x)= x3 +3x . Find f . 1 1 1 B (A) 2 3 (C) 6 (D) None of them Ans: (C) − 1 ′ 1 1 1 ( 4 )= ′ = = − 1 ′ . )
(By derivative of the inverse, f ( ( 4 )) ( 1 ) 6
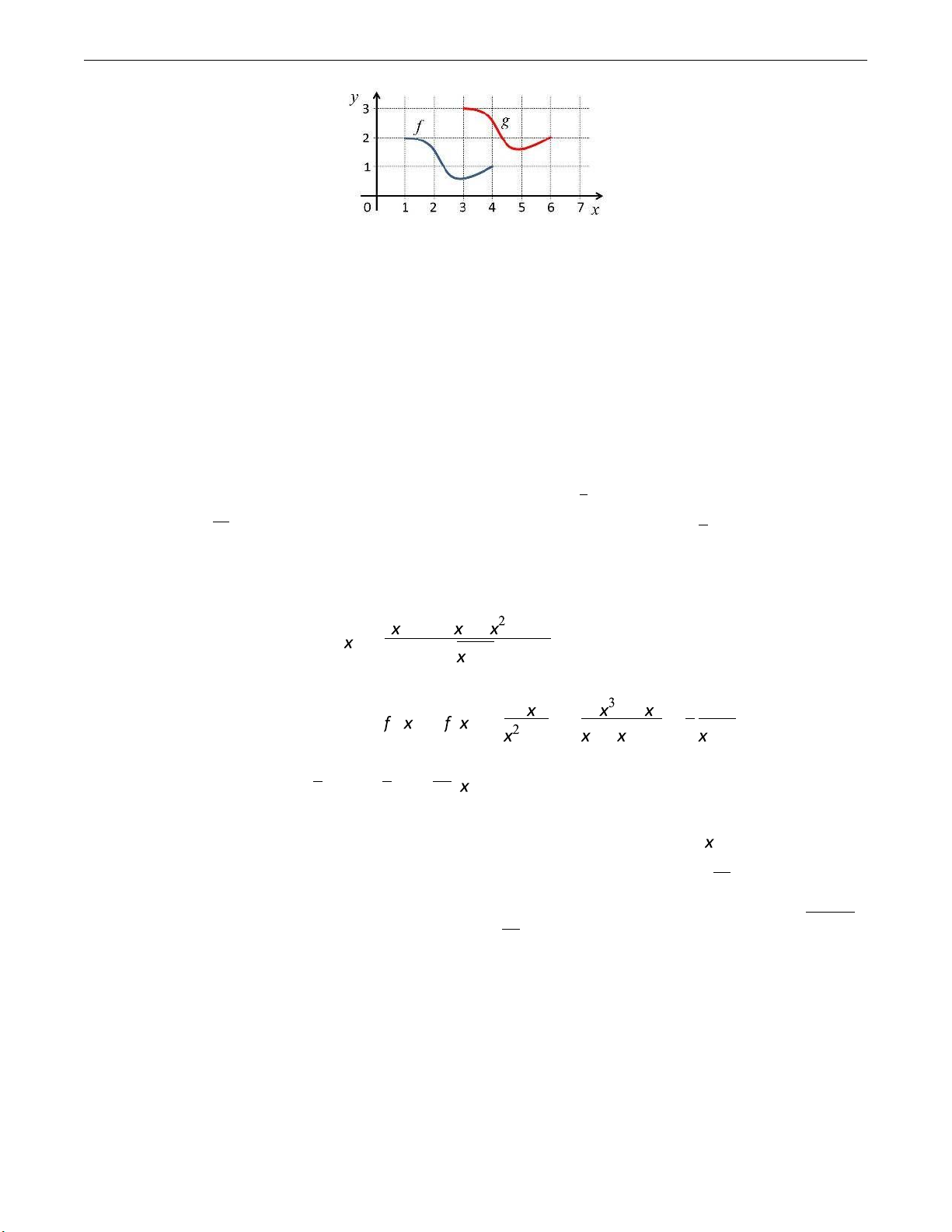
20. Consider the functions f and g shown in the figure. The relationship between these functions is: lOMoARcPSD|364 906 32 Calculus 1 Answer key for the Midterm Page 5 of 5
(A) g(x)= f(x+1)−2
(C) g(x)= f(x−1)+2
(B) g(x)= f(x+2)+1
(D) g(x)= f(x−2)+1 Ans: (D) —END OF PART 1— Answer key for Part 2 1 1
1. Since f ′(x)= √ , the linearization of the function f(x)= √x at x = 16 is L(x)= 4+ (x−16). 2 x 8 2. Given ( 5 + 1 ( 2 + 1 ) 4 − )= √ f + 4
for x > −4. ′ 2 4 − 2 1 1 ( )= ( ) × ( + 5 − ) + 1 4 − 2 + 1 2 + 4
(a) Use logarithmic differentiation: . 1 1 1
( 0 , ) is y − = − ( − 0 ) (b) The tangent line at 2 2 16 . ′ = 4 −
3. (a) Differentiate 4x2 +y2 = 4 with respect to x, to obtain 8x+2yy′ = 0 so y y . x y xy
(b) To obtain y′′, differentiating both sides of y′ = −
4 with respect to x, it yields: y′′ = −4 −2 ′ . y y
Hence, at (0,−2) we have y′ = 0 and, therefore, y′′ = 2. -The end- lOMoARcPSD|364 906 32 Calculus 1 Answer key for the Midterm Page 6 of 5
Document Outline
- PART 1: MULTIPLE CHOICE QUESTIONS
- Answer: (B)
- Answer: (D)
- Answer: (D) (1)
- Answer: (C)
- Answer: (A)
- Answer: (B) (1)
- Answer: (D) (2)
- Ans: (A)
- Ans: (B)
- 1 1 (C) 0
- Ans: (D)
- Ans: (C)
- Ans: (A)
- Ans: C
- Ans: (C) (1)
- Answer key for Part 2