THE INTERNATIONAL UNIVERSITY (IU) Course: Engineering Probability and Statistics
Department of Industrial and Systems Engineering MIDTERM EXAMINATION
ENGINEERING PROBABILITY AND STATISTICS Duration: 120 minutes Head of Dept of ISE Lecturer: Student ID: Date: November 14th, 2018 Dr. Ha Thi Xuan Chi Name: Dr. Phan Nguyen Ky Phuc Dr. Nguyen Van Hop Ho Thanh Vu INSTRUCTIONS: 1.
This is an open book examination. 2.
Calculator is allowed. Laptops, mobile phone and the other electrical devices are strictly prohibited. 3.
All answers should be presented in the Answer sheet. Total pages: 0 (including this page) Question 01: (15 pts)
The monthly sales of a bookstore is given as follow: 13 43 53 44 14 33 18 55 50 25 27 45
Use the given data to answer questions below:
a) Calculate the mean and variance (5 pts)
b) Calculate the median and third quartile (5 pts)
c) Given that at random days, the sales are 30, and 40 books. Which percentile these data value belong to? (5 pts) Question 02: (10 pts)
A tennis tournament with knockout format is arranged for 8 players will consist of quarterfinal round, semifinal round,
and a grand final match. In any match either player has a probability 0.50 of winning. Assume we have two specific
player called Djokovic and Federer among the players.
a) What is the probability that they meet each other in the semi-finals? (5 pts)
b) What is the probability that they meet each other in the grand final? (5 pts) Question 03: (10 pts)
The following game is offered. There are 10 cards face-down numbered 1 through 10. You can pick one card. Your
reward is $0.50 if the number on the card is less than 3, $1 if less than 5, and is the dollar value on the card otherwise.
a) What is the probability your reward is between $2 and $6 inclusively? (5 pts)
b) What are the expected value and the standard deviation of your reward? (5 pts) Question 04: (15 pts)
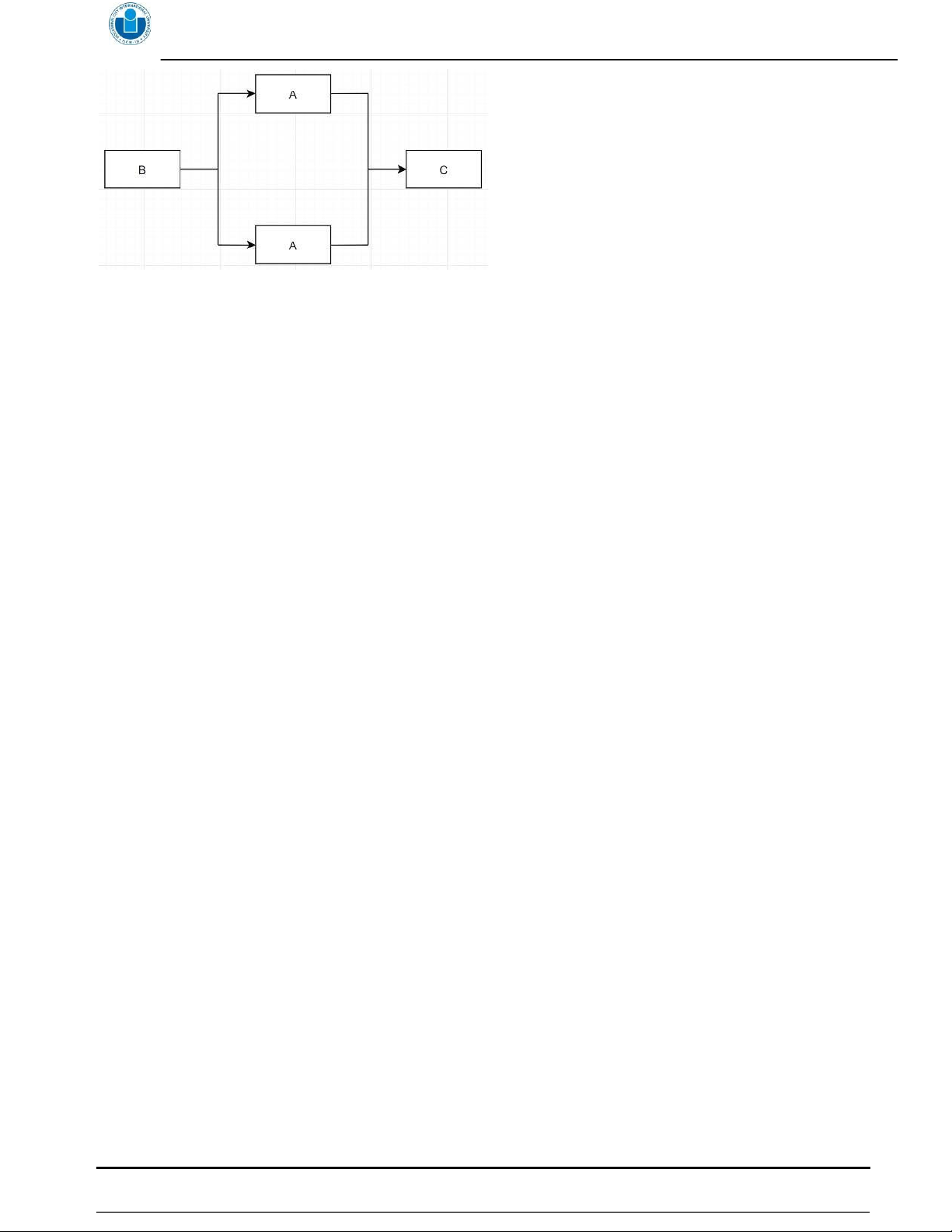
The lifetime of electrical components A, B, and C are known to follow an exponential distribution with mean 15, 20, and 25 days, respectively.
a) If component A is used separately, what is the probability that it will fail after 23 days? (5 pts)
b) Consider a system constructed as in the figure given below, calculate the probability that the system will fail
after 30 days? (10 pts)
Engineering Probability and Statistics – Midterm Exam
THE INTERNATIONAL UNIVERSITY (IU) Course: Engineering Probability and Statistics
Department of Industrial and Systems Engineering Question 05: (20 pts)
Andrew plays the following game. First he flips a coin with a 1 painted on one side and a 2 painted on the other. The
number on the coin determines how many marbles he gets to draw (without replacement) from an urn containing 7
blue marbles and 5 green marbles (he draws the marbles in succession, not at the same time). Once he’s drawn the
allowed number of marbles, he wins if at least one of the marbles drawn is green
a) What is the probability of winning the game? (10 pts)
b) Given that Andrew win the game, what is the probability that he had gotten 2 on the coin flip? (10 pts) Question 06: (10 pts)
There is a game consists of 2 players called X and Y. They are competing in a series of games in which probability of
X wins over Y is independent of each round with a constant rate of 0.57. Each time a player win a round he/she
receives 1 point, the series stops when a player got 3 points.
a) What is the probability that the series stop after 4 rounds? (5 pts)
b) Assume they can reset the series and play again for another 7 time. What is the probability among these 7
times, the series stop after 4 rounds exactly 4 times? (5 pts) Question 07: (20 pts)
International University is interested to know more about IU student’s part time job, in both quality and quantity.
First they start with the most crucial factor: the salary. After a long and tedious process they observe that the
monthly salary of IU’s student part time job is actually a random variable, which follows Normal distribution with
mean 㔇 = $200, and standard deviation 㔎 = $45.
a) Select a random IU student who does have a part time job, what is the probability that his/her monthly salary
is between $190 and $235? (5 pts)
b) Select a group of 40 IU students who all have part time job, what is the probability that their total monthly
salary exceeds $8,300? (5 pts)
c) Select a group of 12 IU students who all have part time job, what is the probability that their average monthly
salary exceeds $220? (5 pts)
d) Furthermore, a monthly salary over $220 is referred as who all have part time job. What is the probability we have at most 4 (5 pts)
ALL INFORMATION USED IN THIS EXAMINATION IS FICTIONAL AND CREATED SOLELY
FOR THE PURPOSE OF TESTING STUDENTS. GOOD LUCK!
Engineering Probability and Statistics – Midterm Exam