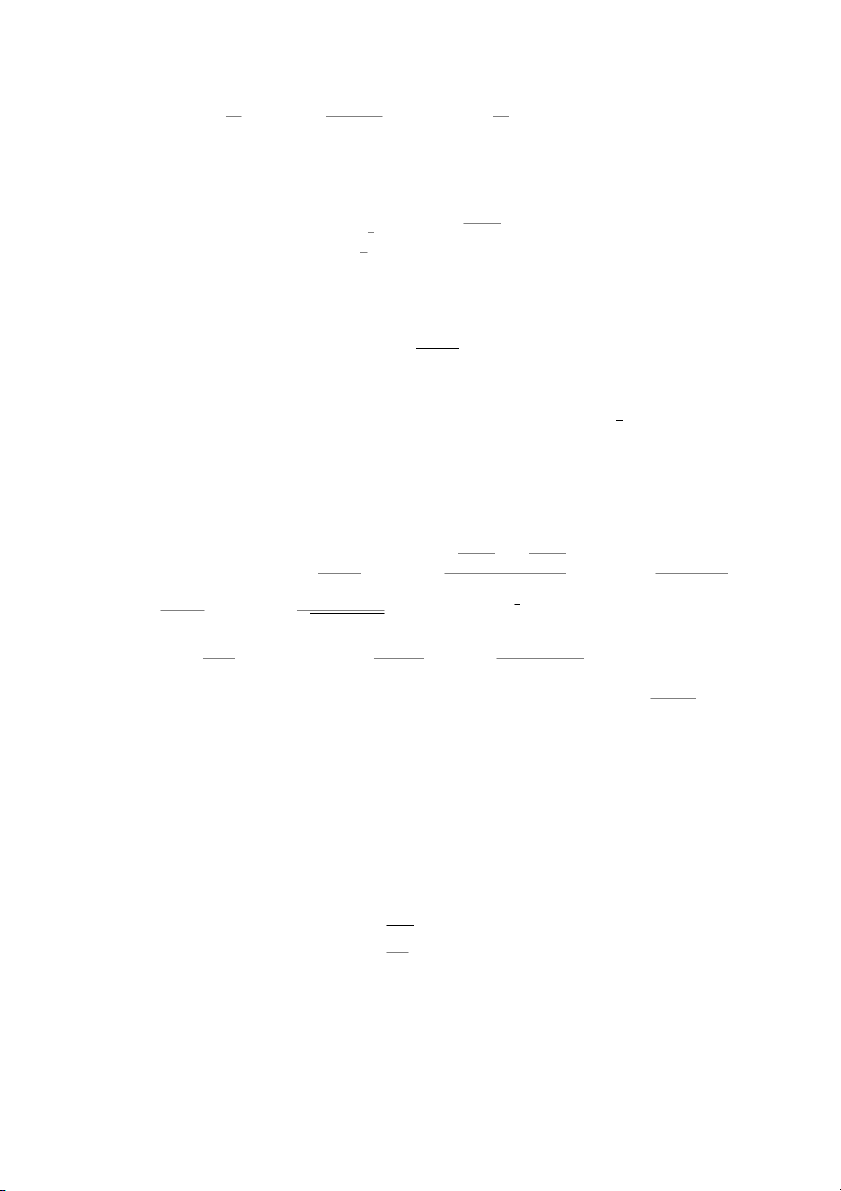
Preview text:
EXERCISES for Chapter 1 of Calculus 1 Dr Janet, Sem 1, 2020/21
Write your answers on A4-sized paper. Some weeks your work will be collected for marking.
*Question 10 does not need to be submitted.
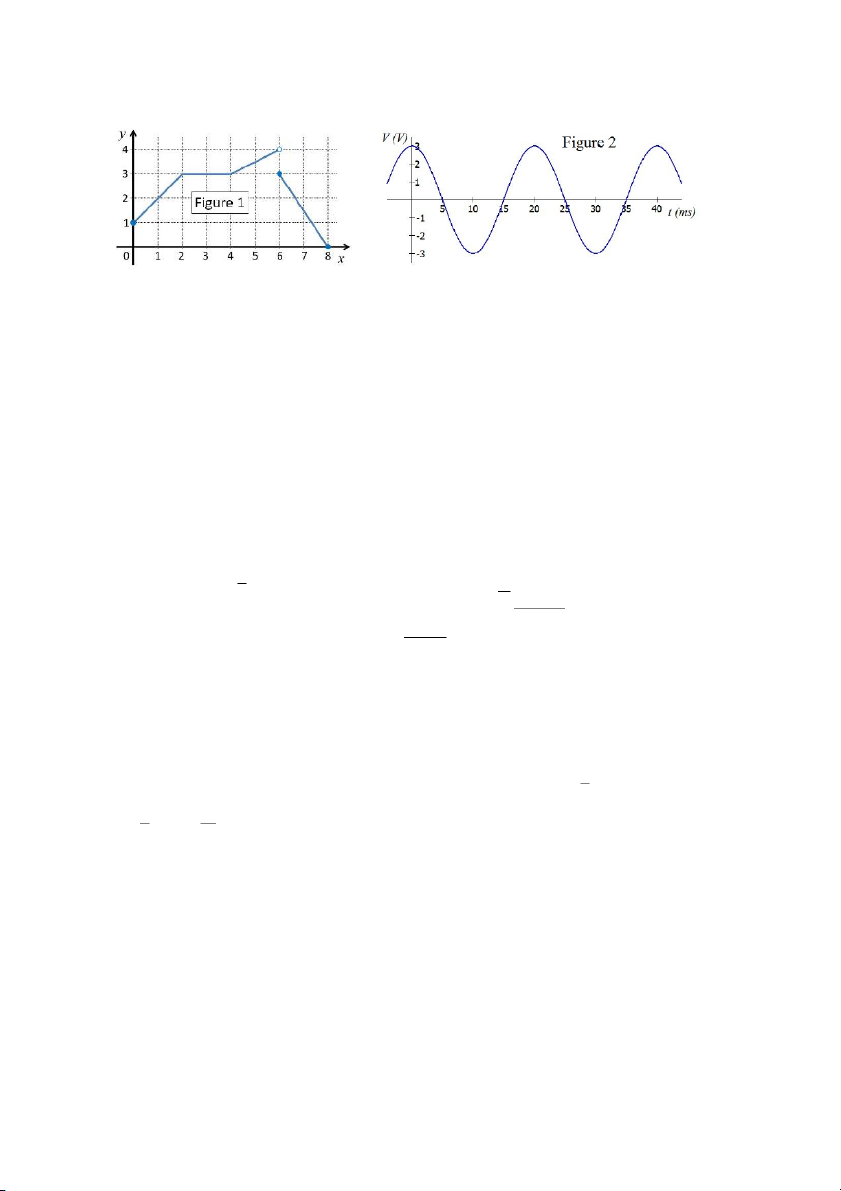
1. Consider the function in Figure 1 (above). State the slope of the graph at a) x = 1, b) x = 3, c) x = 5, d) x = 7. 2.
Sketch graphs of the following equations. (Do this not by plotting points but by
identifying the slope and y-intercept.) a) y = x + 2, b) y = 2x − 4, c) x + 2y = 3.
3. Biologists have observed that the chirping rate of a certain insect is linearly related to
temperature. At a temperature of 150C, the insect produces 47 chirps per minute, and at
300C produces 209 chirps per minute. a) Sketch a graph of the chirping rate as a function
of temperature, and find the equation of the line. b) State the slope of the line, including
its units, and explain its meaning to the biologists.
c) If the insects are chirping at 236
chirps per minute, what is the temperature?
4. Each day, a certain furniture factory currently produces 60 chairs with a total production
cost of $570. The production cost may be considered to be linearly dependent on the number
of chairs produced. It is estimated that for every 8 extra chairs produced, production costs
will increase by $52. Find an equation for the cost function (that is, the production cost as
a function of number of chairs produced). For $1000, how many chairs can be produced? √
5. Let f (x) = 1 + 3 x. Find a) f (8), b) f (x + 2), c) f ( 1 ). x3 √
6. a) State the domain and range of the function f (x) = 4 − 3x2.
b) State the domain of the function g(x) = x . x2−x−2
7. Find a formula for the area of an equilateral triangle as a function of the side length, x. 8.
Consider a closed cylindrical can with volume 1 m3. Find an expression for the total
surface area as a function of the base radius r.
9. Sketch an approximate graph of distance as a function of time for your daily journey from your home to IU.
10.* Check that you can sketch the graphs of the following common equations (from memory √
– without plotting points or other working): y = x2, y = x3, y = x, y = |x|, 1 1 y = , y =
, y = sin x, y = cos x, y = tan x, y = ex, y = ln x, x2 + y2 = a2. x x2
11. Circle C is centred at (2, 0) and has radius 3. Write a function y = f (x) for the upper half of circle C.
12. Is the given function is odd, even or neither? a) f (x) = xe−x2, b) g(x) = x3 + 1.
13. A taxi company charges a fixed price of 10,000 VND for travelling up to 500 m, then an
additional 2000 VND for every additional 100 m (or part of 100 m). Write a formula for the
total cost C (in VND) as a function of the distance x traveled (in meters) for 0 < x < 1000,
and sketch the graph of this function. [Hint: This is a piecewise function.]
14. Write an equation for the piecewise function shown in Figure 1 (above) and state the
domain and range of the function. 1
15-16. Sketch graphs of the following equations. (Do this not by plotting points but by
starting with the graph of a known function and applying appropriate transformations.) 1 1 1 15. a) y = , b) y = , c) y = 1 − . 16. y = |1 − x2| x2 (x + 2)2 x2
17. Let f (x) = cos x, 0 ≤ x ≤ 2π. a) Sketch the graph of f(x). Also find expressions for
and sketch graphs of: b) 3f (x), c) f (πx), d) f (x − π/4).
18. Figure 2 shows a sinusoidal electrical voltage. Write an expression for V (t) where time
t is measured in milliseconds (ms). [Note: A sinusoidal function is a sine or cosine function.] √ 19.
Given f (x) = 1 + cos x, g(x) = 2, h(x) =
x − 1, a) find f ◦ g ◦ h and state its x
domain and range, b) find (g ◦ h ◦ f)(π). 3
20. For the function f (x) shown in Figure 1, find f (3), f (6), (f ◦ f)(5) and (f ◦ f)(8).
21. Draw a curve of a function y = f (x) with the given properties:
a) domain [0, 3], range [0, 2], one-to-one; b) domain (0, 3], range [0, 2), not one-to-one. 1 − ex
22. Find the inverse of the function f (x) = . 1 + ex
23. Write down parametric equations: a) for the line segment from (1, 5) to (3, −1),
b) to traverse the circle (x − 1)2 + y2 = 4 once travelling i) anti-clockwise, ii) clockwise.
24. Using a table of values, sketch the parametric curve x = t2, y = 2 sin( πt), −3 ≤ t ≤ 3. 2 Mark the direction of travel.
25. A bullet is fired from ground level at time t = 0 and follows a trajectory x = 45t,
y = 60t − 5t2, 0 ≤ t ≤ 12 where x is horizontal distance and y is vertical height, both
measured in metres, after t seconds. Assuming the ground is flat, how far away does the bullet hit the ground?
26-32. Find the limit or show that it does not exist. √ √ 4 − t 1 + x − 1 − x 1 + x − x3 26. lim cos(x+sin x), 27. lim , 28. lim , 29. lim . x→0 t→4+ |4 − t| x→0 x x→∞ 2 + x2 x2 − 1 t + 2 30. lim , 31. lim √ ,
32. lim x2ecos 2x [Hint: use Squeeze theorem]. x→−∞ x3 + 3 t→∞ 3t2 − t + 1 x→0 sin x sin(3x) 3x − sin(2x) 33. Given lim = 1, find a) lim , b) lim . x→0 x x→0 x x→0 x + sin x 2x − 1
34. Identify all the horizontal and vertical asymptotes of the function f (x) = . x − 2 ex if x ≤ 0
35. Consider the function g(x) = x2 if 0 < x < 1 a/x if x ≥ 1
a) Find the limits lim g(x), lim g(x), and lim g(x). Is g(x) continuous at x = 0? x→0− x→0+ x→0
b) Find the value of the constant a such that g(x) is continuous at x = 1.
c) Using the value of a found in part b), sketch the graph of g(x).
36. The gravitational force F of Earth on a unit mass at a distance r (r ≥ 0) from Earth’s
centre is given below. M is the mass of Earth, R is Earth’s radius and G is the gravitational
constant. Is F (r) a continuous function? Justify your answer. ( GM r if r < R R3 F (r) = GM if r ≥ R r2
37. Use the intermediate value theorem to show that the equation f (x) = x3 + x − 1 has a root on the interval (0, 1).
38. Prove that the equation 2πx2 = ex has at least two root in the interval (0, ∞). 2