Solutions Cal1 Final - Calculus 1 | Trường Đại học Quốc tế, Đại học Quốc gia Thành phố HCM

Solutions Cal1 Final - Calculus 1 | Trường Đại học Quốc tế, Đại học Quốc gia Thành phố HCM được sưu tầm và soạn thảo dưới dạng file PDF để gửi tới các bạn sinh viên cùng tham khảo, ôn tập đầy đủ kiến thức, chuẩn bị cho các buổi học thật tốt. Mời bạn đọc đón xem!

30 15 lượt tải Tải xuống
Chia sẻ Báo cáo tài liệu

Môn: Calculus 1 (MA001IU)

Trường: Trường Đại học Quốc tế, Đại học Quốc gia Thành phố Hồ Chí Minh

Thông tin:

3 trang 4 tháng trước

Tác giả:

Profile Mai Nguyệt
CALCULUS 1
Solutions for Final Examination
Question 1.
f
(x x) =
2
e
−2x
.
(a)
f
0
(x) = 2xe xe .
−2x
− 2x
2
e
−2x
= 2
−2x
(1 − x)
The increasing/decresing intervals are given in the following chart.
x 0 1
f
0
(x) - 0 + 0 -
f(x) & % &
(b) According to part (a), has local maximum and local minimum value
f f(1) = e
−2
f(0) = 0.
(c)
f
0
(x) = 2(x − x
2
)e
−2x
.. So
f
”(x x x x) = 2(1 − 2 )e
−2x
− 4(x −
2
)e
−2x
= 2(1 − 4x + 2
2
)e
−2x
,
which has the same sign as
p(x) = 1−4 +2 ( 2 1+ 2 2,x x
2
. Since p x) = 0 iff x = 1−
√
/2 < x <
√
/
and
p( 2 2x) < 0 iff 1 −
√
/2 < x < 1 +
√
/2, the f(x) is concave upward in the intervals
x <
1−
√
2/ /2 and x > 1+ 2
√
2, and concave downward in the interval 1−
√
2 1+ 2 2./2 < x <
√
/
The graph of
f has two inflection points: (1 ±
√
2 2 (3 2) )./ , /2 ±
√
e
−2∓
√
2
(d) Sketch the graph of , see Figure 1.f
Figure 1: Graph of in Question 1
f(x) = x
2
e
−2x
Question 2.
a) Let y =
1 − sin
1
x
2x
. Then
lim
x
→∞
ln y = lim
x
→∞
2x ln
1 − sin
1
x
= 2 lim
x→∞
ln
1 − sin
1
x
1/x
= 2 lim
x→∞
( cos(1 )( 1 sin(1 ))
− /x − /x
2
)/(1 − /x
−1/x
2
= 2 lim
x
→∞
−cos(1 )/x
1
− sin(1/x)
= −2.
Thus, lim
x→∞
y = 1 ./e
2
1
b) Let f(x) = 2x − −1 sin(x). We use Newton’s method with starting point = 1 to findx
0
the root of f (x) = 0.
x x
n+1
=
n
−
f(x
n
)
f
0
(x
n
)
= x
n
−
2x
n
− −1 sin(x
n
)
2
− cos(x
n
)
,
which gives us
x
1
= 0. .891395995328754, x , x
2
= 0.887865749400352
3
= 0 887862211574412, x
4
= 0.887862211570866
Question 3. Evaluate the integrals
a)
I
=
Z
2
1
x x
2
ln(
3
)dx =
Z
2
1
ln
xdx
3
=
x
3
ln x
2
1
−
Z
2
1
x
3
(1/x dx)
= 8 ln 2
−
Z
2
1
x x
2
dx = 8 ln 2 −
3
/3
2
1
= 8 ln 2 − 7/3 = 3. .2118
b) Set
u = ln x, dv =
1
√
x
dx. Then, du = dx/x, v = 2
√
x. Thus
Z
e
1
ln x
√
x
dx = (ln x)2
√
x
e
1
−
Z
e
1
2
√
x
x
dx
= 2
√
e −
Z
e
1
2
√
x
dx
= 2
√
e − 4
√
x
e
1
= −2
√
e + 4 = 0 7026.
Question 4.
Z
∞
1
ln x
x
2
dx =
Z
∞
1
ln
xd
−1
x
=
−ln x
x
∞
1
+
Z
∞
1
1
x
2
dx
= 0
−
1
x
∞
1
= 1.
(b)
x
+ e
−x
x
2
>
1
x
, x ≥ 1
The integral
R
∞
1
(1 )/x dx is divergent, therefore, the given integral is also divergent, by Com-
parison Test.
Question 5.
(a) The equation 2 + 1) = 0 gives intersection points− −x (x
2
−2 )(x) = (2 −x x
at x = −1, x = 2. Area:
A
=
Z
2
−1
(2
− x − (x
2
− 2 ))x dx =
Z
2
−1
(2 +
x − x
2
)dx
= (2
x + x
2
/2 − x
3
/3)
2
−
1
= 9/2
2
Figure 2: Area in Question 5, (a)
(b) y = sin( = 4. Radius: = 4x), 0 ≤ ≤x π, and y = 0 about the line x R −x, circumference
2π(4 − x), and height sin x. So,
V
=
Z
π
0
2 8
π(4 − x) sin xdx = − π cos x
π
0
+
Z
π
0
2πxd cos x
= 16
π + 2π(x cos x
π
0
−
Z
π
0
cos )xdx
= 16
π + 2π(−π − sin x
π
0
) = 16π −2π
2
= 30 5263.
3
| 1/3
| | Tải xuống

Có thể bạn quan tâm:

Preview text:

CALCULUS 1
Solutions for Final Examination Question 1. f (x) = x2e−2x. (a)
f 0(x) = 2xe−2x − 2x2e−2x = 2xe−2x(1 − x).
The increasing/decresing intervals are given in the following chart. x 0 1 f 0(x) - 0 + 0 - f (x) & % &
(b) According to part (a), f has local maximum f (1) = e−2 and local minimum value f (0) = 0.
(c) f 0(x) = 2(x − x2)e−2x.. So
f ”(x) = 2(1 − 2x)e−2x − 4(x − x2)e−2x = 2(1 − 4x + 2x2)e−2x, √ √
which has the same sign as p(x) = 1−4x+2x2. Since p(x) = 0 iff x = 1− 2/2 < x < 1+ 2/2, √ √
and p(x) < 0 iff 1 − 2/2 < x < 1 + 2/2, the f(x) is concave upward in the intervals √ √ √ √
x < 1− 2/2 and x > 1+ 2/2, and concave downward in the interval 1− 2/2 < x < 1+ 2/2. √ √ √
The graph of f has two inflection points: (1 ± 2/2, (3/2 ± 2)e−2∓ 2).
(d) Sketch the graph of f , see Figure 1.
Figure 1: Graph of f (x) = x2e−2x in Question 1
Question 2. a) Let y = 1 − sin 1 2x. Then x  1  lim ln y = lim 2x ln 1 − sin x→∞ x→∞ x ln 1 − sin 1  = 2 lim x x→∞ 1/x
(− cos(1/x)(−1/x2)/(1 − sin(1/x)) = 2 lim x→∞ −1/x2 − cos(1/x) = 2 lim = −2. x→∞ 1 − sin(1/x) Thus, lim y = 1/e2. x→∞ 1
b) Let f (x) = 2x − 1 − sin(x). We use Newton’s method with starting point x0 = 1 to find the root of f (x) = 0. f (x ) 2x − 1 − sin(x ) x − n = x − n n , n+1 = xn f n 0(x ) 2 ) n − cos(xn which gives us
x1 = 0.891395995328754, x2 = 0.887865749400352, x3 = 0.887862211574412, x4 = 0.887862211570866
Question 3. Evaluate the integrals a) Z 2 Z 2 I = x2 ln(x3)dx = ln xdx3 1 1  2 Z 2 = x3 ln x − x3(1/x)dx 1 1 Z 2 2 = 8 ln 2 −
x2dx = 8 ln 2 − x3/3 = 8 ln 2 − 7/3 = 3.2118.  1 1 √ b) Set u = ln x, dv = 1 √ dx. Then, du = dx/x, v = 2 x. Thus x √ Z e ln x √ e Z e 2 x √ dx = (ln x)2 x − dx  x 1 x 1 1 √ Z e 2 = 2 e − √ dx x 1 √ √ e √
= 2 e − 4 x = −2 e + 4 = 0.7026 1 Question 4. Z ∞ ln x Z ∞ −1 dx = ln xd x 1 x2 1 − ln x∞ Z ∞ 1 =  + dx x  1 x2 1 1 ∞ = 0 −  = 1. x 1 (b) x + e−x 1 > , x ≥ 1 x2 x
The integral R ∞(1/x)dx is divergent, therefore, the given integral is also divergent, by Com- 1 parison Test.
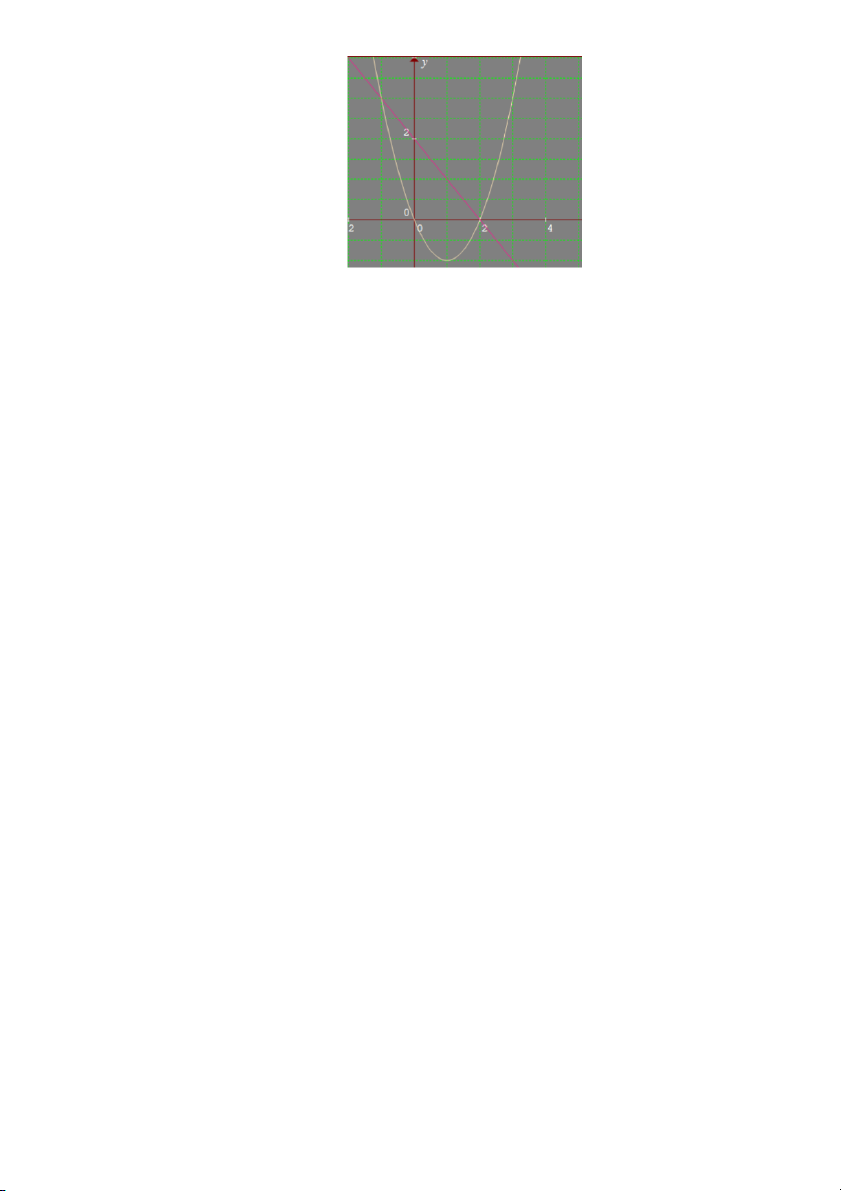
Question 5. (a) The equation 2 − x − (x2 − 2x) = (2 − x)(x + 1) = 0 gives intersection points at x = −1, x = 2. Area: Z 2 Z 2 A = (2 − x − (x2 − 2x))dx = (2 + x − x2)dx −1 −1  2 = (2x + x2/2 − x3/3) = 9/2 −1 2
Figure 2: Area in Question 5, (a)
(b) y = sin(x), 0 ≤ x ≤ π, and y = 0 about the line x = 4. Radius: R = 4−x, circumference
2π(4 − x), and height sin x. So, Z π π Z π V =
2π(4 − x) sin xdx = −8π cos x + 2πxd cos x  0 0 0 π Z π = 16π + 2π(x cos x − cos xdx) 0 0 π
= 16π + 2π(−π − sin x ) = 16π − 2π2 = 30.5263 0 3