THE INTERNATIONAL UNIVERSITY (IU)
Course: Engineering Probability and Statistics
Department of Industrial & System Engineering
FINAL EXAMINATION Duration:
ENGINEERING PROBABILITY AND STATISTICS 150 minutes Student ID: Date: Head of ISE Dept. Lecturer: January, 13, 2012 Name:
Assoc Prof. Ho Thanh Phong Assoc Prof. Ho Thanh Phong INSTRUCTIONS:
1. There are two parts in this exam. Students do Part I in the question paper, Part II in the answer paper.
2. This is an open book examination. No laptors, PDA,
3. Use of calculator is allowed; discussion and material transfer are strictly prohibited. Total pages: 7 (including this page)
PART I: Multiple Choice Questions (40%)
(Each question is assigned 2 points except the ones getting “1 point” remark) 1.
In a statistical test of hypothesis, what happens to the rejection region when the level of significance is reduced? a.
The rejection region is reduced in size.
b. The rejection region is increased in size.
c. The rejection region is unaltered.
d. The answer depends on the form of the alternative hypothesis. e. None of the above.
Using the following data for the next 3 questions:
A water filling machine that automatically controls the volume of water filled in a bottle. This machine will be
judged to be effective if the standard deviation σ of the volume of water is less than 10 ml. A sample of 10 water
bottles yields a sample standard deviation of s = 11 ml. Assume that the process has normal distribution.
2. The testing hypotheses are: (1 point) a. H0: s2 ≤ 100 H1: s2 > 100 b. H0: s2 ≥ 100 H1: s2 < 100 c. H0: s2 = 100 H1: s2 ≠ 100 d. H0: s2 ≥ 121 H1: s2 < 121
e. None of the above, specify…………………………
3. With α = 0.1, the critical point would be: a. 4.168 b. 14.684 Engineering
Probability and Statistics – Final Exam 1
THE INTERNATIONAL UNIVERSITY (IU)
Course: Engineering Probability and Statistics
Department of Industrial & System Engineering c. 16.919 d. 21.523
e. None of the above, specify…………………………
4. The test statistic value χ 2t is: a. 13.32 b. 18.56 c. 10.89 d. 15.27
e. None of the above, specify…………………………
5. Which of the following statements is correct?
a. An extremely small p-value indicates that the actual data differs markedly from that expected if the null hypothesis were true.
b. The p-value measures the probability that the hypothesis is true.
c. The p-value measures the probability of making a Type II error.
d. The larger the p-value, the stronger the evidence against the null hypothesis
e. A large p-value indicates that the data is consistent with the alternative hypothesis.
Using the following data for the next 3 questions:
An economist thinks that at least 60 percent of recently arrived immigrants who have been working in the health
profession in the United States for more than 1 year feel that they are underemployed with respect to their training.
Suppose a random sample of size 450 indicated that 294 individuals (65.3 percent) felt they were underemployed. Is
this strong enough evidence to prove that the economist is correct?
6. The testing hypotheses are: (1 point)
a. H0: p ≥ 0.6 H1: p < 0.6 b. H0: p = 0.6 H1: p ≠ 0.6 c. H0: p ≥ 0.653 H1: p < 0.653
d. H0: p < 0.6 H1: p ≥ 0.6
e. None of the above, specify…………………………
7. The test statistic value is: a. 1.324 b. -2.309 c. 2.309 d. -1.324
e. None of the above, specify………………………… Engineering
Probability and Statistics – Final Exam 2
THE INTERNATIONAL UNIVERSITY (IU)
Course: Engineering Probability and Statistics
Department of Industrial & System Engineering 8. The p-value is: a. 0.0105 b. 0.0209 c. 0.4635 d. 1.4322
e. None of the above, specify…………………………
Using the following data for next 3 questions:
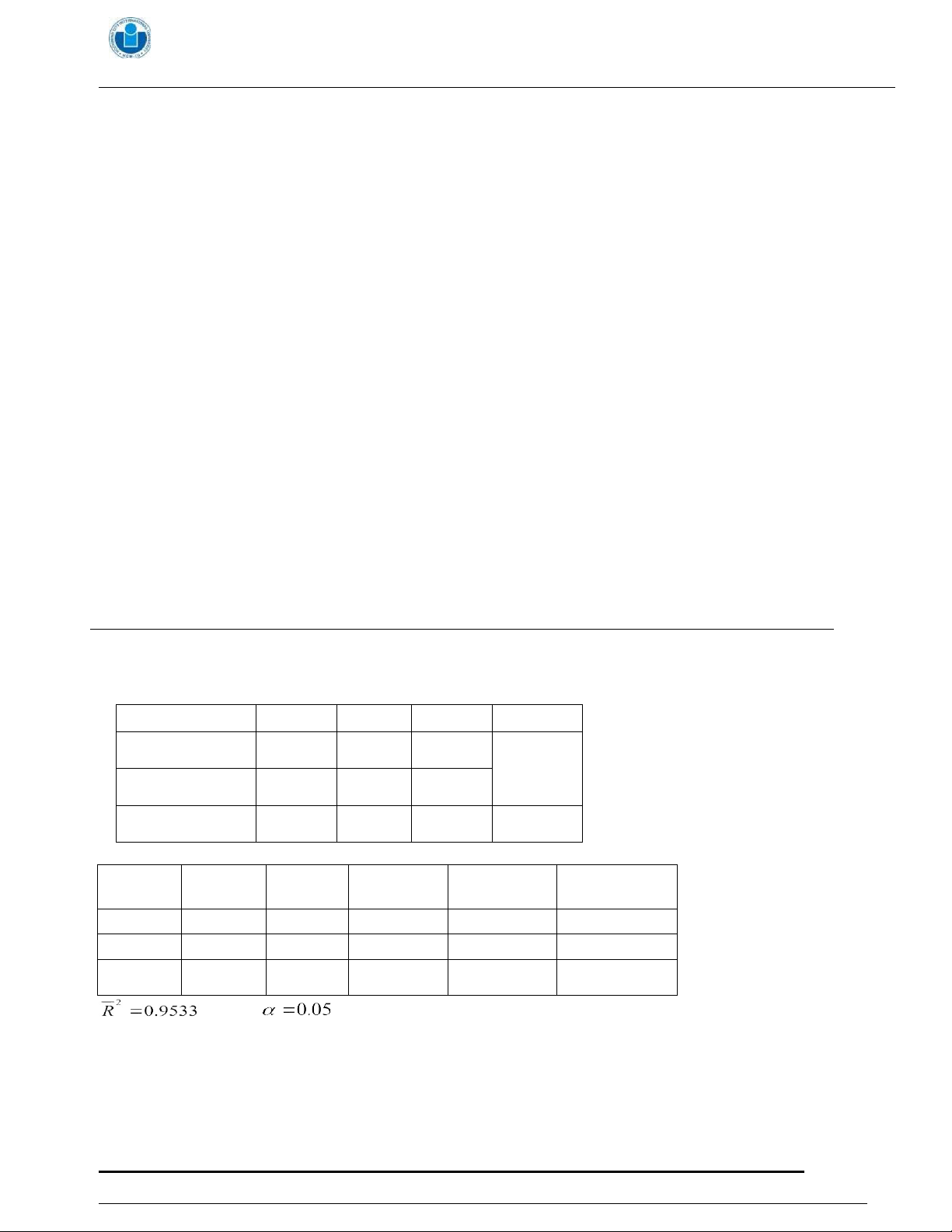
In an ANOVA testing, the number of treatments is 5, each treatment has 10 observations; complete the following ANOVA table: Degrees of Source Sum of Squares Mean Square Freedom F ratio Treatmen t Error 13156.4 Total 17581.5
9. The value of the MSTR will be: a. 4229.1 b. 17581.5 c. 292.4 d. 1106.3
e. None of the above, specify…………………………
10. The value of F ratio will be: a. 4.123 b. 7.296 c. 2.619 d. 3.784
e. None of the above, specify…………………………
11. Using α = 0.05, the null hypothesis of the above ANOVA testing is not rejected: (1 point) a. True b. False
Using the following data for the next 3 questions:
Traffic authorities claim that traffic lights are red for a time that is normal with mean 30 seconds and standard
deviation 2.4 seconds. To test this claim, a sample of 20 traffic lights was checked. If the average time of the 20
red lights observed was 31.2 seconds, can we conclude, at the 5 percent level of significance, that the authorities are incorrect?
12. The Hypotheses should be: (1 point) Engineering
Probability and Statistics – Final Exam 3
THE INTERNATIONAL UNIVERSITY (IU)
Course: Engineering Probability and Statistics
Department of Industrial & System Engineering
a. H0: µ ≥ 30 H1: µ < 30 b. H0: µ = 30 H1: µ ≠ 30
c. H0: µ ≤ 30 H1: µ > 30 d. H0: µ ≠ 30 H1: µ = 30
e. None of the above, specify…………………………….
13. The test statistic value is: a. 1.824 b. -2.042 c. 2.042 d. 2.236
e. None of the above, specify…………………………
14. Do not reject the null hypothesis with α = 5% is: (1 point) a. True b. False
15. To estimate μ, the average nicotine content of a newly marketed cigarette, 44 of these cigarettes are randomly
chosen, and their nicotine contents are determined. It is known from past experience that the standard deviation
of the nicotine content of a cigarette is equal to 0.7 milligrams. If the average nicotine finding is 1.74 milligrams,
what is a 95% confidence interval estimator of μ?? a. (1.468 ; 2.012) b. (1.533 ; 1.947) c. (1.566 ; 1.914) d. (1.588 ; 1.892)
e. None of the above, specify…………………………
Using the following data for the next 3 questions:
To learn about the feeding habits of bats, a collection of 22 bats were tagged and tracked by radio. Of these 22 bats,
12 were female and 10 were male. The distances flown (in meters) between feedings were noted for each of the 22
bats, and the following summary statistics were obtained:
Female bats: nf = 12;Sf = ; 92 Male bats: nm = 10;; Sm = 86 Assume that two populations
are normally distributed with the same variance. Test the hypothesis
that the mean distance flown between feedings is the same for the populations of male and female bats. 16. The pooled variance is: a. 7983.4 b. 4260.5 c. 7456.4 d. 6906.7 Engineering
Probability and Statistics – Final Exam 4
THE INTERNATIONAL UNIVERSITY (IU)
Course: Engineering Probability and Statistics
Department of Industrial & System Engineering
e. None of the above, specify…………………………
17. The test statistic value is: a. -1.15 b. -3.662 c. 3.662 d. 1.15
e. None of the above, specify…………………………
18. Reject the null hypothesis with α = 5% is: (1 point) a. True b. False
19. A random sample of 125 individuals working in a large city indicated that 42 are dissatisfied with their working
conditions. Construct a 90% confidence interval the percentage of all workers in that city who are dissatisfied
with their working conditions. a. (0.2272 ; 0.4448) b. (0.2532 ; 0.4188) c. (0.2665 ; 0.4055) d. (0.2819 ; 0.3901)
e. None of the above, specify…………………………
20. Since α = probability of Type I error, then 1 − α is
a. Probability of rejecting H0 when H0 is true.
b. Probability of not rejecting H0 when H0 is true.
c. Probability of not rejecting H0 when H1 is true.
d. Probability of rejecting H0 when H1 is true e. 1 − β.
Using the following data for the next 2 questions:
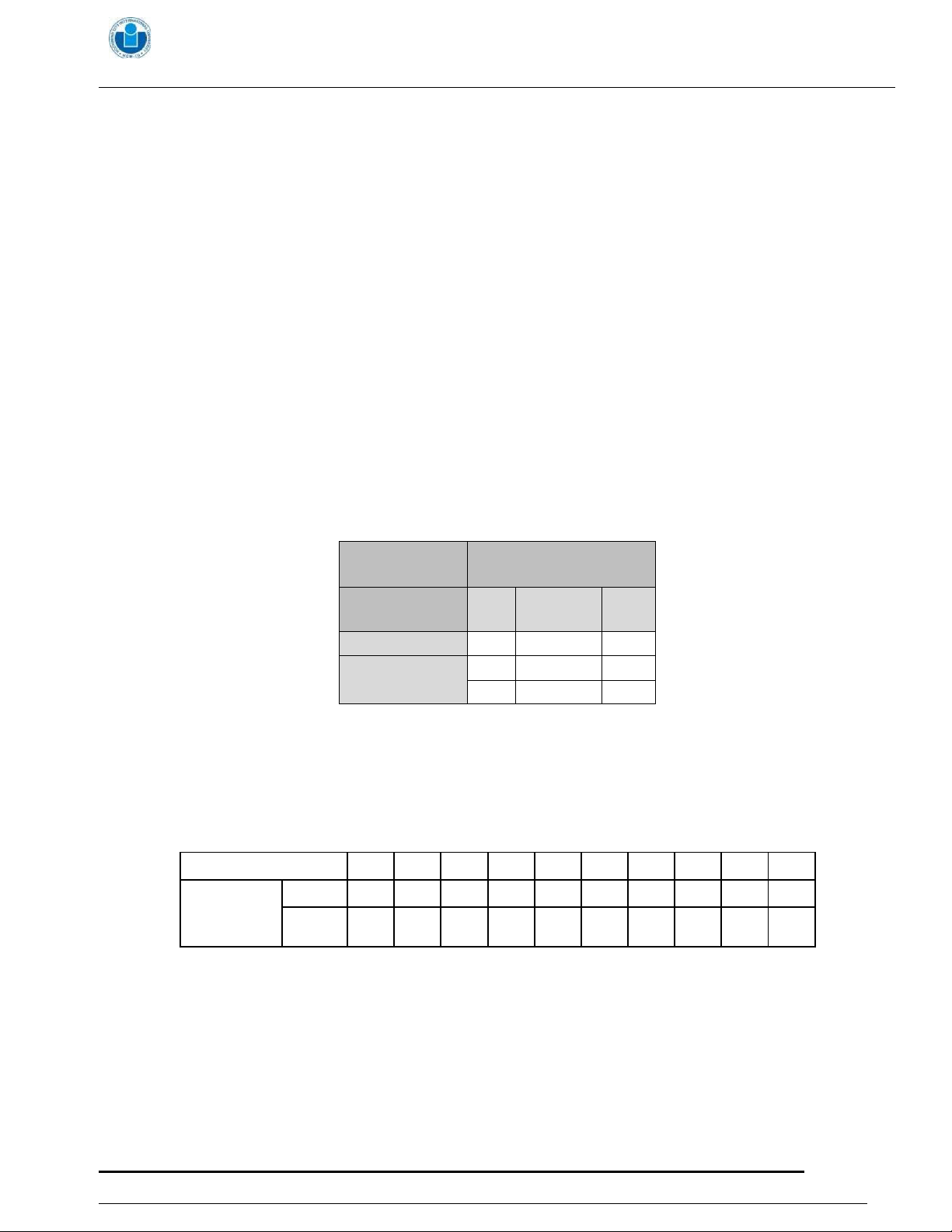
A random sample of 100 student absences yielded the following data on the days of the week on which the absences occurred: Day
Monday Tuesday Wednesday Thursday Friday Frequency 27 19 13 15 26
Conduct the Chi-square test to check the same absences of students in all days.
21. What is the test statistics? a. 8.5 b. 9.5 c. 8.0 d. 9.0 Engineering
Probability and Statistics – Final Exam 5
THE INTERNATIONAL UNIVERSITY (IU)
Course: Engineering Probability and Statistics
Department of Industrial & System Engineering
e. None of the above, specify…………………………
22. What is the critical value? a. 8.523 b. 11.143 c. 9.488 d. 11.071
e. None of the above, specify…………………………
23. From past experience it is known that the weights of salmon grown at a commercial hatchery are normal with a
standard deviation that is 0.3 pounds. If we want to be 90% certain that our estimate of the mean weight of a
salmon is correct to within ±0.1 pounds, how large a sample is needed? a. 20 b. 25 c. 30 d. 35
e. None of the above, specify………………………… PART II (60%) Question 1: (10 pts)
An article in Psychology and Marketing describes four variables that have been found to impact the effectiveness of
commercials for high-performance automobiles: sincerity, excitement, ruggedness, and sophistication. Suppose that
the random sample of size 13 leads to the outcome below: ANOVA Table Source SS df MS F Regn. Error Total 1999.2 Coefficient Table Excitemen Intercept Sincerity Ruggedness t Sophistication b -80.018 0.184 1.309 2.938 -0.330 s(b) 24.104 0.231 0.314 1.129 0.276 t . Given
a. Fill in the tables above. Is there a regression relation here between commercial effectiveness and any of the
independent variables? Explain.
b. Write out the regression equation. Which independent variables should be removed from the equation? Engineering
Probability and Statistics – Final Exam 6
THE INTERNATIONAL UNIVERSITY (IU)
Course: Engineering Probability and Statistics
Department of Industrial & System Engineering Question 2: (10 pts)
A random sample of 220 female and 210 male coffee drinkers were questioned. The result was that 71 of the women
and 58 of the men indicated a preference for decaffeinated coffee. Do these data establish, at α = 0.05, that the
proportion of female coffee drinkers who prefer decaffeinated coffee differs from the corresponding proportion for men? What is the p-value? Question 3: (10 pts)
A company supplies plastic sheets for industrial use. A new type of plastic has been produced, and the company would
like to prove to an independent assessor that the average stress resistance of this new product is greater than 30.0,
where stress resistance is measured in pounds per square inch necessary to crack the sheet. A random sample of size
12 yielded the following values of stress resistance: 32.1 29.8 32.2 29.4 28.8 31.6 28.8 29.4 33.5 27.6 33.9 31.4
a. Conduct the test for this company with α = 5%.
b. Construct the 90% confident interval for the above data. Question 4: (10 pts)
A random sample of 160 patients at a health maintenance organization yielded the following information about their
smoking status and blood cholesterol counts: Blood cholesterol count Smoking
Low Moderate High status Heavy 6 14 24 Light 12 23 15 Nonsmoker 23 32 11
Conduct the test to check the independence between blood cholesterol count and smoking status. Using α = 5%. Question 5: (10 pts)
An industrial safety program was recently instituted in the computer chip industry. The average weekly loss
(averaged over 1 month) in man-hours due to accidents in 10 similar plants both before and after the program are as follows: Plant 1 2 3 4 5 6 7 8 9 10
ManHours Before 30.5 18.5 24.5 32 16 15 23.5 25.5 28 18 Loss After 23 21 22 28.5 14.5 15.5 24.5 21 23.5 16.5
Conduct the pairwise comparison to determine, at the 5% level of significance, whether the safety program has been proven to be effective. Question 6: (10 pts)
A mechanical factory wants to prove the efficiency of new method in their production system by comparing the
variance of the product dimensions between the new method and the old one. The method which has the smaller
variance is the better. They collect two random samples corresponding with two methods as following: Engineering
Probability and Statistics – Final Exam 7
THE INTERNATIONAL UNIVERSITY (IU)
Course: Engineering Probability and Statistics
Department of Industrial & System Engineering
Old method: nold = 31, sold = 12 ;
New method: nnew = 41, snew = 9 Assume that two
populations follow Normal distribution. Given α = 5%.
Conduct the test for this factory. What is your conclusion? GOOD LUCKS! Engineering
Probability and Statistics – Final Exam 8