Silde bài giảng chương 2 môn toán cao cấp ( Tiếng anh chất lượng cao )
• N= number of periods, which is equal to the number of years multiplied by the number of periods per year. Ex: PV= Present value; CN = $1 million r = (12% divided by 4 periods per year) N = 80 (4 periods multiplied by 20 years) Substitute the values of CN, r, and N in the formula, and compute the present value.Tài liệu giúp bạn tham khảo, ôn tập và đạt kết quả cao. Mời bạn đọc đón xem !
Môn: Toán Cao Cấp (KTHCM)
Trường: Đại học Kinh tế Thành phố Hồ Chí Minh
Thông tin:
Tác giả:
Tài liệu liên quan:
Preview text:
lOMoAR cPSD| 46578282 7/29/2020 CHAPTER 2: EXPONENTS AND LOGARITHMS 1 lOMoAR cPSD| 46578282 7/29/2020 7/29/2020
B03013 – Chapter 2: Exponents and 2 2 lOMoAR cPSD| 46578282 7/29/2020 Logarithms
PREPARED BY: FINANCE DEPARTMENT COURSE CODE: B03013 7/29/2020
B02005 – Chapter 0: Introduction 1 LEARNING OBJECTIVES
Introduce the present value.
Define the form of the annuities.
Identify and explain the optimal holding time.
Introduce the logarithmic derivative. 3 lOMoAR cPSD| 46578282 7/29/2020 7/29/2020
B03013 – Chapter 2: Exponents and 4 4 lOMoAR cPSD| 46578282 7/29/2020 Logarithms CONTENT • 1 Present value • 2 Annuities • 3 Optimal holding time • 4
Logarithmic Derivative. 7/29/2020
B03013 – Chapter 2: Exponents and 3 Logarithms CONCEPTS
• Exponent and exponential function.
• Logarithm and logarithmic function Exponents
• Exponent: a number must be multiplied by itself
a specified number of times. • f(x)=ax
• a: the base; x: the exponent.
• Example: 25=2x2x2x2x2=32
• Mathematical notation: “Two to the fifth power is 32”. 5 lOMoAR cPSD| 46578282 7/29/2020 • Law of exponents 6 lOMoAR cPSD| 46578282 7/29/2020 7/29/2020
B03013 – Chapter 2: Exponents and 5 Logarithms Application
• To calculate the present value,
• To calculate the future value • To evaluate the stock Logarithm
• To solve mathematical expressions that include exponents. • Ex: 10x=100
• Could be iterated as “To what power must the
number 10 be raised to equal 100?”. 7/29/2020
B03013 – Chapter 2: Exponents 7 and Logarithms Logarithm • But in logarith ms: 7/29/2020
B03013 – Chapter 2: Exponents and 6 Logarithms 7 lOMoAR cPSD| 46578282 7/29/2020
+ 1st : to solve t he question as a logarithm.
+ Then: solve f or x using a logarithm table.
• The relationsh ip 10x=100 can be expressed
using logarith notation: x=log10100=2
• => « log base 10 of 100 is 2 ». 7/29/2020
B03013 – Chapter 2: Exponents 8 and Logarithms Logarithm
• Logarithmic derivative:
• The derivative of the logarithmic function y =lnx
• Derivative of y = ln u (where u is a function of x) 8 lOMoAR cPSD| 46578282 7/29/2020 7/29/2020
B03013 – Chapter 2: Exponents and 9 Logarithms Logarithm • Example 4 Y= 𝑥22−1 𝑥 +1 To compute the derivative 7/29/2020
B03013 – Chapter 2: Exponents and 10 Logarithms 9 lOMoAR cPSD| 46578282 7/29/2020 Applications
• Present value: the amount you would have to invest today.
• To solve for the present value, would use the
formula for the present value of an investment with compound interest: • PV=CN x (1+r)-N • PV: Present value
• CN: Cash flow at time period N
• r= periodic rate of interest, which is calculated as: 7/29/2020
B03013 – Chapter 2: Exponents and 11 Logarithms Applications
• N= number of periods, which is equal to the
number of years multiplied by the number of periods per year.
• Ex: PV= Present value; CN = $1 million r = (12%
divided by 4 periods per year) N = 80 (4 periods multiplied by 20 years)
• Substitute the values of CN, r, and N in the formula,
and compute the present value. 7/29/2020
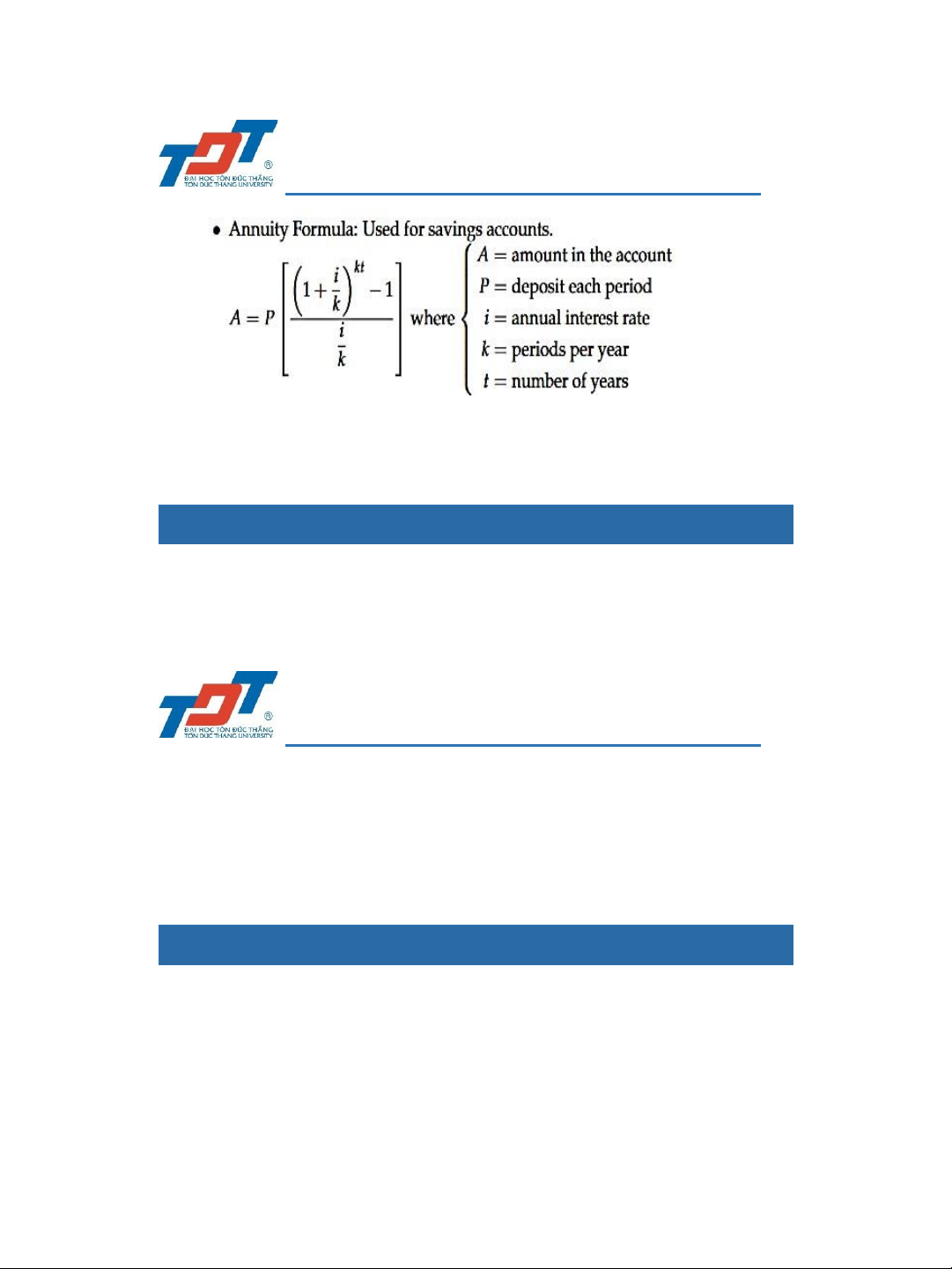
B03013 – Chapter 2: Exponents 12 and Logarithms • Annuities: 10 lOMoAR cPSD| 46578282 7/29/2020 Applications 7/29/2020
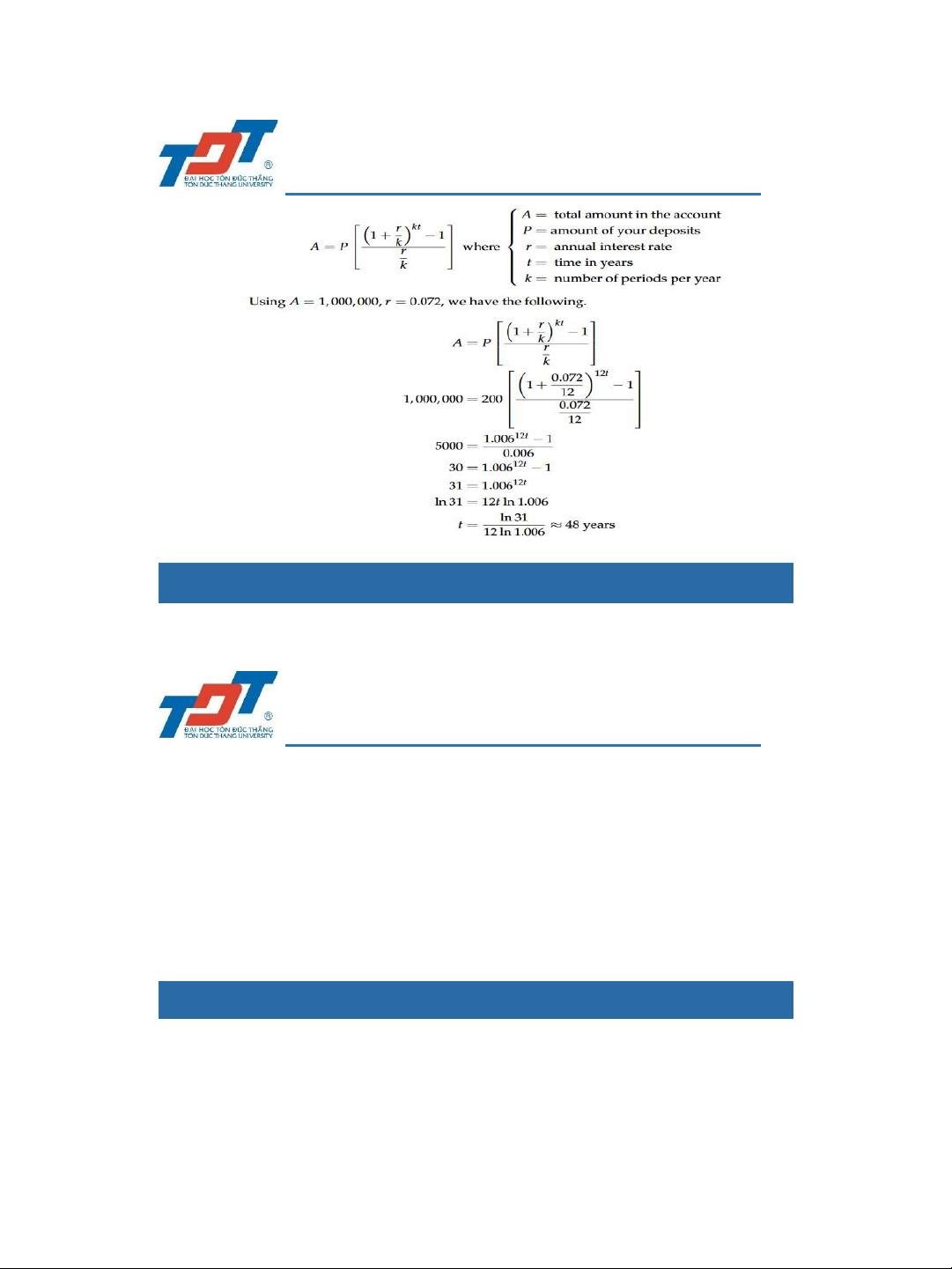
B03013 – Chapter 2: Exponents and 13 Logarithms Applications Example:
You have started your first job and decide to put
$200 a month into an annuity. The annuity earns
7.2% interest per year, compounded monthly.
How long (in months and years) will it take for the
account to be worth $1,000,000? 7/29/2020
B03007 – Chapter 2: Exponents and 14 Logarithms 11 lOMoAR cPSD| 46578282 7/29/2020 Applications 7/29/2020
B03007 – Chapter 2: Exponents and 15 Logarithms Applications
• Optimal holding time: a decision to hold an asset in a certain period.
• There are many assets: appreciate/depreciate
over time- vintage wine, real estate, forestry
plantation and mining to name a few.
• Compare an investment to others by the present value. 7/29/2020
B03007 – Chapter 2: Exponents and 16 Logarithms
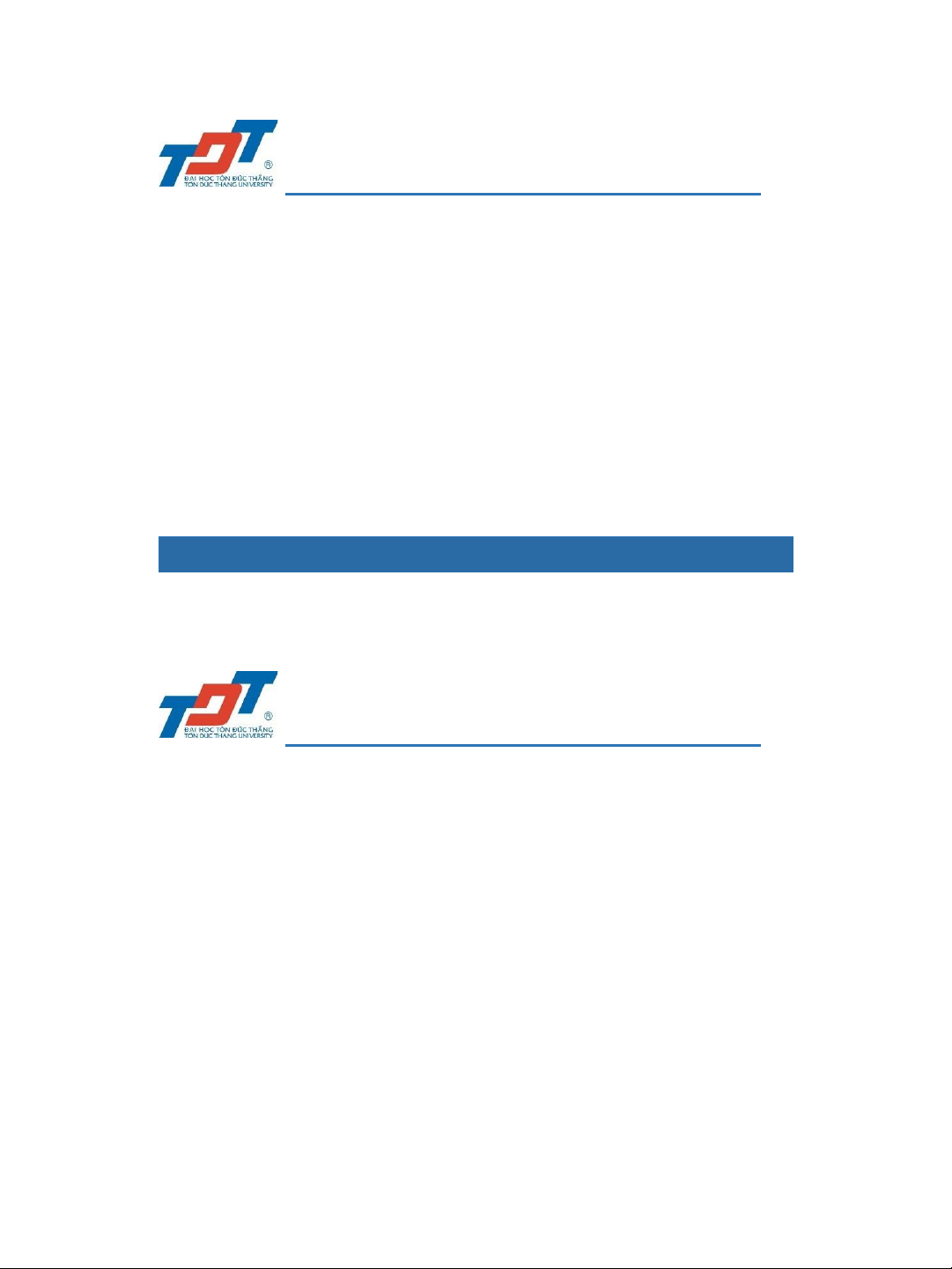
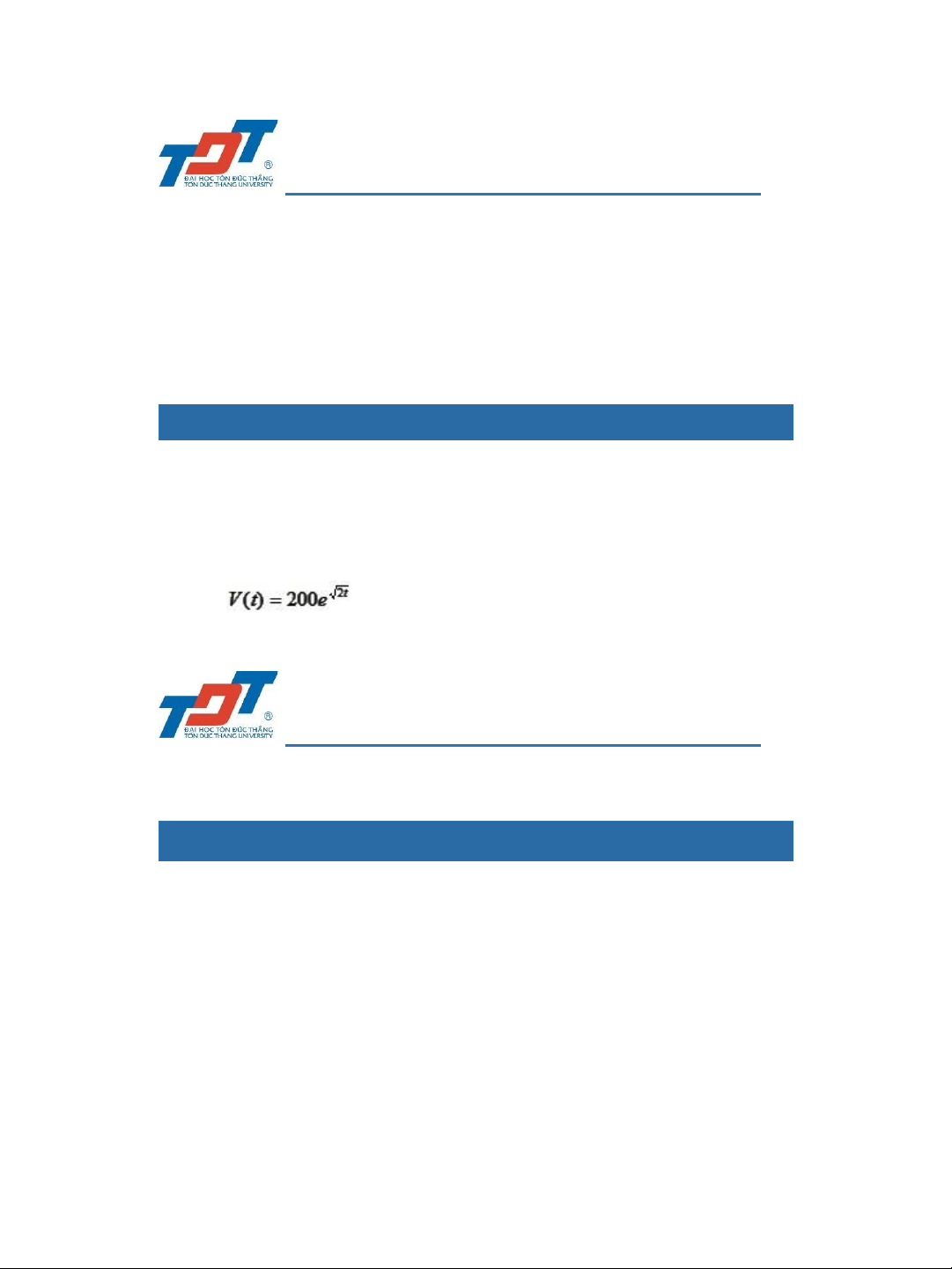
Example 1: You own estate the market value of
which t years from now is given by the function
10.000𝑒√𝑡. Assuming the interest rate for the
foreseeable future will remain at 6 percent, the 12 lOMoAR cPSD| 46578282 7/29/2020 Applications
optimal selling time is given by maximizing the present value. 7/29/2020
B03007 – Chapter 2: Exponents and 17 Logarithms Applications
• Example 2: Suppose your family owns a rare
book whose value t years from now will be
dollars. If the prevailing interest rate
remains constant at 6% per year compounded
continuously, when will it be most advantageous
for your family to sell the book and invest the proceeds? 7/29/2020
B03007 – Chapter 2: Exponents and 18 Logarithms
• Example 3: You have 500 USD, you sent it in
your bank account. It the interest rate is 8% per
year. How much your account will increase to after 5 years? 13 lOMoAR cPSD| 46578282 7/29/2020 Applications 7/29/2020
B03007 – Chapter 2: Exponents and 19 Logarithms Exercise
1. At 10 percent annual interest rate, which of the
following has the largest present value a) $215 two years from now
b) $100 after each of the next two years, or
c) $100 now and $95 two years from now
2. Assuming a 10 percent interest rate
compounded continuously, what is the present
value of an annuity that pay $500 a year a) For next five years b) Forever? 7/29/2020
B03007 – Chapter 2: Exponents and 20 Logarithms 14 lOMoAR cPSD| 46578282 7/29/2020 Exercise
3) Suppose you own a rare book whose value at
time t years from now will be B(t) = 2√𝑡 dollars.
Assuming a constant interest rate of 5%, when is
the best time to sell the book and invest the proceeds 7/29/2020
B03007 – Chapter 2: Exponents and 21 Logarithms Summary
• Reading the law of exponents and logarithmic.
• Applying the forms of those functions in
economics (interest compounding, optimal holding time…).
• Your discussions: how can we calculate some macro-factors in Vietnam. 7/29/2020
B03007 – Chapter 2: Exponents and 22 Logarithms 15